Overpressure calculation model of sphere charge blastingwith moving velocity
-
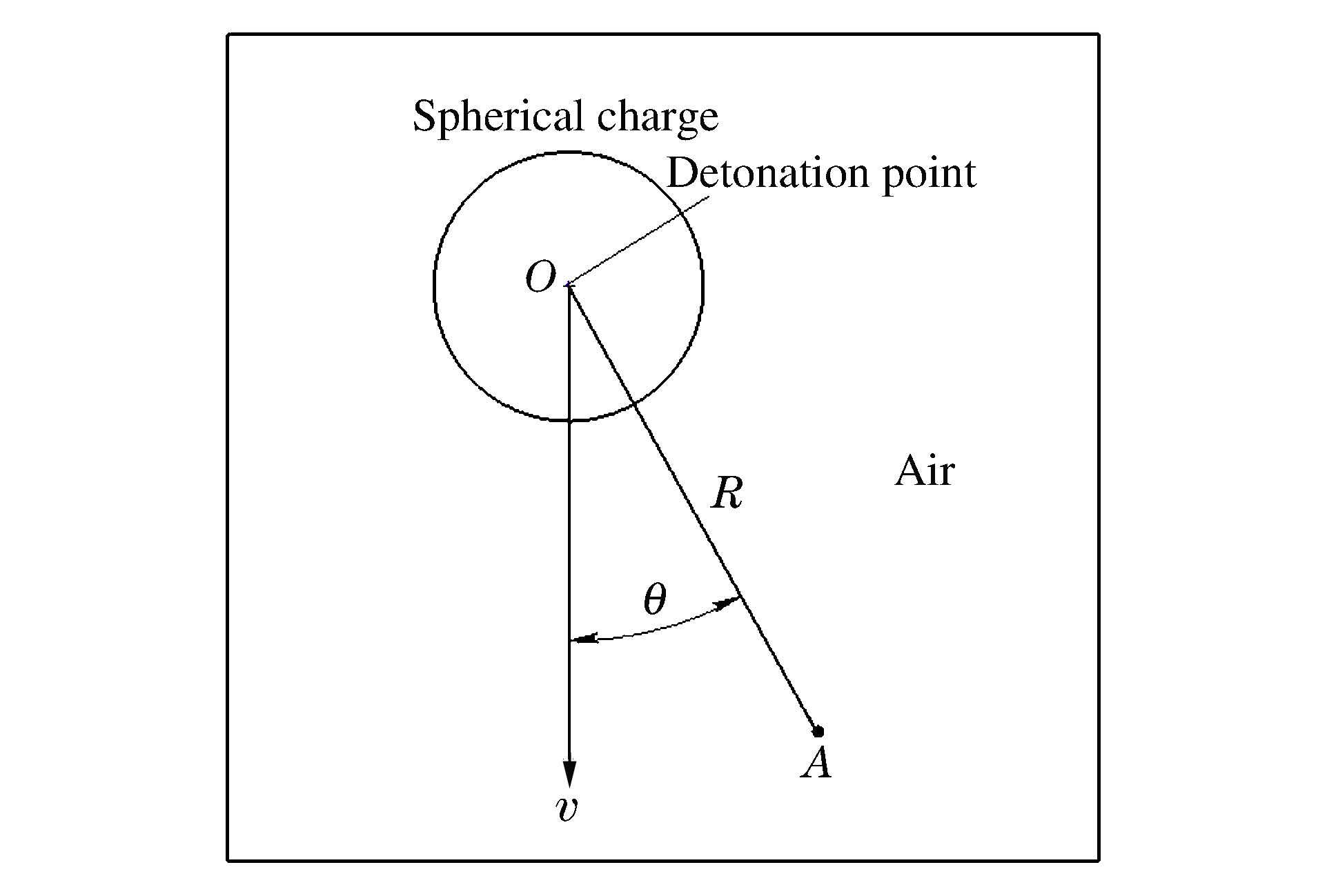
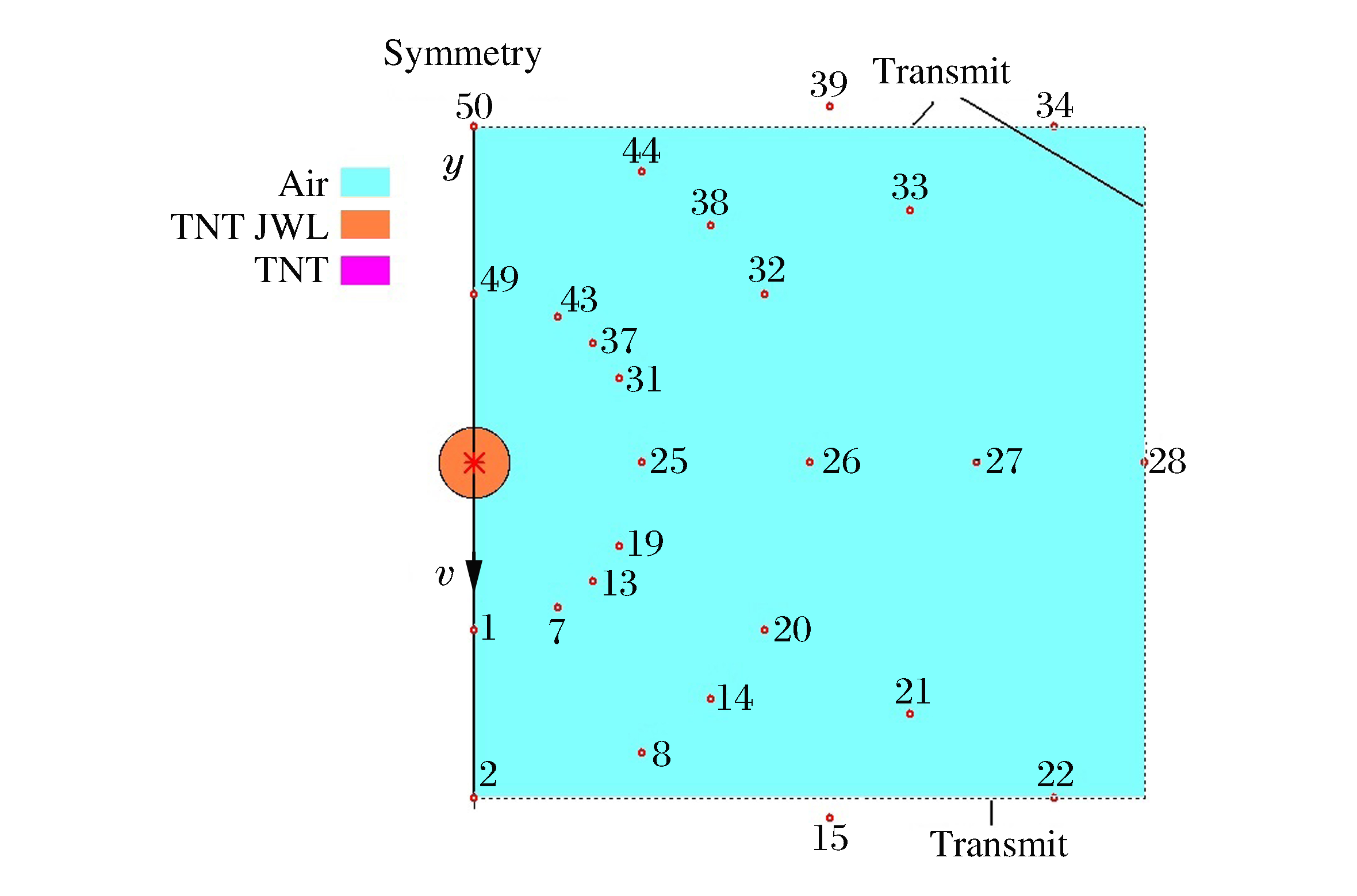
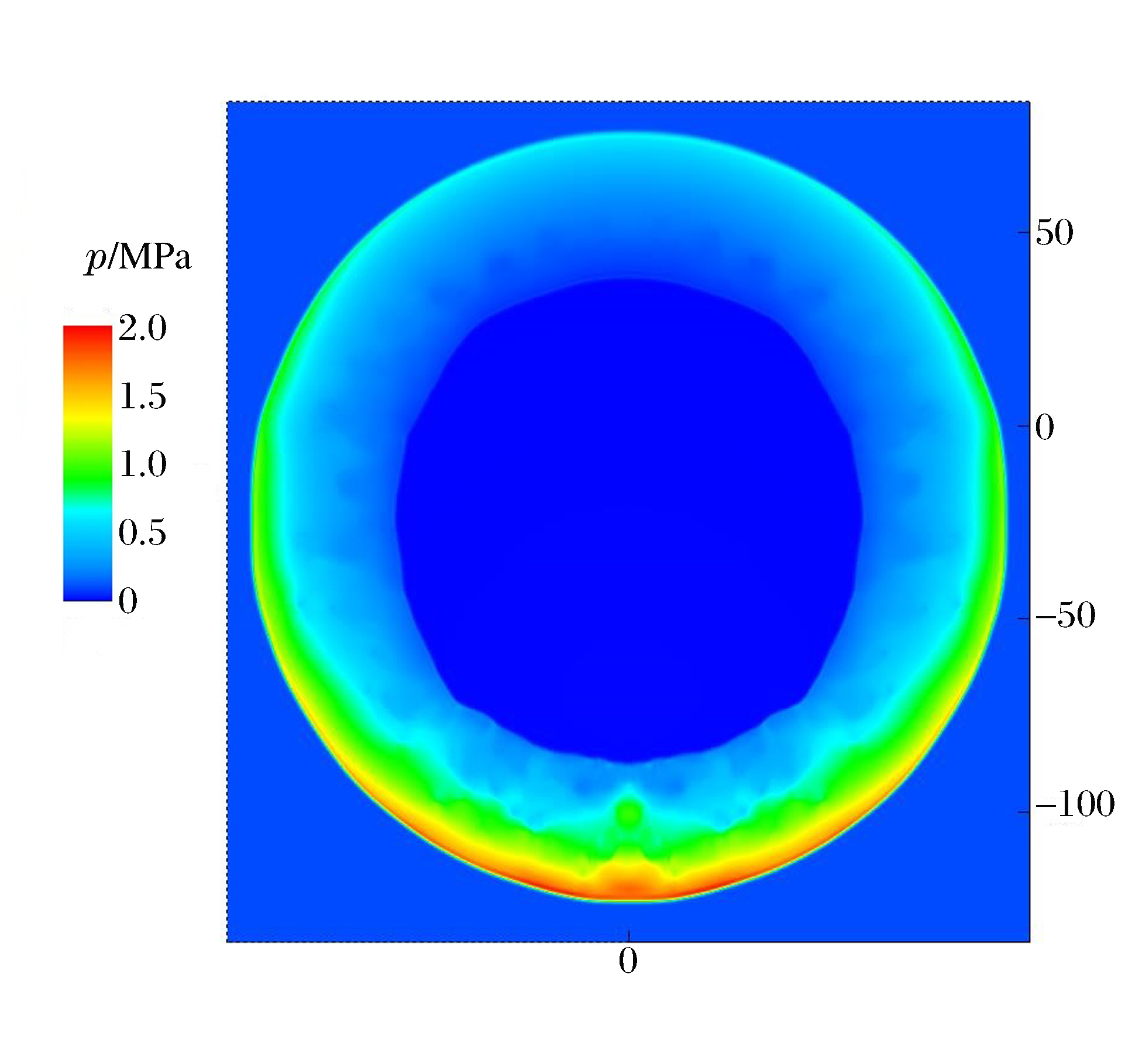
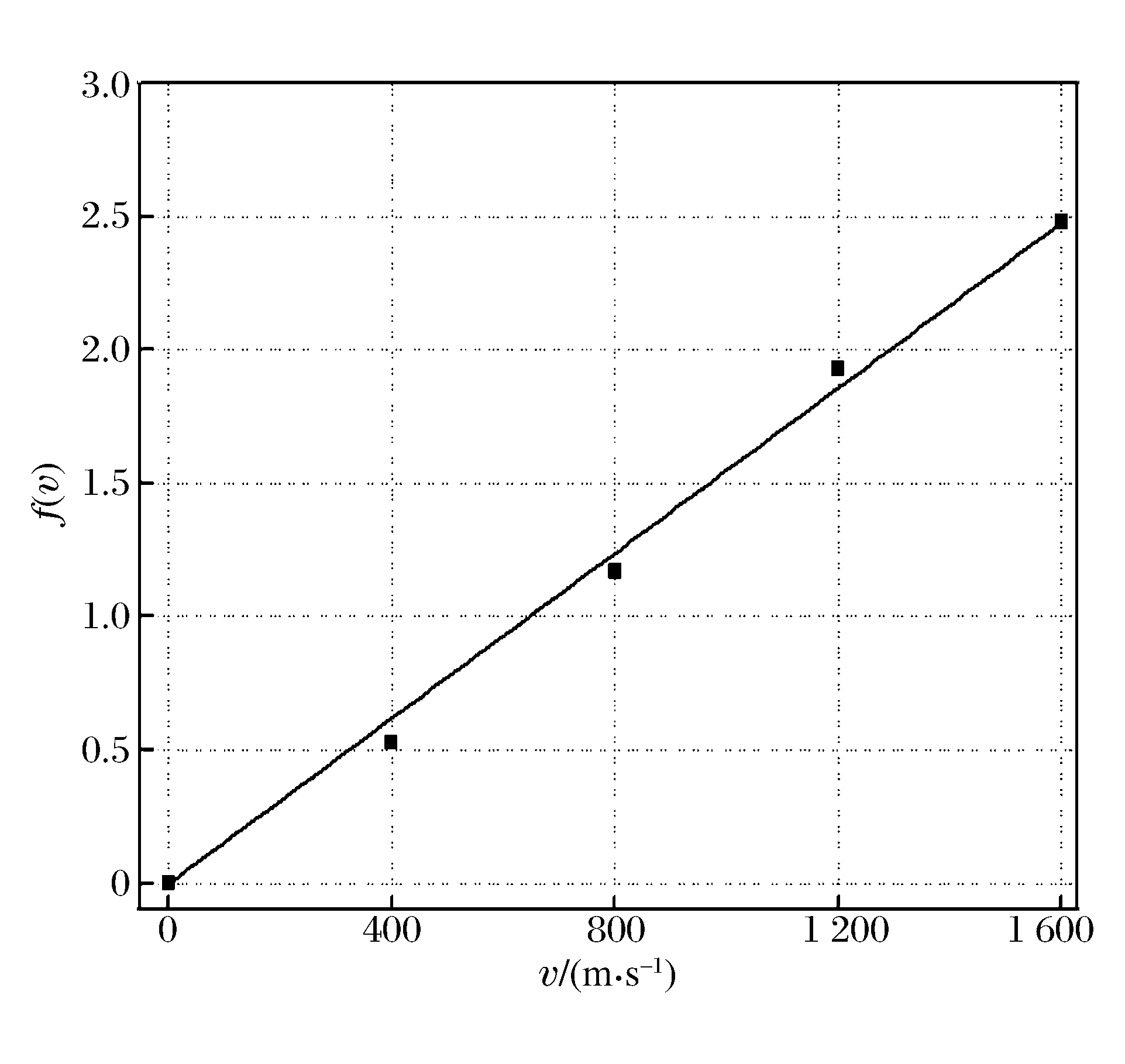
摘要: 为获得球形装药动态爆炸冲击波超压场计算模型，对静态爆炸冲击波超压Baker计算公式加入修正因子进行修正，并建立了构造包含装药运动速度、对比距离和方位角的修正因子函数的方法。为获得修正因子的函数表达式，采用高精度显式欧拉流体动力学软件SPEED针对具有典型运动速度的球形装药空中爆炸过程进行了数值模拟，得到了沿装药不同对比距离和方位角处的动态爆炸冲击波超压峰值。在对数值模拟结果处理的基础上，经过数据拟合获得了动态爆炸冲击波超压场计算模型。校验结果表明，该模型能较准确描述动态爆炸冲击波超压分布，具有普适性。Abstract: In order to establish a calculation model for the dynamic blast overpressure field, a correction factor was introduced into the Baker formula, a model for calculating the peak overpressure only in a static blast. The method to obtain the correction factor containing the moving velocity, the azimuth and the scaled distance was established. For getting the function of the correction factor, spherical charge models with the typical moving velocity were established using the shock physical explicit Eulerian dynamic (SPEED) to simulate the dynamic blast process in the air. The peak overpressure in the typical azimuth and scaled distance was obtained. Based on the numerical results, a new calculation model was constructed using the data fitted. The result of the numerical simulation indicates that the corrected formula is a universal calculation model, capable of predicting the peak overpressure in dynamic blast.
-
Key words:
- shockwave overpressure /
- dynamic blast /
- moving velocity /
- overpressure calculation model
-
图 3 v=1 200 m/s的装药爆炸后500 μs时的压力云图
Figure 3. Overpressure contour of initiated chargewith v=1 200 m/s in 500 μs
图 5 不同v时的f(Z)曲线和平均值及拟合曲线
Figure 5. Calculated curve of f(Z) in different v and their average value and fitted curve
图 6 不同v和Z时的f(θ)曲线和平均值及拟合曲线
Figure 6. Curve of f(θ) in different v and Z, and their average value and fitted curve
-
[1] 张光莹, 周旭, 黄咏政, 等.动爆冲击波特性分析方法研究[C]//第四届全国计算爆炸力学会议论文集.2008: 282-287. http://www.wanfangdata.com.cn/details/detail.do?_type=conference&id=7079475 [2] Henrych J, Abrahamson G R. The dynamics of explosion and its use[M]. New York: Elsevier Scientific Pub Co, 1979:218. [3] Baker W E. Explosions in air[M]. Austin: University of Texas Press, 1974:6-10. [4] Sadovskyi M A. Mechanical action of air shock waves of explosion, based on experimental data[M]. Moscow: Izd Akad Nauk SSSR, 1952:1-2. [5] 北京工业学院八系.爆炸及其作用[M].北京:工业出版社, 1978. [6] Izadifard R A, Foroutan M. Blastwave parameters assessment at different altitude using numerical simulation[J]. Turkish Journal of Engineering & Enviromental Sciences, 2010, 34(1):25-41. http://d.old.wanfangdata.com.cn/NSTLQK/NSTL_QKJJ0216335761/ [7] NUMERICS GmbH. SPPED user's Manual[Z]. 2012. [8] Lee E, Finger M, Collins W. JWL equation of state coeffients for high explosives: UCID-16189[R]. 1973. 期刊类型引用(15)
1. 王振宁，尹建平，李旭东，伊建亚，张渝. 有限厚钢筋混凝土参数对破片侵彻性能的影响. 兵器装备工程学报. 2023(05): 180-185 . 
百度学术2. 朱擎，李述涛，陈叶青. 配筋对超高性能混凝土抗侵彻性能的影响. 工程力学. 2023(S1): 62-73+91 . 百度学术3. 黄成龙，陈叶青，李述涛，张生，王振清. 弹着点对钢筋混凝土侵彻深度的影响. 应用数学和力学. 2023(09): 1097-1111 . 百度学术4. 朱灿伟，周刚，王可慧，龙志林，邹慧辉. 钢筋对弹体贯穿钢筋混凝土靶的剩余速度影响. 兵器装备工程学报. 2022(02): 35-43 . 百度学术5. 刘锐，王健. 钻地弹侵彻发射井上盖毁伤研究. 兵器装备工程学报. 2021(04): 68-73 . 百度学术6. 程毅，刘军，郑雨晴，张远. 钢板混凝土复合靶抗贯穿性能的理论与数值分析. 应用力学学报. 2020(04): 1441-1449+1853 . 百度学术7. 吴翰林，屈可朋，周涛. 钢筋混凝土靶侵彻阻力模型研究进展. 兵器装备工程学报. 2020(09): 19-24 . 百度学术8. 程毅，刘军，刘晓峰，金胜兵，张远. 垂直侵彻下钢筋混凝土靶抗侵彻性能的理论与数值分析. 科学技术与工程. 2019(01): 97-103 . 百度学术9. 武海军，张爽，黄风雷. 钢筋混凝土靶的侵彻与贯穿研究进展. 兵工学报. 2018(01): 182-208 . 百度学术10. 张廷磊，李海超，张艳萍. 爆点深度对桥墩侵爆毁伤的影响规律研究. 国防交通工程与技术. 2018(05): 33-36 . 百度学术11. 李海超，张廷磊，刘锡鑫，黄晨旭. 配筋率对桥墩侵爆毁伤的影响规律. 军事交通学院学报. 2018(10): 76-80 . 百度学术12. 林琛，徐建军，杨晋伟，张海良. 基于HJC模型的钢筋混凝土侵彻仿真失效准则与参数. 探测与控制学报. 2017(02): 100-105 . 百度学术13. 解加庆，赵捍东，焦军虎，罗旭，黄延平，吴建萍. 动能弹斜侵彻钢筋混凝土性能影响研究. 兵器材料科学与工程. 2014(01): 107-110 . 百度学术14. 施存程，王德荣，范新，范鹏贤，王明洋. 掘开式工程口部毁伤工程量分析. 解放军理工大学学报(自然科学版). 2013(06): 623-629 . 百度学术15. 吴昊，方秦，龚自明，赵建魁. 冲击爆炸作用对核电站安全壳毁伤效应研究的进展. 防灾减灾工程学报. 2012(03): 384-392 . 百度学术其他类型引用(22)
-
下载:
百度学术
图(7)
计量
- 文章访问数: 4466
- HTML全文浏览量: 1254
- PDF下载量: 475
- 被引次数: 37