Elastic-plastic transition behaviors of HMX crystal under ramp wave compression
-
摘要: 开展了(010)、(011)晶向HMX晶体的斜波压缩实验,获得了约15 GPa压力下的速度响应剖面。实验结果表明,HMX单晶存在明显弹塑性转变行为,且速度波形有下降趋势,这是材料的黏性效应导致,材料的弹性极限随着样品厚度增加而变化,不同晶向的材料动力学特性存在差异。结合Hobenemser-Prager黏弹塑性本构关系和三阶Birch-Murnaghan物态方程开展了HMX晶体斜波压缩物理过程的数值模拟,计算结果可以很好地描述HMX晶体的弹塑性转变这一物理过程。Abstract: The dynamics of HMX single crystals under ramp wave loading was studied experimentally and numerically. The ramp wave compression experiments of (010) and (011) crystal oriented HMX crystals within 15 GPa were carried out with the magnetic driven device CQ-4, which can provide a loading pressure with a rising time of 450−600 ns. The velocity curves of the interface between the HMX single crystal and the LiF single crystal were obtained with dual laser heterodyne velocimetry (DLHV). The experimental results show that there is an obvious elastic-plastic transition behavior in the loading section. The velocity waveforms have a downward trend in the elastic-plastic transition section, which is caused by the viscous effect of the HMX single crystal. The elastic limit of the HMX single crystal changes with the increase of the sample thickness. The Lagrange sound speed-particle velocity data and pressure-specific volume curves of (010) and (011) crystal oriented HMX crystals were obtained with the iterative Lagrange data processing method for dynamic impedance mismatch. The Lagrange sound speed-particle velocity relationships in the different crystal directions are different. The pressure-specific volume curve is close to isentropic experimental data by Sandia laboratory. The numerical simulation of the physical process of ramp wave compression of the HMX crystal was carried out with the viscoelastic plastic constitutive relation of Hobnemser-Prager and the third-order Birch-Murnaghan equation of state. The calculation results can well describe the physical process of the elastic-plastic transformation of HMX crystal.
-
Key words:
- HMX crystal /
- elastic-plastic transition /
- ramp wave compression /
- equation of state
-
炸药的力学性能参数与其反应机理和化爆安全性紧密相关[1-4]。单质高能炸药由于受到炸药大单晶生长困难的影响,不能直接加工成药柱等构件。目前,炸药力学性能均基于非均质炸药(PBX)为研究对象。研究表明,单质炸药的晶体特性对PBX的性能如感度、安定性、机械强度等有重要影响[5-12]。
HMX是目前综合性能最好的高能炸药,在武器中应用广泛。HMX 晶体因具有较多晶型以及复杂的相变问题而受到广泛的关注,它具有β、δ、α和γ等4种晶型, 其中β-HMX、δ-HMX和α-HMX 是固体,γ-HMX是液体[13-15]。 这几种晶型具有不同的稳定性和对外界刺激的敏感程度, 不同相之间可以发生相互转变。在常温常压下, 4种晶型的稳定性由强到弱依次为为β、γ、α、δ[14]。 β-HMX 是在室温下最稳定的晶型[15]。人们已开始研究HMX在动态加载条件下的非弹性行为[16]。 Menikoff等[17]和 Dick等[18]的实验研究结果表明,β-HMX单晶受平面冲击加载后呈现各向异性的弹塑性波结构。 Dick等[18]的认为对于这种脆性的分子晶体,其主要作用机制是塑性机制。Jaramillo等[19]通过分子动力学计算发现,β-HMX 的弹塑性转变机制是位错滑移运动。Sewell等[20]和Zaug等[21]也对β-HMX单晶的弹塑性行为开展了冲击加载实验研究。
冲击加载下炸药单晶温升剧烈,由于其动力学响应特性的高感度,很难获得较高压力下的实验结果。利用斜波加载实验技术[22],样品压缩过程中获得高压状态的同时依然可以保持样品材料中较低的温升,炸药不易发生化学反应。本研究利用磁驱动加载实验技术,开展了斜波加载下β-HMX两个晶向在15 GPa内的动力学响应研究,目的在于通过双光源外差测速技术(dual laser heterodyne velocimetry, DLHV)测量单晶的速度响应曲线,获得β-HMX单晶不同晶向弹塑性转变信息的同时获得炸药的压力-相对比容关系。
1. HMX单晶炸药的斜波压缩实验
HMX是一种具有各向异性力学性能的单斜晶体,本文中采用的厘米量级大块体样品由中北大学制备,样品如图1所示。
HMX晶体斜波压缩实验条件见表1,HMX单晶样品有(011)、(010)两个晶向,实验窗口为LiF单晶,加载电极为高导纯铝材料。单发实验对不同厚度样品进行斜波压缩,利用DLHV测试HMX样品/LiF窗口的界面速度。
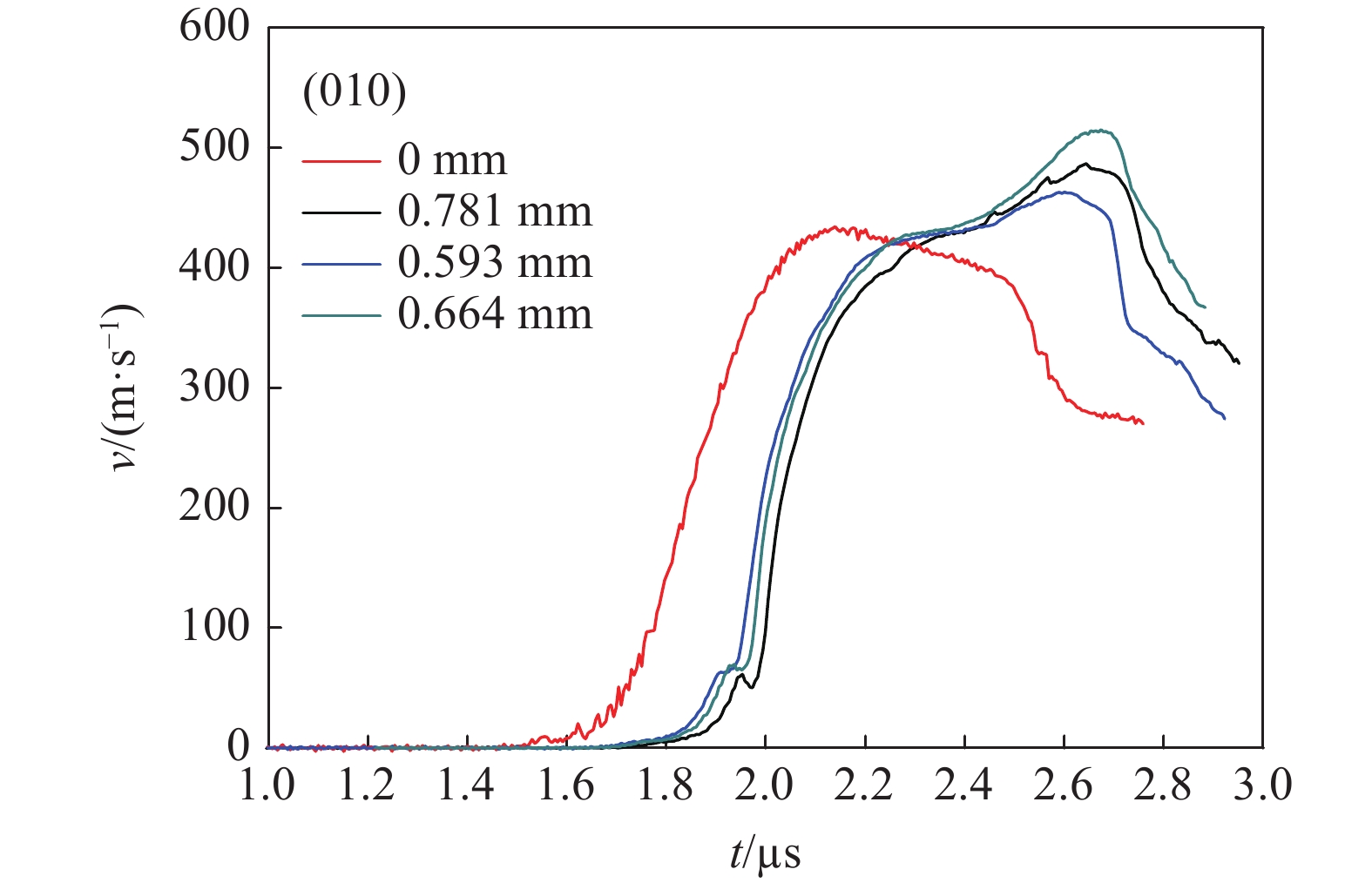
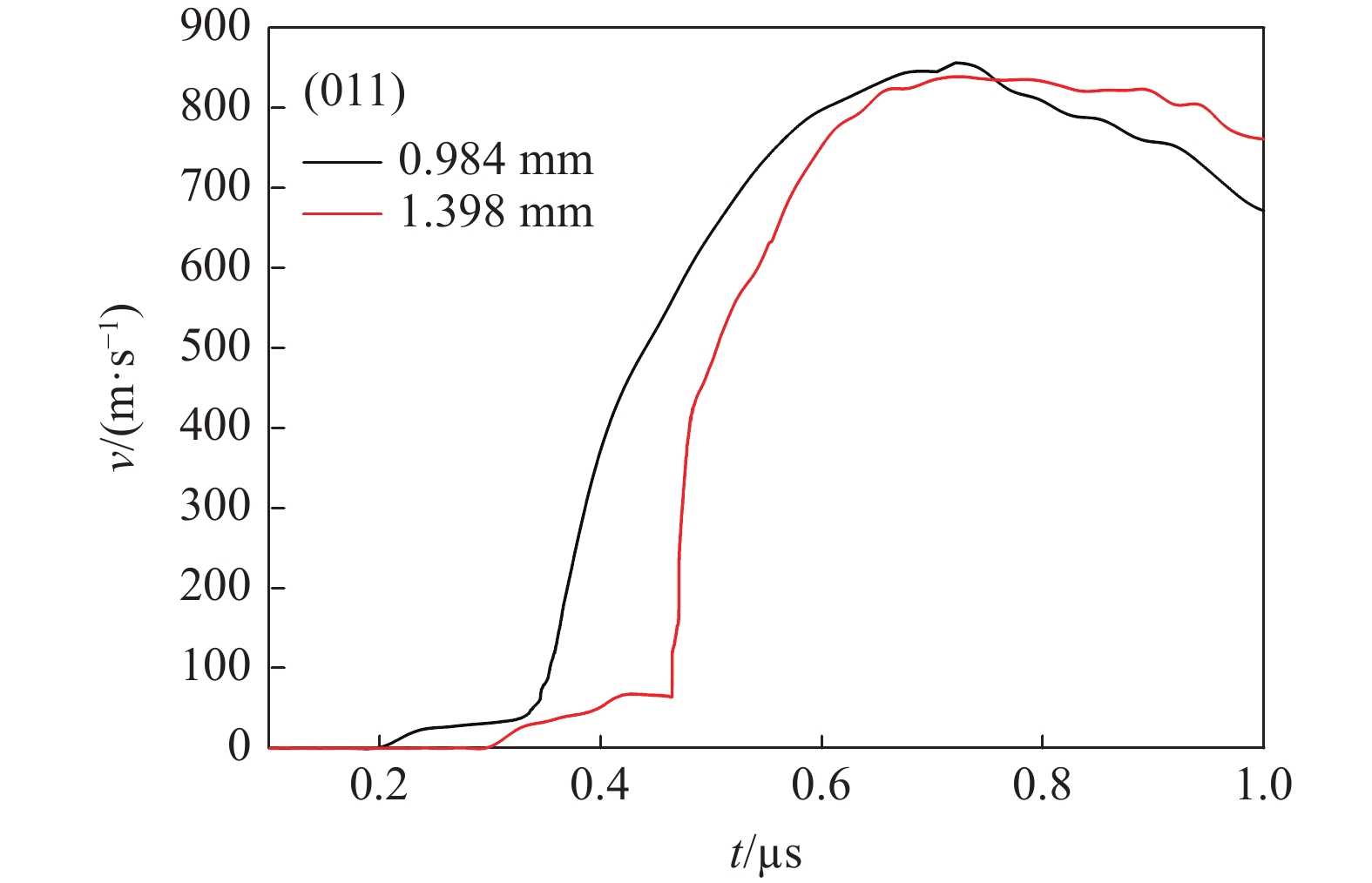
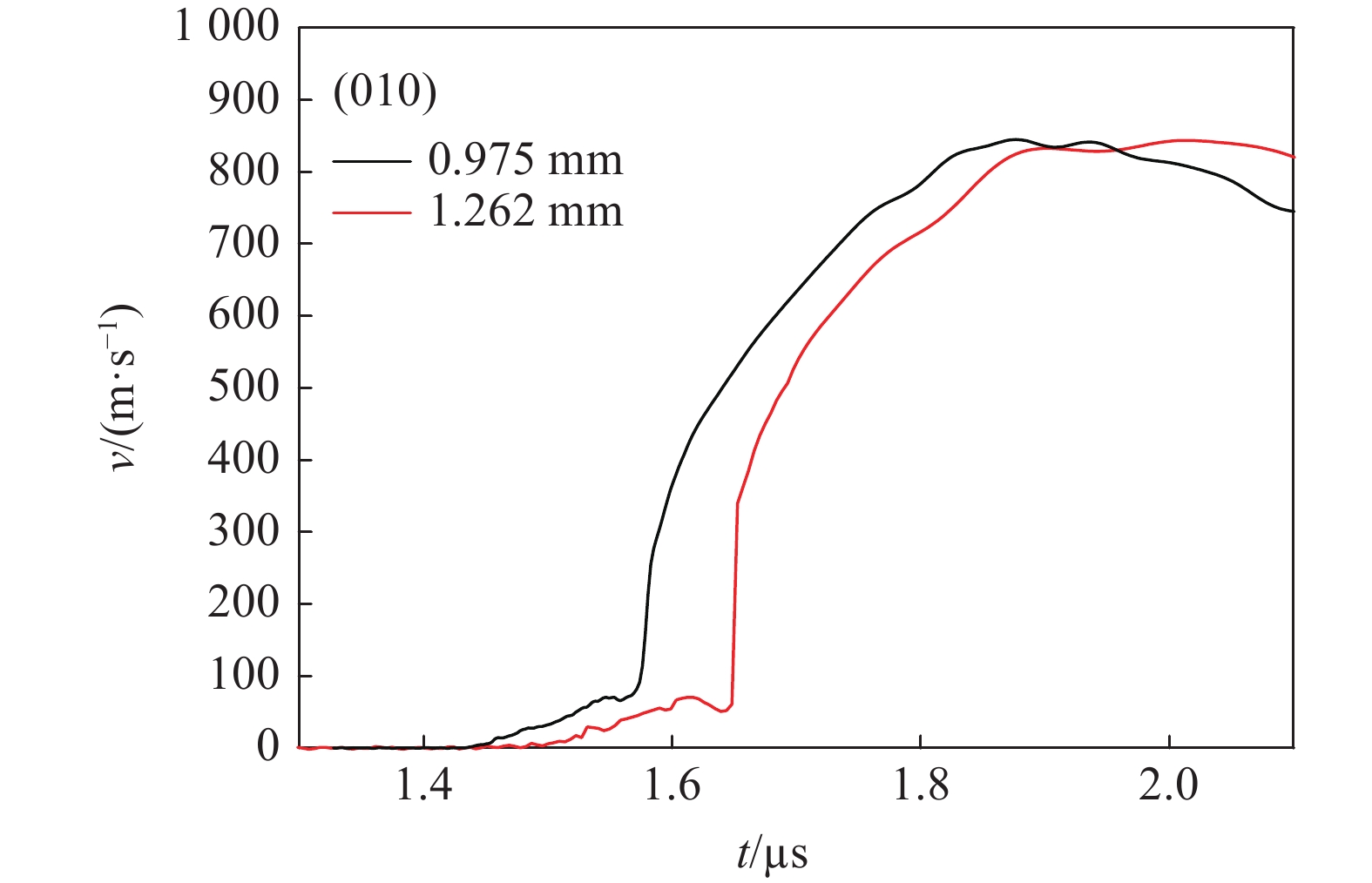
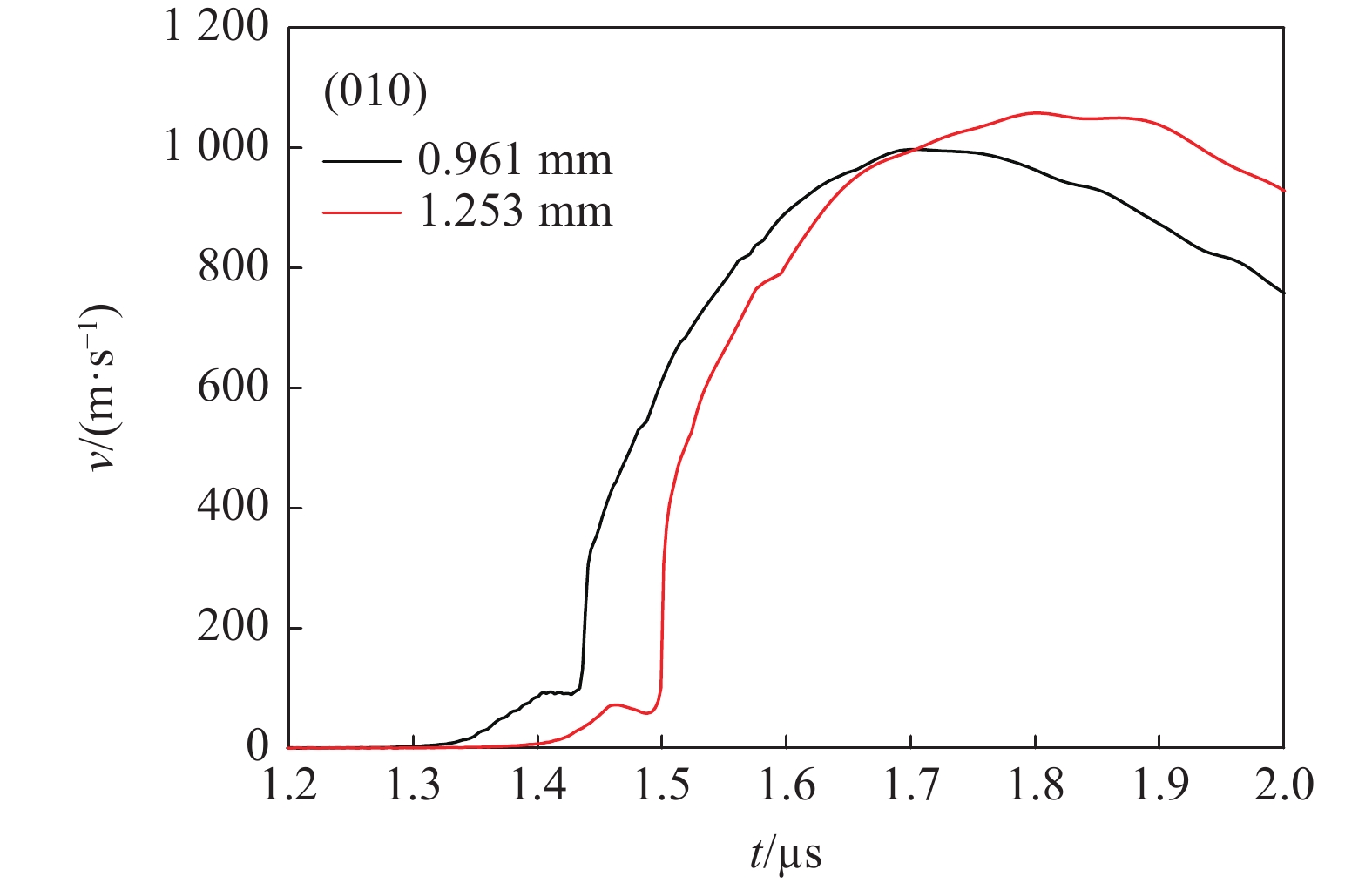
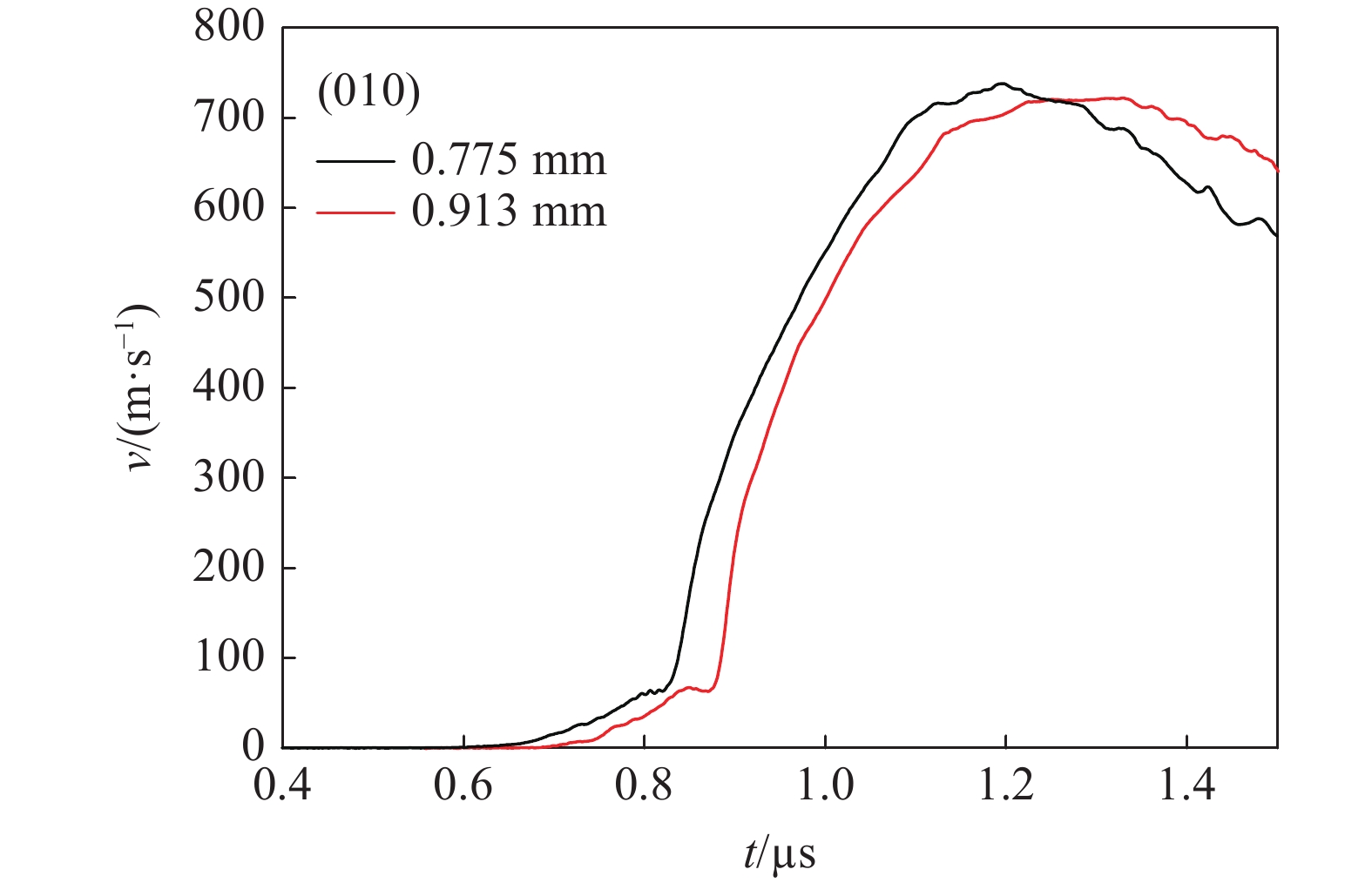
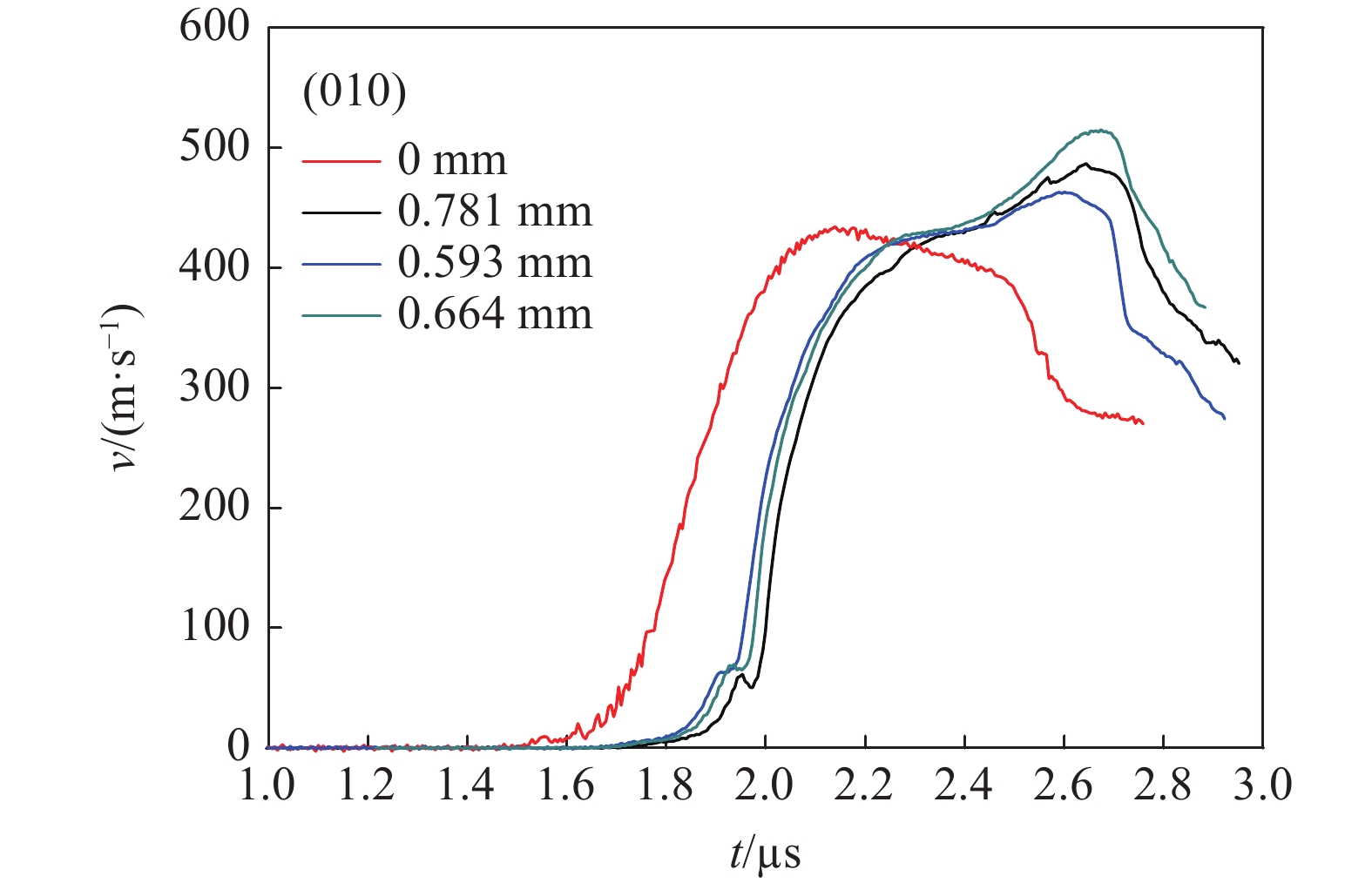
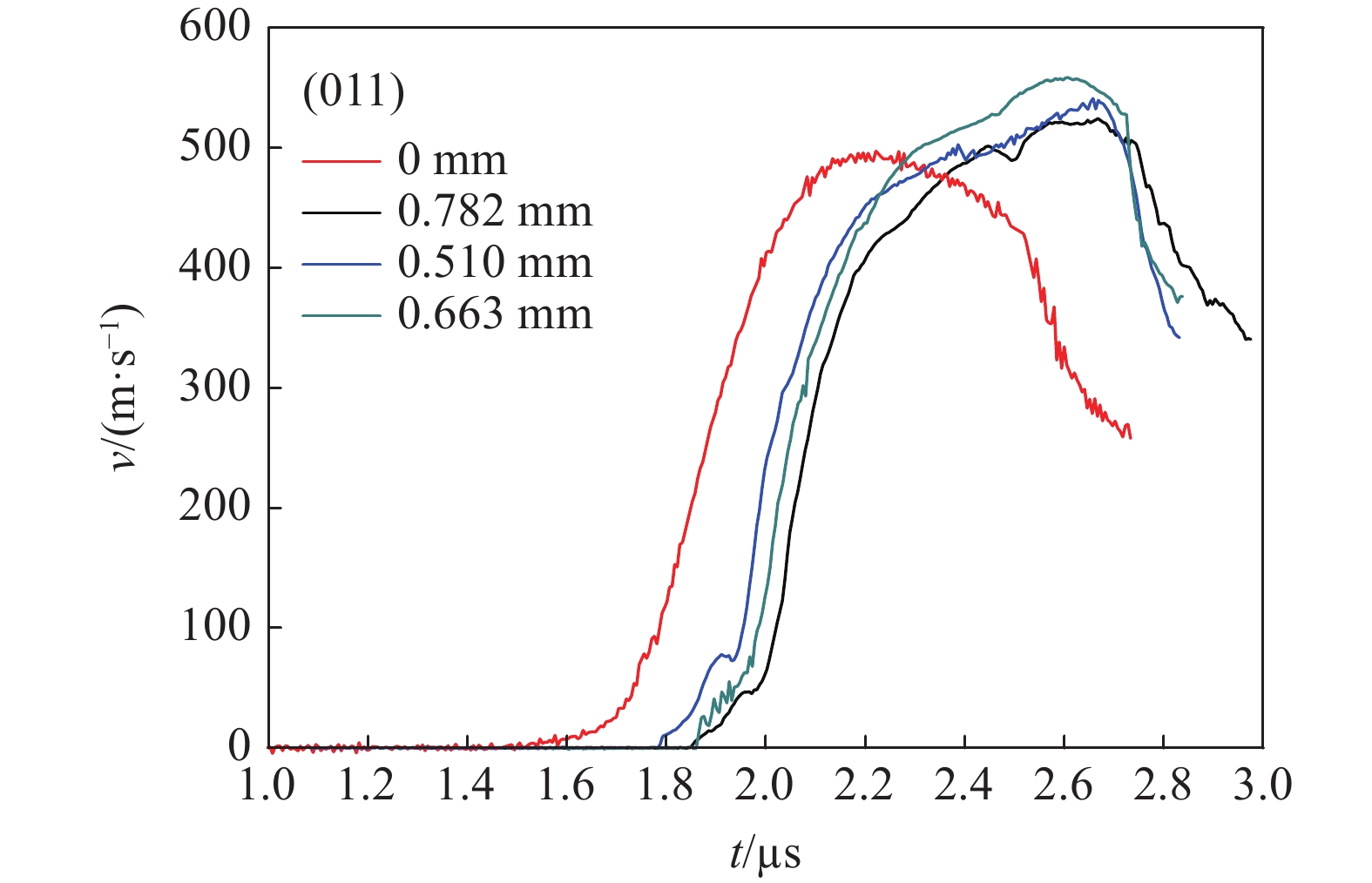
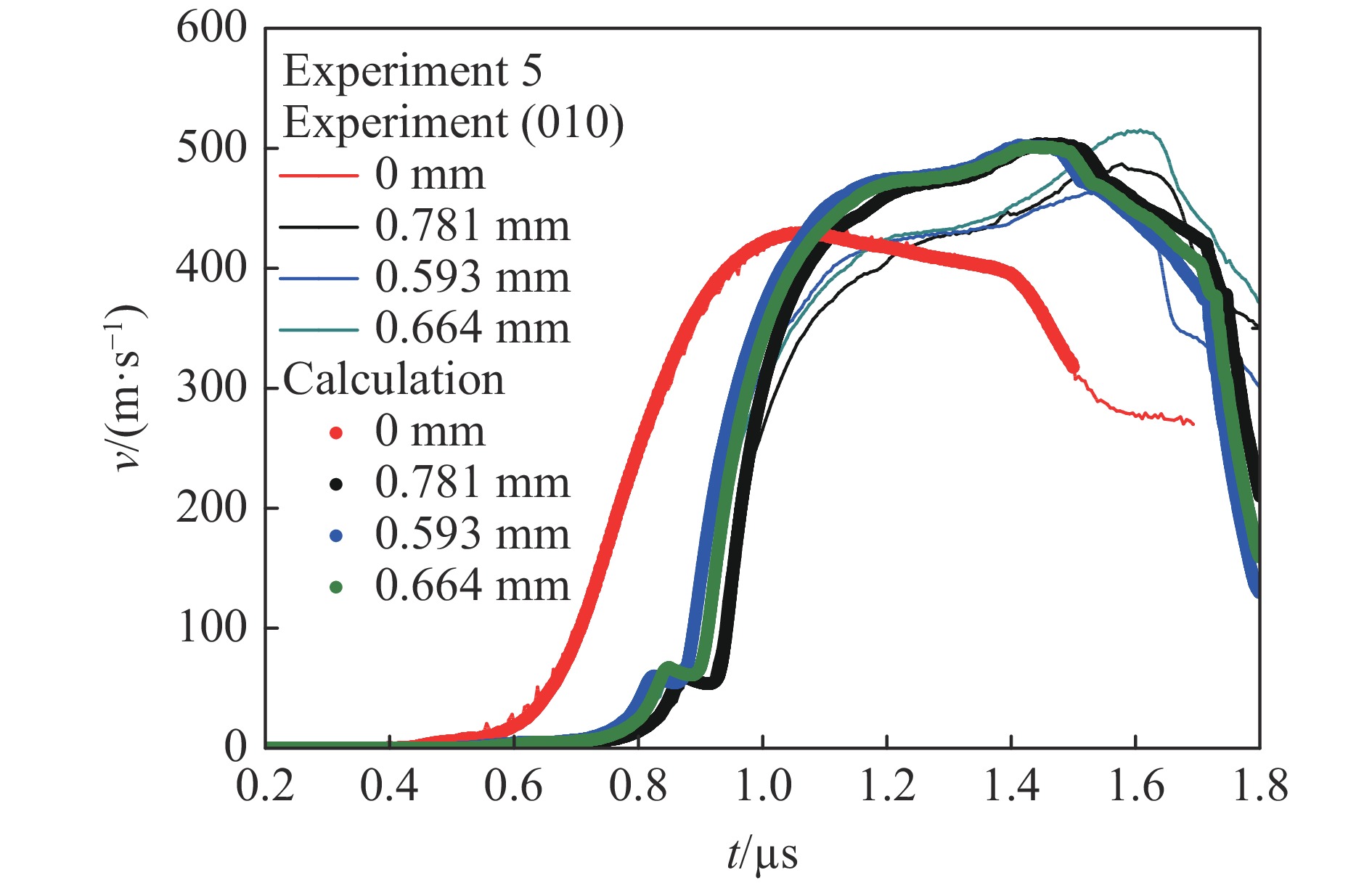
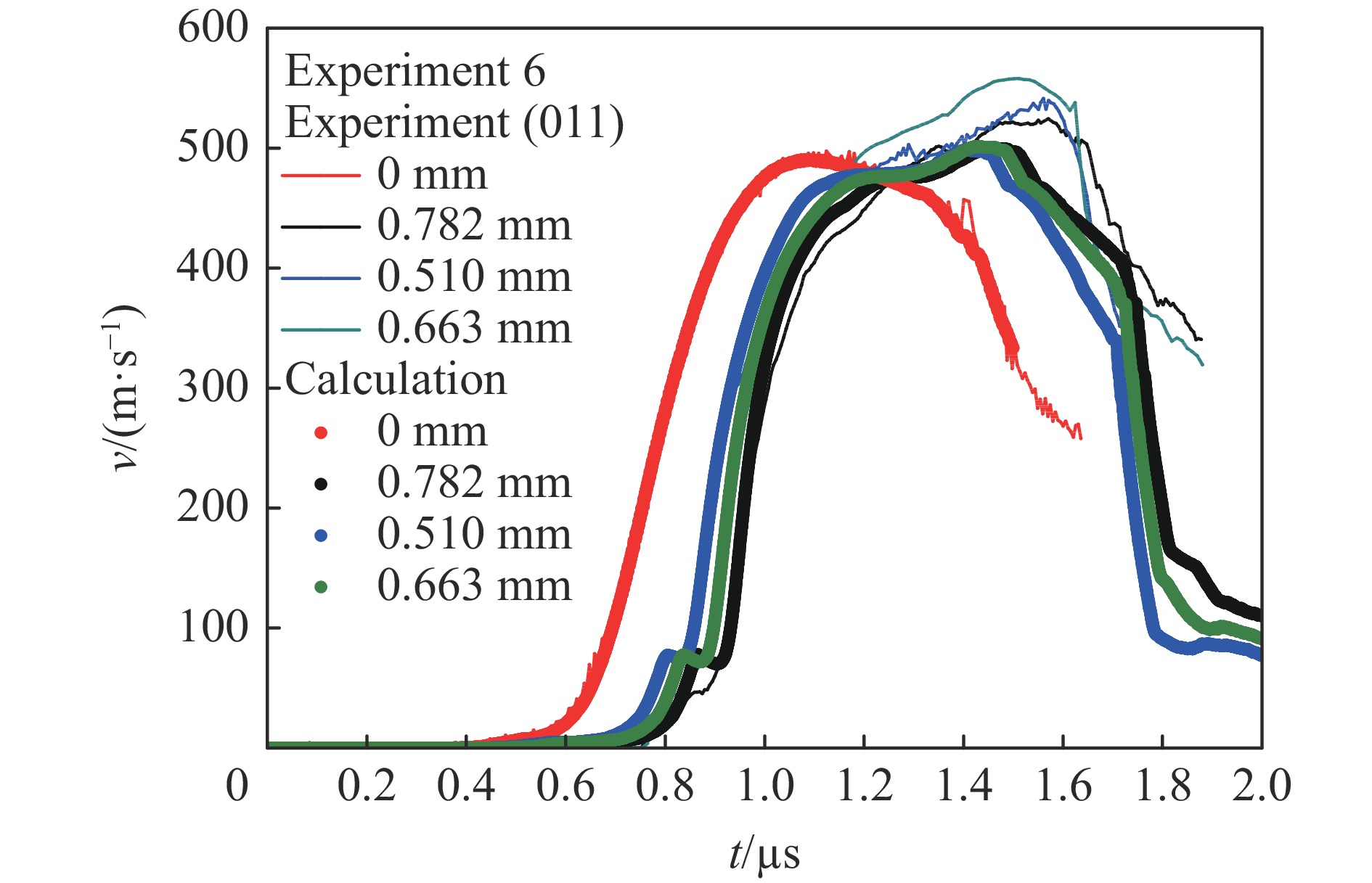
表 1 实验条件Table 1. Experimental condition实验编号 晶向 样品厚度/mm 1 (011) 1.398 0.984 2 (010) 1.262 0.975 3 (010) 1.253 0.961 4 (010) 0.775 0.913 5 (010) 0.593 0.664 0.781 6 (011) 0.510 0.663 0.782 本文进行了2轮实验:第1轮实验完成了1发HMX(011)晶向实验和3发HMX(010)晶向实验;第2轮实验降低了加载压力,2种晶向各完成了1发实验。第1轮实验获得的速度历史曲线见图2~5,4发实验都是两组不同厚度的HMX晶体上下对称布局。第2轮实验获得的速度历史曲线见图6~7。由实验结果得,速度波剖面都是弹塑性双波结构,且在弹塑性转变区出现了明显的速度松弛现象,有十几米每秒的速度降低。实验2和实验3实验结果显示,在厚样品的塑性后形成了冲击波。实验3中厚样品的速度峰值比薄样品的高,这可能是由于冲击波引起样品中有部分化学反应发生。为了避免样品中形成冲击波,减小HMX晶体样品厚度,实验4~6中样品中都没有形成冲击波。
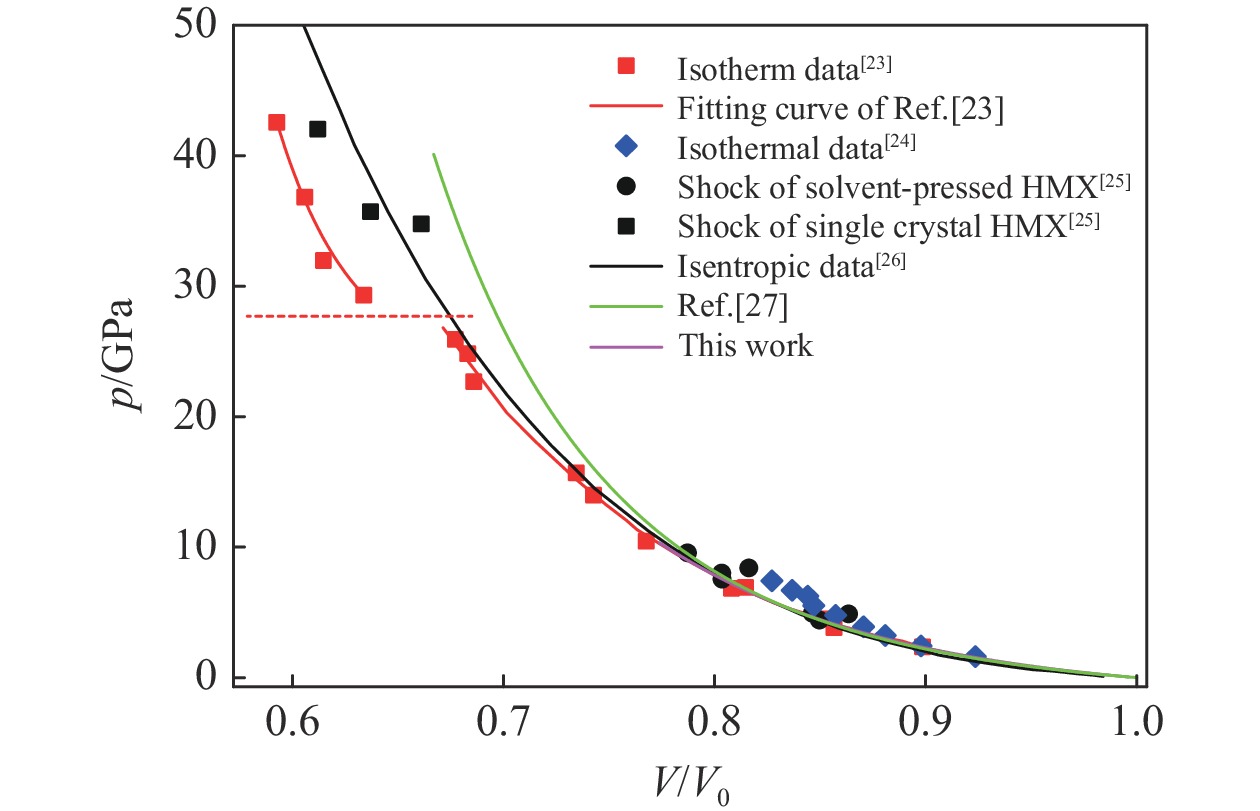
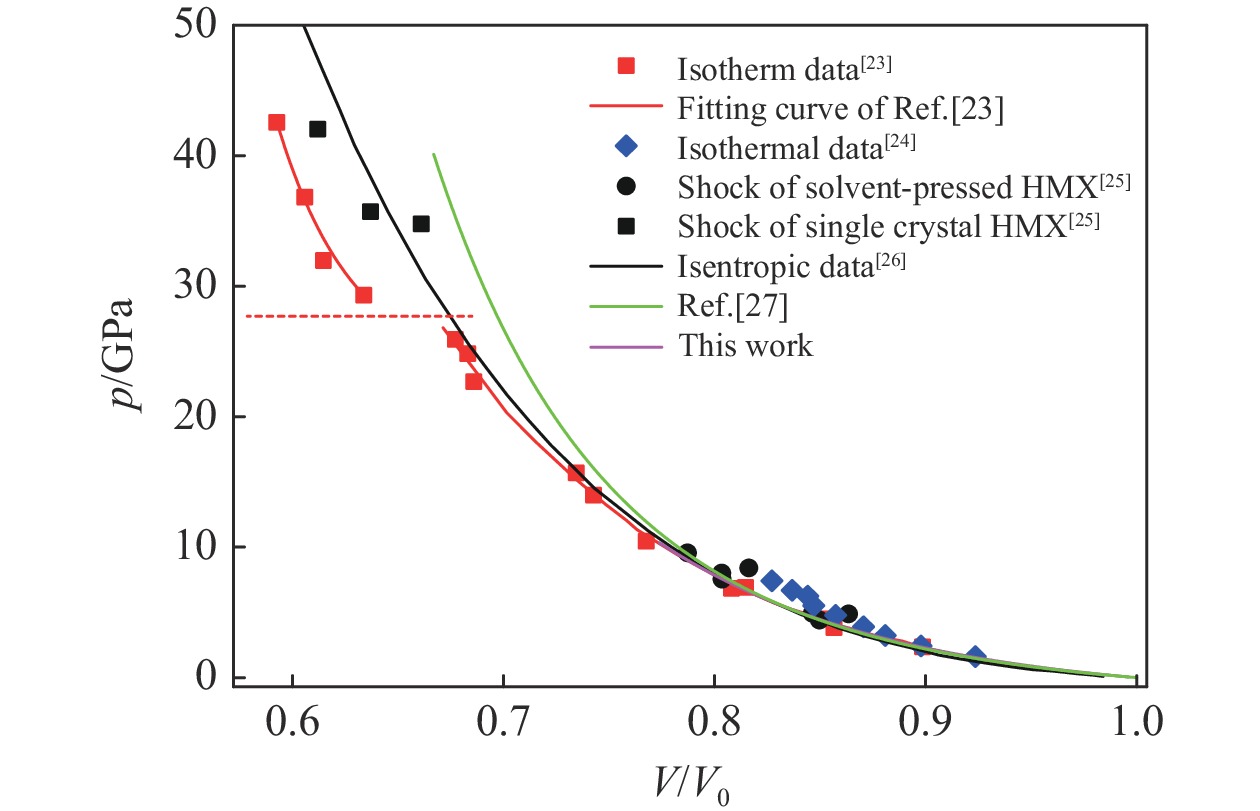
基于考虑阻抗失配修正的迭代Lagrange数据处理方法,完成了实验1和实验3两发实验的数据分析,获得了(011)和(010)两个晶向HMX晶体的压力-相对比容和声速-粒子速度曲线。(011)和(010)晶向HMX基于Hugoniot关系拟合的声速-粒子速度关系分别为us=2.728+2.234up和us=2.756+2.249 up。由于(011)和(010)两个晶向的p-V/V0关系参数基本一致,图中只给出(011)晶向的结果。静压实验结果[23-24]、LASL冲击实验数据[25]、准等熵加载实验结果[26]、苏锐等[27]采用分子动力学的计算结果和本文中结果见图8,本文工作结果与Yoo等[23]的静高压实验、文献[25]、Daniel等[26]的准等熵实验以及文献[27]中的计算结果基本吻合,说明15 GPa压力范围内未反应HMX晶体的等温线、准等熵和冲击Hugoniot线在压力-相对比容热力学平面未完全分离。
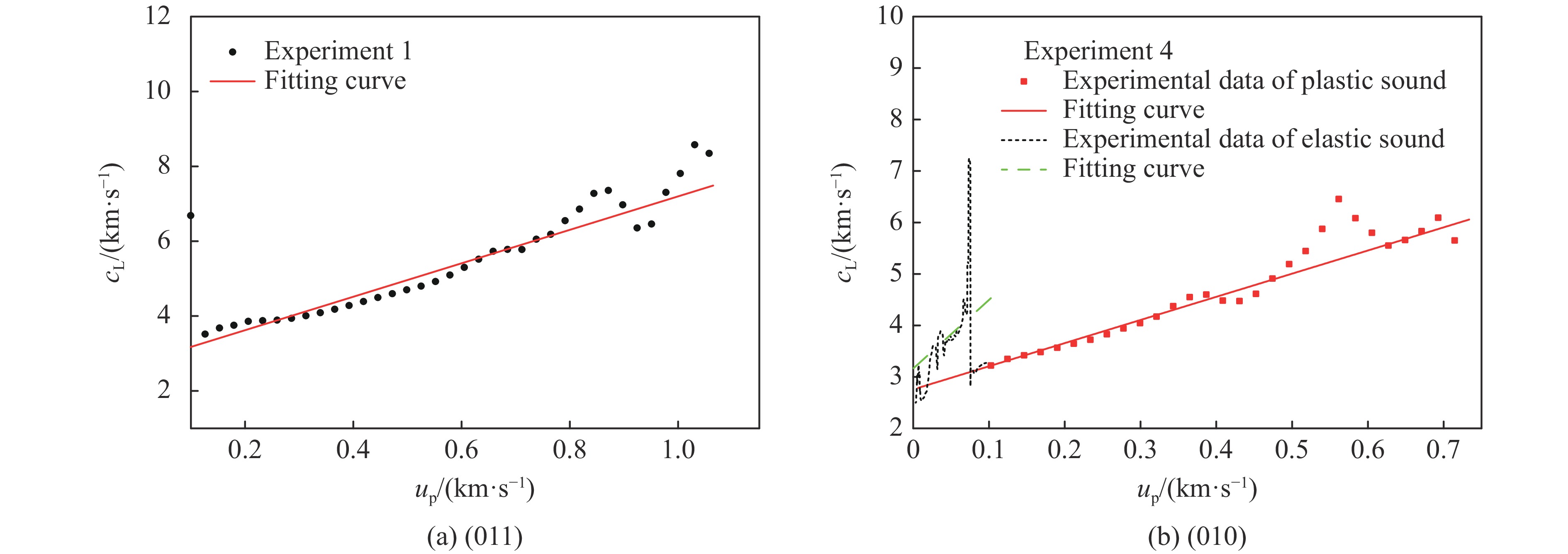
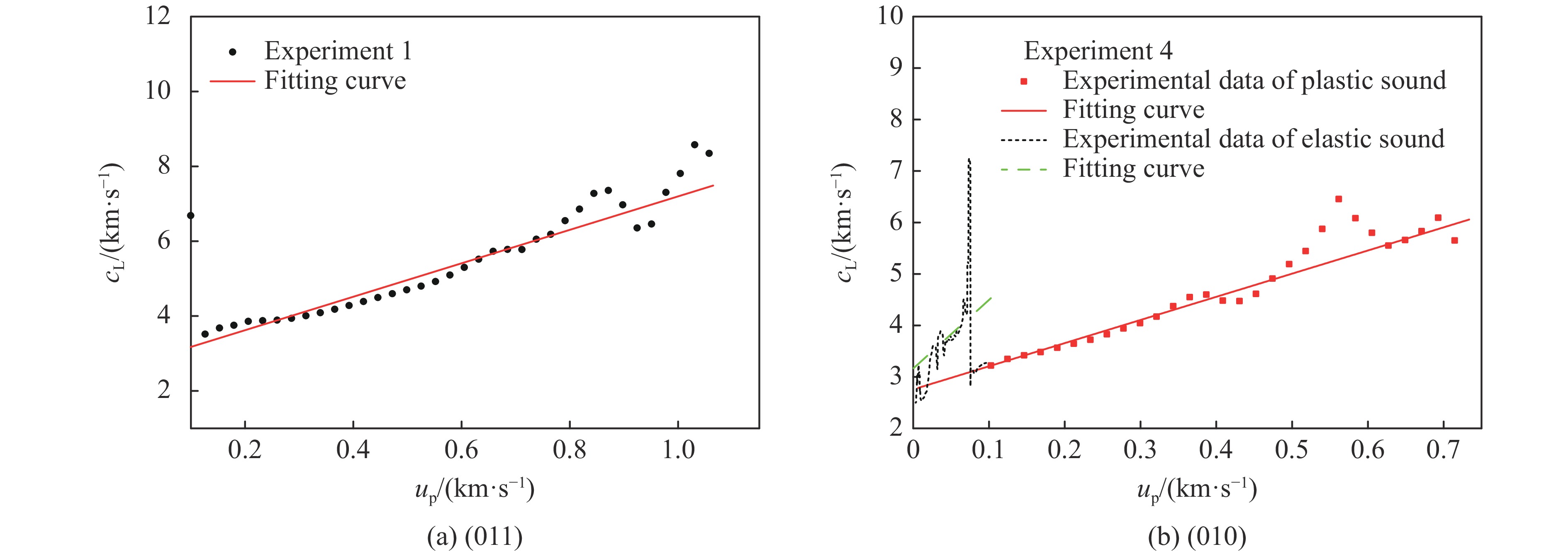
斜波加载实验对应样品一个连续的压缩过程,每发实验结果进行处理可获得压力峰值内连续变化的声速曲线,(011)和(010)晶向HMX晶体的拉氏声速曲线见图9。由实验1获得了(011)晶向HMX塑性段的拉氏声速-粒子速度曲线(图9(a)),线性拟合得到线性关系为cL=2.728+2×2.234up。由实验6获得了(011)晶向HMX塑性段线性关系为cL=2.765+2×2.226up。由实验4获得了(010)晶向HMX弹性段和塑性段的拉氏声速-粒子速度曲线(图9(b)),线性拟合得到弹性段线性关系为cL=3.022+15.867up,塑性段线性关系为cL=2.756+2×2.249up。由实验2获得了(010)晶向HMX塑性段线性关系为cL=2.713+2×2.255up。由实验3获得了(010)晶向HMX塑性段线性关系为cL=2.713+2×2.255up。由实验4获得了(010)晶向HMX塑性段线性关系为cL=2.741+2×2.242up。文献[28]对本文中的实验技术和数据处理不确定度进行了研究,实验得到的拉氏声速不确定度为1.5%。
由界面连续性条件可得,HMX样品与LiF窗口界面处的粒子速度和应力相等,可用弹塑性转变点处已知物性材料LiF窗口的应力代替HMX单晶的应力弹性极限σIEL。表2所示为本文中实验HMX晶体的弹塑性转变特征速度、样品厚度和计算的弹性极限。计算时,LiF单晶物性参数取密度ρ0=2.638 g/cm3,声速c0=5.15 km/s,声速对粒子速度的一阶系数 s=1.35。
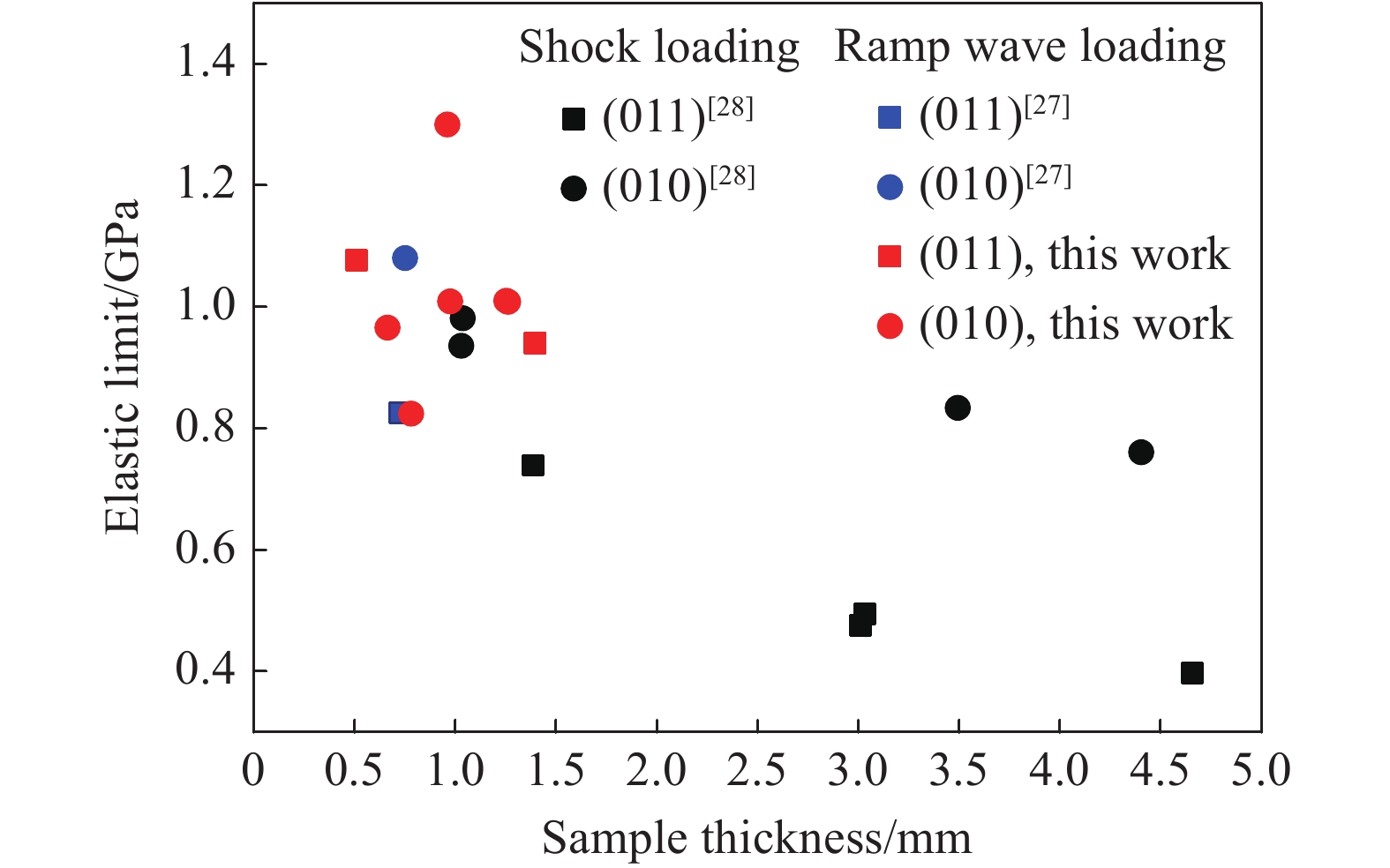
表 2 HMX晶体的屈服Table 2. Yield of HMX crystalsHMX晶向 厚度/mm 屈服速度/(m·s−1) 弹性极限 /GPa (011) 1.398 67.05 0.927 0.510 77.63 1.076 (010) 0.975 69.80 0.966 1.262 70.30 0.973 0.961 63.90 1.263 1.253 71.50 0.990 0.775 63.90 0.883 0.913 67.10 0.928 0.664 69.78 0.966 0.781 59.69 0.824 图10为动态加载下HMX晶体弹性极限与厚度的关系,其中Baeri等[29]的斜波加载实验数据和Dick等[18]的冲击加载实验结果作为参考。三方实验数据总体趋势相同:(010)晶向的屈服极限高于(011)晶向的屈服极限;随着样品厚度的增加,HMX晶体弹性极限出现变化。
2. 数值模拟
实验速度波剖面在弹-塑性转变过程中有明显的速度弛豫现象,这是由于有机大分子单晶材料的黏性造成的。为了更好地描述HMX晶体的斜波压缩物理过程,本文中采用Hobenemser-Prager本构关系和弹-黏塑性模型[30],高压物态方程采用适用于等熵热力学过程的三阶Birch-Murnaghan模型[31]。Hobenemser-Prager黏弹塑性本构关系的具体形式为:
˙eij={12G˙Sij+1−k√J22ηSij√J2>k12G˙Sij √J2≤k (1) 式中:G为弹性剪切模量,
˙eij 为偏应变率,η 为材料黏性系数,Sij 为应力偏量,J2 为应力偏量第二不变量,k为剪切屈服值。三阶Birch-Murnaghan物态方程的具体形式为:
p(V)=32KT0[(V0V)7/3−(V0V)5/3]{1+34(K′T0−4)[(V0V)2/3−1]} (2) 式中:V为比容,V0为初始比容,
KT0 为等温体模量,K′T0 为等温体模量对压力的一阶系数。基于以上物理模型及表3的模型参数(其中KT0和参考文献[32]并利用本文实验数据对其修正),对HMX晶体的斜波加载实验过程进行了数值模拟,计算和实验结果如图11~12所示。这里以Al/LiF窗口界面粒子速度计算的电极内表面压力历史为输入边界。由图11~12可得,计算结果与实验结果整体上吻合较好,尤其在弹塑性转变部分,计算结果能较好再现弹塑性区域的速度弛豫现象,说明本文中选择的物理模型及参数适用于HMX晶体斜波压缩动力学过程的描述。
表 3 模拟计算所用的模型参数Table 3. Model parameters used in simulation晶向 σy /GPa G/GPa η/(Pa·s) KT0/GPa K′T0 (010) 0.55 7 110 9.75 15.0 (011) 0.60 11 90 13.00 10.5 3. 结 论
利用磁驱动加载装置CQ-4和激光干涉测速技术,开展了15 GPa压力内两种晶向HMX晶体的斜波加载实验,获得了包含弹塑性转变信息的速度波剖面。实验结果显示,HMX晶体两个晶向的动力学参数存在差异,通过数据处理获得了两个晶向HMX晶体的压力-相对比容曲线和声速-粒子速度曲线。结合Hobenemser-Prager弹-黏塑性本构关系和三阶Birch-Murnaghan物态方程对实验过程开展了数值模拟,计算结果可以较好再现HMX晶体斜波压缩下弹塑性转变对应的速度弛豫过程。
感谢吴刚、邓顺益、税荣杰和胥超等在实验运行和测试方面的帮助。
-
表 1 实验条件
Table 1. Experimental condition
实验编号 晶向 样品厚度/mm 1 (011) 1.398 0.984 2 (010) 1.262 0.975 3 (010) 1.253 0.961 4 (010) 0.775 0.913 5 (010) 0.593 0.664 0.781 6 (011) 0.510 0.663 0.782 表 2 HMX晶体的屈服
Table 2. Yield of HMX crystals
HMX晶向 厚度/mm 屈服速度/(m·s−1) 弹性极限 /GPa (011) 1.398 67.05 0.927 0.510 77.63 1.076 (010) 0.975 69.80 0.966 1.262 70.30 0.973 0.961 63.90 1.263 1.253 71.50 0.990 0.775 63.90 0.883 0.913 67.10 0.928 0.664 69.78 0.966 0.781 59.69 0.824 表 3 模拟计算所用的模型参数
Table 3. Model parameters used in simulation
晶向 σy /GPa G/GPa η/(Pa·s) KT0/GPa K′T0 (010) 0.55 7 110 9.75 15.0 (011) 0.60 11 90 13.00 10.5 -
[1] 谭武军. 含能晶体力学性能研究[D]. 绵阳: 中国工程物理研究院, 2008.TAN W J. Studies on the mechanical properties of energetic crystals [D]. Mianyang: China Academy of Engineering Physics, 2008. [2] 李明, 陈天娜, 黄明, 等. RDX晶体的破碎与细观断裂行为 [J]. 含能材料, 2013, 21(2): 200–204. DOI: 10.3969/j.issn.1006-9941.2013.02.008.LI M, CHEN T N, HUANG M, et al. Rupture and mesoscale fracture behaviors of RDX crystals [J]. Chinese Journal of Energetic Materials, 2013, 21(2): 200–204. DOI: 10.3969/j.issn.1006-9941.2013.02.008. [3] 王国栋, 刘玉存. 神经网络在炸药晶体密度预测中的应用 [J]. 火炸药学报, 2007, 30(1): 57 –59. DOI: 10.3969/j.issn.1007-7812.2007.01.016.WANG G D, LIU Y C. Application of artificial neural network in predicting the density of explosives [J]. Chinese Journal of Explosives and Propellants, 2007, 30(1): 57 –59. DOI: 10.3969/j.issn.1007-7812.2007.01.016. [4] 花成, 傅华, 田勇, 等. 冲击波作用下HMX晶体的细观响应 [J]. 火炸药学报, 2010, 33(3): 5– 8. DOI: 10.3969/j.issn.1007-7812.2010.03.002.HUA C, FU H, TIAN Y, et al. Mesoscale response of HMX crystal under the shock war effect [J]. Chinese Journal of Explosives and Propellants, 2010, 33(3): 5– 8. DOI: 10.3969/j.issn.1007-7812.2010.03.002. [5] 黄明, 李洪珍, 徐容, 等. 高品质 RDX 的晶体特性及冲击波起爆特性 [J]. 含能材料, 2011, 19(6): 621–626.HUANG M, LI H Z, XU R, et al. Evaluation of crystal properties and initiation characteristics of decreased sensitivity RDX [J]. Chinese Journal of Energetic Materials, 2011, 19(6): 621–626. [6] HOWE P M. Effects of microstructure on explosive behavior [J]. Progress in Astronomics and Aeronautics, 2000, 185: 141. [7] JOHANSEN Ø H, KRISTIANSEN J D, GJERSØE R, et al. RDX and HMX with reduced sensitivity towards shock initiation-RS-RDX and RS-HMX [J]. Propellants, Explosives, Pyrotechnics, 2008, 33(1): 20–24. DOI: 10.1002/prep.200800203. [8] VAN DER HEIJDEN A E D M, BOUMA R H B, VAN DER STEEN A C. Physicochemical parameters of nitramines influencing shock sensitivity [J]. Propell. Explos. Pyrotech, 2004, 29(5): 304–313. DOI: 10.1002/prep.200400058. [9] WALLEY S M, FIELD J E, GREENAWAY M W. Crystal sensitivities of energetic materials [J]. Materials Science and Technology, 2006, 22(4): 402–413. DOI: 10.1179/174328406X91122. [10] CAULDER S M, MILLER P J, GIBSON K D, et al. Effect of particle size and crystal quality on the critical shock initiation pressure of RDX/HTPB formulations [C]// Proceedings of 13th Symposium (International) on Detonation. Norfolk, VA, USA, 2006: 656–661. [11] VANDER STEEN A C, VERBEEK H, MEULENBRUGGE J J. Influence of RDX crystal shape on the shock sensitivity of PBXs [C]// Proceedings of 9th Symposium (International) on Detonation. Portland, Oregon, USA, 1989: 83–88. [12] 花成, 张盛国, 高大元. 冲击波作用下炸药安全性QMU评估 [J]. 火炸药学报, 2015, 38(4): 31–34.HUA C, ZHANG S G, GAO D Y. QMU evaluation of explosive safety under shock wave effect [J]. Chinese Journal of Explosives and Propellants, 2015, 38(4): 31–34. [13] GOETZ F, BRILL T B, FERRARO J R. Pressure dependence of the raman and infrared spectra of α-, β-, γ-, and δ-octahydro-1, 3, 5, 7-tetranitro-1, 3, 5, 7-tetrazocine [J]. Journal of Physics Chemistry B, 1978, 82(17): 1912–1917. DOI: 10.1021/j100506a011. [14] CHOI C S, BOUTIN H P. A study of the crystal structure of β-cyclotetramethylene tetranitramine by neutron diffraction [J]. Acta Crystallographica Section B, 1970, 26(9): 1235–1240. DOI: 10.1107/S0567740870003941. [15] HORST J H, KRAMER H J M, ROSMALEN G M, et al. Molecular modelling of the crystallization of polymorphs: Part Ⅰ: the morphology of HMX polymorphs [J]. Journal of Crystal growth, 2002, 237: 2215–2220. [16] CLEMENTS B E, MAS E M. A theory for plastic-bonded materials with a bimodal size distribution of filler particles [J]. Modelling and Simulation in Materials Science and Engineering, 2004, 12(3): 407–421. DOI: 10.1088/0965-0393/12/3/004. [17] MENIKOFF R, DICK J J, HOOKS D E. Analysis wave profiles for single-crystal cyclotetramethylene tetranitramine [J]. Journal of Applied Physics, 2005, 97(2): 023529. DOI: 10.1063/1.1828602. [18] DICK J J, HOOKS D E, MENIKOFF R, et al. Elastic-plastic wave profiles in cyclotetramethylene tetranitramine crystals [J]. Journal of Applied Physics, 2004, 96(1): 374–379. [19] JARAMILLO E, SEWELL T D. Inelastic Deformation in shock loaded: HMX LA-UA-06-3716 [R]. Los Alamos National Laboratory Report, 2005. [20] SEWELL T D, BEDROV D, MENIKOFF R, et al. Elastic properties of HMX [J]. AIP Conference Proceedings, 2002, 620(1). DOI: 10.1063/1.1483562. [21] ZAUG J M. Elastic constants of β-HMX and tantalum, equation of state of supercritical fluids and fluid mixtures and thermal transport determinations [C]//The 11th International Detonation Symposium. Snowmass Conference Center. Snowmass Village, Colorado. 1998: 498−509. [22] HALL C A, ASAY J R, KNUDSON M D, et al. Experimental configuration for isentropic compression of solids using pulsed magnetic loading [J]. Review of Scientific Instruments, 2001, 72(9): 3587–3595. DOI: 10.1063/1.1394178. [23] YOO C S, CYNN H. Equation of state, phase transition, decomposition of β-HMX (octahydro-1,3,5,7-tetranitro-1,3,5,7-tetrazocine) at high pressures [J]. The Journal of Chemical Physics, 1999, 111(22): 10229. DOI: 10.1063/1.480341. [24] OLINGER B, ROOF B and CADY H H. The linear and volume compression of β-HMX and RDX [C]//Actes du Symposium International sur le Comportement des Milieux Denses Sous Hautes Pressions Dynamiques, Commissariat a l’Energie Atomique, Paris, 1978: 3−8. [25] MARSH S. LASL shock Hugoniot data [M]. Berkeley: University of California Press, 1980. [26] DANIEL, HOOK E and HAYES D B. Isentropic compression of cyclotetramethylene tetranitramine (HMX) single crystals to 50 GPa [J]. The Journal of Applied Physics, 2006, 99(12): 124901. DOI: 10.1063/1.2203411. [27] 苏锐, 龙瑶, 姜胜利, 等. 外部压力下β相奥克托金晶体弹性性质变化的第一性原理研究 [J]. 物理学报, 2012, 16(20): 336–341.SU R, LONG Y, JIANG S L, et al. Elastic properties of β–HMX under extra pressure: a first principle study [J]. Acta Physica Sinica, 2012, 16(20): 336–341. [28] 罗斌强, 张红平, 种涛, 等. 磁驱动斜波压缩实验结果的不确定度分析 [J]. 高压物理学报, 2017, 31(3): 295–300. DOI: 10.11858/gywlxb.2017.03.011.LUO B Q, ZHANG H P, CHONG T, et al. Experimental uncertainty analysis of magnetically driven ramp wave compression [J]. Chinese Journal of High Pressure Physics, 2017, 31(3): 295–300. DOI: 10.11858/gywlxb.2017.03.011. [29] BAER M, ROOT S, DATTELBAUM D, et al. Shockless compression studies of HMX-based and TATB-based explosives [C] // The 16th APS Topical Conference on Shock Compression of Condensed Matter. Nashville, Tennessee, 2009:699–702. [30] 贾乃文. 粘塑性力学及工程应用 [M]. 北京: 地震出版社, 2000. [31] HRBEK G M. Invariant functional forms for the second, third, and fourth order Birch-Murnaghan equation of state for materials subject to hydrodynamic shock. [J]. Aip Conference Proceedings, 2000, 505(1): 169–173. DOI: 10.1063/1.1303448. [32] 郭昕, 南海, 齐晓飞, 等. RDX和HMX晶体力学性能的分子动力学模拟及其撞击加载响应 [J]. 含能材料, 2013, 21(4): 485–489. DOI: 10.3969/j.issn.1006-9941.2013.04.016.GUO X, NAN H, QI X F, et al. Molecular dynamics simulation on mechanical properties of RDX and HMX crystals and their impacting loading response [J]. Chinese Journal of Energetic Materials, 2013, 21(4): 485–489. DOI: 10.3969/j.issn.1006-9941.2013.04.016. 期刊类型引用(1)
1. 种涛,傅华,李涛,莫建军,蔡进涛. 不同装药密度PBX-3炸药的高压声速和状态方程. 火炸药学报. 2022(03): 364-369 .  百度学术
百度学术其他类型引用(0)
-








 下载:
下载:











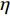








 下载:
下载:
 百度学术
百度学术