Research progress of target resistance model of cavity expansion theory and its application
-
摘要: 从静/动态空腔膨胀模型的理论体系出发,介绍了空腔膨胀模型在不同方向上取得的成果,主要涉及理想侵彻条件的空腔膨胀压力计算模型及数值模拟方法和空腔膨胀模型在典型侵彻问题及复杂弹靶条件下的应用。在理想侵彻条件下的空腔膨胀压力计算模型中,主要讨论了靶体材料、屈服准则和状态方程对空腔边界应力的影响规律及空腔膨胀模型的适用性问题;根据数值模拟中初始条件的不同,介绍了空腔表面恒定速度/恒定压力两种数值模拟方法,证明了数值模拟方法的可靠性;整理了空腔膨胀模型的基本假设、适用范围、工程应用特点,列举了其在典型侵彻问题及多层复合靶板、约束靶体、弹体刻槽和异形截面形状弹体等复杂弹靶条件下的应用。针对空腔膨胀模型的研究现状,总结了目前空腔膨胀模型在冲击动力学领域的应用方向,归纳了空腔膨胀模型应用中尚存在的问题,展望了空腔膨胀模型下一步的重点发展方向。Abstract: The cavity expansion theory is one of the main basic theories for the theoretical analysis of penetration problems. It is mainly used to analyze the failure response characteristics of typical target materials under impact load, and then to determine the penetration resistance of the target. It is widely used in the analysis of high-speed impact penetration and failure problems. Domestic and foreign scholars have made abundant research achievements on plastic and (quasi) brittle materials based on the theory of cylindrical and spherical cavity expansion. Starting from the theoretical system of the static/dynamic cavity expansion model, the results of the cavity expansion model in different directions are introduced, mainly involving the cavity expansion pressure theoretical calculation model and numerical simulation method under ideal penetration conditions, and the application of cavity expansion model to typical penetration problems and complex missile target conditions. The theoretical calculation model under ideal penetration conditions based on cavity expansion theory mainly discusses the influence aspects of target material, yield criterion and equation of state on target resistance and the applicability of the cavity expansion model. According to the different initial conditions in the numerical simulation, two numerical simulation methods of cavity surface constant velocity/constant pressure are introduced, and the reliability of the numerical simulation method is proved. The basic assumptions, application scope and engineering application characteristics of the cavity expansion model are summarized, and its applications in typical penetration problems and complex missile targets such as multilayer composite target plate, constrained target, projectile grooves and projectile body with special cross-section are listed. Based on the current status of the cavity expansion model, we summarized the current cavity expansion model application direction in the field of impact dynamics, and the problems existing in the application of the cavity expansion model, as well as the key development direction in the cavity expansion model.
-
Key words:
- penetration /
- cavity expansion theory /
- resistance model
-
随着固体动力学、弹塑性动力学、计算力学、材料力学以及数学分析工具的发展,人们不再满足于实验研究这种只能给出弹靶作用最终结果的研究方法。弹体侵彻过程最基本的问题是确定靶体对弹体的作用,即阻力函数。目前,应用较多的研究方法有空腔膨胀模型[1-3]、局部相互作用理论[4]、微分面力法[5]、速度势和速度场理论[6]、土盘模型[7-8]等。其中较为成熟且应用广泛的确定靶体阻力函数方法为空腔膨胀模型,该模型综合考虑了靶体强度(由屈服准则描述)、体积模量(由状态方程描述)和密度等多种因素的影响,主要用于分析典型靶体材料在冲击载荷下的破坏响应特性,进而确定靶体的抗侵彻阻力。学者们先后以柱形、球形空腔膨胀理论为基础,针对塑性、(准)脆性材料,取得了丰富的研究成果。准静态球形和柱形空腔膨胀模型的控制方程最早由Bishop等[1]提出,并求解得到了锥形弹体低速侵彻金属靶体的阻力。Bishop等[1]和Hopkins[3]首次给出了动态空腔膨胀的控制方程,通过求解方程得到了不可压缩理想弹塑性靶体对弹体的阻力。Hunter等[9]首先假设材料的屈服应力和密度之间的比值为常数, 利用相似变换建立了可压缩材料的动态球形空腔膨胀模型, 该研究开创了相似变换在空腔膨胀理论中应用的先河,对空腔膨胀模型的发展应用起到了重要的推动作用。空腔膨胀理论的发展与更新速度很快,其关键问题则集中于材料本构关系和破坏准则的修正,即将材料体积变形方程从不可压缩向跃变压缩、线性压缩、非线性压缩发展;强度模型则从理想弹塑性向线性硬化、幂次硬化、压力相关强度准则、分阶段强度准则过渡,并可考虑脆性断裂效应,其发展路线图如图1所示。
Hopkins[3]全面总结了第二次世界大战后关于空腔膨胀理论的相关研究工作。自此以后,学者们以空腔膨胀理论为基础,发展了可用于描述多种类靶体材料的阻力模型计算方法,较好地应用于工程实际问题中,成为靶体阻力计算的重要手段。本文中主要介绍空腔膨胀模型的起源及理论体系、塑性及脆性材料的响应分区、空腔径向应力的理论及数值求解方法、空腔膨胀模型在多个方向的应用等,结合弹靶侵彻效应发展特点给出空腔膨胀模型理论体系及应用的未来研究方向。
1. 空腔膨胀模型理论体系
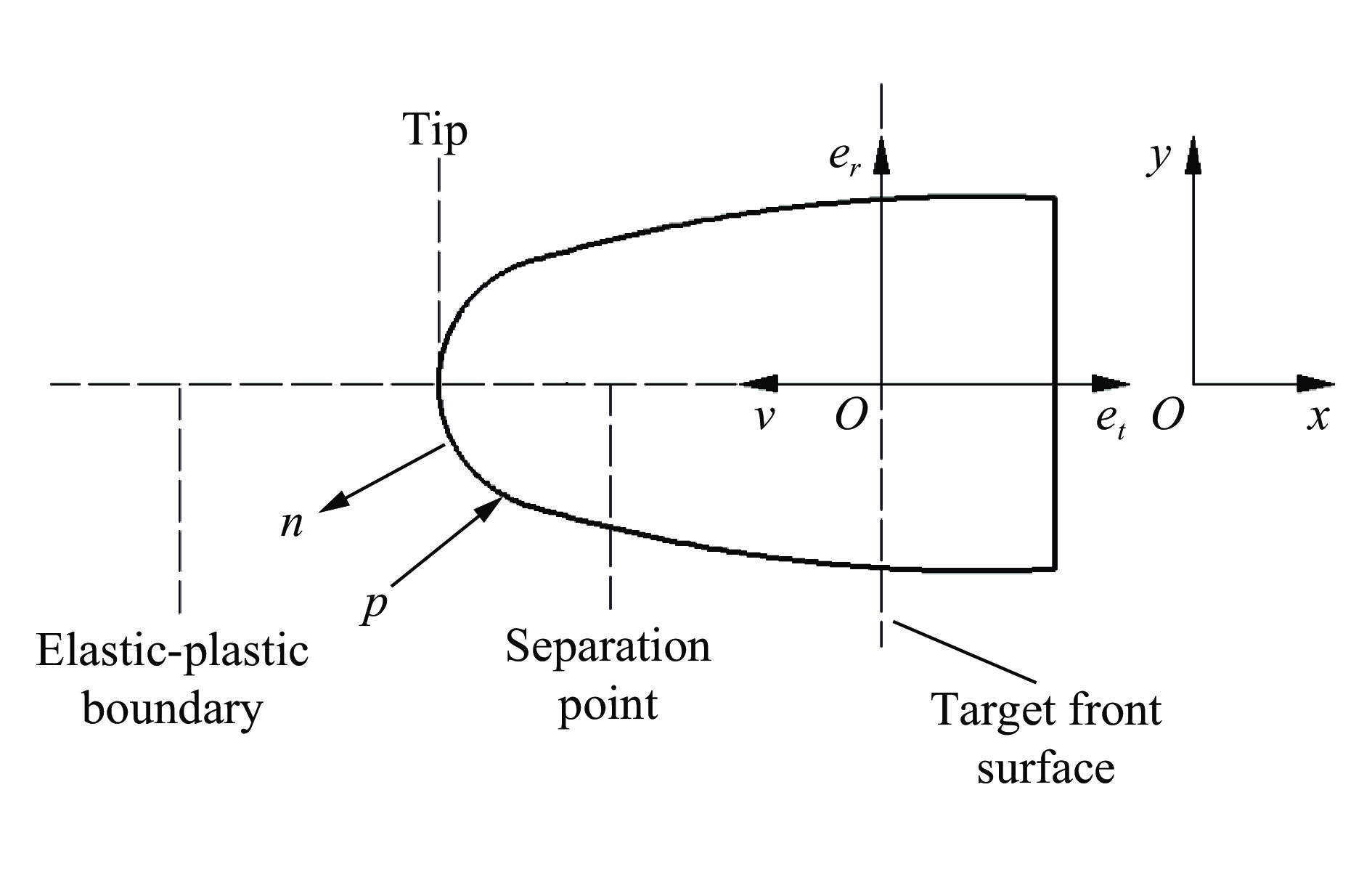
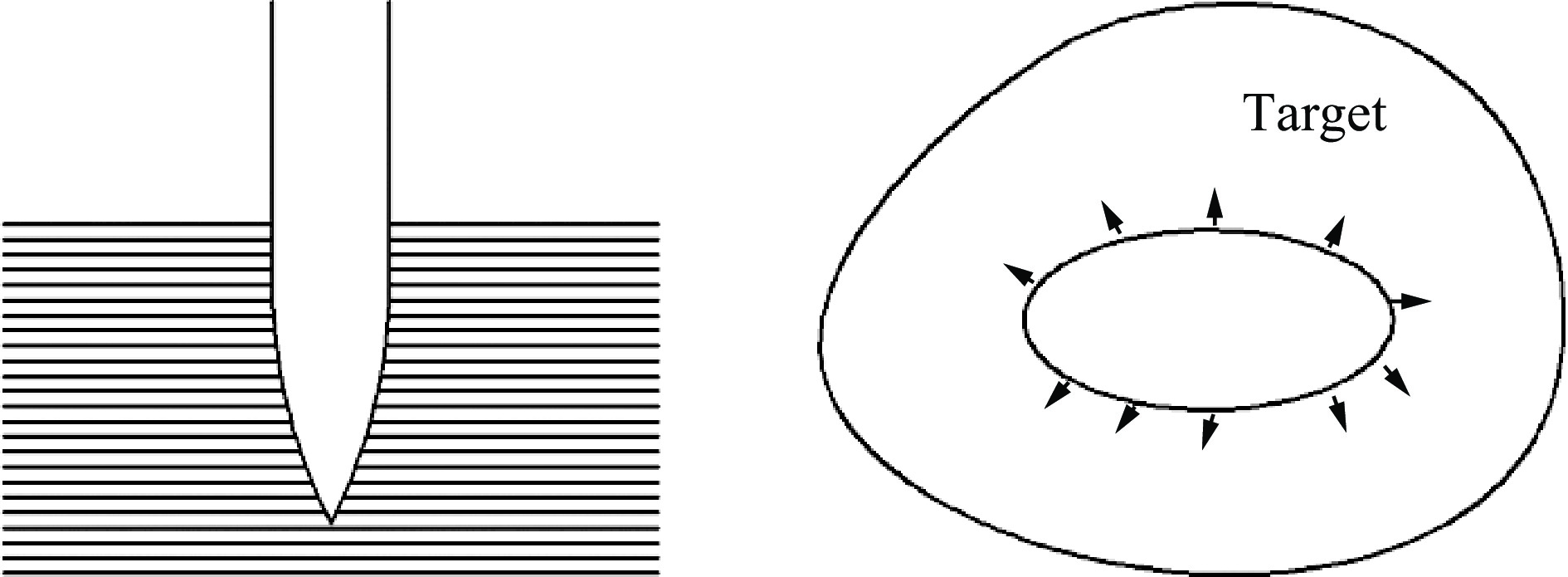
相关研究工作表明,当加载于材料上的载荷超过材料本身的屈服极限时,材料出现层次分明的不同响应区。Collombet等[27]、Curran等[28]、Wei等[29]分别开展了典型塑性及脆性材料在高速冲击下的响应特性研究,获得了如图2~3所示的靶体破坏形式。这与弹体高速撞击到装甲上时,发散的球面波在装甲内传播的情形类似;而在弹体侵彻靶体中的稳定侵彻阶段,靶体中的开孔与弹体侵彻形成的空腔类似。因此,靶体对弹体的抗侵彻阻力可以通过无限域介质内部空腔膨胀的压力来模拟。
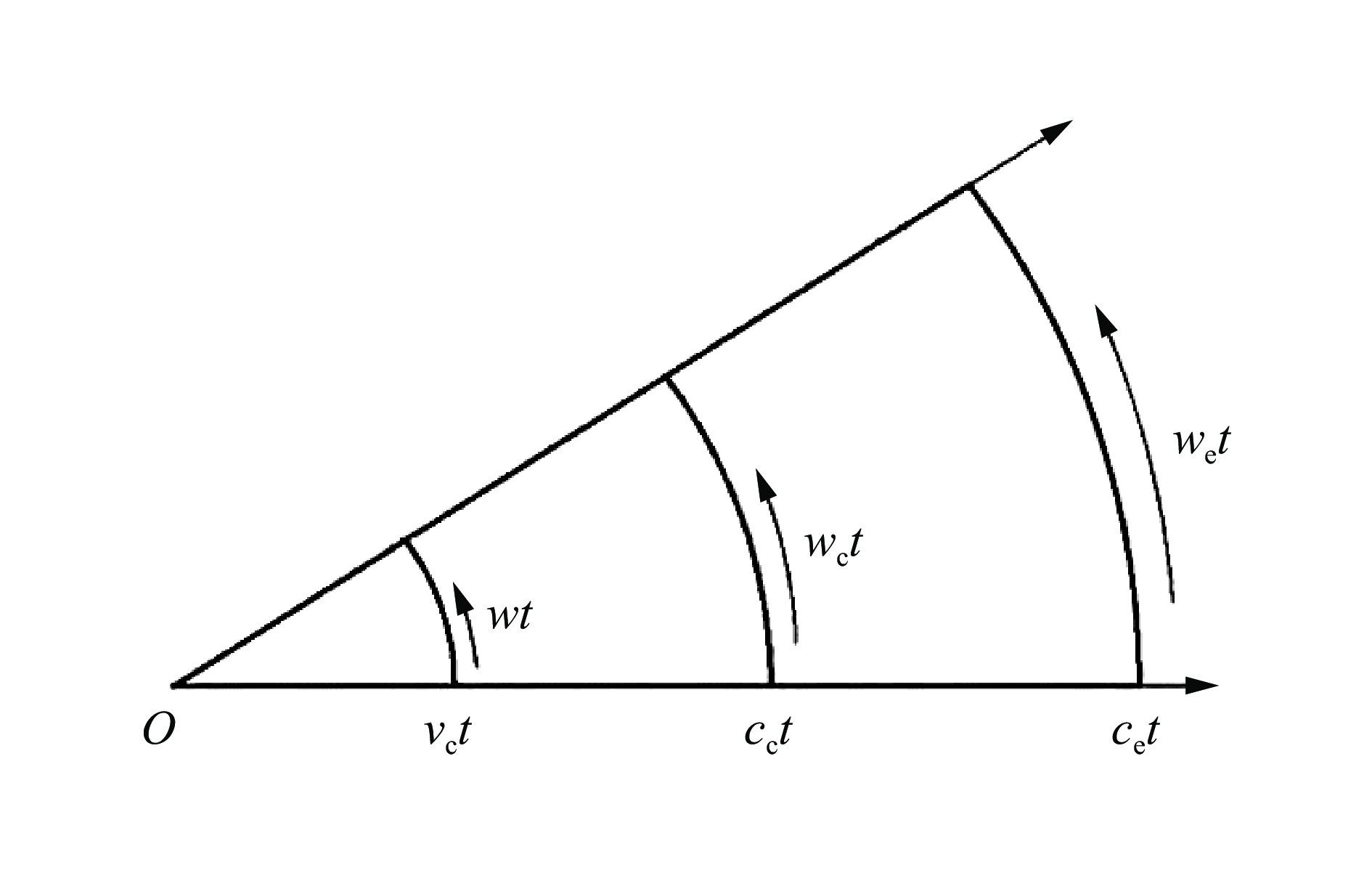
空腔膨胀模型的基本假设是:用空腔在无限大介质中膨胀时所产生的表面径向应力,替代弹体侵彻过程中表面法向所受到的阻力。材料在空腔扩展下的响应分区与材料自身动态力学特性相关,塑性与脆性材料的空腔响应及分区特点区别明显,图4为响应区示意图,其中cc、cd、cp、ce分别为弹性区与开裂区、开裂区与粉碎区、弹性区与塑性区的界面传播速度及弹性波波速。通常对金属材料都假定空腔扩展会在靶体内产生弹性-塑性响应分区[30]。对脆性材料而言,当膨胀速度较低时,空腔扩展会在靶体内形成弹性-开裂-粉碎响应分区;当膨胀速度较高时,则为弹性-粉碎响应分区[31]。
空腔膨胀模型的求解一般是从质量和动量守恒方程出发,并根据连续介质力学的基本原理以及材料的本构方程来推导空腔表面径向应力的大小,所得结果一般可以表示为空腔膨胀速度的二次函数形式[32]。根据模型假定不同,空腔膨胀模型分为球形/柱形空腔膨胀,球形和柱形[32-33]空腔膨胀理论适用于不同的研究对象,两种模型对不同弹体头部形状的计算精度不同。一般而言,在球、柱坐标系下,靶板介质的质量和动量守恒方程分别为:
ρ(∂v∂r+kvr) = −(∂ρ∂t+v∂ρ∂r) (1) ρ(v∂v∂r+∂v∂t) = ∂σr∂r+kr(σr−σθ) (2) 式中:v为质点速度,r为径向坐标,
σr 、σθ 分别为径向、环向应力,ρ 为材料密度;当k=1 时,为柱坐标系;当k=2 时,为球坐标系。空腔膨胀模型的基本方程包括守恒方程和材料的本构方程,可结合边界条件、初始条件和波阵面间断条件或弹塑性边界的连续性条件等求解。边界条件一般为位移边界条件和应力边界条件,空腔壁处质点位移等于空腔半径
ura=ra 以及空腔外侧边界处径向应力σre=σ(t) ,其中σ(t)=0 时为自由边界。各响应分区的交界面满足Hugoniot跳跃条件,质量守恒、动量守恒形式的Hugoniot跳跃条件为:ρ−(v−−c)=ρ+(v+−c) (3) σ−+ρ−v−(v−−c) = σ + +ρ + v + (v + −c) (4) 式中:c为波阵面速度,下标+、−分别代表波前、波后介质状态。
最理想化的模型为弹塑性不可压缩材料的静态空腔膨胀模型,模型假设在弹塑性材料的空腔边界
(r=r0) 处施加一个恒定载荷p0 ,并不考虑惯性作用和波动效应,当载荷逐渐增加至临界值、材料达到稳定膨胀时,空腔边界径向应力σr 则为材料静阻力Rt 。Bishop等[1]、Hill等[34]、Chadwick[2]、Hopkins[3]、Huang[35]和Forrestal等[10]分别给出了静态空腔膨胀解的几个等效推导,典型结果如下式:σr=2Y3[1 + ln(2E3Y)] (5) 式中:Y为材料的屈服强度,E为弹性模量。
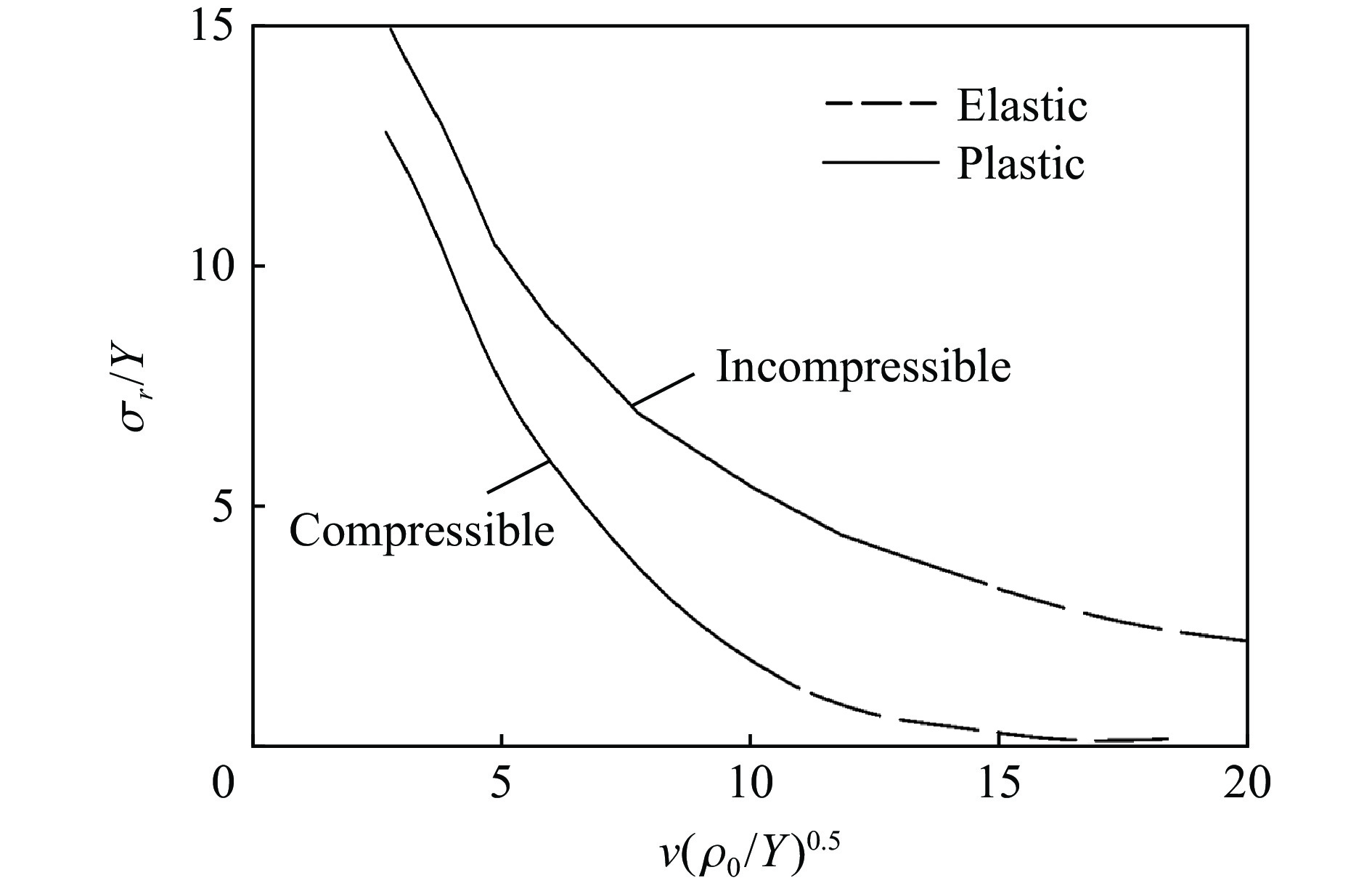
静态空腔膨胀模型未考虑材料的惯性效应,而当膨胀速度较高时,惯性效应对空腔径向应力计算结果的影响不可忽略。Hill[34]第一个给出了不可压缩延性材料的动态空腔膨胀模型的解析解。通过假设材料不可压缩并结合小变形假设,得出弹塑性边界c与空腔边界a的关系为
c(t)/a(t)=E/(1+ν)Y ,空腔径向应力为:σr=2Y3[1 + ln(2E3Y)]+32ρv2Y (6) 式中:第1项为准静态项,与静态空腔膨胀模型结果相同;第2项为与速度相关的惯性项。
材料的不可压缩假设有利于空腔膨胀模型解析解的求解,但正如Chadwick[2]和Hunter等[9]所指出的,不可压缩假设导致了弹性波速是无穷大的,且由于空腔膨胀速度与塑性波速具有恒定的比例,空腔膨胀速度不可能超越塑性波速,与实际情况不符。
Hunter等[9]指出,对于以恒定速度扩张空腔的情况,由于侵彻过程主要是由稳态侵彻部分控制,而稳态侵彻具有自相似性,因此与稳态侵彻相关的动态空腔膨胀也具有自相似性,可以采用相似变换求解,其中应力、速度和密度取决于单个相似性变量。Hunter等[9]假设在整个空腔扩展过程中,屈服应力与密度之比是恒定的,研究结果表明,随着空腔扩张速度的增加,弹塑性界面速度逐渐增加,并最终达到弹性波速度。
Forrestal等[11]在Hunter等[9]的基础上,给出了以恒定速度扩展的可压缩材料的动态空腔膨胀解决方案。通过使用Hugoniot跳跃条件以及材料在弹塑性界面的任一侧屈服的条件,Forrestal等[11]计算得出粒子速度、径向应力在弹塑性边界上是连续的,并引入了以下无量纲参数:
S=σrK,T=YK,U=vc,ζ=rct,ε=uc,β=ccp (7) 式中:
cp=√E/ρ ,u为空腔膨胀速度,K为体积模量,v为粒子速度,S、T、U、ξ 、ε 、β 均为无量纲变量。通过相似变换,径向无量纲应力可以表示为:
Sc=S2−β2(ε2−U22)2+2(U2β2+T)(1−ε)ε(1−β2)−2Tlnε+Tln(1−β2ε21−β2)+Tβεln[(1+β2ε)(1−β)(1−β2ε)(1+β)] (8) 式中:S2和U2通过弹塑性边界两侧应力、位移连续得出,S2和U2由下式确定:
U2=T(1+v)3(1−2v),S2=2T3[1+(1+v)α2(1−2v)(1+α)] (9) 式中:
α=c/cd ,cd=√E(1−υ)/[ρ0(1+υ)(1−2υ)] 。为了求解式(8),必须确定弹塑性界面速度c。通过空腔从零初始半径开始的条件,弹塑性界面速度c可以从以下超越方程中解出:
Tβε+β3ε3−β(U2β2+T)(1−β2ε2)1−β2−T(1−β2ε2)2ln(1+β2ε)(1−β)(1−β2ε)(1+β)=0 (10) 空腔膨胀模型理论体系是由基本假定、响应模式、守恒方程、本构模型(屈服准则和状态方程)和求解条件(边界、界面和应力波条件等)组成的完备体系。材料的稳定响应区划分、屈服准则、状态方程、求解条件等影响因素不是割裂的,共同影响模型计算的空腔边界径向压力
σr ;当采用不同假设条件时,相同材料参数计算出来的空腔径向应力也会有所差别。2. 理想侵彻条件的空腔膨胀压力计算模型
Poncelet公式是最古老和最经典的计算平头弹撞击金属靶侵彻阻力的公式:
F=A0(a1+b1v2) ,其中A0 是弹体的横截面积,v是撞击速度,a1、b1为实验确定的材料常数。理想侵彻条件指具有一般凸形头部形状的刚性弹垂直侵彻半无限靶目标[19],在考虑了弹形几何因素后,理想侵彻条件下的空腔膨胀压力计算模型[11]得到了与Poncelet公式几乎完全相同的侵彻阻力计算式,即侵彻阻力由靶体材料的静强度项和流动阻力组成,动态空腔膨胀理论进一步提供了Poncelet公式的理论基础。自20世纪80年代以来,美国Sandia国家实验室Forrestal团队分别对球形和柱形动态空腔膨胀理论进行了详细的研究,同时进行了大量侵彻实验对其验证。此后有关空腔膨胀的工作主要集中在:
(1)不同的靶体材料,如铝合金[11-12]、混凝土[13, 36-37]、土[10]、岩石[14-15]、沙子[38]和陶瓷[16]等;
(2)靶体塑性区屈服准则,Tresca屈服准则[12-13]、Drucker-Prager屈服准则[37]、Mohr-Coulomb屈服准[14-15, 36, 38]等;
(3)塑性区状态方程,线性静水压力-体应变状态方程[30, 36]、线性锁应变模型[10, 13]、三阶段非线性状态方程[37]以及p-α状态方程[38]等。
2.1 靶体材料
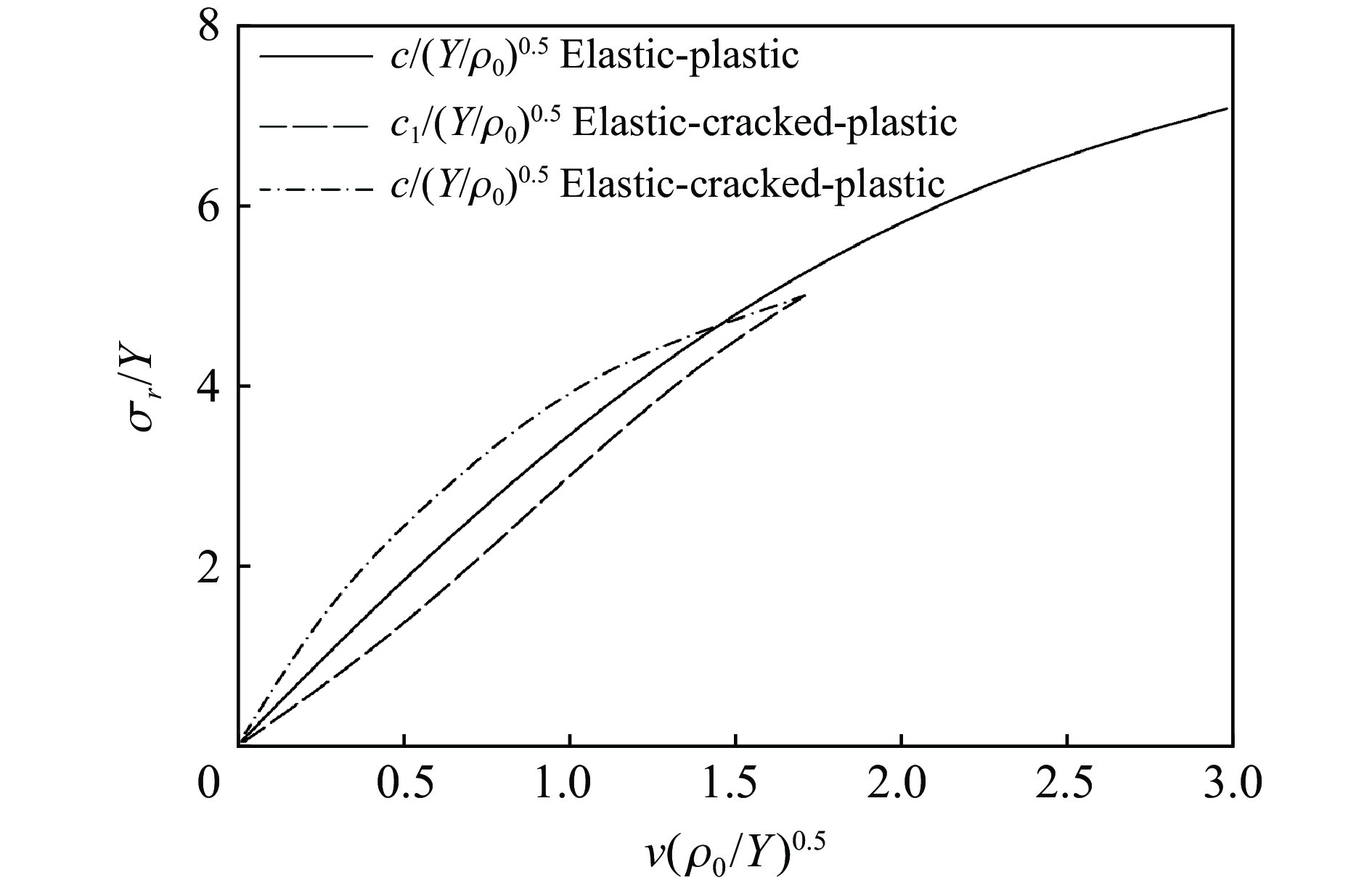
陈小伟等[19]和Forrestal等[11-12]针对铝合金材料,假定材料为幂应变硬化、速率无关的弹塑性材料,建立了空腔初始半径从零开始的动态膨胀模型,计算结果如图5所示。Forrestal等[11-12]分析了可压缩性对材料的影响,发现材料的不可压缩假设会高估空腔阻力。Forrestal等[30]借鉴金属材料空腔膨胀理论,考虑混凝土的拉伸断裂,将混凝土的响应区分为塑性区-裂纹区-弹性区和塑性区-弹性区两种模式,假设塑性区满足Mohr-Coulomb 失效准则,裂纹区的环向应力为零,考虑不可压缩和可压缩线性压力-应变关系两种情况,计算结果如图6所示。研究表明,响应区划分对塑性区应力影响较大,在混凝土材料响应区中加入裂纹区会降低空腔径向应力值;而不同的响应区划分下,弹性-裂纹区界面移动速度大于塑性-裂纹区界面移动速度。
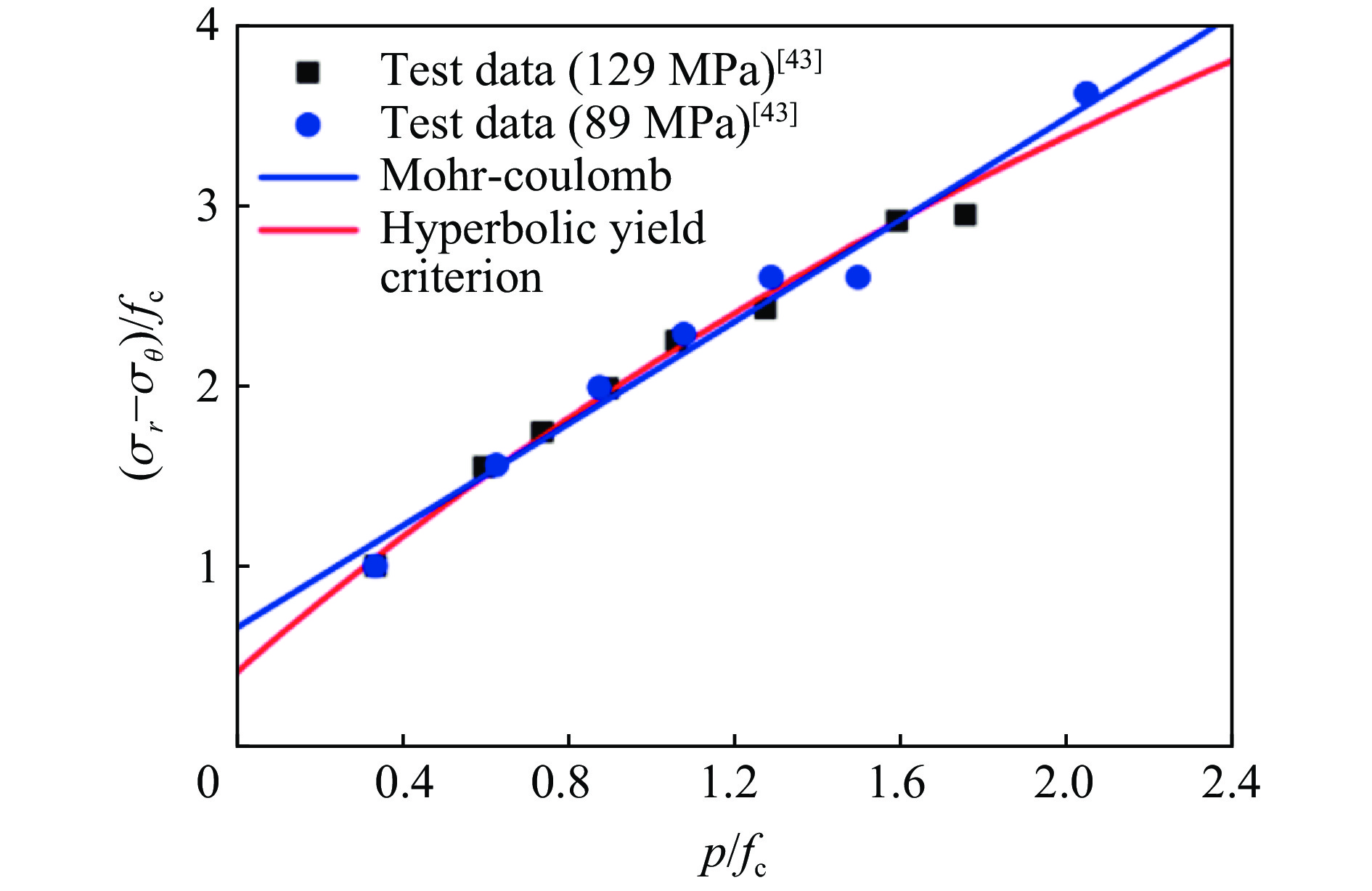
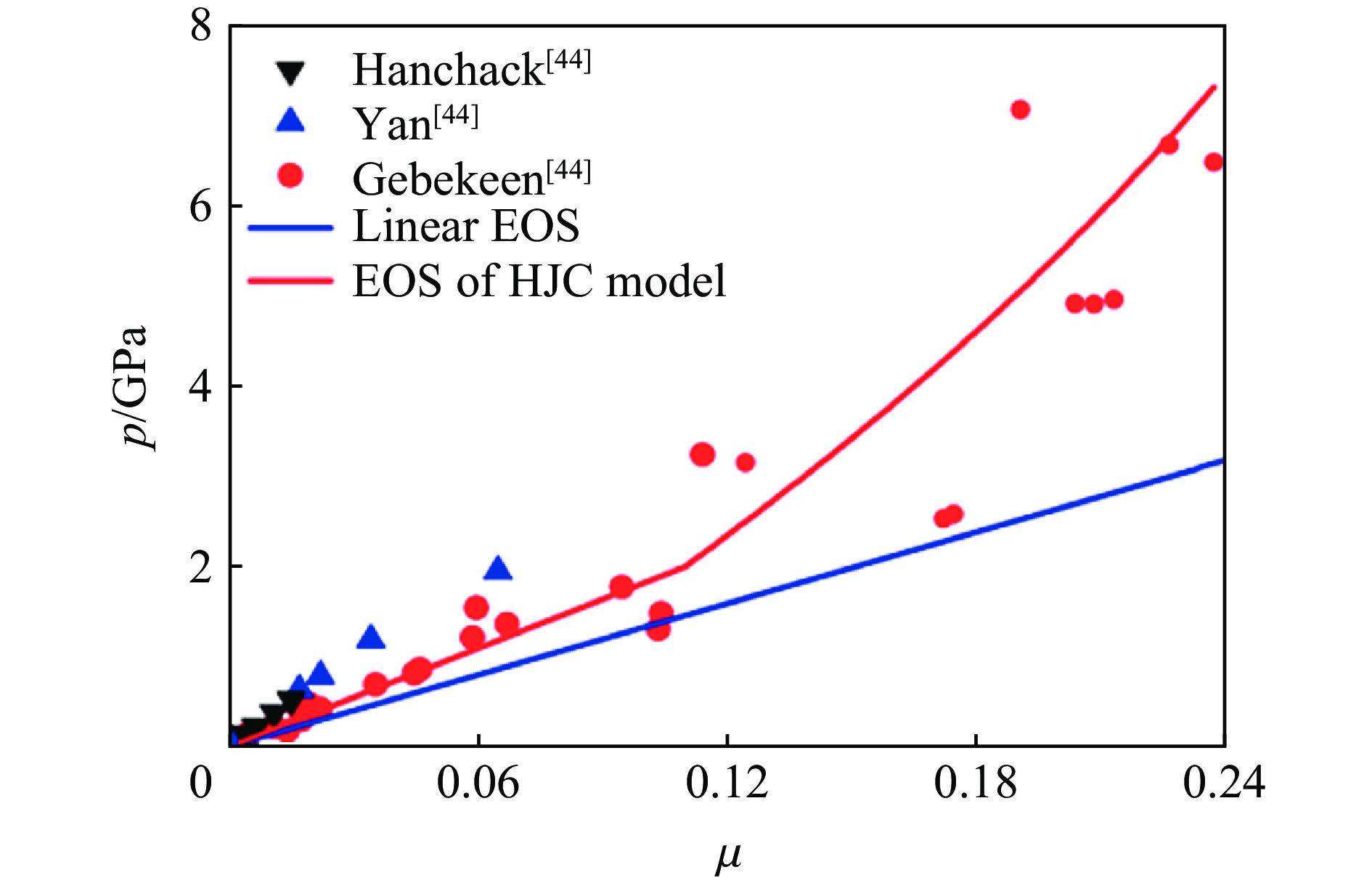
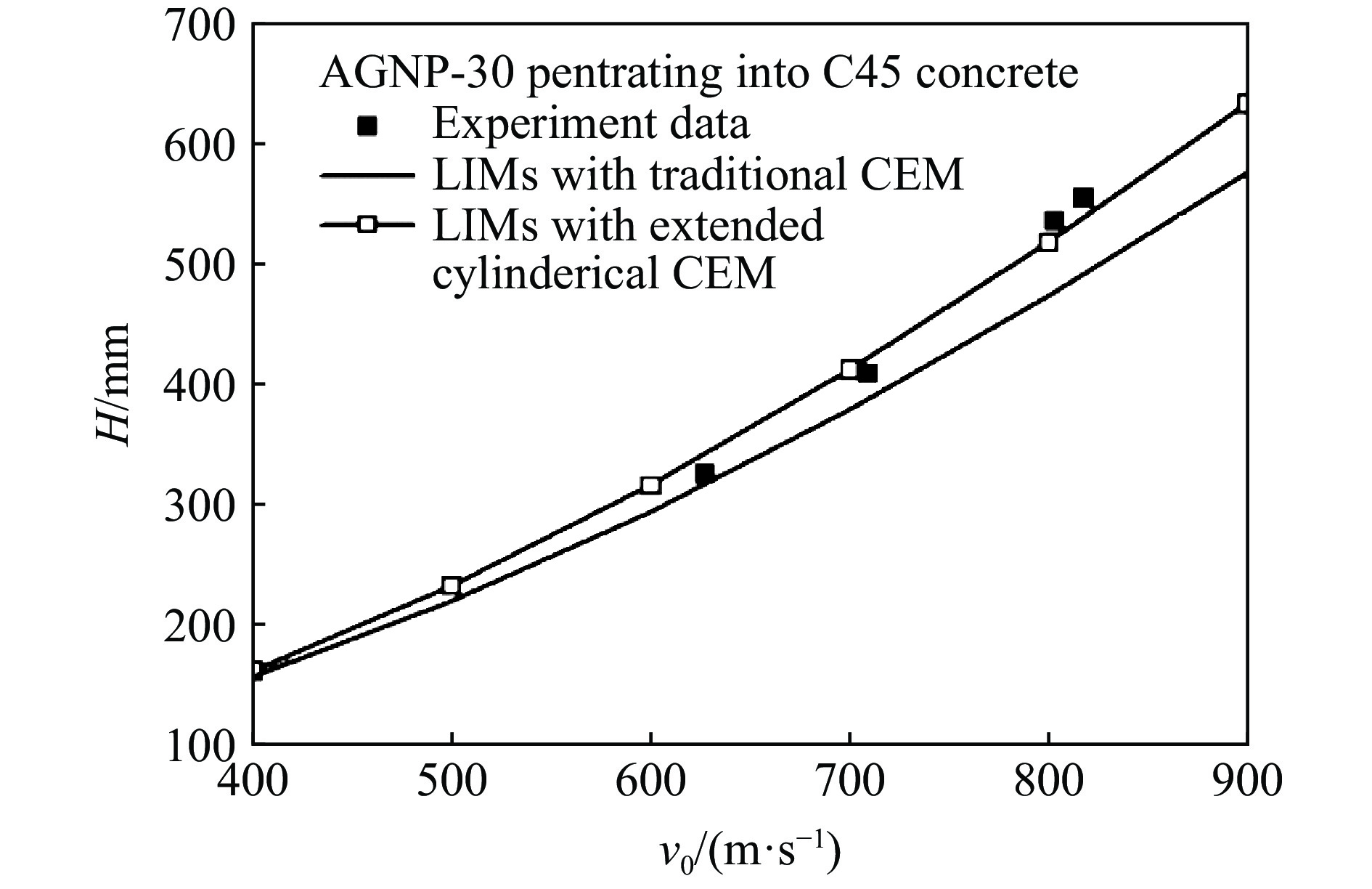
与传统混凝土相比,超高强度混凝土(ultra-high performance cement based composite,UHPCC)具有更加优异的抗侵彻性能[17-18, 39-43],对于高速弹体侵彻UHPCC目标,弹体-目标界面周围的压力可以达到数吉帕,其中压力-体积应变的关系表现出非线性特征,Kong等[44]、Peng等[45]引入了双曲屈服准则和非线性状态方程来描述弹体侵彻下UHPCC材料的塑性行为,提出了一种改进的弹体侵彻UHPCC的动态空腔膨胀模型。图7~8为有关屈服准则和状态方程的实验数据,从图中可以看出,基于线性屈服准则和EOS的经典空腔膨胀模型不适用于UHPCC,双曲屈服准则和非线性EOS分别在拟合抗剪强度-压力数据和压力-体积应变数据时更实用和灵活。
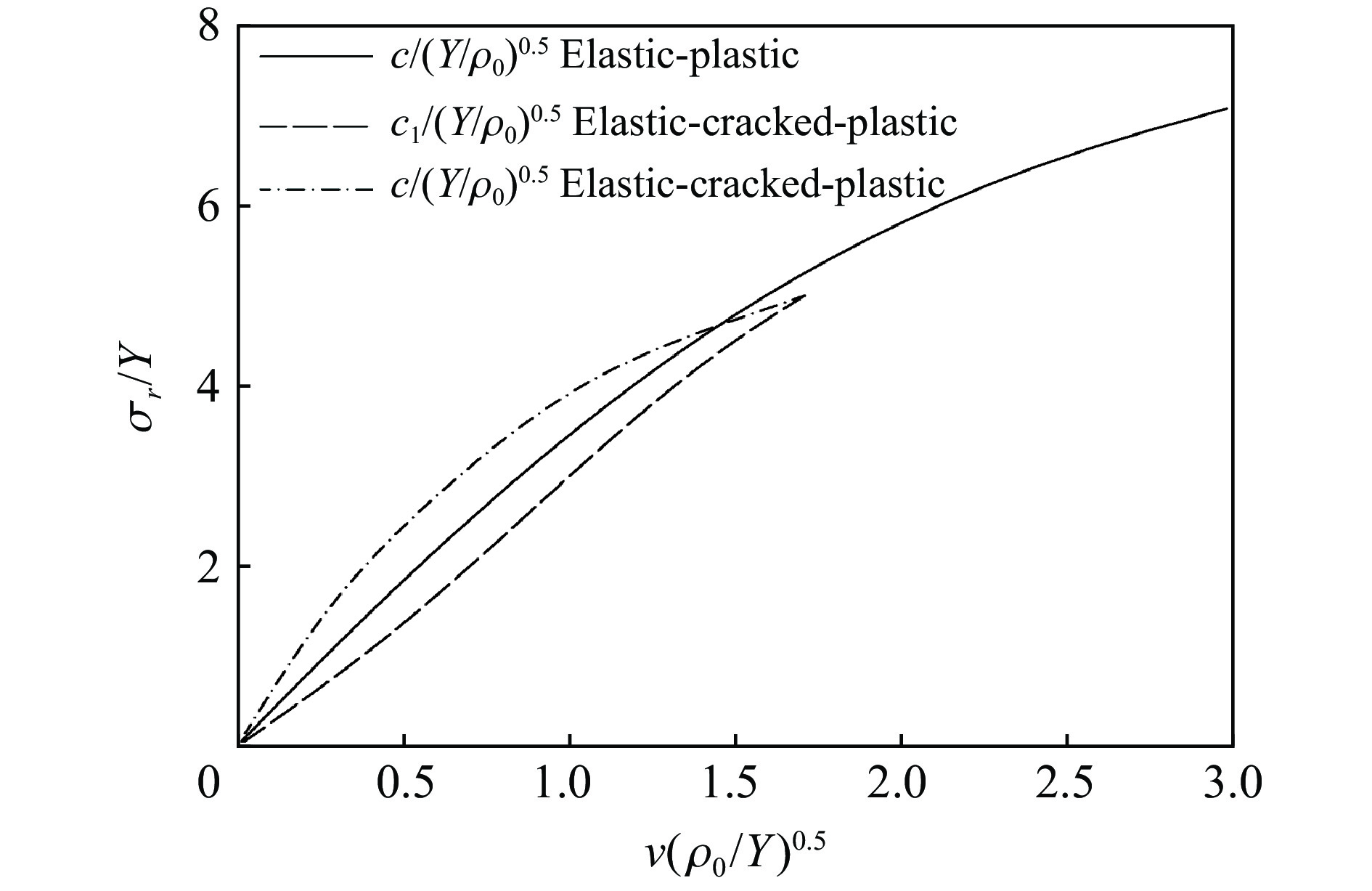
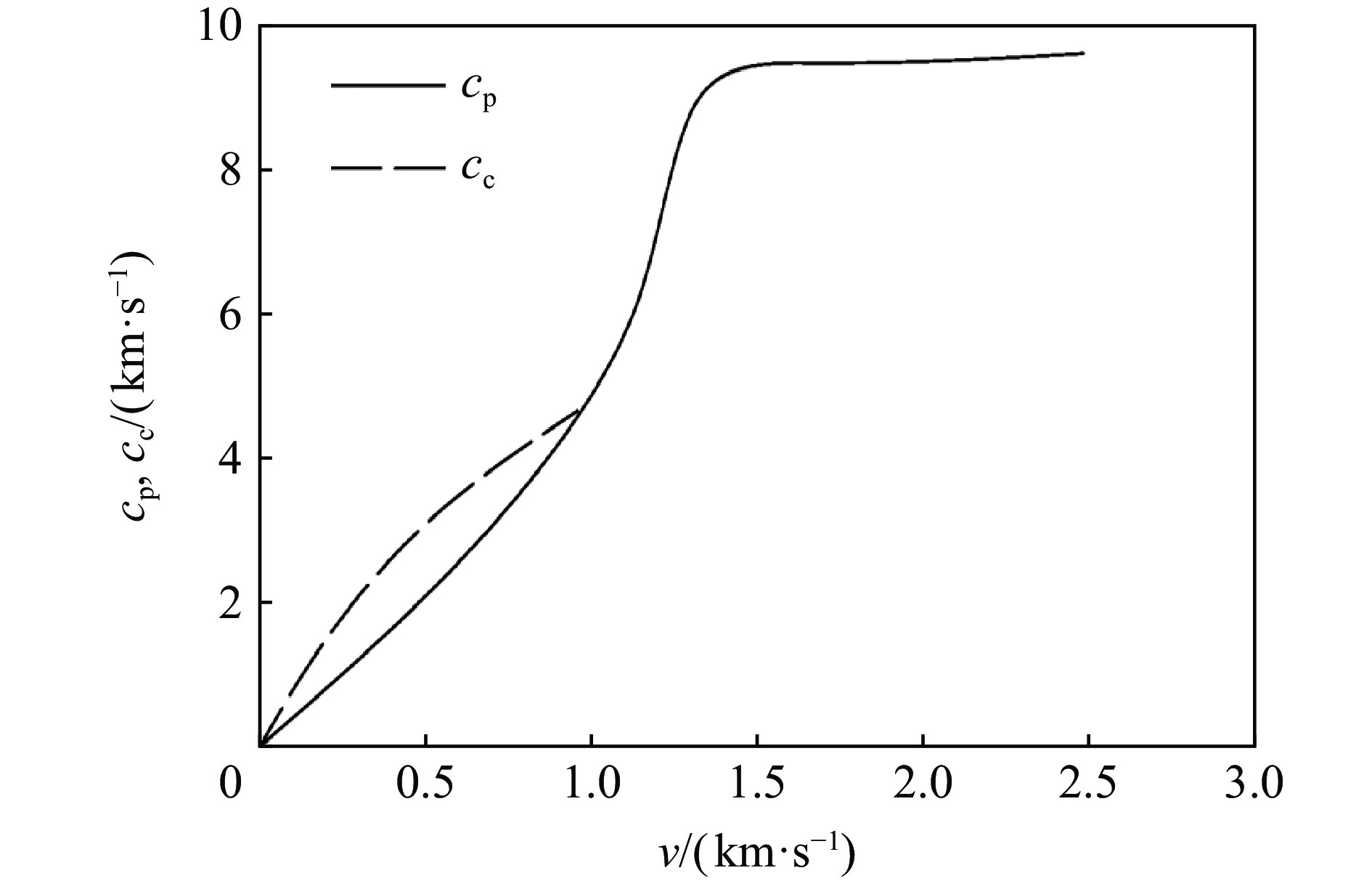
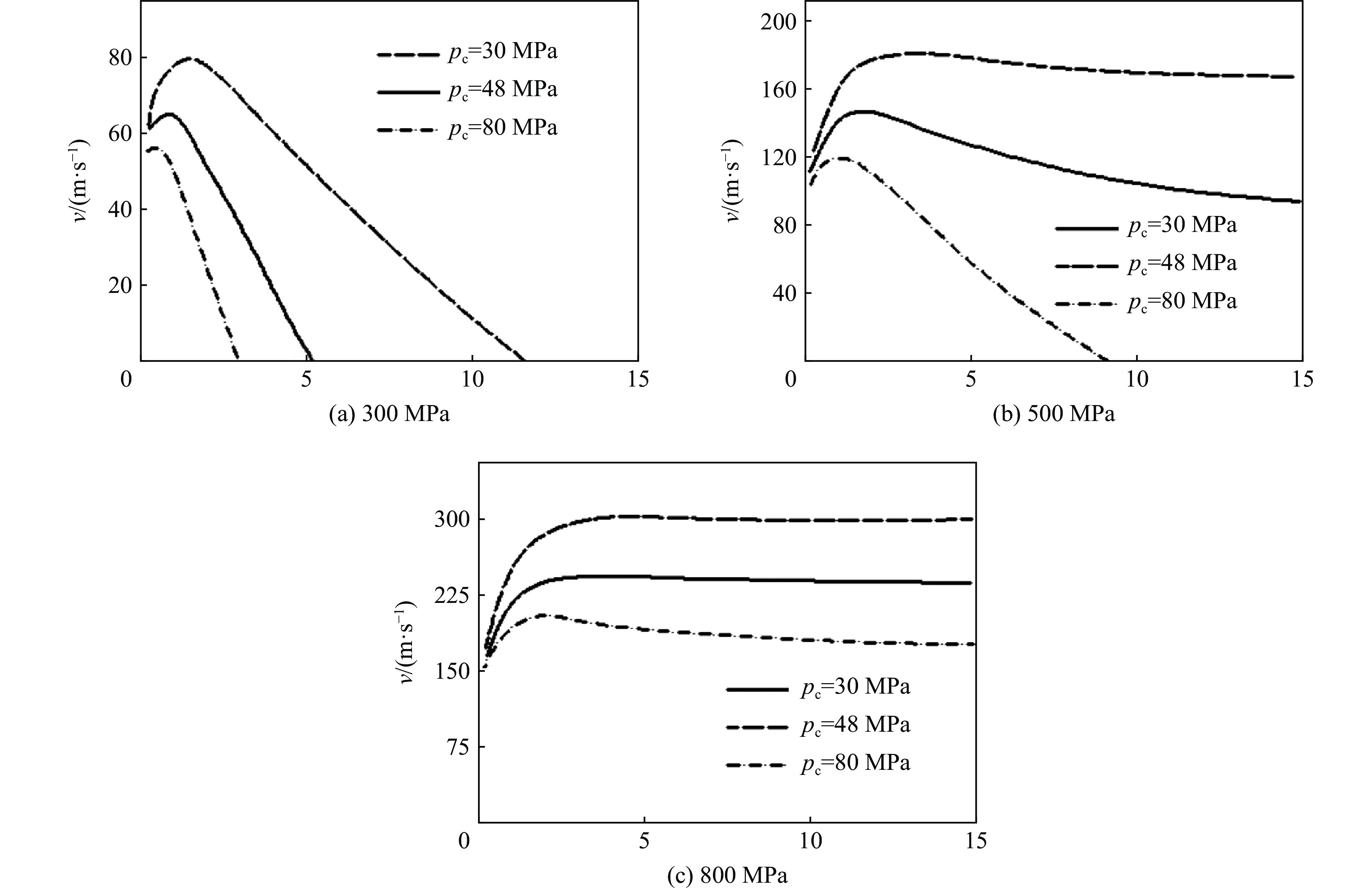
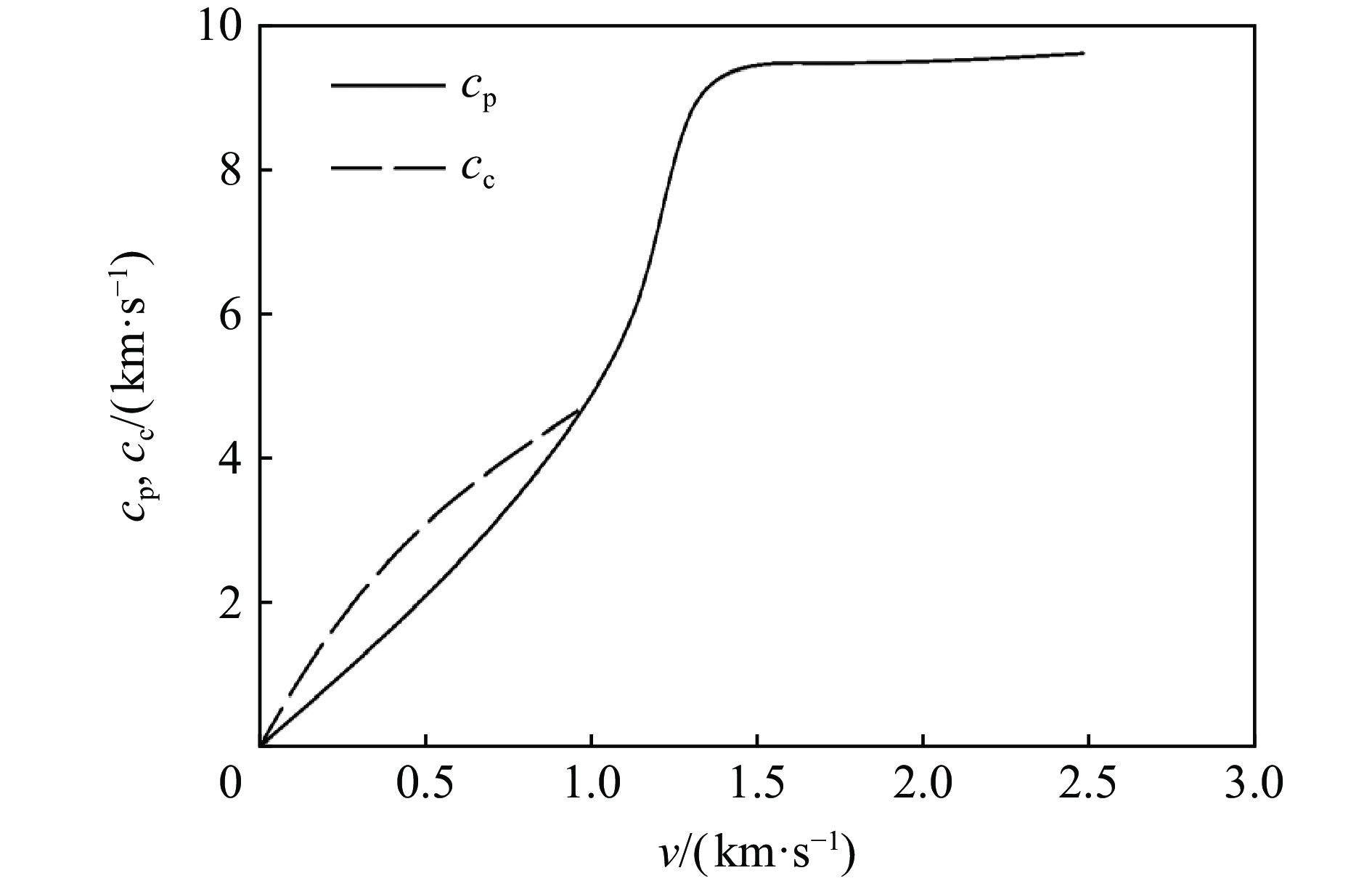
Satapathy[16]研究陶瓷材料以恒定速度打开球形空腔所需的压力时发现,随着空腔膨胀速度逐渐增加,粉碎区边界速度
cp 逐渐逼近并超过破裂区边界速度cc ,即破碎区消失,如图9所示。在很高的空腔膨胀速度下,粉碎区域的传播速度会以略低于纵向波速的水平达到饱和[46]。Hunter等[9]首先将空腔膨胀模型应用于球形弹体对土介质的侵彻问题。其后Hanagud等[47]将其推广至可压缩介质中,Norwood[48]则采用柱形空腔膨胀理论求解了土介质的侵彻问题。Forrestal等[10]将土壤的压力-体积应变理想化为线性关系,采用Morh-Coulomb和Tresca屈服准则描述塑性区,推导了不同剪切强度模型的球形空腔膨胀问题的方程,计算结果如图10所示。Forrestal等[10]的研究表明,不同屈服准则对空腔径向应力有较大影响,随着
λ 的增加,空腔表面径向应力逐渐增大,实际应用中应合理选择描述材料屈服行为的准则。Shi等[38]采用p-α状态状态方程和Mohr-Coulomb-Tresca极限屈服准则来描述砂土的本构关系,建立了考虑砂土可压缩性的球形空腔膨胀模型,图11~12为空腔速度与空腔径向应力、弹塑性界面移动速度的关系,结果表明,对于含孔隙的砂土类材料,忽略可压缩性将高估空腔径向压力。Satapathy[16]在Bless等[49]工作的基础上,将响应区划分为空腔-粉碎-径向裂纹-弹性-无扰动,假设粉碎区材料满足Morh-Coulomb屈服准则,模型预测的结果与实验[50]吻合程度较好。
靶体材料对空腔膨胀模型的影响首先体现在响应区的划分上,材料的力学特性决定了其响应区的复杂性;从简单的塑性-弹性分区到复杂的塑性-密实-孔隙压实-开裂-弹性分区,不同响应分区下计算结果有较大差异,正因为分区的复杂性,应谨慎根据材料破坏特性准确地划分响应分区。
2.2 屈服准则及状态方程
许多学者致力于采用更加准确的塑性区屈服准则描述空腔膨胀模型中塑性区的力学特性。常用的屈服准则有:Mohr-Coulomb屈服准则、Griffith屈服准则、Drucker-Prager盖帽模型、Hoek-Brown准则、统一强度理论、应变梯度塑性流动理论、Voce应变硬化准则等。此外,材料的状态方程从简单的线性压力-体积应变逐渐向非线性多段式状态方程演化,并考虑材料的可压缩性、剪切饱和等。空腔膨胀模型之间主要区别在于对塑性区(粉碎区)本构的描述。总的来讲,材料强度准则、状态方程的不断修正以及考虑响应过程中材料的可压缩性,使得空腔膨胀模型能够更加全面、准确地描述材料的动态响应,但也使得需要求解的参数增加,方程无法得到解析解,计算过程越来越繁琐。常见的屈服准则与状态方程如表1所示。
表 1 常见的几种屈服准则与状态方程Table 1. Several common yield criteria and equations of state屈服准则/状态方程 函数表达式 备注 Mohr-Coulomb |σr−σθ|=Y σr和σθ分别为径向应力与环向应力,Y为屈服强度 Tresca |σr−σθ|=λp+τ0 τ0=3−λ3Y Griffith (σr−σθ)2=Y(σr + σθ) σr和σθ分别为径向应力与环向应力,Y为屈服强度 Drucker-Prager {σr−σθ=λp+τ0p<pmσr−σθ = (τ0 + λpm)p1−pp1−pmpm≤p≤p1σr−σθ = 0p>p1 σr和σθ分别为径向应力与环向应力,
Y为屈服强度,p1、pm为临界压力Hoek-Brown (σr−σθ)2=Y(m0σr + σθ) 无量纲数m0与材料强度及脆性程度有关 统一强度理论 11 + b(σ1+bσ2)−γσ3=σt γ=σt/σc为靶体材料拉压比,σt和σc分别为靶体材料的
抗拉和抗压强度,b为中间主应力的效应参数Voce应变硬化 σ = {Eεσ≤YY+2∑i=1Qi(1−exp(Ciε))σ>Y Qi和Ci为硬化参数 线性压力-体积应变 pm=Kε ε为应变 三段式线性状态方程 pm={Kεp≤pcpc+Kc(μ−μc)pc<p≤p1p1+K1(μ−μp)p>p1 K、Kc、K1为弹性区、孔隙压实区和密实区的
体积模量,pc、p1为临界压力应变率、剪胀性、压力-体积应变等对空腔膨胀模型计算结果的影响主要体现在本构方程上,进而影响模型计算的空腔边界速度、弹塑性边界速度、空腔边界应力等。空腔膨胀模型的复杂性在于其本构模型的选取,众多的本构方程使有关空腔膨胀模型方面的成果十分丰富。不同本构方程代入求解后计算所得空腔边界应力差异较大,需要结合实际情况,合理分析、选取恰当的本构方程描述材料的力学特性。
2.3 空腔膨胀模型的适用性
数十年来,柱形空腔膨胀(cylindrical cavity expansion,CCE)和球形空腔膨胀(spherical cavityexpansion,SCE)模型的研究都在Goodier[51]工作的基础上进行。CCE和SCE模型基于以下两个基本假设:
(1)施加在弹体表面上的接触压力p可以近似为在CCE或SCE模型中扩张空腔所需的压力p0;
(2)空腔膨胀速度
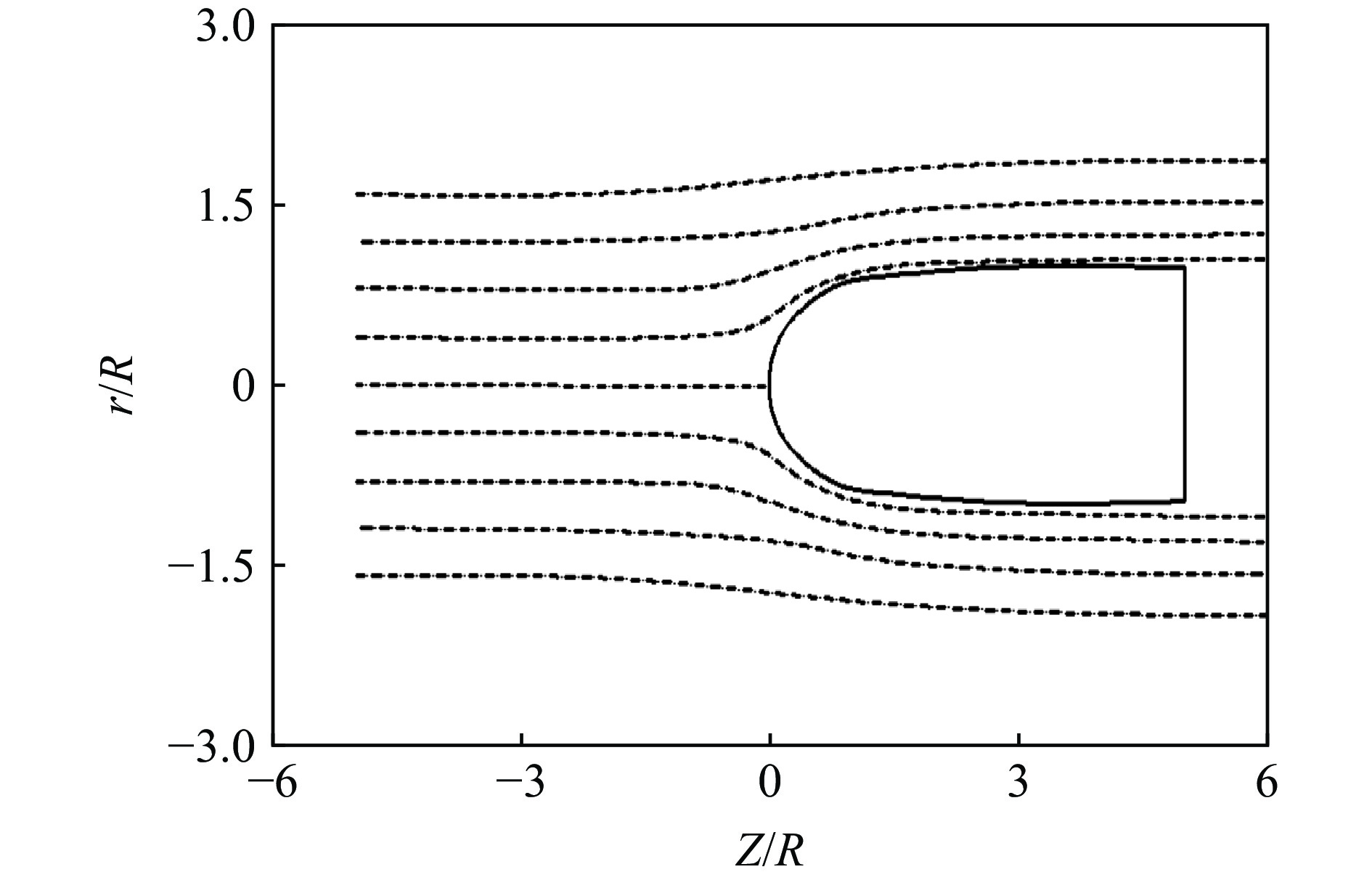
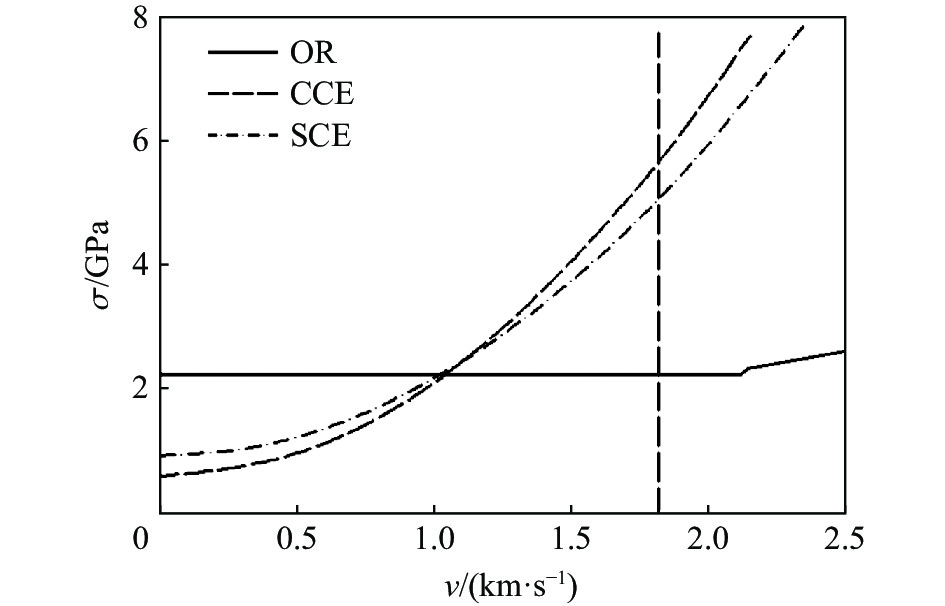
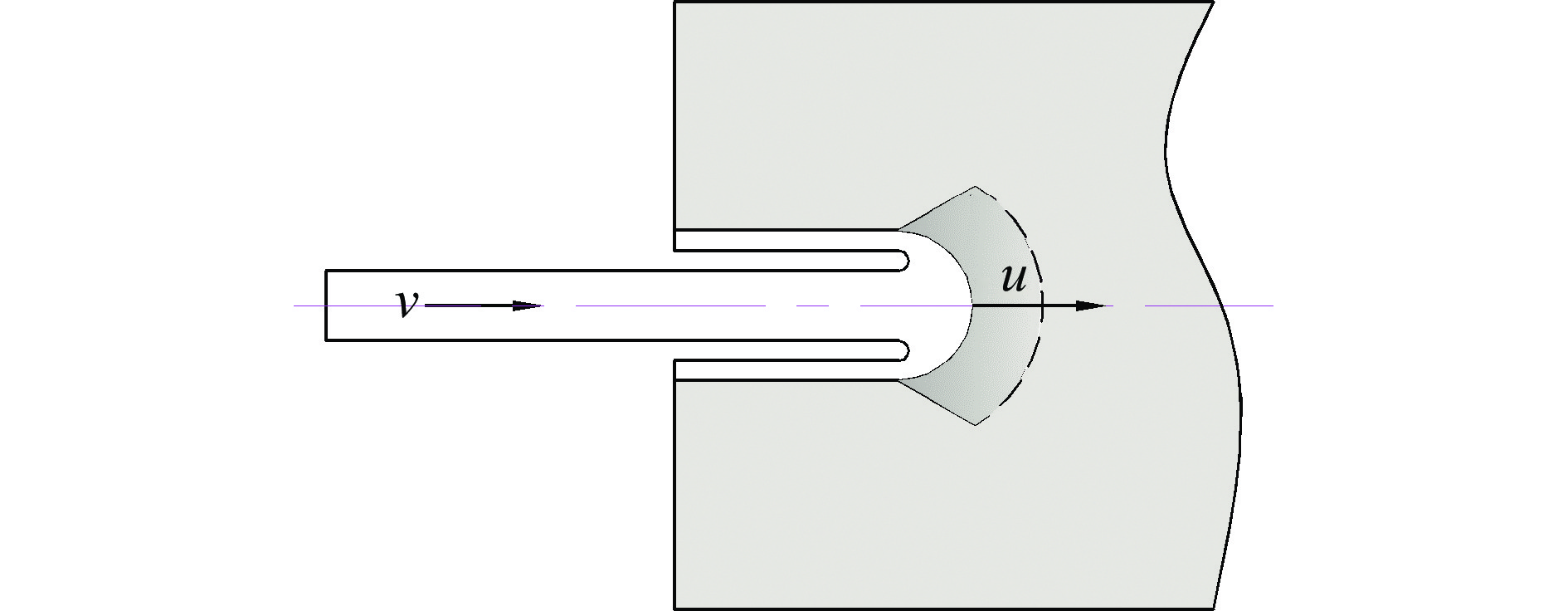
˙a 可由弹体形状以及当前的轴向侵彻速度vz 确定。近年来,一些学者重新讨论了该假设的适用性。Rubin[52]通过使用OR(ovoid of rankine)模型[24, 53]作为基准,与空腔膨胀模型计算结果对比,分析空腔膨胀模型中由于不真实的流场导致的计算侵彻阻力误差。OR模型中的弹体头部形状及稳态流场如图13~14所示,OR模型用于分析刚性弹体侵彻不可压缩的理想弹塑性材料的运动过程,其弹体形状与稳态真实流场一致。Rubin[52]认为CCE和SCE模型均低估了静态阻力值
Rt ,惯性项的存在弥补了部分侵彻阻力预测偏差值。总的来说,空腔膨胀模型对于较低的冲击速度值会低估侵彻阻力,而对于较高的冲击速度值会高估侵彻阻力,如图15所示。Rosenberg等[22-23]、Kawata[54]、Kong等[55]、Yankelevsky等[25-26]、Feldgun等[56]研究表明,在临界速度范围内(空化速度),撞击金属目标的刚性弹体上的侵彻阻力实际上是恒定的,而不是速度依赖性的,当速度超过了临界速度,则必须将惯性项加入到侵彻阻力中。恒定的侵彻阻力
Rt 取决于材料的特性(屈服强度和杨氏模量)以及弹头的头部形状。即只要侵彻隧道区直径与弹体直径相同,可以认为施加在弹体轴向方向的侵彻阻力是恒定的,侵彻深度可以由下式得出:P=ρpLeffv20/2Rt (11) 式中:
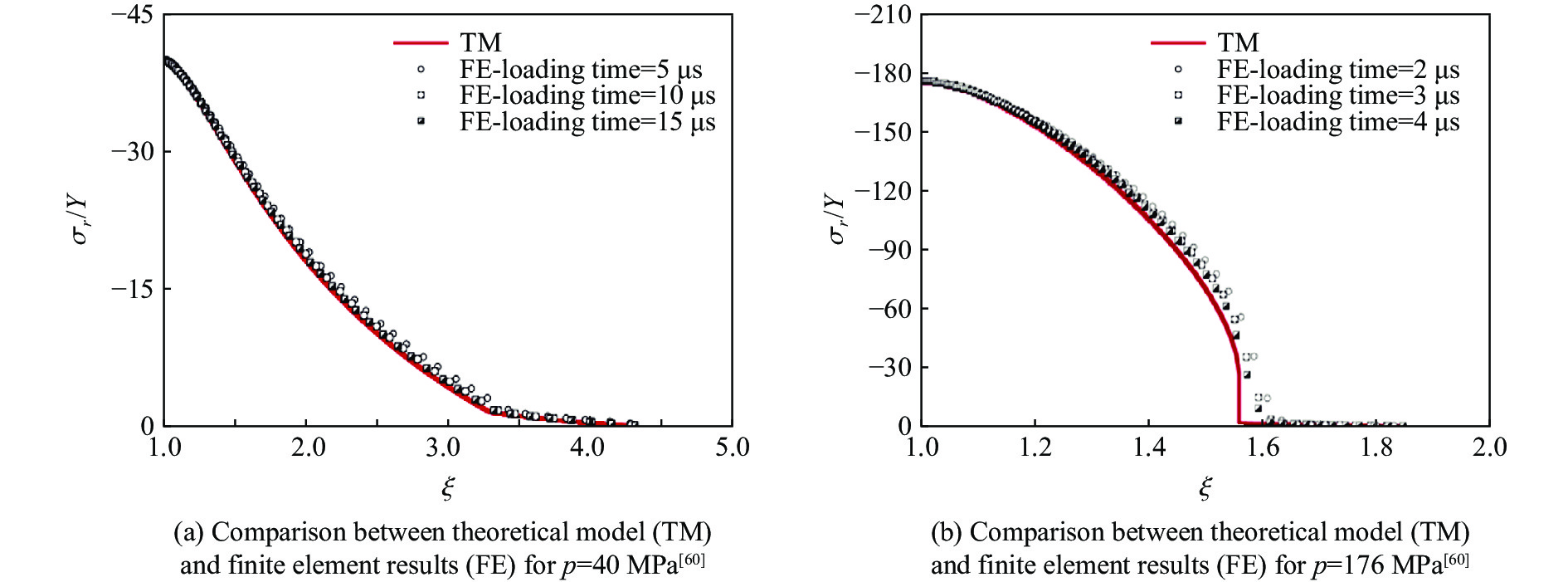
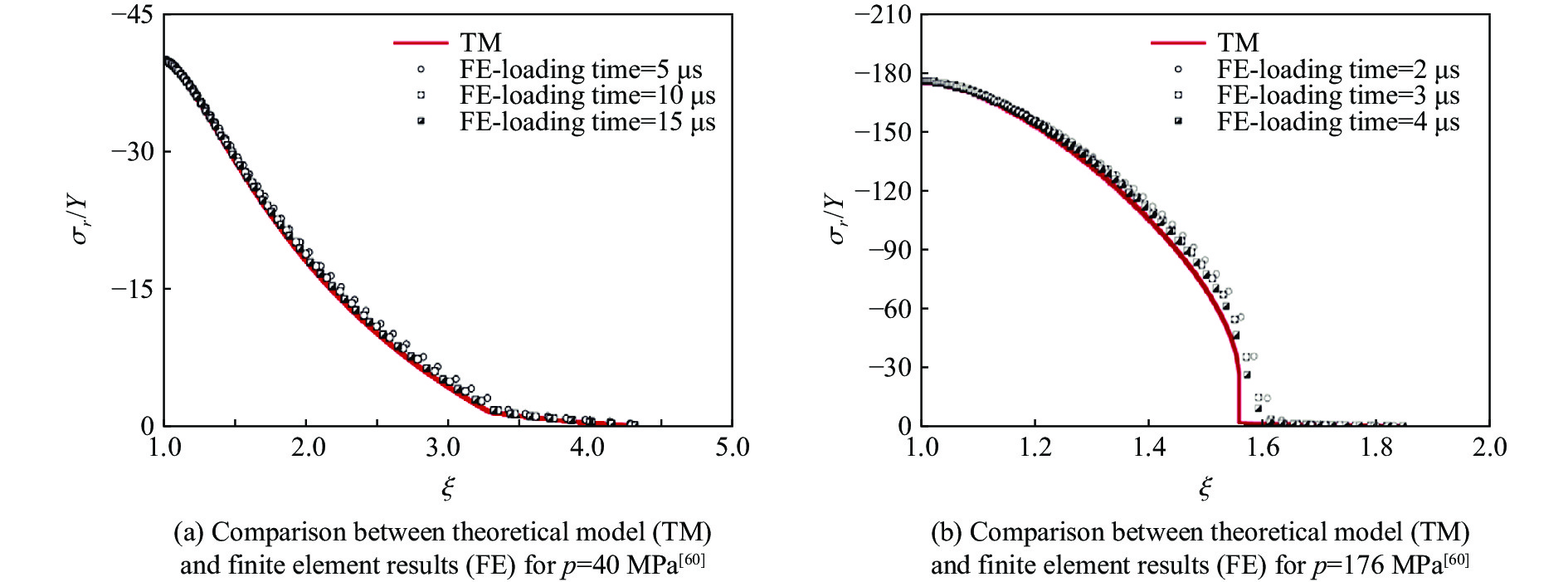
ρp 是弹体密度,Leff 为弹体有效长度,v0 为侵彻初始速度。动态空腔膨胀模型普遍采用相似变换求解[57-59],理论求解一般不考虑材料的瞬态响应行为,以获得稳定的自相似响应。近年来,Rodríguez等[60]、Masri等[61]研究了自相似稳态场的时间尺度,利用数值模拟方法分析了空腔膨胀初始阶段材料的瞬态响应问题。图16为不同时间下空腔边界应力的数值模拟与理论计算值对比,从图中可以看出,随着时间的增加,数值模拟结果逐渐靠近理论解。Rodríguez等[60]研究表明,相似变换所假设的自相似稳态场存在,达到自相似稳态场的时间在2.5~40 µs (与材料有关)。因此当采用空腔膨胀模型求解薄板侵彻问题时,由于自相似稳态场的形成需要时间,应慎重考虑采用空腔膨胀模型求解的合理性。
侵彻模型(如Poncelet模型、Recht-Ipson模型、A-T模型、空腔膨胀模型、Ravid-Bodner模型和Walker-Anderson模型等)各有其假设与适用条件[62]。尽管空腔膨胀模型存在着诸如无法预测空化现象、模型流场与实际有误差等问题,但其仍然是侵彻问题研究中重要的分析手段。
3. 靶体空腔膨胀阻力的数值模拟方法
空腔膨胀模型的中心思想是为了求得空腔表面径向应力与膨胀速度的关系,传统的空腔膨胀理论研究方法均是基于理论分析和推导,然后进行大量的理论计算,但面对复杂的本构条件、屈服准则以及状态方程时则遇到了瓶颈。利用数值模拟研究空腔膨胀过程是十分有效的, 数值模拟可以代替模型中某些复杂的理论推导部分, 根据模拟结果可以拟合得到空腔表面径向应力与膨胀速度的关系。
3.1 空腔表面恒定速度数值模拟
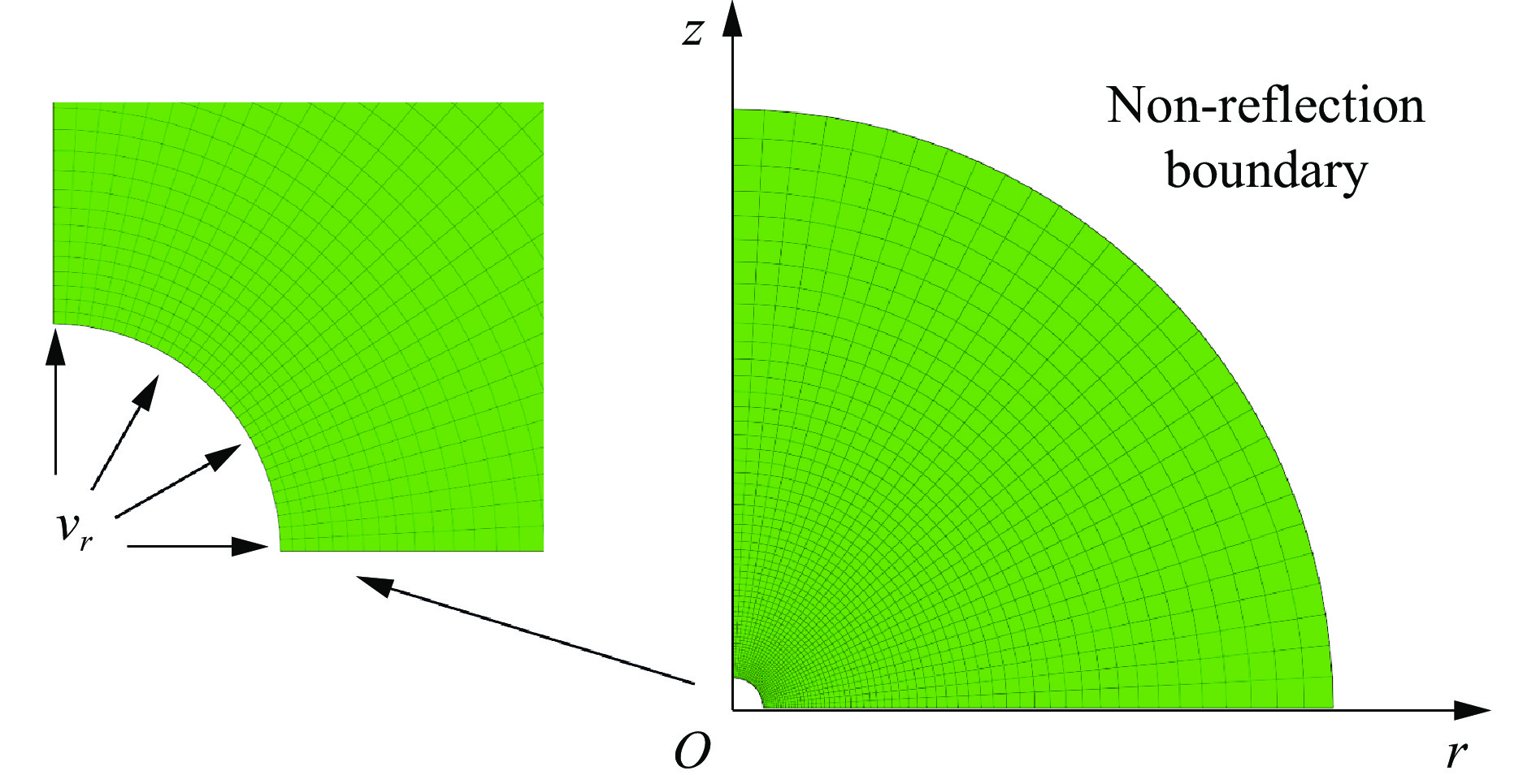
Warren等[63]在研究混凝土的侵彻问题时采用了数值模拟的方法来确定球形空腔表面应力,有限元模型与计算结果如图17~18所示。模拟假设空腔表面以恒定速度向外扩展,当材料响应趋于稳定时,空腔表面的应力也将趋于一个恒定值。通过改变空腔的膨胀速度,经过一系列的模拟,可以得到径向应力与空腔膨胀速度的拟合关系式。
何涛等[5]分别采用轴对称单元和平面应变单元来模拟典型靶体球形空腔膨胀和柱形空腔膨胀作用过程,如图19~20。模型中靶板材料采用双线性本构,模拟结果与可压缩的理想弹塑性解接近,表明采用有限元数值模拟方法可以较为准确地计算出空腔膨胀响应力结果。
Rosenberg等[59, 64]对半无限理想弹塑性金属材料进行了一系列的研究,通过材料参数的单因素影响模拟研究,结合理论分析,得到了球形空腔径向应力系数与弹塑性材料基本参数的表达式。对于任意的弹塑性材料,均可由表达式计算出径向应力,误差不超过5%,说明数值模拟可以直接反映出简单本构中各参数的联系,增强了数值模拟的适用性。
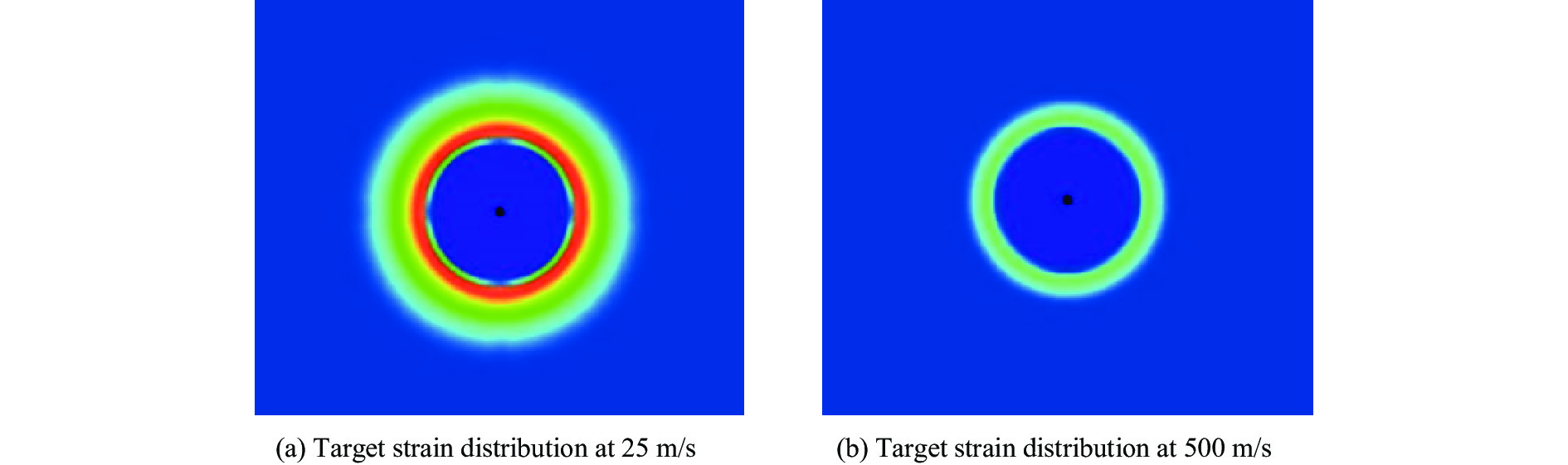
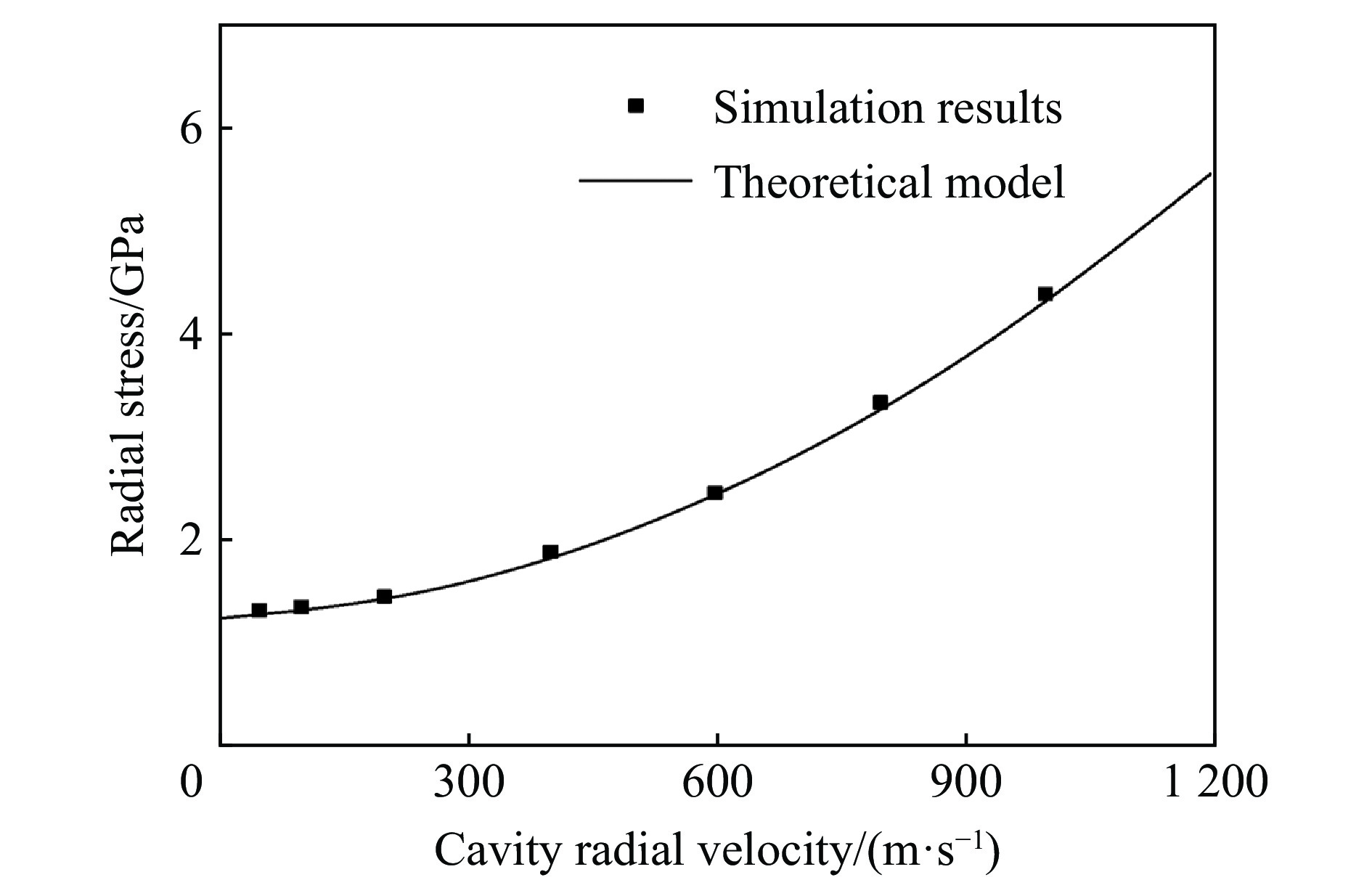
图21是长杆弹以2 km/s的速度撞击材料和空腔以2 km/s的速度压缩材料时材料的速度分布图。两幅图中的颜色代表材料的流动速度,且具有相同的标尺,即颜色相同代表流动速度相同。从图中可以看出,弹坑和空腔的形状以及他们周围的材料速度分布非常相似,证明了模拟方法的有效性。
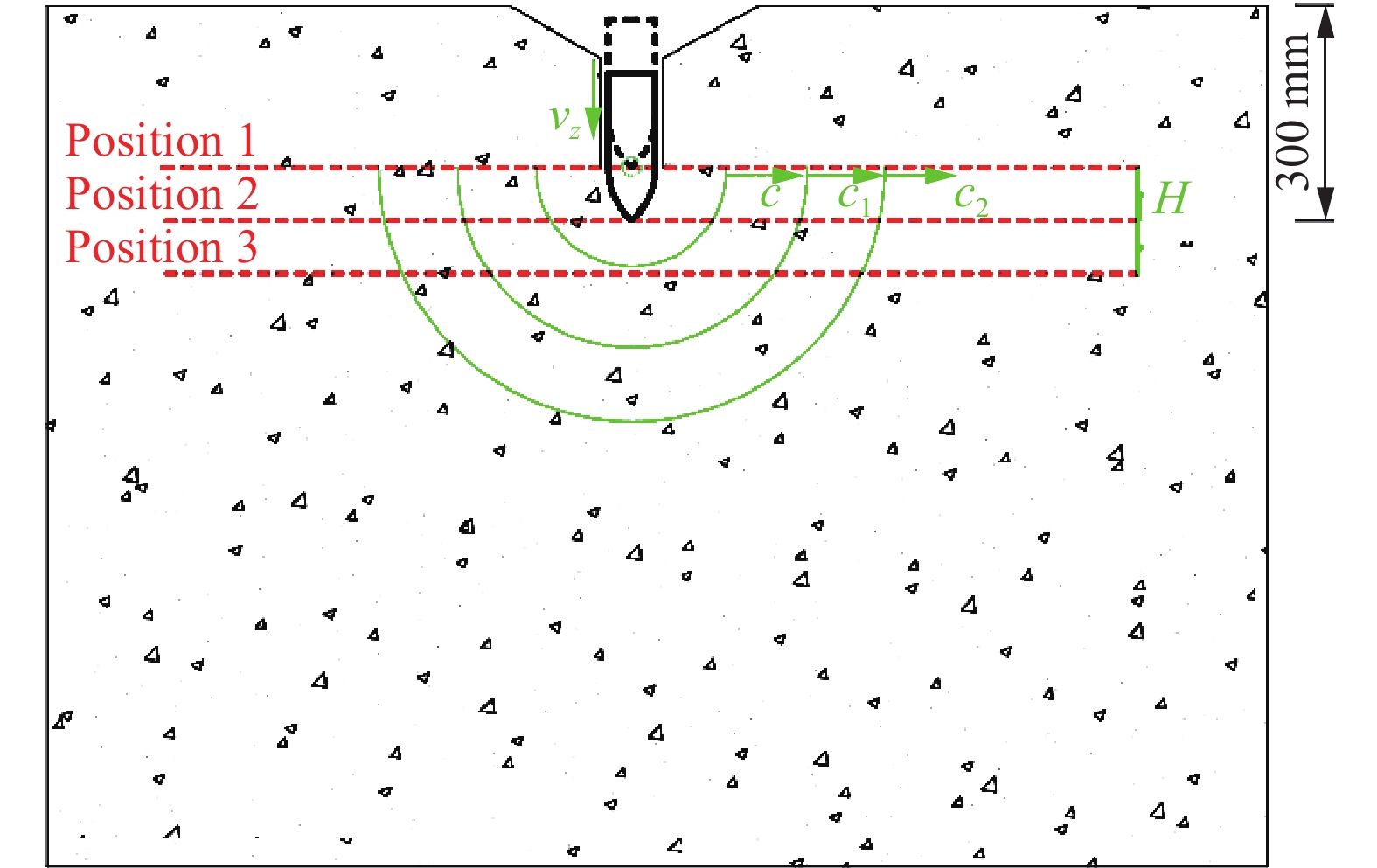
前人大部分工作集中在膨胀速度恒定的条件下得出各区域的大小,而实际情况中空腔膨胀速度是不断变化的。牛振坤等[65]采用刚性弹侵彻混凝土,研究靶体材料各响应区域的大小,并讨论侵彻速度(空腔膨胀速度)对混凝土各响应区域的影响。图22~23表明,随着弹体侵彻速度的增高,混凝土粉碎区和破裂区边界速度也随之增高。
3.2 空腔表面恒定压力数值模拟
王一楠等[66]、晋小超等[67]等通过改变数值模拟中材料的失效判据,对侵彻过程中金属、混凝土靶空腔膨胀响应区域进行了识别划分,得到了侵彻过程中混凝土各响应区的区域大小。
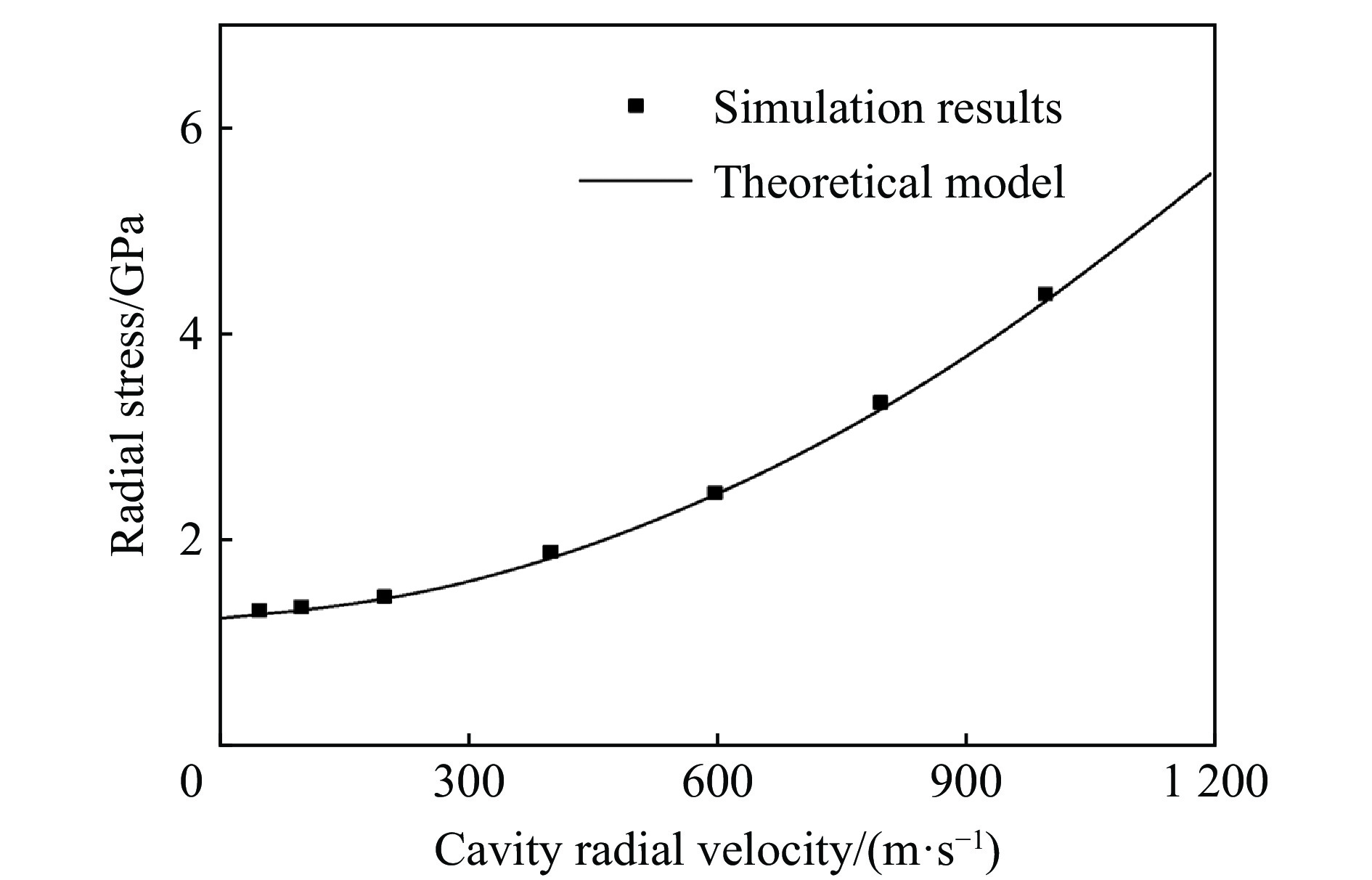
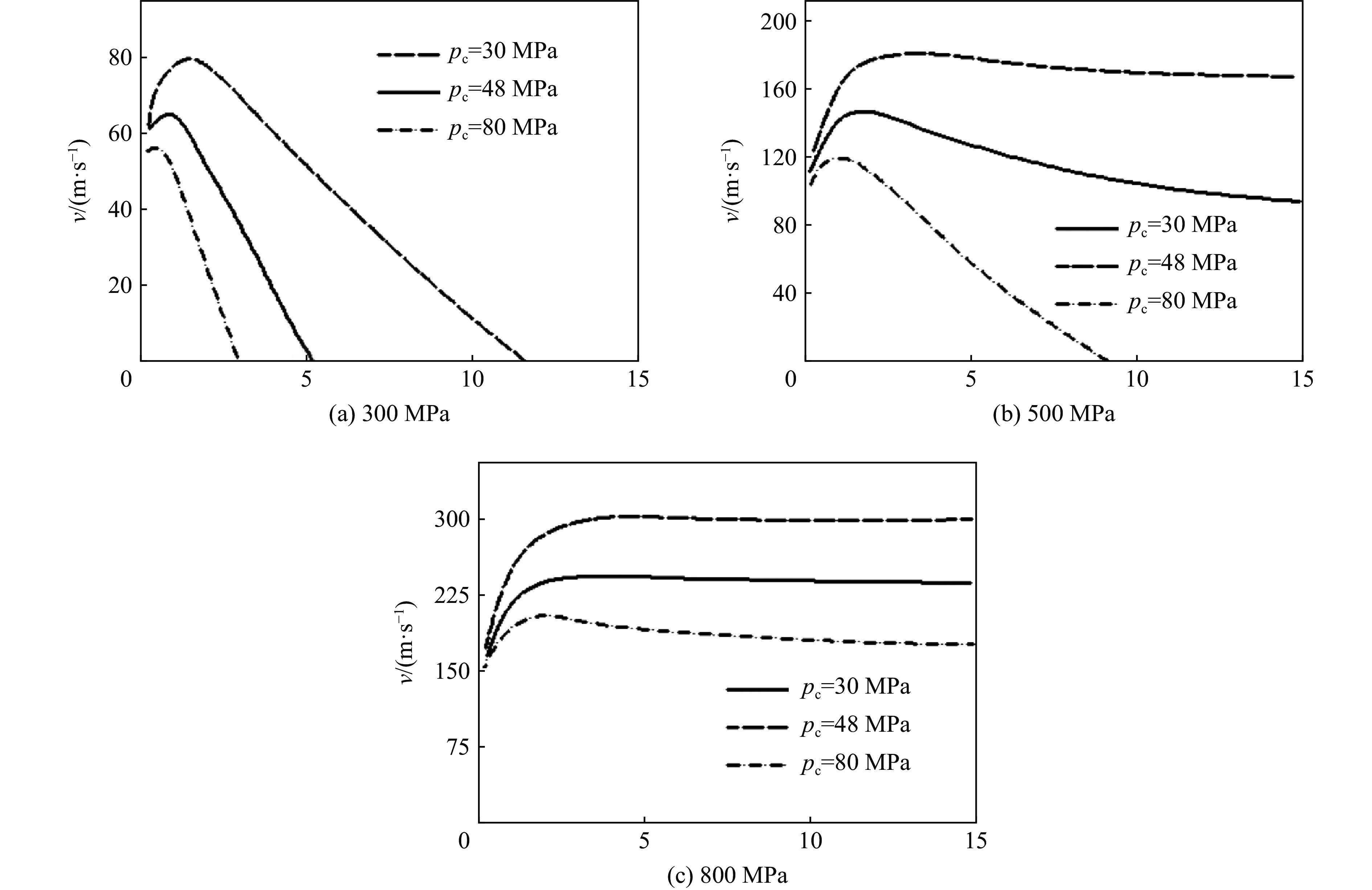
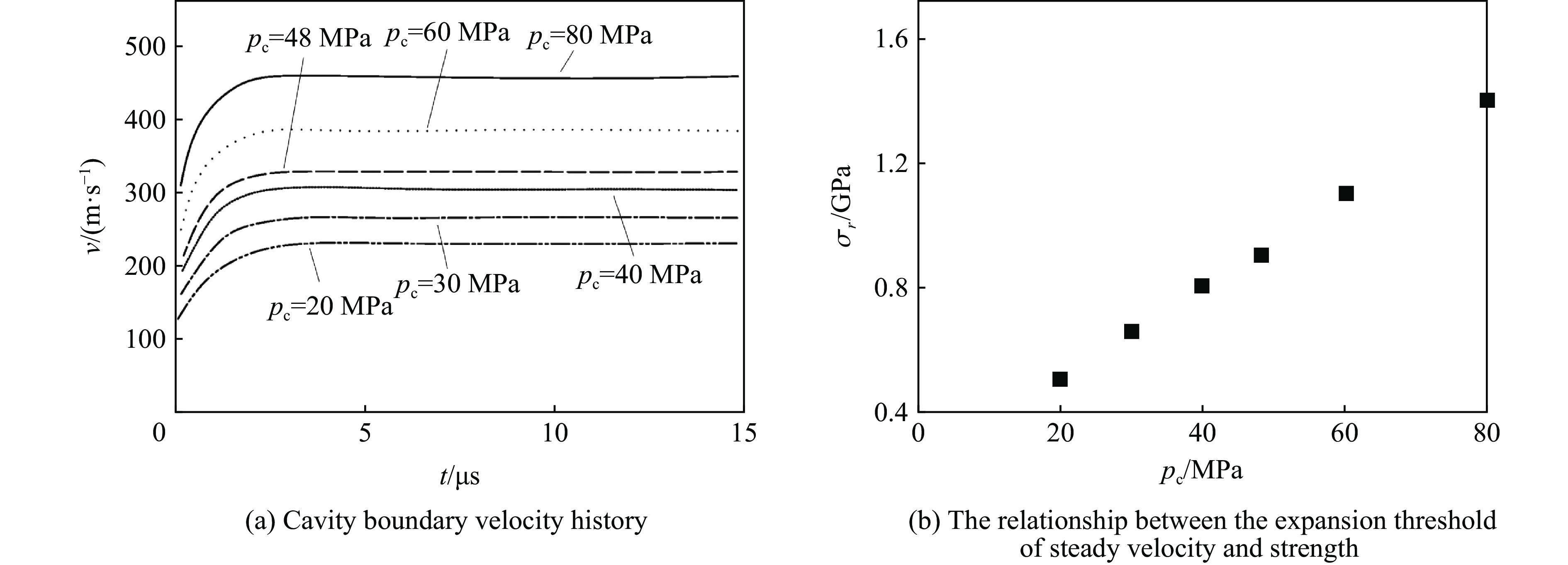
图24~25为模型计算结果,该结果表明,在达到一定膨胀压力后,空腔边界将以对应于膨胀压力的恒定速度膨胀,证明存在理论所示的函数关系;进一步分析表明,空腔边界以恒定速度膨胀所需要的膨胀压力阈值与混凝土的抗压强度基本呈线性关系。
采用数值模拟方法可以比较容易地确定出空腔膨胀时径向应力的大小,从而避免繁琐的理论推导过程,同时充分考虑了材料在空腔膨胀过程中的实际响应情况。另一方面,数值模拟手段可以应用到各种复杂的本构材料中,为解决具有复杂力学响应特点的靶体材料的空腔膨胀作用过程提供了一个有效的模拟方法。
目前空腔膨胀的数值模拟工作集中在空腔表面单元法线方向施加恒定的速度以研究单元膨胀过程压力,或在单元法线方向施加恒定压力载荷以研究单元膨胀速度,主要针对理想侵彻条件的稳定侵彻阶段,即均匀扩孔速度对应于恒定空腔膨胀压力;尚未见到有限空腔膨胀的数值模拟,其原因是由于径向的弹性位移受到限制,有限空腔膨胀的均匀扩孔速度不对应于恒定空腔膨胀压力[68]。
4. 非理想侵彻条件的空腔膨胀压力计算模型
空腔膨胀模型将弹靶理想化,一般假设为柱形、球形弹体侵彻均质无限厚靶体。随着战斗部搭载平台的演变、弹体结构设计和防护工程等技术的不断发展,弹靶条件变得复杂,经典空腔膨胀模型适用性降低,需要针对经典空腔膨胀模型进行改进。空腔膨胀阻力模型在多层复合靶板、间隔靶板、约束靶体、弹体刻槽和异形截面形状弹体等弹靶条件下的应用问题成为了一个重要的研究方向。
4.1 简单多层复合靶体的空腔膨胀模型
一维半无限柱形/球形空腔膨胀模型中,空腔径向压力完全取决于材料自身的属性,而实际应用中会涉及到具有有限几何尺寸的防护结构。研究表明[69],有限边界的存在会严重影响防护结构的抗侵彻能力,将空腔膨胀模型推广到复杂靶体结构,特别是多层复合靶体是侵彻力学研究者近期关注的热点之一[70]。
Satapathy[71]分析了陶瓷材料铺覆在半无限金属材料上的抗侵彻阻力特性,假定横向尺寸是无限的,可以忽略有限的横向边界的影响。针对不同的边界条件,提出了如图26的4种不同的响应分区。
4种响应求解过程类似,在此列举图26(a)所示响应区的求解过程,此时陶瓷材料具有4个区域:空腔、粉碎、破裂和弹性,金属基底是弹性的。不同区域的解可以通过空腔膨胀模型求得(
σr 即为空腔表面径向应力):{σr=Y{3YE[1−hahc(1−ν)+hahc3σfE(1−ν)]}−2α/3(hbhc)3=6K(μm/μ−1)4μm+3K[(ha/hc)2+σf/Y(ha/hc)2−2σf/Y](hha)3=3YE[1−hahc(1−ν)+hcha3σfE(1−ν)] (12) 式中:ha、hb、hc、hd、h为分区常数,
σf 为屈服极限,μ 为拉梅常数,μm 为金属的剪切模量。Satapathy[71]的研究表明,对于侵彻体尖端至边界距离大于15倍空腔直径的情况,可以忽略有限边界的影响;当该比值小于10时,必须考虑边界效应。
4.2 给定载荷下任意截面轮廓空腔边界应力分析
在一维空腔膨胀理论方面已有大量的研究成果,但在任意截面轮廓空腔膨胀理论的研究方面却鲜有报道。任意截面轮廓空腔膨胀理论面临的主要问题是确定将塑性区与弹性区域分开的轮廓(弹塑性边界),以使应力和位移在整个边界两侧都是连续的函数,一旦弹塑性边界确定,模型就可以简化为纯弹性区与纯塑性区。
Woo[72]依据弹塑性动力学理论推导了任意截面形状的柱形空腔膨胀模型,靶体材料分为空腔区、弹性区和塑性区。通过应力状态判断靶体介质的屈服状态及介质所处的空腔膨胀模型分区位置。利用最小二乘法拟合与迭代法最终可得到椭圆形空腔膨胀过程中边界径向应力与速度的关系:
σn=A1K+A2ρtv2n (13) 式中:A1的值取为1.422,K和
ρt 分别为靶体材料体积模量和密度,A2是与椭圆截面弹体横截面尺寸有关的参数。任意截面轮廓空腔膨胀模型与传统空腔膨胀模型所求得的弹靶接触面阻力函数形式类似,都表示为静阻力项和惯性项之和,且惯性项都表示为速度的二次方函数。但不同的是,传统空腔模型中静阻力项与材料的无围抗压强度有关,而任意截面轮廓空腔模型中静阻力项与材料的体积模量相关。
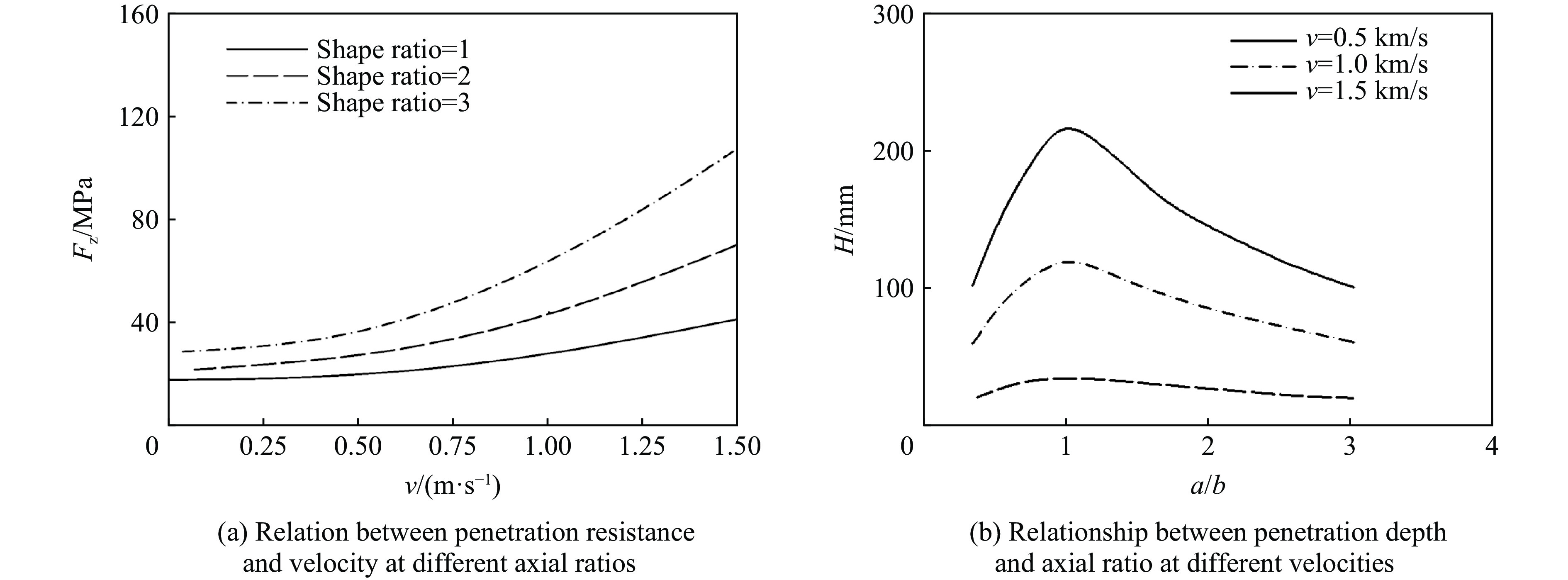
Woo[72]结合阻力函数建立了侵彻动力学模型,进一步分析了椭圆长短轴比对侵彻阻力、深度的影响规律,计算结果如图27所示。研究表明,侵彻阻力与长短轴比呈正相关,长短轴比越大,侵彻阻力越大、侵彻深度越小。
4.3 椭圆空腔膨胀过程中空腔边界应力分析
随着弹靶侵彻问题研究的不断深入,优化后的弹体形状并不都是传统的圆截面,侵彻过程中靶内所形成的空腔也并不都是传统的球形和柱形。
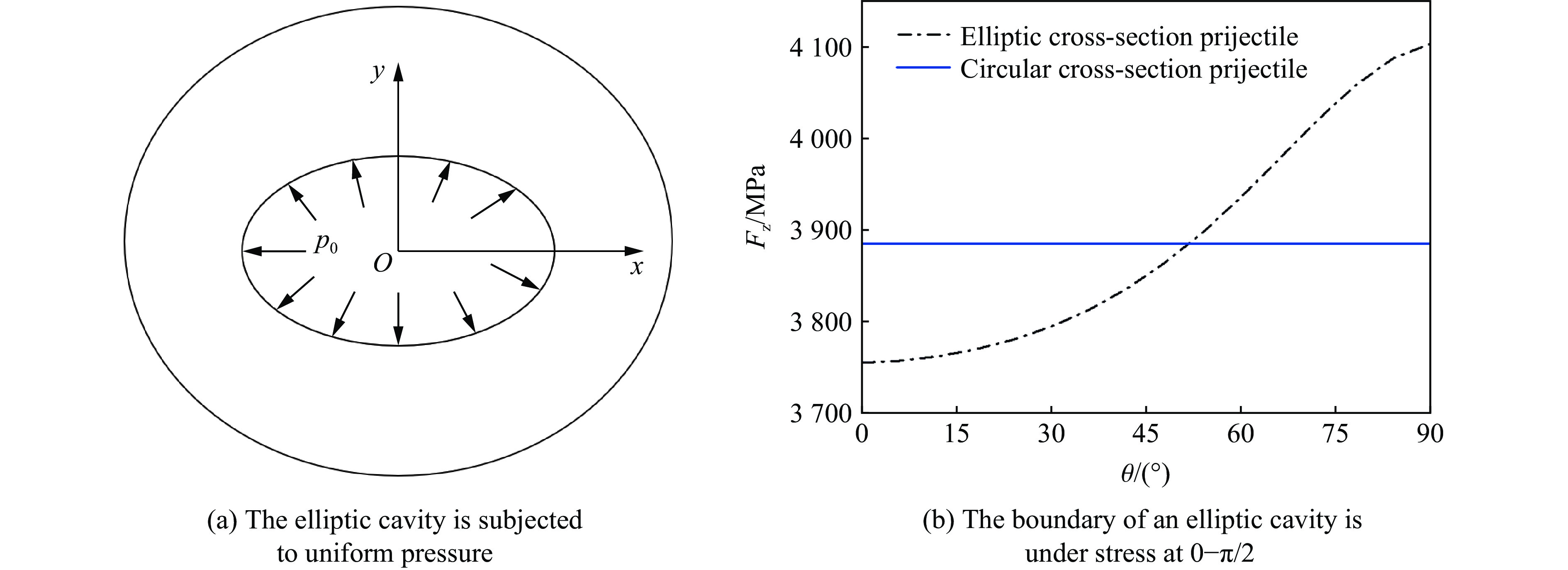
为了描述椭圆截面弹体侵彻过程中椭圆孔洞的扩张过程,王文杰等[73]采用静态柱形空腔膨胀理论,通过假设靶体材料为理想线弹性材料,计算了椭圆孔口周围的受力状态,模型示意图如图28所示。
忽略分布在物体体积内的力,例如重力和惯性力等,弹性力学平面问题归结于求解双调和方程:
∇4U=0 (14) 通过引入复变数,利用保角变换,加上应力边界条件,最终求得复势函数φ(ξ)和ψ(ξ)。将求得的φ(ξ)和ψ(ξ)代入应力函数便可求得椭圆空腔扩张过程中孔周应力分布情况:
φ(ζ)=−12πi(3−4υ)∫f0(σ)σ−ζdσ (15) 3−4υ2πiψ(ξ)=−12πi∫ˉf0(ˉσ)σ−ξdσ−ξmξ2+1ξ2−mφ′(ξ) (16) 当椭圆空腔受均匀压力向外扩张时,图29为计算所得椭圆空腔内壁在
0∼π/2 上的受力曲线。由图29可知,椭圆空腔内壁受均匀压力向外膨胀时,空腔长轴端点处受力最大,而在短轴端点处受力最小。4.4 考虑轴向压缩-切向剪切联合作用下的空腔膨胀模型
基于混凝土材料抗压不抗剪的特点,邓佳杰等[4]提出一种通过头部刻槽实现非对称结构形式的弹体。该类型弹体在侵彻过程中可改变传统结构弹体侵彻单一压缩破坏混凝土的模式,实现较优的弹体侵彻毁伤能力。
假设在头部非对称刻槽弹体侵彻作用下,混凝土材料中出现柱形空腔,空腔分区示意图如图30所示。此时,柱形空腔面以速度
vc 及旋转角速度ω 由初始零半径向外径向膨胀,混凝土材料在受径向应力的同时在各响应区间产生周向旋转作用,材料受力表现为轴向压缩及切向剪切联合作用力,此时柱坐标下的空腔膨胀过程为二维受力状态。假设切向位移
uθ 与径向位移ur 满足线性比例关系,uθ=kur ,且旋转角速度ω 与径向速度vc 同时满足ω=kvc 。在考虑切向位移的情况下,屈服面半径为Cs ,常数C1 、C2 的表达式为:C1=YGCs√12(1+k2),C2=kYGCs√12(1+k2) (17) 结合塑性区的屈服条件,得到考虑剪切效应的靶体空腔膨胀径向应力随空腔膨胀过程中空腔半径间的关系式:
σr=−4Y√12(1+k2)[−1+ln(√12(1+k2)G−Y√12(1+k2)G)YG] (18) 计算
k=0 与k≠0 的径向应力值,可得出剪切效应弱化靶体响应力的无量纲因子S∗ :S∗=ln[(√12(1+k2)Gfc−f2c/√12G4(1+k2))]−1√(1+k2)[ln((√12Gfc−f2c)/√12G4)−1] (19) 式中:
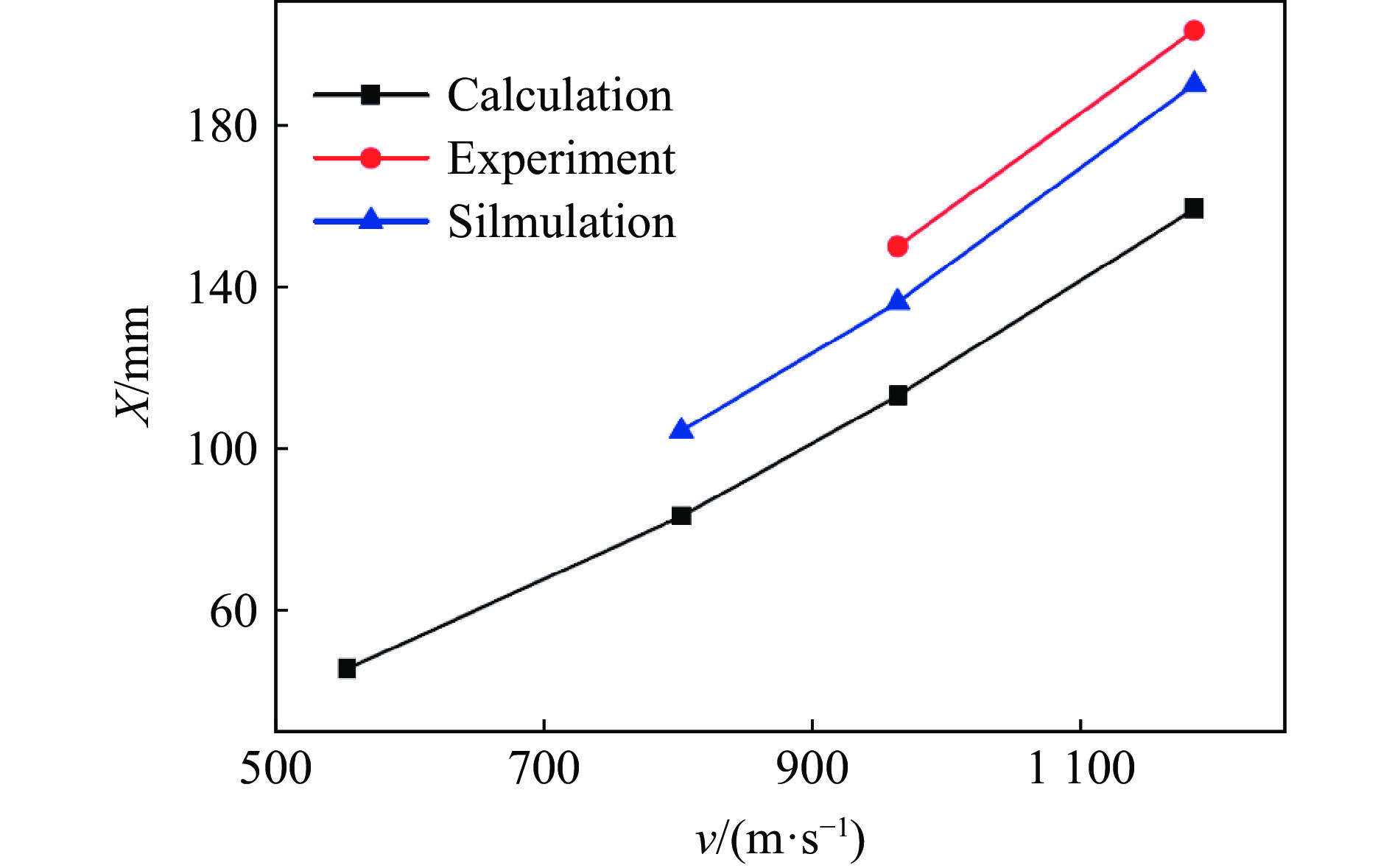
fc 为材料的抗压强度。如图31所示,考虑剪切效应的二维空腔膨胀理论及局部相互作用模型的理论计算结果与实验结果吻合较好。
4.5 有限/约束靶体的空腔膨胀理论
对于斜侵彻和有限尺寸金属靶侵彻问题,自由边界的影响不可忽略。Littlefield等[74]运用实验、近似解析解和数值模拟相结合的方法对有限直径金属厚靶侧面自由边界的影响进行了探讨,结果表明:自由边界和靶体直径对侵彻模式和侵彻深度有显著影响,当靶体与弹体直径的比值小于20时,靶体的侵彻阻力急剧降低。
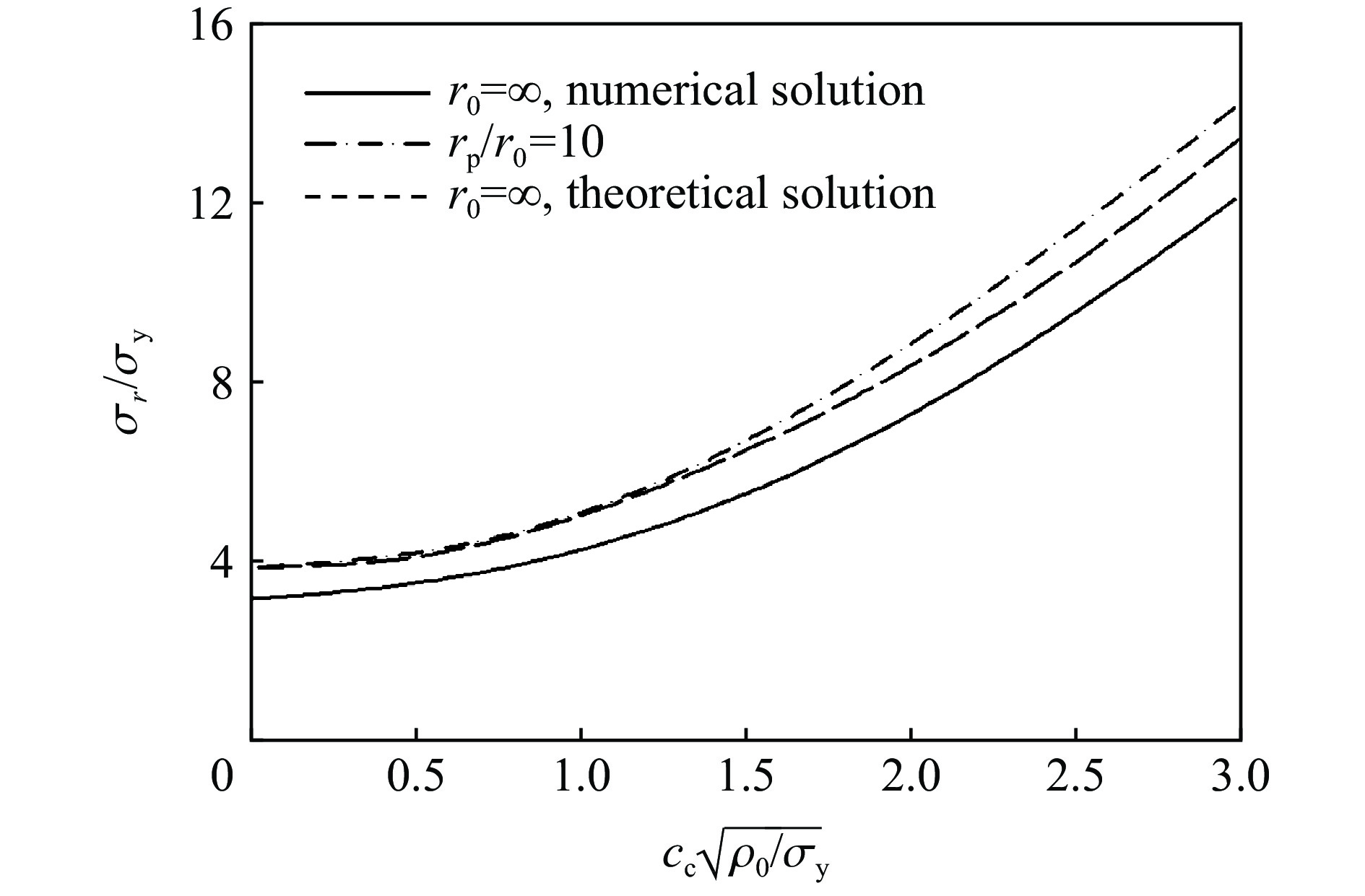
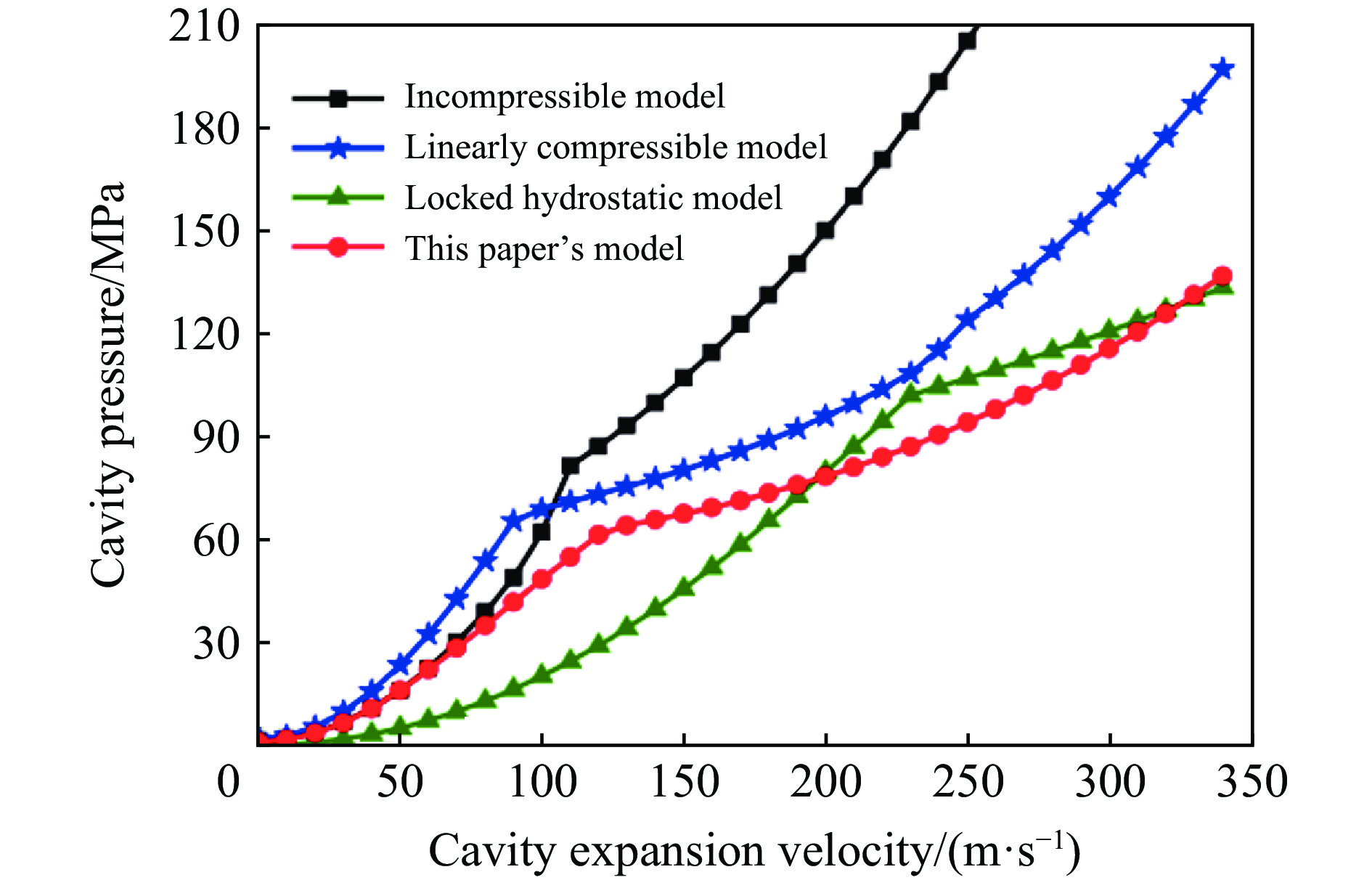
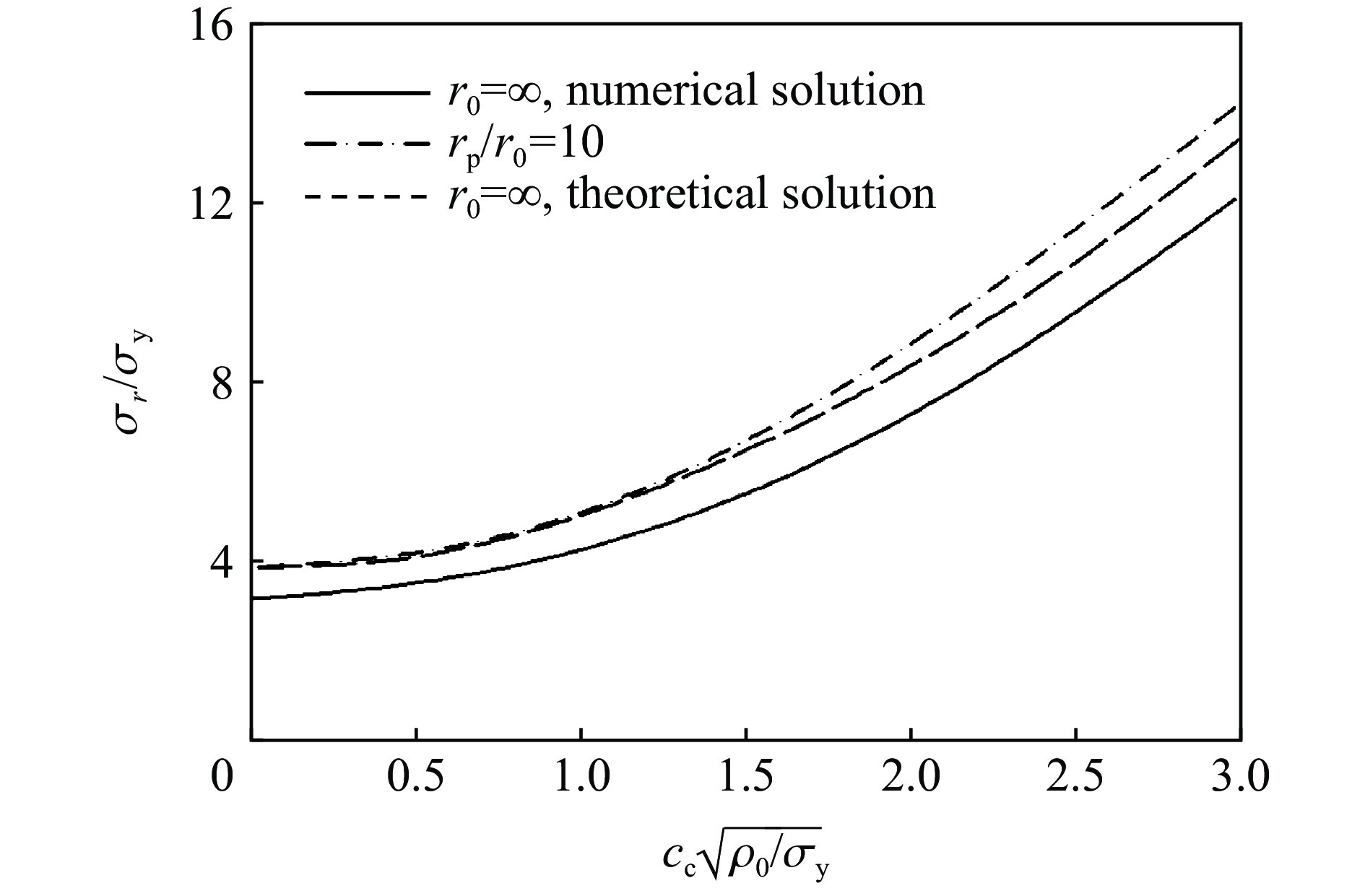
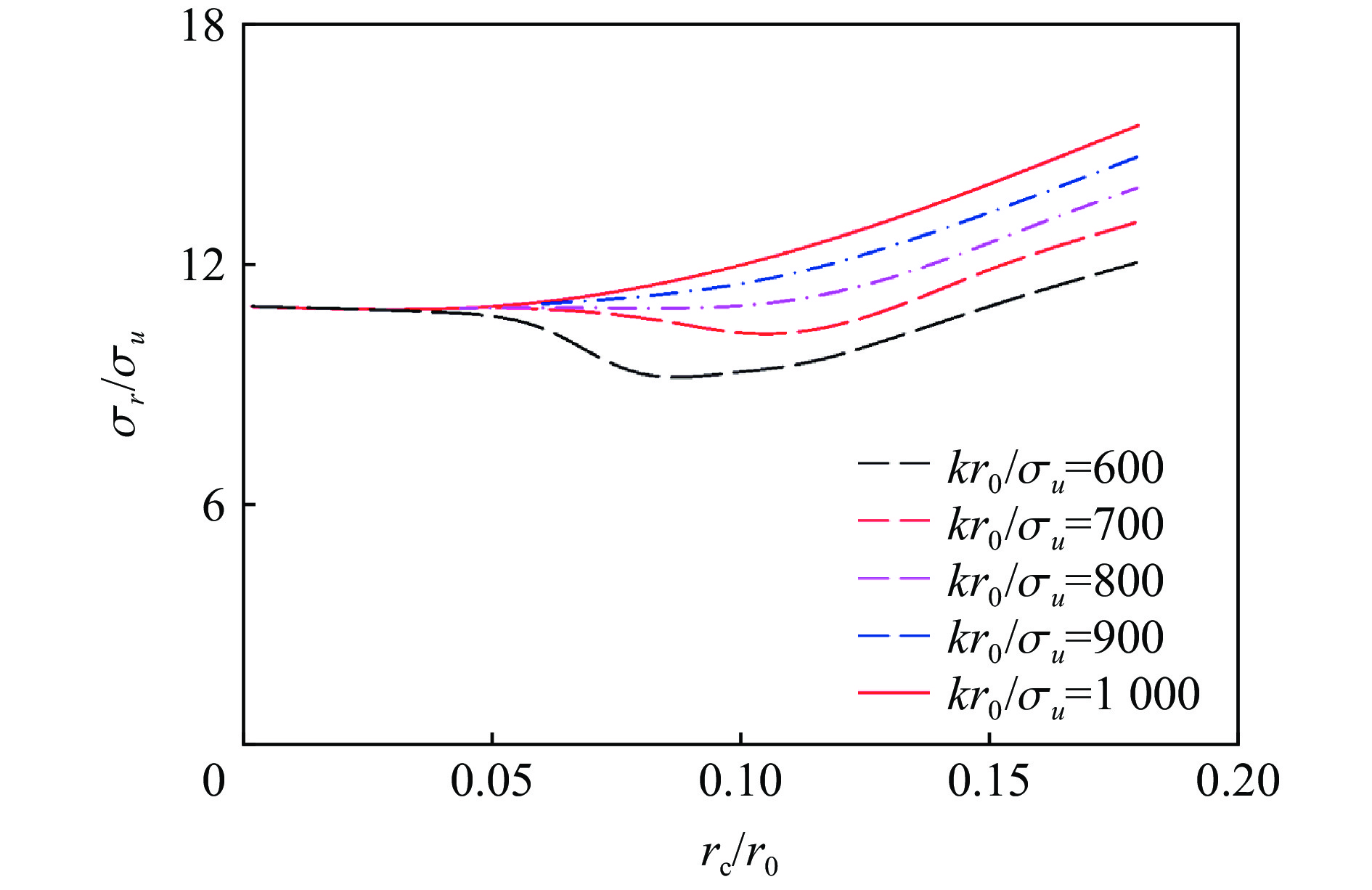
Zhen等[75-76]考虑材料可压缩性和自由边界径向位移,改进了现有不可压缩理想弹塑性材料有限空腔膨胀理论,得到了更精确的空腔边界压力理论解。图32为弹塑性阶段空腔压力与空腔膨胀速度的关系。其中,
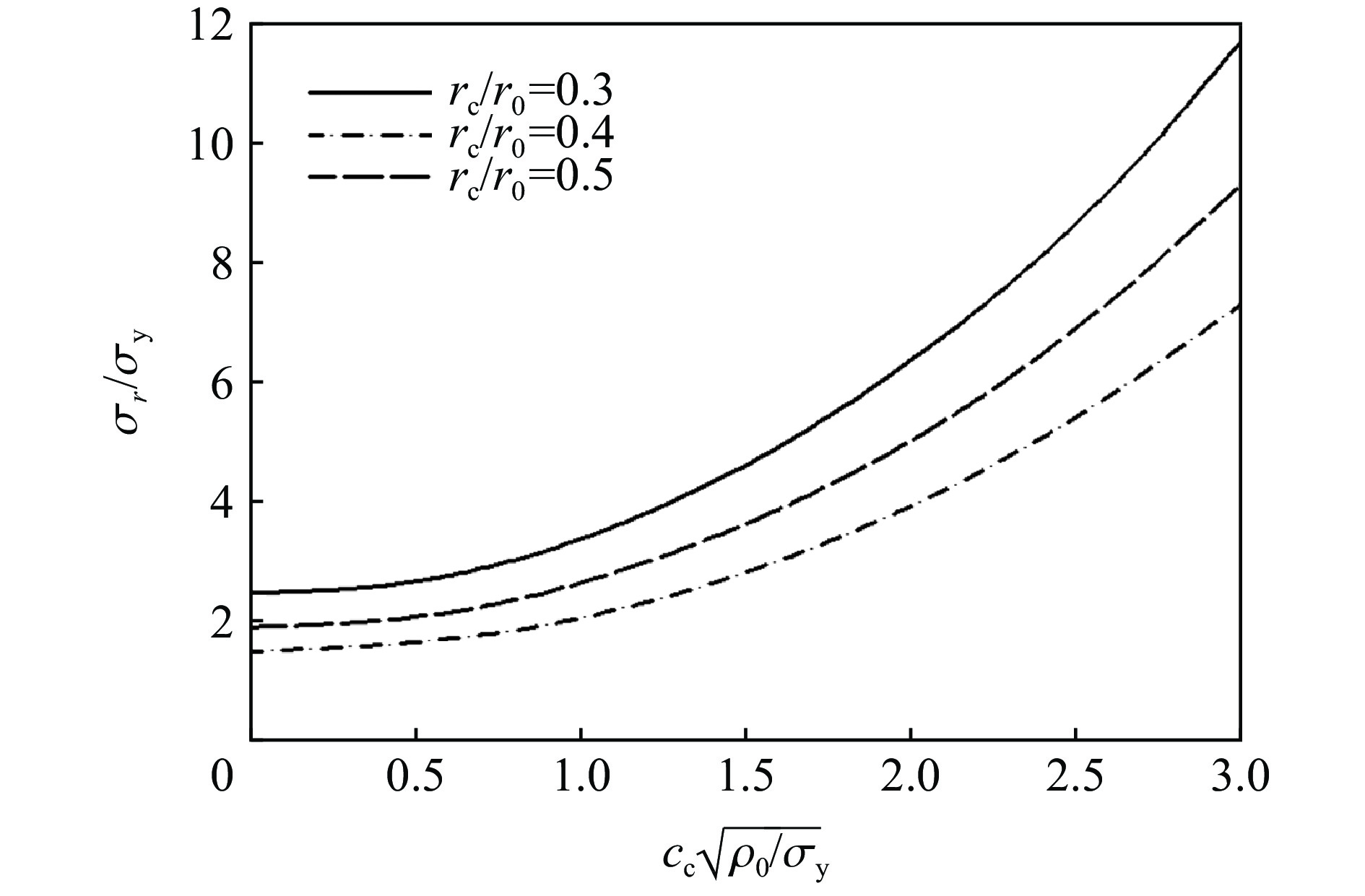
r0=∞ 和rp/r0=1 分别代表无线球形空腔膨胀和有限球形空腔膨胀的弹塑性阶段。结果表明,无限球形空腔膨胀模型比球形空腔膨胀模型的空腔压力高9.3%~17.2%。图33为有限球形空腔膨胀模型塑性阶段半径大小对空腔压力的影响,rc 为给定空腔半径,r0 为球体半径。结果表明,随着rc/r0 的增加,空腔压力减小;rc/r0=0.5 情况下的空腔压力比rc/r0=0.3 时低39.6%~37.5%,对于给定的空腔半径rc ,球体的初始半径越小,空腔压力越小。混凝土三向受压状态下的极限强度和极限应变比单轴抗压高很多。采用结构措施改善应力状态是提高普通混凝土抗侵彻性能的重要方法,对混凝土施加约束是提高混凝土抗侵彻性能的新途径[68, 77-79]。
曹扬悦也[80]基于粉碎区的Hoek-Brown准则,建立了约束混凝土准静态柱形和球形空腔膨胀模型,得到了各响应模式的空腔边界压力及模式转化条件。研究表明,约束混凝土的准静态扩孔过程可分为4个阶段,即无限介质空腔膨胀阶段、弹性-裂纹-粉碎响应阶段、裂纹-粉碎响应阶段和完全粉碎阶段。各阶段粉碎区混凝土均处于三向受压状态,扩孔压力随空腔半径增大而增大,进入完全粉碎阶段后增长迅速,且柱腔模型增幅更显著。图34为柱形/球形空腔膨胀模型下约束强度对空腔边界压力的影响规律。
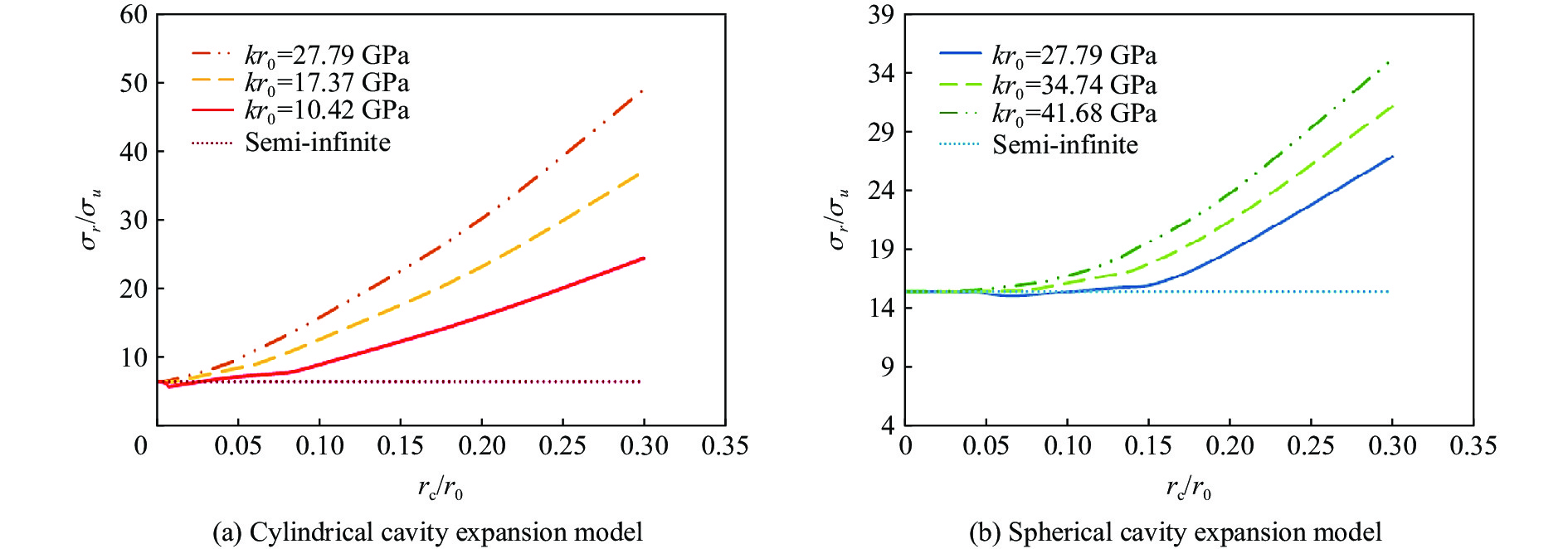
Meng等[68]研究了钢管约束的混凝土(STC)目标,基于改进的Griffith强度准则,提出了具有径向弹性限制的约束空腔膨胀模型,计算结果如图35所示。研究结果表明,约束混凝土中的空腔膨胀模型与半无限材料中的空腔膨胀模型不同,由于径向的弹性位移受到限制,因此在给定膨胀速度下其空腔边界应力值不是恒定的。
综上所述,通过合理简化问题,针对任意空腔截面、压剪耦合、有限/约束边界等复杂弹靶条件,通过空腔膨胀模型可以得出问题的近似解。但模型不能反映侵彻问题的全部物理过程,也只适用于解决一定范围内的侵彻问题,且求解过程繁琐,因此在适用性和可靠性方面受到限制。
5. 空腔膨胀阻力模型在典型侵彻问题中的应用
有关空腔膨胀模型的应用,一方面是通过求解空腔膨胀模型,给出弹体侵彻阻力的解析表达式,然后根据运动学原理建立弹体运动方程,根据边界条件和初始条件求解运动方程,得出侵彻过程中力、速度、位移随时间变化的函数;另一方面利用空腔膨胀模型结果替代弹头受到的法向压力,并与有限元法相结合进行数值模拟,可以大幅减少有限元法的计算时间。
5.1 基于空腔膨胀模型阻力函数的高速侵彻流体动力学模型
在高速侵彻过程中,由于弹、靶界面的压力远大于其材料的屈服强度,弹体头部进入流体侵彻状态,其侵彻过程可以用流体动力学方法近似描述。Tate[81-82]提出考虑弹、靶强度的修正伯努利方程来描述侵蚀弹体侵彻过程,其弹、靶界面平衡方程为:
12ρp(v−u)2+Yp=12ρtu2+Rt (20) 式中:v为弹体尾部速度,u为弹体侵彻速度,Yp为弹体强度表征项,Rt为靶体强度表征项,ρp为弹体密度,ρt为靶体密度。
Rt值接近于靶体内空腔扩展速度稳定时的空腔边界上的径向应力值,即
Rt=−σr ,部分学者基于空腔膨胀模型给出了Rt值的确定方法。图36为流体侵彻示意图。Forrestal等[83]确定了从零初始半径打开球形空腔所需的准静态压力,在Forrestal的基础上,Anderson等[84]根据无限介质中圆柱空腔膨胀的方法给出了Rt的计算方法; Godwin等[85]基于任意假定的二维流动模式的分析模型定性地给出了Rt的值;Galanov等[86-87]采用动态强度代替Satapathy[71]提出的模型中的静态强度,给出了脆性材料的两段式Rt计算公式;Lan等[88]对界面粒子速度和压力分布以及目标中的响应区域进行了假设,给出的Rt取决于弹体侵彻速度以及目标材料在高温下的力学性能。Rubin等[89]通过空腔膨胀理论研究靶板中弹塑性边界位置的极限值,并引入一个经验常数来表征这些极限值之间的过渡,给出了Rt的显示表达式;表2列举了几种典型的Rt表达式。
表 2 不同Rt 的表达式Table 2. Different values of Rt来源 Rt表达式 备注 Bishop[1] Rt=σy√3{1+ln[√3E(5−4v)σy]} σy为靶体屈服强度 Rubin[89] Rt=ln(4ς2)Yp−ft−(2/3+ln4)σy Yp为弹体屈服强度,σy为靶体屈服强度, ft为与靶体材料相关的常数 Godwin[85] Rt=(2 + 2√1 - Yp/Yt)σy Yp为弹体屈服强度,σy为靶体屈服强度 A-W模型[84] Rt=73ln(αk)σt αk为与靶体材料相关的常数 S-W-Z-S模型[90] Rt=23σy(1+ln2E3σy)+227π2E σy为靶体屈服强度,E为弹性模量 L-W模型[88] Rt=S+Cρt(UF0exp(−(u−UF0nUF0)2))2−12ρt(u−UF0exp(−(u−UF0nUF0)2))2 UF0=√YH/ρt,S为靶体静态阻力,YH为材料动态屈服强度 5.2 高速动能弹体侵彻动力学模型
针对刚体侵彻问题已开展了较多的研究工作[65-66, 91-100],已获得了较为丰富的理论成果。其主要思想是结合已知的靶体阻力,将弹体头部表面离散化,计算每个微元上的受力,通过曲面积分的方法,最终获得弹体轴向方向的阻力,联立弹体运动方程建立侵彻理论模型。
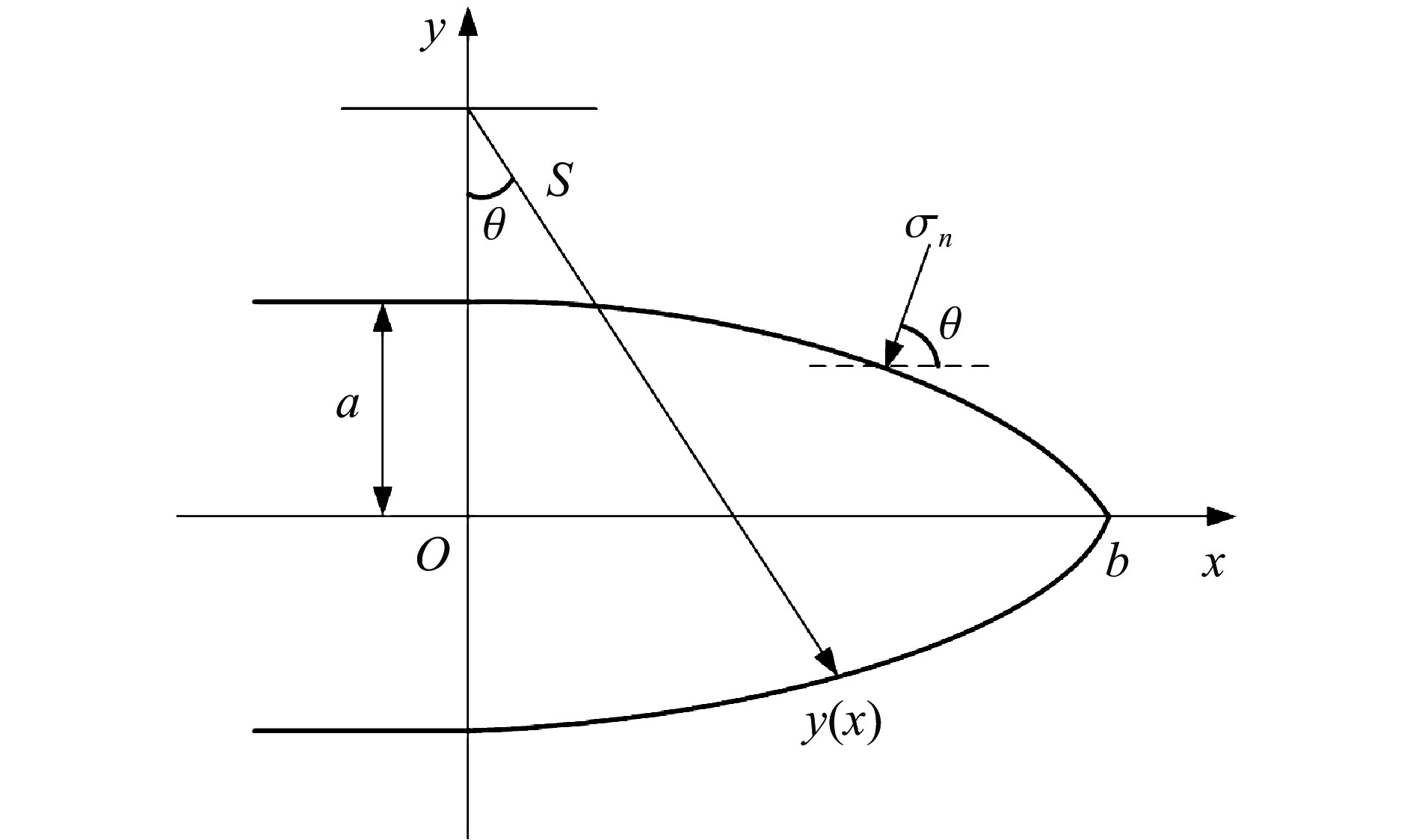
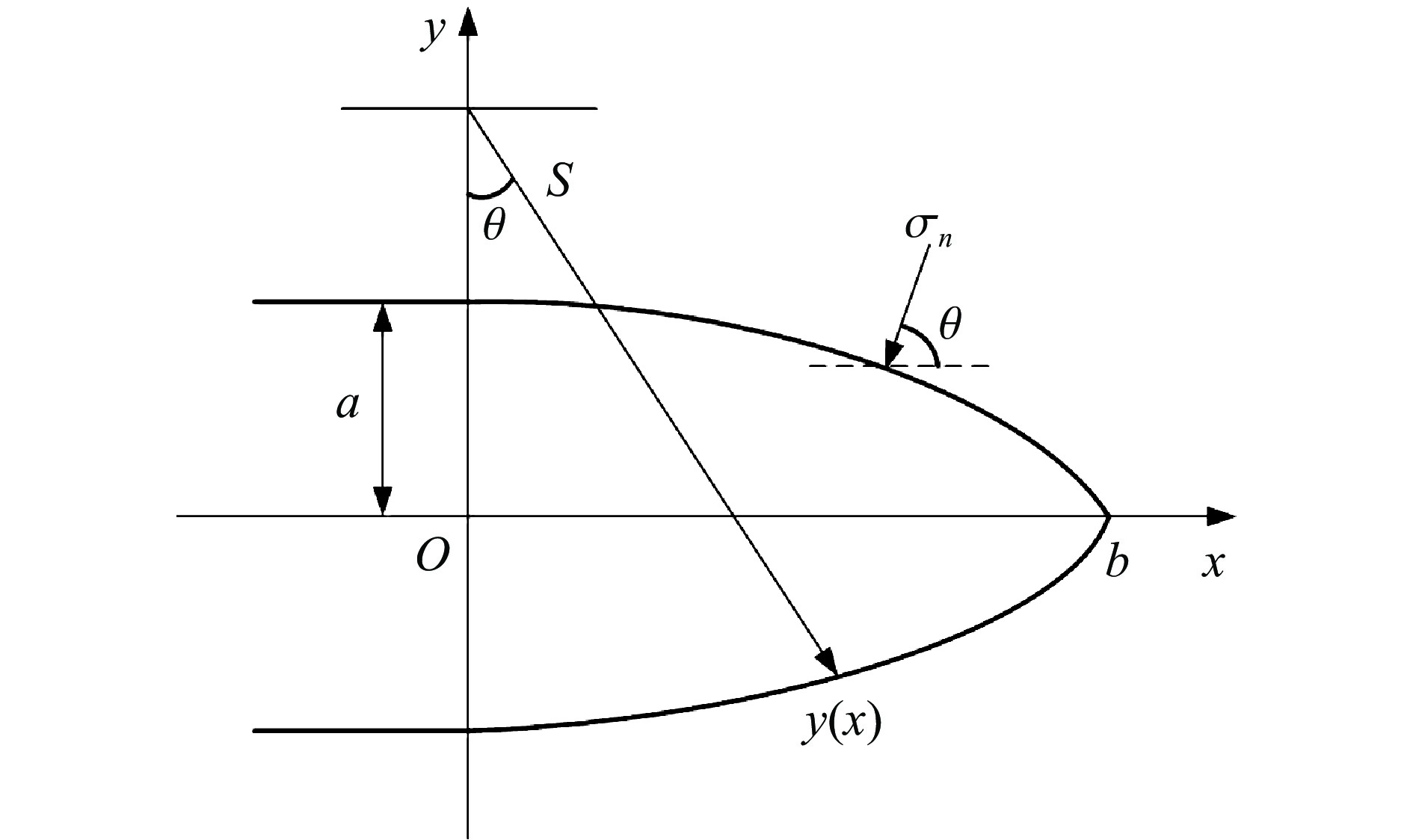
Forrestal等[10]假设空腔膨胀径向应力等于弹体表面受力,结合弹体运动方程,得出了弹体侵彻动力学模型。对于如图37所示的任意尖卵形弹体,其头部曲径比
ϕ=s/D ,则轴向受力可以表示为:Fz=2πs2∫π 2θ0{[sinϕ−(2s−D2s)](cosϕ+μsinϕ)}σn(vz,ϕ)dϕ (21) 式中:D为弹体直径,s为曲率半径,
μ 为摩擦因数,σn 为弹体表面受力。通过空腔膨胀求解的径向应力等效为弹体头部受力,弹体轴向受力可以简化为如下所示的与速度相关的函数:
Fz=αs+βsv2z (22) 式中:
αs 为靶板阻力中静阻力项,βs 为惯性项。结合式(22)与牛顿第二定律,弹体运动方程可以表示为:
{mdvzdt=−(αs+βsv2z)P=m2βsln(1+βsv20αs) (23) 通过给定弹体初始速度
v(t=0)=v0 ,积分式(23)可以得出弹体加速度a、速度v变化情况以及最终侵彻深度P。表3中列举了其他几种常见的采用空腔膨胀模型推导的侵彻深度P的预测公式。
表 3 侵彻深度理论预测公式Table 3. Theory prediction formula of penetration depth来源 侵彻深度预测公式 Frew等[101] P(L+2a/3)=1C(ρpρ0){ln[1+2B3A(√ρ0Yv)+C2A(√ρ0Yv)2]+4B√18AC−4B2[arctan2B√18AC−4B2−arctan[3C√ρ0/Yv+2B√18AC−4B2]]} Warren[91] P=m2π a2ρ0Nln(1+Nρ0v2R)+4a Wen[92] P={(√4ψ−1−2ψcosψ)aP≤LNPL+8ψ3ηa=(ρpρt)ρtv2σe12[1+β√ρtσev]+(√4ψ−1−8ψ3η)aL+8ψ3ηaP>LN Teland等[96] P=2π MNln[[1−π 4R2MX1]v20S+MN−π 4R2MX1MN+π 4X1] + X1 Kong等[17] Pleff=ρp2N2Cρ0ln(AfcN0+N1B√ρ0fcv+N2Cρ0v2AfcN0)+ρpN1B√ρ0fcN2Cρ0√ρ0fc(4ACN0N2−N21B2)×[arctan(N1B√ρ0fc√ρ0fc(4ACN0N2−N21B2))−arctan(N1B√ρ0fc+2N2Cρ0v√ρ0fc(4ACN0N2−N21B2))]+kdleff 注:表中参数与上文一致,A、B、C、N1、N2、k、d、M、leff等为与材料或弹体结构相关的常数。 5.3 基于空腔膨胀模型阻力函数的弹靶分离法
弹靶分离方法的基本思想是将侵彻过程中靶体的响应简化为与瞬时速度相关的函数,即靶体响应力函数。在数值模拟过程中,用靶体响应力函数代替靶体对弹体的作用,从而省略靶体网格划分及其变形过程中产生的网格畸变,同时无需考虑复杂的弹靶接触问题,从而缩短运算时间[90-91],其作用原理图如图38所示。
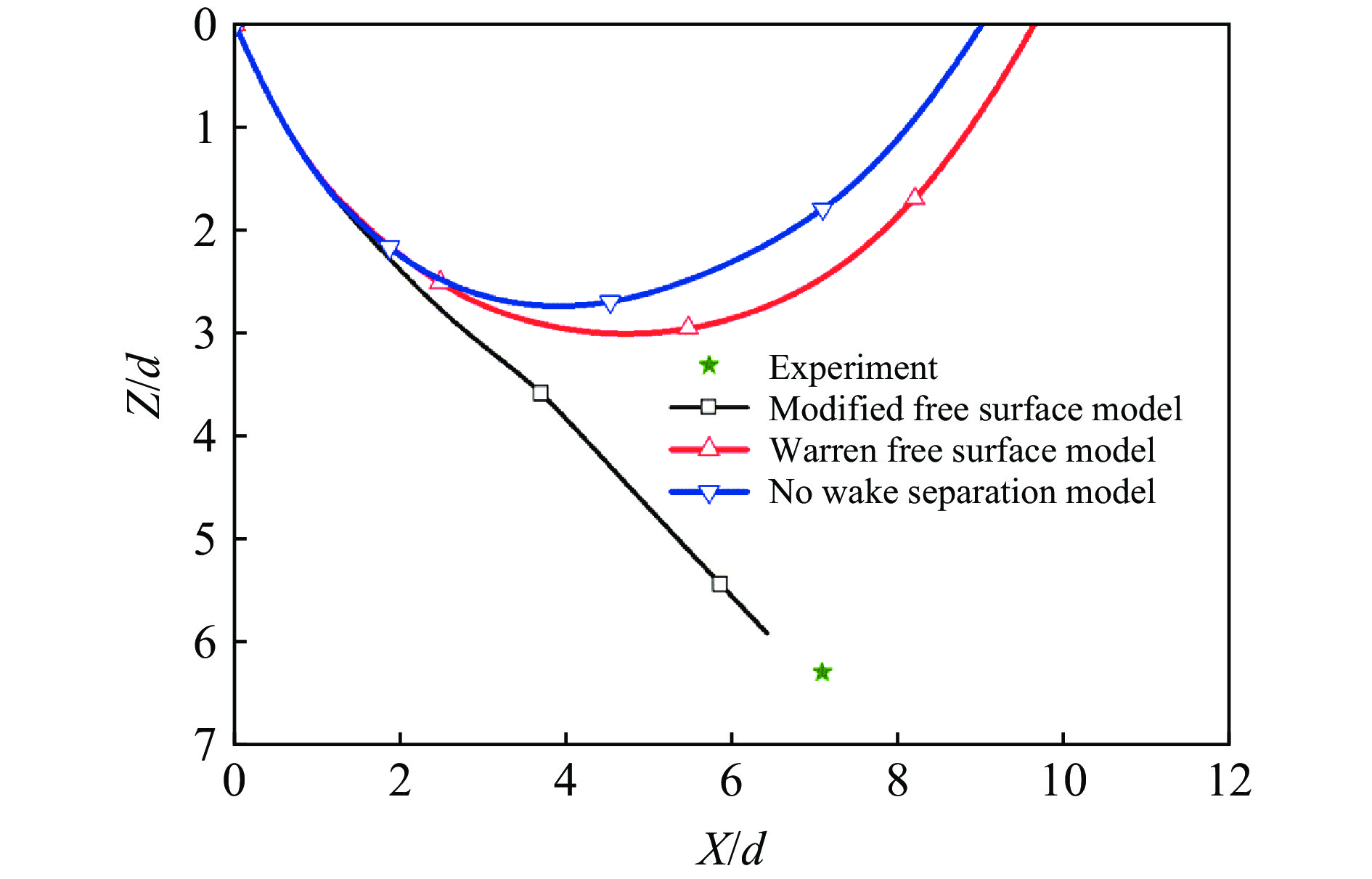
Warren[91]将基于动态球形空腔膨胀理论得到的阻力函数嵌入到有限元程序PRONTO3D中,并且加入自由面效应函数进行修正,模拟弹体斜侵彻靶体,其计算结果如图39所示。研究表明,通过计算得到弹体侵彻过程中的变形和实验结果较为符合,验证了弹靶分离方法的可靠性。
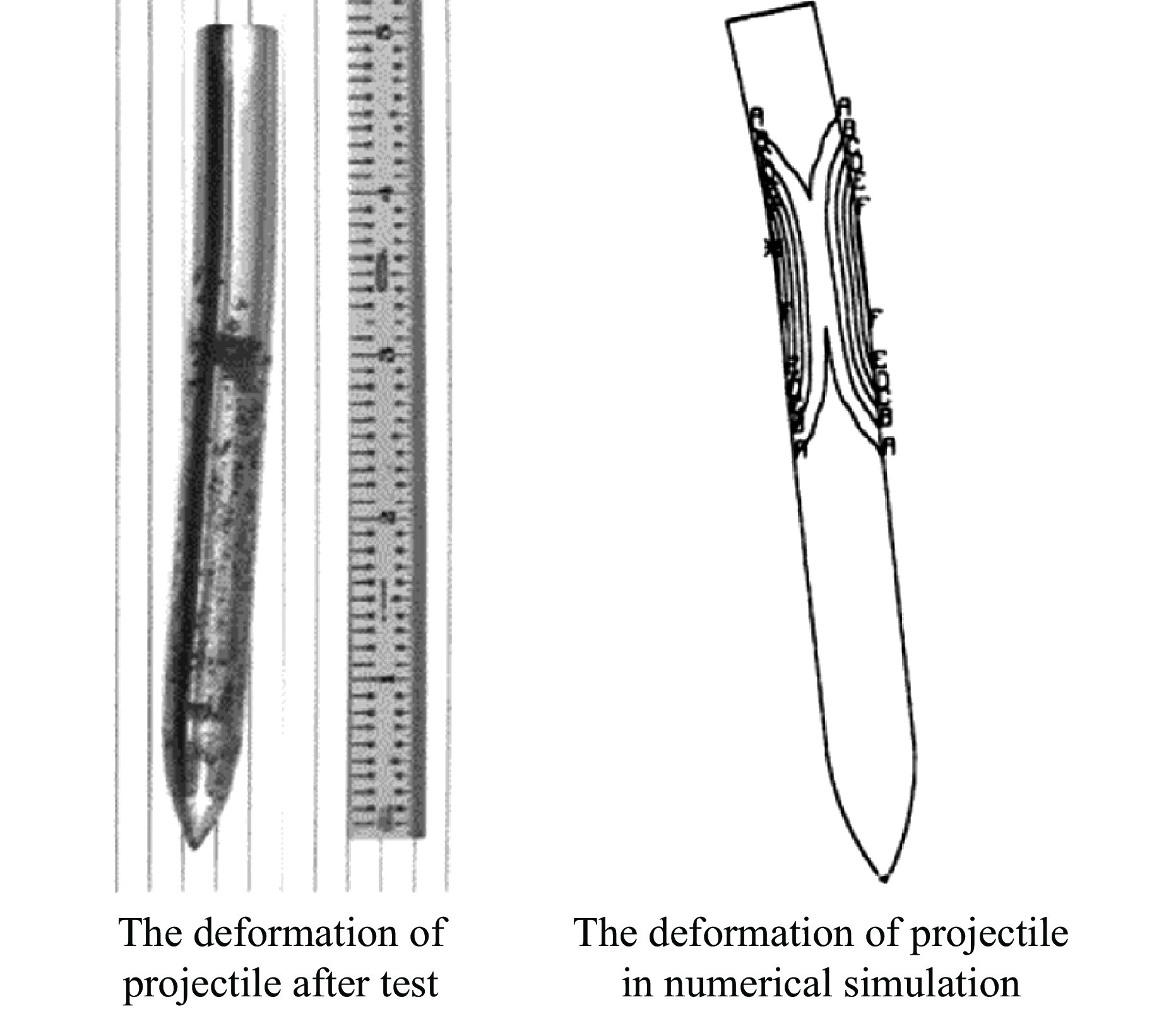
何涛等[5]基于Warren的处理方法系统地给出了预测动能弹侵彻和贯穿不同靶板的方法。王松川[102]基于弹靶分离的思想,考虑自由面效应和弹靶分离再接触效应的影响,采用Fortran语言编写斜侵彻弹道计算程序,对刚性弹斜侵彻半无限石灰石靶和6061-T6511铝靶等问题进行了计算研究,图40为弹体以45°入射角侵彻铝靶实验与仿真结果对比图。研究结果表明,采用靶体响应力函数的方法只适用于弹体以小碰撞角侵彻的情况,弹体速度较低时的计算结果与实际情况相差很大。
康海峰等[103]基于弹靶分离的思想,根据空腔膨胀理论计算弹体侵彻阻力,考虑自由面效应,进而对弹体进行受力分析,再根据刚体运动学理论,推导弹体侵彻运动微分方程,建立动能侵彻弹非正侵彻混凝土介质的动力学分析模型,可以快速计算弹体侵彻轨迹。闪雨等[104]基于空腔膨胀理论、改进的自由面效应模型及弹靶分离再接触效应建立了刚性/侵蚀弹体非正侵彻贯穿混凝土弹道轨迹预测方法,研究了弹靶结构参数及弹体着靶姿态对侵彻弹道的影响规律,计算结果如图41所示。研究表明,计算非正侵彻弹道时,需考虑自由面效应及弹靶分离再接触效应;对于混凝土靶,采用改进的自由面模型可有效预估弹体的侵彻弹道。
综上所述,在高速/超高速侵彻方面,通过与空腔膨胀理论相结合,A-T模型及改进的A-T模型发展迅速,但仍然存在诸如采用静态空腔膨胀得到的常数Rt与实验数据反向拟合和数值模拟计算所得结论相悖,大部分改进模型或不具备预测能力或存在大量经验性参数或太过复杂不便工程应用等缺点;在刚性侵彻方面,利用靶体响应力函数,可以通过侵彻动力学模型或弹靶分离的方法,快速获得弹体终点参数,但计算精度取决于靶体响应力函数,且在较高速度下,计算误差逐渐增大。
6. 总结与展望
近年来,空腔膨胀理论研究一方面集中在对材料本构方程、初始边界条件的修正,在侧向约束、有限边界、含钢筋或骨料混凝土等方面取得较大进展;另一方面,研究者们通过数值模拟的方法避开繁琐的理论求解,加深了对模型中各参数间的理解。空腔膨胀模型作为侵彻问题理论分析的主要基础理论之一,其考虑因素全面、适用情况广泛、便于工程应用等特点,为厘清高速侵彻问题提供了有力支撑。通过对相关文献查阅、整理与分析,得到的主要结论与建议如下。
(1)空腔膨胀理论准确性依赖于模型假设与材料特性,但真实、全面反映材料的屈服准则与状态方程复杂度增加了模型的求解难度。目前的研究对材料特性进行了大量简化,针对复杂材料(多孔、复合材料等)、复杂本构(应变梯度、各向异性等)的空腔膨胀理论研究亟待开展。
(2)理想弹靶条件下空腔膨胀模型预测结果与实验结果吻合较好,但在实际应用中,弹靶条件更加恶劣,弹体着靶存在着角、攻角,靶体存在自由面效应、侧向约束、随机骨料等,解决多层复合靶板、间隔靶板、弹体旋转、弹体头部刻槽等复杂情况下的空腔膨胀模型仍是一个巨大挑战;结合弹靶边界条件,发展可描述复杂化作用条件空腔膨胀阻力模型和弹体侵彻动力学计算方法,是下一步工作的重点。
(3)绝大多数空腔膨胀理论研究工作将模型简化为球/柱对称。而随着高超声速武器、星载高速动能武器的发展,适应于上述武器平台的异构型弹体的侵彻行为将成为热点问题之一[73, 105]。对于任意截面空腔膨胀问题,使用保角变换[106-107]、傅里叶变换[72]、最小二乘拟合等数学方法可以简化椭圆积分、降低微分方程组的求解难度。如何结合异构型弹体结构特点及新的数学方法,发展可描述任意截面形状的空腔膨胀模型,将是未来发展的重要方向之一。
(4)空腔膨胀理论早期应用在刚体侵彻阶段,后来扩展到高速和超高速阶段。正如Rubin[24, 52]、Rosenberg等[22-23]指出,空腔膨胀理论的基础假设具有局限性,存在稳态流场与假设不一致、高速情况下无法预测“空化”现象等。研究者需要重新审视空腔膨胀理论假设,着眼于求解思路、方法上的进步,不局限在对材料本构上的小修小补,扩大与增加模型的适用性与准确度。
-
表 1 常见的几种屈服准则与状态方程
Table 1. Several common yield criteria and equations of state
屈服准则/状态方程 函数表达式 备注 Mohr-Coulomb |σr−σθ|=Y σr和σθ分别为径向应力与环向应力,Y为屈服强度 Tresca |σr−σθ|=λp+τ0 τ0=3−λ3Y Griffith (σr−σθ)2=Y(σr + σθ) σr和σθ分别为径向应力与环向应力,Y为屈服强度 Drucker-Prager {σr−σθ=λp+τ0p<pmσr−σθ = (τ0 + λpm)p1−pp1−pmpm≤p≤p1σr−σθ = 0p>p1 σr和σθ分别为径向应力与环向应力,
Y为屈服强度,p1、pm为临界压力Hoek-Brown (σr−σθ)2=Y(m0σr + σθ) 无量纲数m0与材料强度及脆性程度有关 统一强度理论 11 + b(σ1+bσ2)−γσ3=σt γ=σt/σc为靶体材料拉压比,σt和σc分别为靶体材料的
抗拉和抗压强度,b为中间主应力的效应参数Voce应变硬化 σ = {Eεσ≤YY+2∑i=1Qi(1−exp(Ciε))σ>Y Qi和Ci为硬化参数 线性压力-体积应变 pm=Kε ε为应变 三段式线性状态方程 pm={Kεp≤pcpc+Kc(μ−μc)pc<p≤p1p1+K1(μ−μp)p>p1 K、Kc、K1为弹性区、孔隙压实区和密实区的
体积模量,pc、p1为临界压力表 2 不同
Rt 的表达式Table 2. Different values of Rt
来源 Rt表达式 备注 Bishop[1] Rt=σy√3{1+ln[√3E(5−4v)σy]} σy为靶体屈服强度 Rubin[89] Rt=ln(4ς2)Yp−ft−(2/3+ln4)σy Yp为弹体屈服强度,σy为靶体屈服强度, ft为与靶体材料相关的常数 Godwin[85] Rt=(2 + 2√1 - Yp/Yt)σy Yp为弹体屈服强度,σy为靶体屈服强度 A-W模型[84] Rt=73ln(αk)σt αk为与靶体材料相关的常数 S-W-Z-S模型[90] Rt=23σy(1+ln2E3σy)+227π2E σy为靶体屈服强度,E为弹性模量 L-W模型[88] Rt=S+Cρt(UF0exp(−(u−UF0nUF0)2))2−12ρt(u−UF0exp(−(u−UF0nUF0)2))2 UF0=√YH/ρt,S为靶体静态阻力,YH为材料动态屈服强度 表 3 侵彻深度理论预测公式
Table 3. Theory prediction formula of penetration depth
来源 侵彻深度预测公式 Frew等[101] P(L+2a/3)=1C(ρpρ0){ln[1+2B3A(√ρ0Yv)+C2A(√ρ0Yv)2]+4B√18AC−4B2[arctan2B√18AC−4B2−arctan[3C√ρ0/Yv+2B√18AC−4B2]]} Warren[91] P=m2π a2ρ0Nln(1+Nρ0v2R)+4a Wen[92] P={(√4ψ−1−2ψcosψ)aP≤LNPL+8ψ3ηa=(ρpρt)ρtv2σe12[1+β√ρtσev]+(√4ψ−1−8ψ3η)aL+8ψ3ηaP>LN Teland等[96] P=2π MNln[[1−π 4R2MX1]v20S+MN−π 4R2MX1MN+π 4X1] + X1 Kong等[17] Pleff=ρp2N2Cρ0ln(AfcN0+N1B√ρ0fcv+N2Cρ0v2AfcN0)+ρpN1B√ρ0fcN2Cρ0√ρ0fc(4ACN0N2−N21B2)×[arctan(N1B√ρ0fc√ρ0fc(4ACN0N2−N21B2))−arctan(N1B√ρ0fc+2N2Cρ0v√ρ0fc(4ACN0N2−N21B2))]+kdleff 注:表中参数与上文一致,A、B、C、N1、N2、k、d、M、leff等为与材料或弹体结构相关的常数。 -
[1] BISHOP R F, HILL R, MOTT N F. The theory of indentation and hardness tests [J]. Proceedings of the Physical Society (1926−1948), 1945, 57(3): 147–159. DOI: 10.1088/0959-5309/57/3/301. [2] CHADWICK P. The quasi-static expansion of a spherical cavity in metals and ideal soils [J]. Quarterly Journal of Mechanics and Applied Mathematics, 1959, 12(1): 52–71. DOI: 10.1093/qjmam/12.1.52. [3] HOPKINS H G. Dynamic expansion of spherical cavities in metals [J]. Progress in Solid Mechanics, 1960, 1(3): 83–164. [4] 邓佳杰, 张先锋, 刘闯, 等. 头部非对称刻槽弹体侵彻混凝土目标性能研究 [J]. 兵工学报, 2018, 39(7): 1249–1258. DOI: 10.3969/j.issn.1000-1093.2018.07.001.DENG J J, ZHANG X F, LIU C, et al. Research on penetration of asymmetrically grooved nose projectile into concrete target [J]. Acta Armamentarii, 2018, 39(7): 1249–1258. DOI: 10.3969/j.issn.1000-1093.2018.07.001. [5] 何涛, 文鹤鸣. 靶体响应力函数的确定方法及其在侵彻力学中的应用 [J]. 中国科学技术大学学报, 2007, 37(10): 1249–1261. DOI: 10.3969/j.issn.0253-2778.2007.10.017.HE T, WEN H M. Determination of the analytical forcing function of target responseand its applications in penetration mechanics [J]. Journal of University of Science and Technology of China, 2007, 37(10): 1249–1261. DOI: 10.3969/j.issn.0253-2778.2007.10.017. [6] TATE A. Further results in the theory of long rod penetration [J]. Journal of the Mechanics and Physics of Solids, 1969, 17(3): 141–150. DOI: 10.1016/0022-5096(69)90028-3. [7] YANKELEVSKY D Z, ADIN M A. A simplified analytical method for soil penetration analysis [J]. International Journal for Numerical and Analytical Methods in Geomechanics, 1980, 4(3): 233–254. DOI: 10.1002/nag.1610040304. [8] FELDGUN V R, YANKELEVSKY D Z, KARINSKI Y S. A new simplified analytical model for soil penetration analysis of rigid projectiles using the Riemann problem solution [J]. International Journal of Impact Engineering, 2017, 101: 49–65. DOI: 10.1016/j.ijimpeng.2016.10.015. [9] HUNTER S C, CROZIER R J M. Similarity solution for the rapid uniform expansion of a spherical cavity in a compressible elastic-plastic solid [J]. The Quarterly Journal of Mechanics & Applied Mathematics, 1968, 21(4): 467–486. DOI: 10.1093/qjmam/21.4.467. [10] FORRESTAL M J, LUK V K. Penetration into soil targets [J]. International Journal of Impact Engineering, 1992, 12(3): 427–444. DOI: 10.1016/0734-743X(92)90167-R. [11] FORRESTAL M J, LUK V K. Dynamic spherical cavity-expansion in a compressible elastic-plastic solid [J]. Journal of Applied Mechanics, 1988, 55(2): 275–279. DOI: 10.1115/1.3173672. [12] FORRESTAL M J, OKAJIMA K, LUK V K. Penetration of 6061-T651 aluminum targets with rigid long rods [J]. Journal of Applied Mechanics, 1988, 55(4): 755–760. DOI: 10.1115/1.3173718. [13] LUK V K, FORRESTAL M J. Penetration into semi-infinite reinforced-concrete targets with spherical and ogival nose projectiles [J]. International Journal of Impact Engineering, 1987, 6(4): 291–301. DOI: 10.1016/0734-743X(87)90096-0. [14] FORRESTAL M J, LONGCOPE D B, NORWOOD F R. A model to estimate forces on conical penetrators into dry porous rock [J]. Journal of Applied Mechanics, 1981, 48(1): 25–29. DOI: 10.1115/1.3157587. [15] FORRESTAL M J. Penetration into dry porous rock [J]. International Journal of Solids and Structures, 1986, 22(12): 1485–1500. DOI: 10.1016/0020-7683(86)90057-0. [16] SATAPATHY S. Dynamic spherical cavity expansion in brittle ceramics [J]. International Journal of Solids and Structures, 2001, 38(32–33): 5833–5845. DOI: 10.1016/S0020-7683(00)00388-7. [17] KONG X Z, WU H, FANG Q, et al. Rigid and eroding projectile penetration into concrete targets based on an extended dynamic cavity expansion model [J]. International Journal of Impact Engineering, 2017, 100: 13–22. DOI: 10.1016/j.ijimpeng.2016.10.005. [18] KONG X Z, WU H, FANG Q, et al. Projectile penetration into mortar targets with a broad range of striking velocities: test and analyses [J]. International Journal of Impact Engineering, 2017, 106: 18–29. DOI: 10.1016/j.ijimpeng.2017.02.022. [19] 陈小伟, 李小笠, 陈裕泽, 等. 刚性弹侵彻动力学中的第三无量纲数 [J]. 力学学报, 2007, 39(1): 77–84. DOI: 10.3321/j.issn:0459-1879.2007.01.010.CHEN X W, LI X L, CHEN Y Z, et al. The third dimensionless parameter in the penetration dynamics of rigid projectiles [J]. Chinese Journal of Theoretical and Applied Mechanics, 2007, 39(1): 77–84. DOI: 10.3321/j.issn:0459-1879.2007.01.010. [20] WARREN T L, TABBARA M R. Simulations of the penetration of 6061-T6511 aluminum targets by spherical-nosed VAR 4340 steel projectiles [J]. International Journal of Solids and Structures, 2000, 37(32): 4419–4435. DOI: 10.1016/S0020-7683(99)00148-1. [21] Warren T L, Forrestal M J. Effects of strain hardening and strain-rate sensitivity on the penetration of aluminum targets with spherical-nosed rods [J]. International Journal of Solids and Structures, 1998, 35(35): 3737–3753. DOI: 10.1016/S0020-7683(97)00211-4. [22] ROSENBERG Z, DEKEL E. The penetration of rigid long rods-revisited [J]. International Journal of Impact Engineering, 2009, 36(4): 551–564. DOI: 10.1016/j.ijimpeng.2008.06.001. [23] ROSENBERG Z, KOSITSKI R, DEKEL E. Comment on: "Rigid and eroding projectile penetration into concrete targets based on an extended cavity expansion model" by Kong et al. Int. J. Impact Eng. (2017) [J]. International Journal of Impact Engineering, 2017, 104: A1–A3. DOI: 10.1016/j.ijimpeng.2017.03.004. [24] RUBIN M B. Analytical formulas for penetration of a long rigid projectile including the effect of cavitation [J]. International Journal of Impact Engineering, 2012, 40−41: 1–9. DOI: 10.1016/j.ijimpeng.2011.09.008. [25] YANKELEVSKY D Z, FELDGUN V R. Issues in modelling the penetration of thick targets by rigid long rods [J]. International Journal of Impact Engineering, 2020, 137: 103474. DOI: 10.1016/j.ijimpeng.2019.103474. [26] YANKELEVSKY D, FELDGUN V. The embedment of a high velocity rigid ogive nose projectile into a concrete target [J]. International Journal of Impact Engineering, 2020, 144: 103631. DOI: 10.1016/j.ijimpeng.2020.103631. [27] COLLOMBET F, TRANCHET J Y. Damage behaviour of alumina submitted to a divergent spherical wave [J]. Journal de Physique IV, 1994, 4(C8): 641–646. DOI: 10.1051/jp4:1994897. [28] CURRAN D R, SEAMAN L, COOPER T, et al. Micromechanical model for comminution and granular flow of brittle material under high strain rate application to penetration of ceramic targets [J]. International Journal of Impact Engineering, 1993, 13(1): 53–83. DOI: 10.1016/0734-743X(93)90108-J. [29] WEI H Y, ZHANG X F, LIU C, et al. Oblique penetration of ogive-nosed projectile into aluminum alloy targets [J]. International Journal of Impact Engineering, 2021, 148: 103745. DOI: 10.1016/j.ijimpeng.2020.103745. [30] FORRESTAL M J, TZOU D Y. A spherical cavity-expansion penetration model for concrete targets [J]. International Journal of Solids and Structures, 1997, 34(31/32): 4127–4146. DOI: 10.1016/S0020-7683(97)00017-6. [31] 李志康, 黄风雷. 混凝土材料的动态空腔膨胀理论 [J]. 爆炸与冲击, 2009, 29(1): 95–100. DOI: 10.11883/1001-1455(2009)01-0095-06.LI Z K, HUANG F L. A dynamic spherical cavity-expansion theory for concrete materials [J]. Explosion and Shock Waves, 2009, 29(1): 95–100. DOI: 10.11883/1001-1455(2009)01-0095-06. [32] 张先锋, 李向东, 沈培辉, 等. 终点效应学[M]. 北京: 北京理工大学出版社, 2017: 33−39.ZHANG X F, LI X D, SHEN P H, et al. Terminal effects[M]. Beijing: Beijing Institute of Technology Press, 2017: 33−39. [33] DAVIS R O, SCOTT R F, MULLENGER G. Rapid expansion of a cylindrical cavity in a rate-type soil [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1984, 21(4): 126. [34] HILL R. A theory of earth movement near a deep underground explosion [Z]. Armament Research Establishment, Memo, 1948: 21−48. [35] HUANG Y, HUTCHINSON J W, TVERGAARD V. Cavitation instabilities in elastic-plastic solids [J]. Journal of the Mechanics and Physics of Solids, 1991, 39(2): 223–241. DOI: 10.1016/0022-5096(91)90004-8. [36] HE T, WEN H M, GUO X J. A spherical cavity expansion model for penetration of ogival-nosed projectiles into concrete targets with shear-dilatancy [J]. Acta MechanicaSinica, 2011, 27(6): 1001–1012. DOI: 10.1007/s10409-011-0505-1. [37] FENG J, LI W B, WANG X M, et al. Dynamic spherical cavity expansion analysis of rate-dependent concrete material with scale effect [J]. International Journal of Impact Engineering, 2015, 84: 24–37. DOI: 10.1016/j.ijimpeng.2015.05.005. [38] SHI C C, WANG M Y, LI J, et al. A model of depth calculation for projectile penetration into dry sand and comparison with experiments [J]. International Journal of Impact Engineering, 2014, 73: 112–122. DOI: 10.1016/j.ijimpeng.2014.06.010. [39] SHAO R Z, WU C Q, LIU Z X, et al. Penetration resistance of ultra-high-strength concrete protected with layers of high-toughness and lightweight energy absorption materials [J]. Composite Structures, 2018, 185: 807–820. DOI: 10.1016/j.compstruct.2017.11.038. [40] WU H, REN G M, FANG Q, et al. Response of ultra-high performance cementitious composites filled steel tube (UHPCC-FST) subjected to low-velocity impact [J]. Thin-Walled Structures, 2019, 144: 106341. DOI: 10.1016/j.tws.2019.106341. [41] CAO Y Y Y, TAN Q H, JIANG Z G, et al. A nonlinear rate-dependent model for predicting the depth of penetration in ultra-high performance fiber reinforced concrete (UHPFRC) [J]. Cement and Concrete Composites, 2020, 106: 103451. DOI: 10.1016/j.cemconcomp.2019.103451. [42] LIU J, WU C Q, SU Y, et al. Experimental and numerical studies of ultra-high performance concrete targets against high-velocity projectile impacts [J]. Engineering Structures, 2018, 173: 166–179. DOI: 10.1016/j.engstruct.2018.06.098. [43] LIU J, WU C Q, CHEN X W. Numerical study of ultra-high performance concrete under non-deformable projectile penetration [J]. Construction and Building Materials, 2017, 135: 447–458. DOI: 10.1016/j.conbuildmat.2016.12.216. [44] KONG X Z, WU H, FANG Q, et al. Analyses of rigid projectile penetration into UHPCC target based on an improved dynamic cavity expansion model [J]. Construction and Building Materials, 2016, 126: 759–767. DOI: 10.1016/j.conbuildmat.2016.09.093. [45] PENG Y, WU H, FANG Q, et al. Modified spherical cavity-expansion model for projectile penetration into concrete targets [J]. Acta MechanicaSinica, 2019, 35(3): 518–534. DOI: 10.1007/s10409-018-0815-7. [46] 张欣欣, 武海军, 黄风雷. 考虑剪胀效应的混凝土动态柱形空腔膨胀理论 [J]. 北京理工大学学报, 2016, 36(10): 1006–1010. DOI: 10.15918/j.tbit1001-0645.2016.10.004.ZHANG X X, WU H J, HUANG F L. Theory of dynamic cylindrical cavity expansion of concrete with shear-dilatancy [J]. Transactions of Beijing Institute of Technology, 2016, 36(10): 1006–1010. DOI: 10.15918/j.tbit1001-0645.2016.10.004. [47] HANAGUD S, ROSS B. Large deformation, deep penetration theory for a compressible strain-hardening target material [J]. AIAA Journal, 1971, 9(5): 905–911. DOI: 10.2514/3.6294. [48] NORWOOD F R. Cylindrical cavity expansion in a locking soil: SLA-74-0201 [R]. Albuquerque: Sandia Labs, 1974. [49] SATAPATHY S, BLESS S. Calculation of penetration resistance of brittle materials using spherical cavity expansion analysis [J]. Mechanics of Materials, 1996, 23(4): 323–330. DOI: 10.1016/0167-6636(96)00022-1. [50] SUBRAMANIAN R, BLESS S J. Penetration of semi-infinite AD995 alumina targets by tungsten long rod penetrators from 1.5 to 3.5 km/s [J]. International Journal of Impact Engineering, 1995, 17(4−6): 807–816. DOI: 10.1016/0734-743X(95)99901-3. [51] GOODIER J N. On the mechanics of indentation and cratering in solid targets of strain-hardening metal by impact of hard and soft spheres [C]// Proceedings of the 7th Symposium on Hypervelocity Impact. New York: AIAA, 1965: 215−259. [52] RUBIN M B. The fundamental assumptions of cavity expansion models for penetration-revisited [J]. International Journal of Impact Engineering, 2020, 146: 103723. DOI: 10.1016/j.ijimpeng.2020.103723. [53] RAPOPORT L, RUBIN M B. Separation and velocity dependence of the drag force applied to a rigid ovoid of Rankine nosed projectile penetrating an elastic-perfectly-plastic target [J]. International Journal of Impact Engineering, 2009, 36(8): 1012–1018. DOI: 10.1016/j.ijimpeng.2008.11.005. [54] KAWATA K, SHIOIRI J. High velocity deformation of solids || an experimental study on the penetration of rigid projectiles into epoxy resin targets [M]. New York: Springer-Verlag, 1978: 345−350. [55] KONG X Z, WU H, FANG Q, et al. Response to: comment on“rigid and eroding projectile penetration into concrete targets based on an extended cavity expansion model”by Kong et al. Int. J. Impact Eng. 2017 by Z. Rosenberg et al. [J]. International Journal of Impact Engineering, 2017, 104: 150–153. DOI: 10.1016/j.ijimpeng.2017.03.003. [56] FELDGUN V R, YANKELEVSKY D Z, KARINSKI Y S. Cavitation phenomenon in penetration of rigid projectiles into elastic-plastic targets [J]. International Journal of Impact Engineering, 2021, 151: 103837. DOI: 10.1016/j.ijimpeng.2021.103837. [57] COHEN T, MASRI R, DURBAN D. Shock waves in dynamic cavity expansion [J]. Journal of Applied Mechanics, 2010, 77(4): 041009. DOI: 10.1115/1.4000914. [58] DENG Y J, CHEN X W, YAO Y. Study on the cavity expansion response of the concrete target under penetration [J]. Scientia Sinica Physica, Mechanica & Astronomica, 2020, 50(2): 024606. DOI: 10.1360/sspma-2019-0184. [59] ROSENBERG Z, DEKEL E. A numerical study of the cavity expansion process and its application to long-rod penetration mechanics [J]. International Journal of Impact Engineering, 2008, 35(3): 147–154. DOI: 10.1016/j.ijimpeng.2007.01.005. [60] RODRÍGUEZ-MARTÍNEZ M, COHEN T, ZAERA R. Approaching steady cavitation: the time scale in hypervelocity cavity expansion in work hardening and transformation hardening solids [J]. International Journal of Impact Engineering, 2014, 73: 43–55. DOI: 10.1016/j.ijimpeng.2014.06.005. [61] MASRI R, DURBAN D. Deep penetration analysis with dynamic cylindrical cavitation fields [J]. International Journal of Impact Engineering, 2009, 36(6): 830–841. DOI: 10.1016/j.ijimpeng.2008.12.006. [62] ANDERSON C E. Analytical models for penetration mechanics: a review [J]. International Journal of Impact Engineering, 2017, 108: 3–26. DOI: 10.1016/j.ijimpeng.2017.03.018. [63] WARREN T L, FOSSUM A F, FREW D J. Penetration into low-strength (23 MPa) concrete: target characterization and simulations [J]. International Journal of Impact Engineering, 2004, 30(5): 477–503. DOI: 10.1016/S0734-743X(03)00092-7. [64] ROSENBERG Z, DEKEL E. Analytical solution of the spherical cavity expansion process [J]. International Journal of Impact Engineering, 2009, 36(2): 193–198. DOI: 10.1016/j.ijimpeng.2007.12.014. [65] 牛振坤, 陈小伟, 邓勇军, 等. 混凝土靶侵彻过程中空腔膨胀响应分区 [J]. 爆炸与冲击, 2019, 39(2): 023301. DOI: 10.11883/bzycj-2017-0368.NIU Z K, CHEN X W, DENG Y J, et al. Cavity expansion response of concrete targets under penetration [J]. Explosion and Shock Waves, 2019, 39(2): 023301. DOI: 10.11883/bzycj-2017-0368. [66] 王一楠, 黄风雷. 混凝土材料动态球形空腔膨胀的数值模拟 [J]. 北京理工大学学报, 2010, 30(1): 5–9. DOI: 10.15918/j.tbit1001-0645.2010.01.010.WANG Y N, HUANG F L. Numerical simulation of dynamic spherical cavity expansion for concrete materials [J]. Transactions of Beijing Institute of Technology, 2010, 30(1): 5–9. DOI: 10.15918/j.tbit1001-0645.2010.01.010. [67] 晋小超, 杨华伟, 王志华, 等. 混凝土材料球形空腔膨胀的数值研究 [J]. 太原理工大学学报, 2016, 47(3): 405–410. DOI: 10.16355/j.cnki.issn1007-9432tyut.2016.03.024.JIN X C, YANG H W, WANG Z H, et al. Numerical study on the dynamic spherical cavity expansion of concrete materials [J]. Journal of Taiyuan University of Technology, 2016, 47(3): 405–410. DOI: 10.16355/j.cnki.issn1007-9432tyut.2016.03.024. [68] MENG C M, TAN Q H, JIANG Z G, et al. Approximate solutions of finite dynamic spherical cavity-expansion models for penetration into elastically confined concrete targets [J]. International Journal of Impact Engineering, 2018, 114: 182–193. DOI: 10.1016/j.ijimpeng.2018.01.001. [69] WOOLSEY P. Ceramic materials screening by residual penetration ballistic testing [C]// Proceedings of13th International Symposium of Ballistics. Stockholm, 1992: 109−116. [70] BEN-DOR G, DUBINSKY A, ELPERIN T. New results on ballistic performance of multi-layered metal shields: review [J]. Theoretical and Applied Fracture Mechanics, 2017, 88: 1–8. DOI: 10.1016/j.tafmec.2016.11.002. [71] SATAPATHY S S. Application of cavity expansion analysis to penetration problems [D]. Austin: The University of Texas at Austin, 1997: 82−100. [72] WOO H J. Cavity expansion analysis of non-circular cross-sectional penetration problems [D]. Austin: The University of Texas at Austin, 1997. [73] 王文杰, 张先锋, 邓佳杰, 等. 椭圆截面弹体侵彻砂浆靶规律分析 [J]. 爆炸与冲击, 2018, 38(1): 164–173. DOI: 10.11883/bzycj-2017-0020.WANG W J, ZAHNG X F, DENG J J, et al. Analysis of projectile penetrating into mortar target with elliptical cross-section [J]. Explosion and Shock Waves, 2018, 38(1): 164–173. DOI: 10.11883/bzycj-2017-0020. [74] LITTLEFIELD D L, ANDERSON C E, PARTOM Y, et al. The penetration of steel targets finite in radial extent [J]. International Journal of Impact Engineering, 1997, 19(1): 49–62. DOI: 10.1016/S0734-743X(96)00001-2. [75] ZHEN M, JIANG Z G, SONG D Y. A dynamic cylindrical cavity expansion model for the penetration of confined concrete targets [J]. Applied Mechanics and Materials, 2013, 341–342: 467–471. DOI: 10.4028/www.scientific.net/AMM.341-342.467. [76] ZHEN M, JIANG Z G, SONG D Y, et al. Analytical solutions for finite cylindrical dynamic cavity expansion in compressible elastic-plastic materials [J]. Applied Mathematics and Mechanics, 2014, 35(8): 1039–1050. DOI: 10.1007/s10483-014-1842-7. [77] FANG Q, KONG X Z, HONG J, et al. Prediction of projectile penetration and perforation by finite cavity expansion method with the free-surface effect [J]. Acta MechanicaSolidaSinica, 2014, 27(6): 597–611. DOI: 10.1016/s0894-9166(15)60005-2. [78] KONG X Z, FANG Q, WU H J, et al. Finite spherical cavity expansion method for layering effect [J]. Acta Mechanica SolidaSinica, 2016, 29(6): 642–654. DOI: 10.1016/s0894-9166(16)30334-2. [79] CHENG Y, YANG H W, SUN D A. Cavity expansion in unsaturated soils of finite radial extent [J]. Computers and Geotechnics, 2018, 102: 216–228. DOI: 10.1016/j.compgeo.2018.06.013. [80] 曹扬悦也. 约束混凝土抗侵彻机理与工程模型研究[D]. 长沙: 国防科学技术大学, 2015: 45−65.CAO Y Y Y. Investigation on the mechanisms and engineering modeling of confined concrete against penetration [D]. Changsha: National University of Defense Technology, 2015: 45−65. [81] TATE A. Long rod penetration models—Part I. A flow field model for high speed long rod penetration [J]. International Journal of Mechanical Sciences, 1986, 28(8): 535–548. DOI: 10.1016/0020-7403(86)90051-2. [82] TATE A. Long rod penetration models—Part II. Extensions to the hydrodynamic theory of penetration [J]. International Journal of Mechanical Sciences, 1986, 28(9): 599–612. DOI: 10.1016/0020-7403(86)90075-5. [83] FORRESTAL M J, LONGCOPE D B. Target strength of ceramic materials for high‐velocity penetration [J]. Journalof Applied Physics, 1990, 67(8): 3669–3672. DOI: 10.1063/1.345322. [84] ANDERSON C E Jr, WALKER J D. An examination of long-rod penetration [J]. International Journal of Impact Engineering, 1991, 11(4): 481–501. DOI: 10.1016/0734-743X(91)90015-8. [85] GODWIN R P, CHAPYAK E J. Apparent target strength in long-rod penetration [J]. International Journal of Impact Engineering, 1998, 21(1–2): 77–88. DOI: 10.1016/S0734-743X(97)00029-8. [86] GALANOV B A, IVANOV S M, KARTUZOV V V. Investigation of penetration resistance using a new modification of the Alekseevskii-Tate model [J]. International Journal of Impact Engineering, 2003, 29(1–10): 263–272. DOI: 10.1016/j.ijimpeng.2003.09.021. [87] GALANOV B A, IVANOV S M, KARTUZOV V V. On one new modification of Alekseevskii-Tate model for nonstationary penetration of long rods into targets [J]. International Journal of Impact Engineering, 2001, 26(1–10): 201–210. DOI: 10.1016/S0734-743X(01)00084-7. [88] LAN B, WEN H M. Alekseevskii-Tate revisited: an extension to the modified hydrodynamic theory of long rod penetration [J]. Science China Technological Sciences, 2010, 53(5): 1364–1373. DOI: 10.1007/s11431-010-0011-x. [89] RUBIN M B, YARIN A L. A generalized formula for the penetration depth of a deformable projectile [J]. International Journal of Impact Engineering, 2002, 27(4): 387–398. DOI: 10.1016/S0734-743X(01)00061-6. [90] 孙庚辰, 吴锦云, 赵国志, 等. 长杆弹垂直侵彻半无限厚靶板的简化模型 [J]. 兵工学报, 1981(4): 1–8.SUN G C, WU J Y, ZHAO G Z, et al. A simplified model of the penetration of the long-rod penetrator against the plates with semi-infinite thickness at normal angle [J]. Acta Armamentarii, 1981(4): 1–8. [91] WARREN T L. Simulations of the penetration of limestone targets by ogive-nose 4340 steel projectiles [J]. International Journal of Impact Engineering, 2002, 27(5): 475–496. DOI: 10.1016/S0734-743X(01)00154-3. [92] WEN H M. Predicting the penetration and perforation of FRP laminates struck normally by projectiles with different nose shapes [J]. Composite Structures, 2000, 49(3): 321–329. DOI: 10.1016/S0263-8223(00)00064-7. [93] WEN H M. Penetration and perforation of thick FRP laminates [J]. Composites Science and Technology, 2001, 61(8): 1163–1172. DOI: 10.1016/S0266-3538(01)00020-3. [94] WEN H M. Predicting the penetration and perforation of targets struck by projectiles at normal incidence [J]. Mechanics of Structures and Machines, 2002, 30(4): 543–577. DOI: 10.1081/SME-120015076. [95] LI Q M, REID S R, WEN H M, et al. Local impact effects of hard missiles on concrete targets [J]. International Journal of Impact Engineering, 2005, 32(1–4): 224–284. DOI: 10.1016/j.ijimpeng.2005.04.005. [96] TELAND J A, SJØL H. Penetration into concrete by truncated projectiles [J]. International Journal of Impact Engineering, 2004, 30(4): 447–464. DOI: 10.1016/S0734-743X(03)00073-3. [97] LI Q M, CHEN X W. Dimensionless formulae for penetration depth of concrete target impacted by a non-deformable projectile [J]. International Journal of Impact Engineering, 2003, 28(1): 93–116. DOI: 10.1016/S0734-743X(02)00037-4. [98] CHEN X W, FAN S C, LI Q M. Oblique and normal perforation of concrete targets by a rigid projectile [J]. International Journal of Impact Engineering, 2004, 30(6): 617–637. DOI: 10.1016/j.ijimpeng.2003.08.003. [99] BEN-DOR G, DUBINSKY A, ELPERIN T. Estimation of perforation thickness for concrete shield against high-speed impact [J]. Nuclear Engineering and Design, 2010, 240(5): 1022–1027. DOI: 10.1016/j.nucengdes.2009.12.029. [100] BEN-DOR G, DUBINSKY A, ELPERIN T. Shape optimization of impactor penetrating into concrete or limestone targets [J]. International Journal of Solids and Structures, 2003, 40(17): 4487–4500. DOI: 10.1016/s0020-7683(03)00212-9. [101] FREW D J, FORRESTAL M J, HANCHAK S J. Penetration experiments with limestone targets and ogive-nose steel projectiles [J]. Journal of Applied Mechanics, 2000, 67(4): 841–845. DOI: 10.1115/1.1331283. [102] 王松川. 弹体斜侵彻弹道快速预测方法研究[D]. 长沙: 国防科学技术大学, 2011: 28−34.WANG S C. Quick prediction method of oblique penetration trajectory [D]. Changsha: National University of Defense Technology, 2011: 28−34. [103] 康海峰, 代廷静, 沈培辉, 等. 弹体形状对侵彻弹道的影响分析 [J]. 弹箭与制导学报, 2012, 32(2): 73–76. DOI: 10.3969/j.issn.1673-9728.2012.02.020.KANG H F, DAI T J, SHEN P H, et al. The analysis of the influence of projectile’s shape on penetration trajectory [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2012, 32(2): 73–76. DOI: 10.3969/j.issn.1673-9728.2012.02.020. [104] 闪雨, 黄风雷, 武海军, 等. 动能弹非正侵彻弹道稳定性研究[C]// 第六届全国强动载效应及防护学术会议暨2014年复杂介质/结构的动态力学行为创新研究群体学术研讨会论文集. 北京: 中国力学学会, 2014: 10. [105] 王浩, 武海军, 闫雷, 等. 椭圆横截面弹体斜贯穿双层间隔薄钢板研究 [J/OL]. 兵工学报, 2020: 1−12[2021-01-06]. https://kns.cnki.net/kns/brief/default_result.aspx. DOI: 10.3969/j.issn.1000-1093.2020.S2.001.WANG H, WU H J, YAN L, et al. Research on oblique perforation of truncated ogive-nosed projectiles with elliptic cross-section into double-layered thin steel plate with gap space [J/OL]. Acta Armamentarii, 2020: 1−12[2021-01-06]. https://kns.cnki.net/kns/brief/default_result.aspx. DOI: 10.3969/j.issn.1000-1093.2020.S2.001. [106] 周航, 孔纲强, 曹兆虎, 等. 椭圆形孔扩张弹性分析 [J]. 固体力学学报, 2015, 36(1): 85–91. DOI: 10.19636/j.cnki.cjsm42-1250/o3.2015.01.011.ZHOU H, KONG G Q, CAO Z H, et al. Elastic analysis of elliptical cavity expansion [J]. Chinese Journal of Solid Mechanics, 2015, 36(1): 85–91. DOI: 10.19636/j.cnki.cjsm42-1250/o3.2015.01.011. [107] 周航, 孔纲强, 刘汉龙. 饱和土体中椭圆孔扩张弹塑性解 [J]. 岩土工程学报, 2014, 36(5): 983–988. DOI: 10.11779/CJGE201405025.ZHOU H, KONG G Q, LIU H L. Elasto-plastic solution for elliptical cavity expansion in saturated soils [J]. Chinese Journal of Geotechnical Engineering, 2014, 36(5): 983–988. DOI: 10.11779/CJGE201405025. 期刊类型引用(6)
1. 陈柏翰,赵生伟,邹慧辉,王伟光,戴湘晖,王可慧. 侵彻弹体过载信号特性与处理技术研究进展. 兵工学报. 2024(09): 2906-2928 .  百度学术
百度学术2. 刘晴昊,李玉琼,李娜,薛健,崔高瞻,邹欧. 土壤原位触探技术与贯入机理研究进展. 光学精密工程. 2023(05): 588-620 .  百度学术
百度学术3. 王振宁,尹建平,李旭东,伊建亚,张渝. 有限厚钢筋混凝土参数对破片侵彻性能的影响. 兵器装备工程学报. 2023(05): 180-185 .  百度学术
百度学术4. 杨云逸. 混凝土抗侵彻的离散化描述及关键因素分析. 山西建筑. 2023(21): 46-51 .  百度学术
百度学术5. 李国辉,刘基程,耿汉生,马林建,李增. 岩石高速-超高速侵彻效应研究进展. 防护工程. 2023(05): 67-78 .  百度学术
百度学术6. 邵伟,范锦彪,耿宇飞,王玮. 基于微元法的侵彻体弹头摩擦升温计算方法. 探测与控制学报. 2022(02): 34-40 .  百度学术
百度学术其他类型引用(7)
-








 下载:
下载:






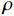













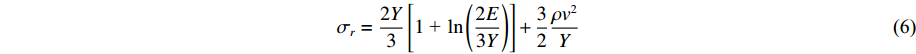
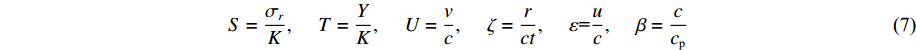





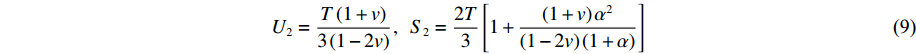
























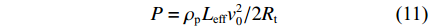


















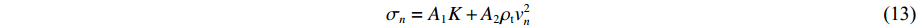






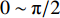


















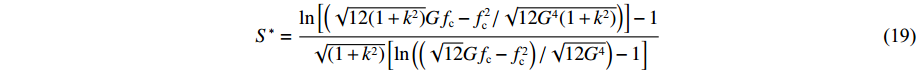









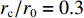










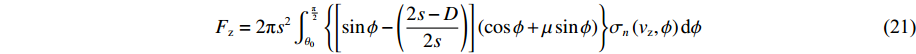





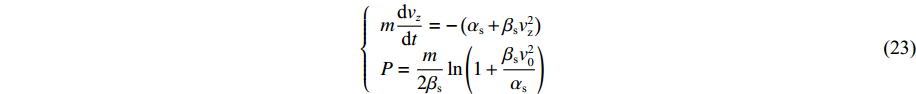





 下载:
下载:







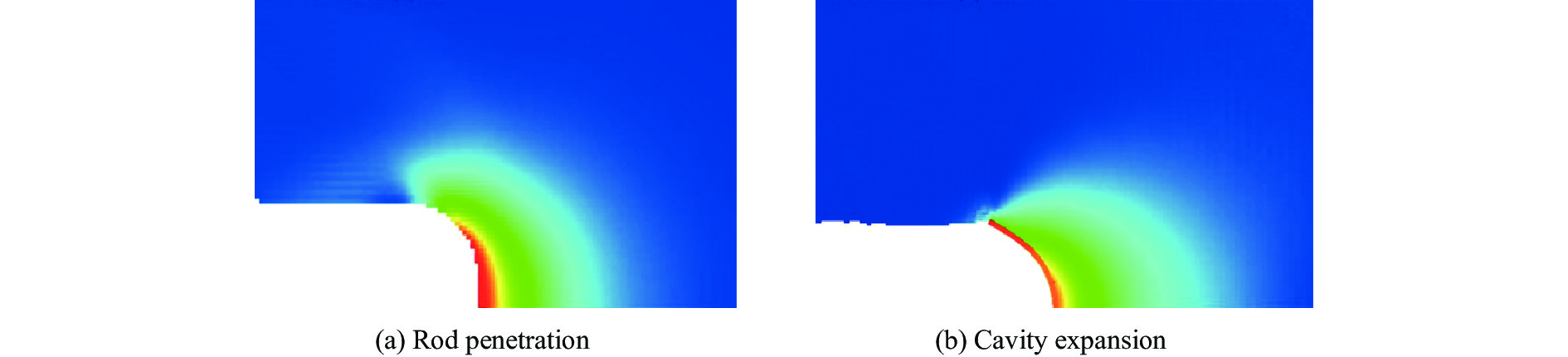






 百度学术
百度学术