爆炸载荷作用下铀气溶胶形成机理研究

刘志勇; 王金涛; 何彬; 罗永锋; 王飞

doi:10.11883/bzycj-2021-0075

爆炸载荷作用下铀气溶胶形成机理研究

doi: 10.11883/bzycj-2021-0075

刘志勇^{1, 2,},
王金涛^1, ,,
何彬¹,
罗永锋²,
王飞¹

1.
火箭军工程大学核工程学院，陕西西安 710025
2.
火箭军研究院，北京 100015

详细信息

作者简介:
刘志勇（1982－　），男，博士研究生，副研究员，ageleil@163.com

通讯作者:
王金涛（1985－　），男，博士，讲师，451128257@qq.com

中图分类号: O385
计量
- 文章访问数: 476
- HTML全文浏览量: 295
- PDF下载量: 122
- 被引次数: 1
出版历程
- 收稿日期: 2021-03-03
- 修回日期: 2021-04-16
- 刊出日期: 2021-05-05

Study on the formation mechanism of uranium aerosol under explosion load

LIU Zhiyong^{1, 2
,},
WANG Jintao^{1
, ,},
HE Bin¹,
LUO Yongfeng²,
WANG Fei¹

1.
Department of Nuclear Engineering, Rocket Force University of Engineering, Xi’an, 710025, Shaanxi, China
2.
Academy of Rocket Force, Beijing 100015, China

摘要

摘要: 针对铀材料在爆炸载荷作用下形成放射性气溶胶的过程，采用光滑粒子流体动力学方法开展了数值模拟和实验研究。通过将颗粒动力学和SPH方法结合，建立了炸药爆轰作用于铀金属壳的数值模拟模型，以铀材料比内能为气溶胶转化判据，获得了铀材料转化为气溶胶的物理过程，得到了在相同爆炸当量下，不同质量铀材料的气溶胶转化效率，并与实验结果进行了对比分析。结果显示，铀材料在爆炸载荷作用下，当其比内能达到1.9 MJ/kg时，即可认为完全转变为气溶胶，对于本文中的爆炸装置结构形式，当炸药质量为铀材料质量的6倍时，转化率超过90%。实验验证了数值模拟结果，表明该方法能够对铀材料的气溶胶转化过程进行准确描述。
- 铀 /
- 气溶胶 /
- 爆炸载荷 /
- 光滑粒子流体动力学方法
Abstract: Aiming at the process of uranium material forming radioactive aerosol under the action of explosion load, numerical simulation and experimental research were carried out based on the smoothed particle hydrodynamic method (SPH method). Through the combination of particle dynamics and SPH method, a numerical simulation model of explosive detonation acting on a uranium metal shell was established, which would be used to describe the formation process of uranium aerosol. The specific internal energy of uranium material was used as the aerosol conversion criterion, and the physical process of uranium material conversion into aerosol was obtained. We found two types of damage mode of the uranium under explosive load, one was overall damage when the uranium shell mass was close to the explosive mass, and the other one was crushing damage when the uranium shell mass was much less than the explosive mass. Under the same explosive equivalent, the aerosol conversion efficiencies of uranium materials with different mass were compared with the experimental results. The results show that uranium material can be considered to be completely converted into aerosol when its specific internal energy reaches 1.9 MJ/kg under explosive load. According to the explosive device structure in this paper, when the explosive mass is six times than the mass of uranium, the conversion ratio exceeds 90%. The experimental results have a good agreement with the numerical simulations, which shows that the method used in this paper can accurately describe the aerosol conversion process of uranium materials.
- uranium /
- aerosol /
- explosion load /
- smoothed particle hydrodynamics method

HTML全文

铀材料是核能领域中具有重要应用的核材料，化爆问题^[1]是核事故中最为严重的一类事故形式，发生化爆事故将使铀材料在爆炸载荷作用下迅速转化为微小颗粒物，即具有放射性的气溶胶（本文所指气溶胶为粒径小于10 μm的大气漂浮颗粒物，即PM10，通常不考虑沉降问题），并在爆炸作用下被抛撒至周围环境中，在大气中不断扩散，对各类装备设施和人员造成极为严重的危害^[2]。对于此类问题的研究，通常可以分为两个阶段：一是爆炸载荷作用下铀气溶胶的形成过程，二是铀气溶胶形成后在大气环境中的扩散过程。其中，第一个问题的研究结果是第二个问题的输入条件，因此，必须首先对铀气溶胶的形成机理进行深入研究，对于科学评估放射性气溶胶的扩散规律，快速进行核事故应急处置，具有重要的理论意义和应用价值。目前，由于在爆炸载荷作用下，铀材料处于极端条件中，在极短时间内受到高温高压作用，加之铀材料具有放射性危害，因此，国内外对于放射性气溶胶的形成过程研究成果较少。

在爆炸载荷作用下金属材料的响应过程中，金属材料的损伤通常从宏观层面进行描述，例如侵蚀、断裂等损伤模式，在实验和数值仿真中都能够较为准确地对材料响应过程进行观测和分析。但是，金属材料破碎成微小颗粒物的过程，利用侵蚀、断裂等描述方法却无法加以分析和研究。这其中除了涉及材料的微观结构变化，还涉及诸多物理量的变化，特别是内能迅速升高与材料相变等等。周建辉等^[3]等对爆轰波对金属球壳的挤压作用进行了研究，通过数值仿真展示了爆轰波对金属球壳的压缩动态响应过程。王凯民等^[4]在考虑受主炸药盖片对撞击入射冲击波衰减作用的基础上，得到了改进的飞片起爆计算模型，经与实验结果对比表明这一改进模型更符合实际系统。这些研究成果对爆轰波与金属的相互过程进行了初步探索，但仍不足以描述气溶胶的形成。

20世纪60年代起，人们开始针对核事故场景中的钚气溶胶源项进行实验和理论研究，研究内容主要包括钚材料的氧化反应和气溶胶生成机制、核事故发生后钚气溶胶在事故源处的分布特性等。随着计算机的发展，人们开发了多种处理钚气溶胶扩散的计算模型。事故源项直接影响计算模型的选择和计算结果的精度，因此进行炸药爆炸条件下的现场试验，作为研究钚在炸药爆炸条件下源项特性的有效方法之一^[5]，能直接提供钚在炸药爆炸条件下的源项参数，但由于现场试验对环境影响大、代价高，所以现场实验数量较少，其中最著名的是1963年的project roller coaster实验^[6]。

Project roller coaster实验包含4个系列的试验：Clean Slate 1 (CS1)、Clean Slate 2 (CS2)、Clean Slate 3 (CS3)和Double Tracks (DT)。试验在美国内华达州Tonopah试验场进行，其中DT和CS1为外场试验，CS2和CS3为掩体内试验。DT试验中，装置在钢板上起爆。Project roller coaster实验中将包含炸药和钚材料的结构置于4种不同环境来模拟钚在不同环境中发生事故时的扩散状况，结果见表1^[6]。

表 1 Project Roller Coaster实验状态

Table 1. The status of Project Roller Coaster

试验代号	炸药质量/kg	环境	爆炸烟云高度/m
DT	53.5	钢板上	220
CS1	481.7	混凝土上	710
CS2	1016.9	掩体内	440
CS3	1016.9	掩体内	520

下载: 导出CSV

| 显示表格

在爆炸形成的高温高压条件下，钚（液滴或蒸气）粒子与空气混合，产生化学反应形成钚的氧化物。钚的氧化物有两种形式：Pu₂O₃和PuO₂。在空气中Pu₂O₃通常进一步氧化为PuO₂，该反应过程非常迅速^[7]。

Haschke等^[8]的研究表明在火灾实验中，要获得完整的气溶胶颗粒尺寸分布是很困难的。由于火灾事故通常为接近等压燃烧，对气溶胶颗粒的驱动能力不足，仅有较小尺寸的气溶胶颗粒会随上升气流飘散，较大尺寸的气溶胶颗粒则迅速沉积，因此通常火灾实验只能给出两部分的单独分布。Martz等^[9]提出：在爆炸作用下，对于炸药周围的裂变材料或其他有毒物质，一般会发生完全的气溶胶化。爆炸产生的高温、高压条件下，核材料产生相变，进一步发生化学反应。最终放射性气溶胶的粒径分布是与物质的化学性质和装置结构等密切相关的，且气溶胶粒径范围变化较大，有些粒子的平均空气动力学直径为20～40 μm，而有些粒子的平均空气动力学直径小于1 μm。源项中的粒径分布可以采用单个或多个对数粒径分布组合来表示。因此，计算中假设放射性物质已经形成了稳定粒径分布，并在后续扩散过程中保持该状态不变。

郝樊华等^[10]开展了化爆试验，以银金属为对象对爆轰条件下气溶胶生成量及粒径分布规律进行了实验研究。

刘文杰等^[11]对核事故条件下钚气溶胶源项的理论和实验研究进行了总结和评述，主要包括钚材料的氧化反应机制、气溶胶的颗粒尺寸分布以及核事故发生后气溶胶从事故源处释放的比率和可吸人率，进一步对比了炸药化学爆炸、静态燃烧及动态燃烧三类核事故场景中钚气溶胶的源项数据。静态燃烧与液滴未爆炸的动态燃烧条件下钚气溶胶的生成机制类似，但前者相对较低的气溶胶释放率源于反应过程中的热量损耗及固态材料对空气流动的阻碍；动态场景中，若液滴发生爆炸并泄露出大量钚蒸汽，将产生更高的气溶胶率及可吸入率；炸药爆炸条件下钚材料的氧化反应是最剧烈的，将产生最高的源项数据。

唐秀欢等^[12]针对核材料化爆事故，采用高斯烟团模型开展了气溶胶扩散过程计算，结果表明1 kg武器级钚在53.52 kg TNT爆炸作用下产生气溶胶，爆炸烟云高度可达249 m，气溶胶质量从顶部向下逐渐减小。

鉴于放射性气溶胶形成过程中核材料转化率为重要源项问题，本文基于光滑粒子动力学（smoothed particle hydrodynamics, SPH）方法^[13-14]，开展系统的理论和数值仿真研究，分析金属铀在爆炸载荷作用下的气溶胶转化的物理过程，计算气溶胶转化效率，并开展相应的实验对计算结果进行验证。

1. 铀气溶胶形成过程的数值仿真

通过数值模型设计，针对内置铀金属球体在球形装药起爆、爆轰波传播、爆轰波冲击挤压等过程中发生的变形、破坏、气化等现象进行数值模拟，建立爆轰波发生发展及铀金属的毁伤效应模型，求解铀金属球壳在爆炸载荷作用下的物理图像，探索不同质量铀金属球壳在相同爆炸载荷下的气溶胶转化率的变化规律。

1.1 数值仿真模型

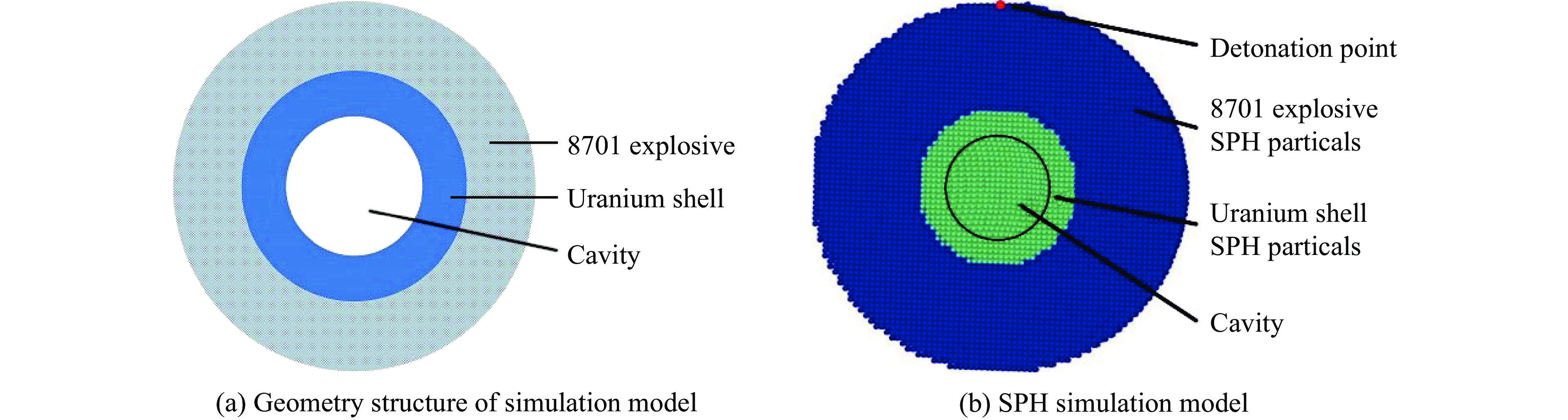
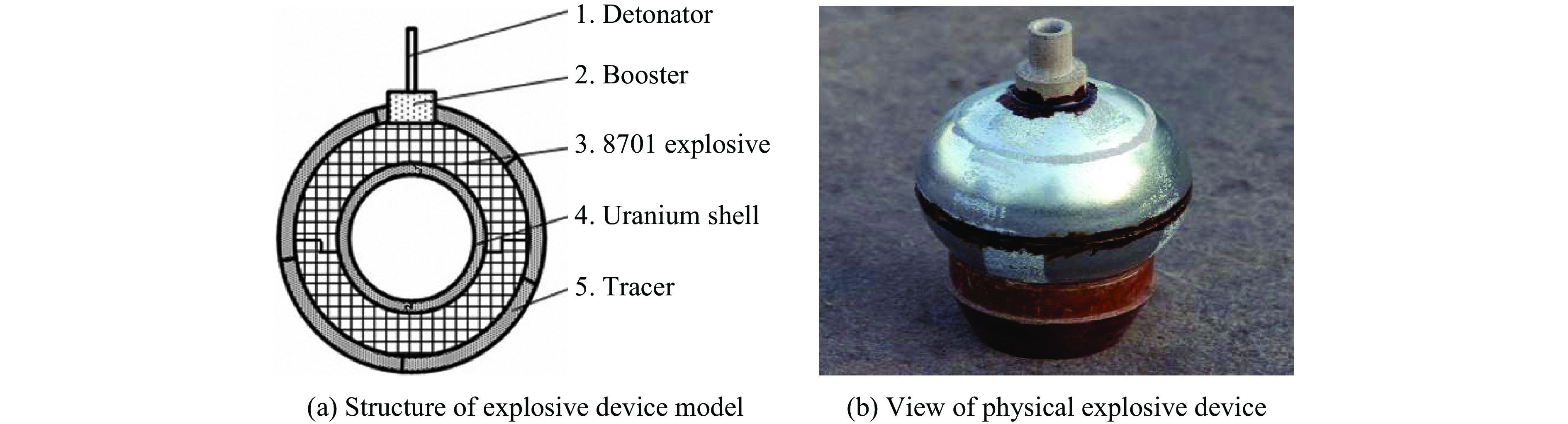
对于外层炸药、内层铀金属的两层球壳结构，首先建立等比例几何模型，其次对几何模型进行有限元网格划分，网格尺寸保持一致均匀，最后将网格转化为SPH粒子^[15]。作为数值模型中的粒子模型，即无网格粒子模拟需要的模型。对于网格与粒子之间的转化采用自主编写的转化程序完成，该程序采用几何等效原则，将单个网格转化为相对应的一个粒子，网格与粒子体积相同，粒子中心的坐标与网格的重心相重合。爆炸装置模型剖面如图1所示，图1（b）所示为三维SPH模型的1/2剖面。炸药为8701炸药，形状为空心球形壳体，内径30 mm，外径70 mm，质量为280 g，爆炸威力为350 g TNT当量；铀金属包裹于炸药件内部，外径30 mm，通过改变其内径达到改变铀金属质量的目的，数值模拟模型共设计10种同质量的铀金属球壳，内径从16 mm增加至28 mm，编号1～10所对应的铀金属球壳内径依次为16、18、20、22、24、26、26.5、27、27.5和28 mm。

图 1 爆炸装置数值模拟模型

Figure 1. Numerical simulation model of the explosive device

下载: 全尺寸图片幻灯片

爆轰波的发生发展及其对铀壳体的冲击挤压过程主要涉及爆轰起爆、爆轰波发展、爆轰波传播、爆轰物质相态转变、铀壳体的损伤破坏、铀材料的相态转变等过程，需要建立的物理模型包括爆轰气体运动模型、爆轰产物状态方程、爆轰波传播物理模型、铀材料的本构模型、铀材料的气溶胶转化准则等。本文中铀材料的本构模型采用Johnson-Cook本构模型^[16-18]，同时对于铀壳体的正压力采用Mie-Grüneisen状态方程进行描述^[19-21]；炸药的爆轰过程采用能够反映由爆轰到膨胀整个过程的JWL模型进行描述^[22-23]。

1.1.1 铀金属的材料模型

为准确描述炸药内部铀材料在挤压冲击过程中受到强冲击作用产生的屈服应力及损伤演化，在考虑应变率效应的基础上，本文选用Johnson-Cook模型，即材料的屈服强度 ${\sigma _{{\rm{eq}}}}$ 与温度T、等效应变 ${\varepsilon _{{\rm{eq}}}}$ 和等效应变率 ${\dot \varepsilon _{{\rm{eq}}}}$ 之间的关系为^[24]：

${\sigma _{{\rm{eq}}}} = \left( {A + B\varepsilon _{{\rm{eq}}}^n} \right)\left( {1 + C\ln {{\dot \varepsilon }_{{\rm{eq}}}}} \right)\left( {1 - {T^{*{{m}}}}} \right)$

(1)

式中：n、m、A、B、C为常数；T^*m=(T−T₀)/(T_m−T₀)，T₀是室温，T_m为熔点； ${\varepsilon _{{\rm{eq}}}} = (1 - D)\varepsilon$ ， ${\dot \varepsilon _{{\rm{eq}}}} = (1 - D)\dot \varepsilon /{\dot \varepsilon _0}$ ，ε为累积塑性应变， ${\dot \varepsilon _0}$ 为自定义的参考应变率；参数D表征了材料的损伤程度：当D=0时，表示材料损伤程度为0；当D=1时，表示材料已经完全达到失效状态；当0<D<1之间时，表示材料已出现部分失效情况，如产生裂纹等，此时可以设置一个临界的失效判据D_C。

参数D可以表示为ε的函数：

$D = \left\{ {\begin{array}{*{20}{l}} 0&\qquad \varepsilon \text{＜}{\varepsilon _{\rm{d}}}\\ {\dfrac{{{D_{\rm C}}}}{{{\varepsilon _{\rm{f}}} - {\varepsilon _{\rm{d}}}}}\varepsilon }&\qquad \varepsilon \text{≥} {\varepsilon _{\rm{d}}} \end{array}} \right.$

(2)

式中：ε_d为损伤阈值；ε_f为断裂塑性应变，与材料的应力三轴度、应变率和温度有关。

在J-C模型中，对于材料剪切损伤，可以用ε_f进行描述：

${\varepsilon _{\rm{f}}} = \left[ {{D_1} + {D_2}\exp \left( {{D_3}{\sigma ^*}} \right)} \right]\left( {1 + {D_4}\ln {p^*}} \right)\left( {1 + {D_5}{T^*}} \right)$

(3)

式中：D₁～D₅为常数；σ^*= $\bar \sigma$ /σ_eq为材料的应力三轴度， $\bar \sigma$ =(σ_x+σ_y+σz)/3为材料的平均正应力。

对于铀材料的状态方程，采用Grüneisen状态方方程形式进行描述，即压力p、密度ρ和比内能e之间的关系为

$p(\rho ,e) = \left( {1 - \frac{1}{2}\varGamma \eta } \right){p_{\rm{H}}}(\rho ) + \varGamma \rho e$

(4)

其中

$\eta = \frac{\rho }{{{\rho _0}}} - 1$

(5)

${p_{\rm H}} = \left\{ {\begin{array}{*{20}{l}} {{a_0}\eta + {b_0}{\eta ^2} + {c_0}{\eta ^3} }&\qquad{\eta \text{＞} 0} \\ {{a_0}\eta }&\qquad{\eta \text{＜} 0} \end{array}} \right.$

(6)

${a_0} = {\rho _0}C_{\rm S}^2,\;{b_0} = {a_0}\left[ {1 + 2\left( {{S_{\rm S}} - 1} \right)} \right],\;\;{c_0} = {a_0}\left[ {2\left( {{S_{\rm S}} - 1} \right) + 3{{\left( {{S_{\rm S}} - 1} \right)}^2}} \right]$

(7)

式中： $\varGamma = 1.99,{\rm{ }}{C_{\rm S}} = 3\;940,\;{\rm{ }}{S_{\rm S}} = 1.489$ 。

本文中所使用的铀材料为贫铀金属（U-238），其各项参数取值见表2^[25]。

表 2 铀金属的材料参数

Table 2. Material parameters of uranium metal

弹性模量/GPa	泊松比	密度/（kg·m⁻³）	A/MPa	B/MPa	n	C	D_C	ε_d	D₁	D₂	D₃	D₄	D₅
132.6	0.3	19 050	800	684	0.083	0.012	0.3	0	0.0705	1.732	−0.54	−0.0123	0

下载: 导出CSV

| 显示表格

1.1.2 炸药的材料模型

炸药爆炸过程是爆轰波在炸药材料中传播的过程，爆轰波是一种带有快速化学反应的强冲击波，理想情况下可认为炸药材料的化学分解反应是在爆轰波阵面上瞬时完成的，这就是经典的爆轰模型—C-J (Chapman-Jouguet)模型。爆轰产物状态方程采用能够反映由爆轰到膨胀整个过程的JWL方程：

$p(E,V) = A_1\left( {1 - \frac{w}{{{R_1}V}}} \right)\exp \left( { - {R_1}V} \right) + B_1\left( {1 - \frac{w}{{{R_2}V}}} \right)\exp \left( { - {R_2}V} \right) + \frac{{w{\rho _0}E}}{V}$

(8)

式中：v=ρ/ρ₀，为相对体积；E为体积内能；A₁、B₁、R₁、R₂、w为常量，与爆炸的环境、材料等因素有关，具体数值可以通过相关实验来获取。本文采用8701炸药，初始密度为1 700 kg/m³，爆速为8 315.0 m/s， A=854.5 GPa，B=2.049 GPa，R₁=4.2。JWL方程是一项得到成功应用的方程，它反映了爆轰气体产物从高压到低压的变化过程，对于爆轰结束后的整个气体产物的膨胀过程都能很好地描述。

1.1.3 SPH算法的实现

本文采用SPH方法对球形装药爆轰波高速挤压铀材料等高速冲击与爆炸问题进行数值模拟。在算法上面首先研究了具有材料强度的SPH流体动力学控制方程的离散，推导了含铀材料的J-C本构方程以及能量传递的离散方程；然后针对炸药爆轰过程中出现密度和光滑长度变化剧烈的问题，建立了适用于爆炸与冲击、大变形、大扭曲等问题的完全变光滑长度SPH方程组，修正了SPH的密度演化方程、动量守恒方程以及能量守恒方程以及与变光滑长度方程的关联效应，从根本上消除了变光滑长度效应在求解极端爆炸问题时产生的偏差；最后，针对铀材料超高速挤压中损伤后的碎片飞散问题，将基于颗粒动力学的SDPH (smoothed discrete particle hydrodynamics)方法^[26-28]引入本文中，处理铀材料受爆轰波挤压破坏后生成大量碎渣的问题。

在完成适用于爆炸与冲击等大变形求解的SPH算法研究的基础上，对SPH程序采用C++程序进行了编写^[29-31]。

控制铀材料气化的量为比内能，根据文献[6]，比内能e的取值范围为1.5～2.5 MJ/kg，间隔为0.1 MJ/kg进行取值。根据不同的比内能判据分别进行模拟计算，通过与实验数据的对比分析，最终确定合理的比内能值。本文中对于超过气化值的粒子直接按照惯性粒子进行计算，不再计算其与其他粒子之间的相互作用力及其能量属性等。

1.2 数值模拟分析

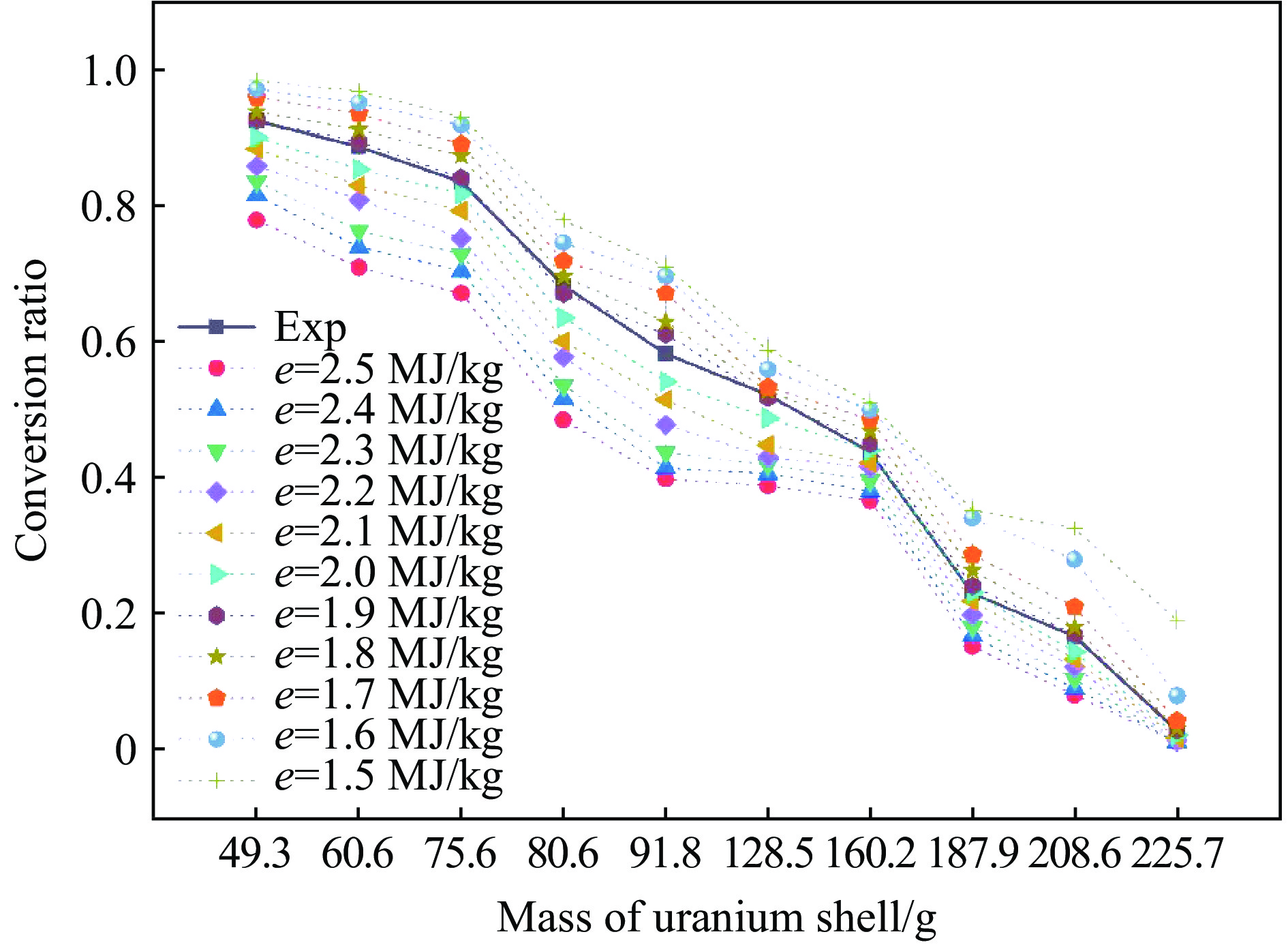
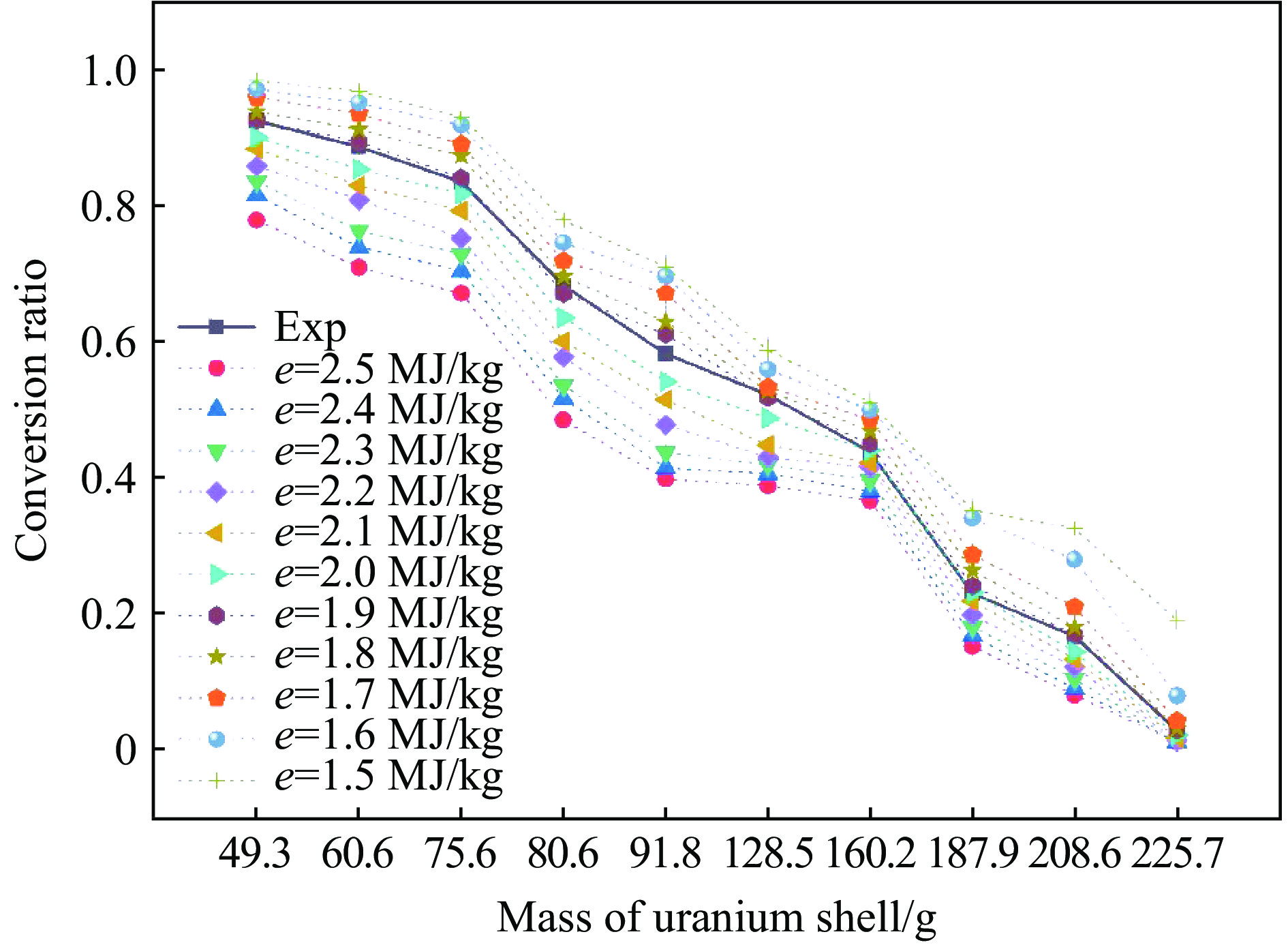
针对10种不同爆炸装置模型和不同的比内能取值，本文分别进行了计算对比，对于不同的比内能，不同质量铀金属球壳的转化率计算结果如图2所示，转化率定义为气溶胶的铀材料质量与铀材料初始质量之比。当铀材料的比内能e达到2.0 MJ/kg时^[7]，铀材料即转化为气溶胶。为进一步研究铀材料在爆炸载荷下的气溶胶转化效率，本文在数值模拟过程中，以e作为能量判据，分析铀材料的转化过程。从图2中可以看出，对于确定质量的铀金属球壳，在爆炸载荷作用下，所设置的比内能值越大，气溶胶转化率越低，这是因为模型中铀金属部分的SPH粒子的能量计算结果是一定的，作为遴选判据的比内能截断值越大，遴选出的SPH粒子数量就越少；同时，由于炸药质量不变，当铀金属球壳质量越小时，铀材料SPH粒子吸收的能量就越多，达到比内能判据值的SPH粒子数量就越多，转化率相应就越高。

图 2 不同比内能截断值时气溶胶转化率计算结果

Figure 2. Calculation results of aerosol conversion ratio at different specific internal energy cut-off values

下载: 全尺寸图片幻灯片

首先，通过提取转化率数值并进行对比分析，发现当比内能e=1.9 MJ/kg时，铀材料转化为气溶胶的转化率与实验结果最为接近，此时对于不同结构的爆炸装置，转化率的实验结果与计算结果对比如表3所示。

表 3 转化率的实验与模拟结果对比(%)

Table 3. Comparison of experimental and simulational results of conversion ratio (%)

实验编号	实验结果	计算结果	绝对误差	实验编号	试验结果	计算结果	绝对误差
1	2.75	2.80	−0.05	6	58.38	61.30	−2.92
2	16.68	16.92	−0.24	7	68.48	67.26	1.22
3	23.03	24.12	−1.09	8	83.75	84.33	−0.58
4	43.87	45.03	−1.16	9	88.91	89.64	−0.73
5	52.29	51.91	0.38	10	92.80	92.85	−0.05

下载: 导出CSV

| 显示表格

通过对结果进行分析发现，球形装药在起爆发生发展的过程中，其结构参数相同，铀材料的物理状态也基本相同，而由于内径不同，球壳厚度也不同，直接造成受挤压变形破坏后的形态也不同，但是通过深入分析发现，基本可以分为两种形态：一种为较厚壳体受冲击挤压下由于气化的物质较少，且处于球壳中心，因此球壳保持连续状封闭状态，二是当壳体厚度较小时，受冲击挤压气化的质量较多，球壳呈现碎片状破碎。因此，为了更详细地分析和展示这两种不同的现象，将两种状态下的炸药爆轰与球壳破坏过程进行分析。

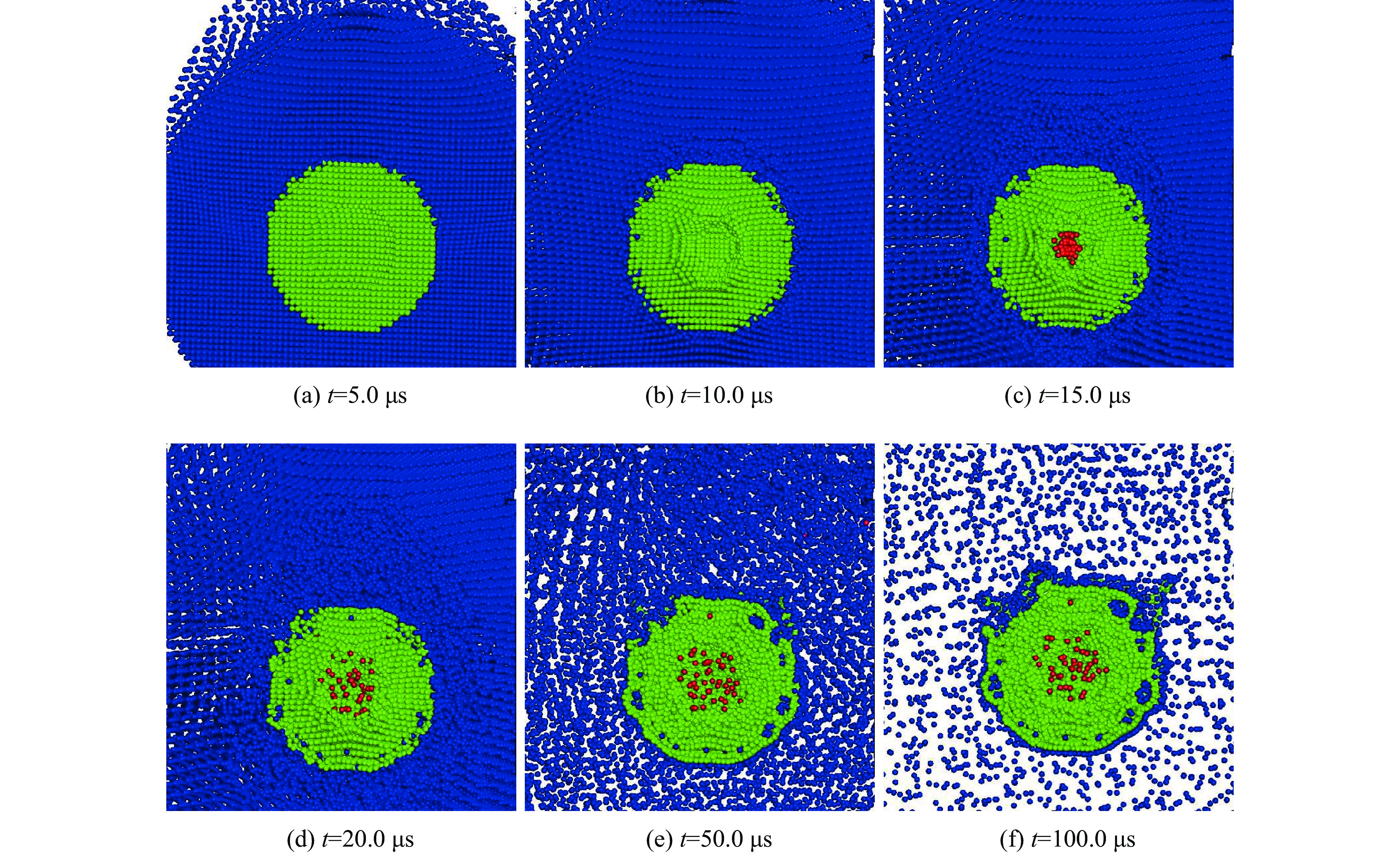
1.2.1 内径为16 mm铀金属壳破坏过程分析

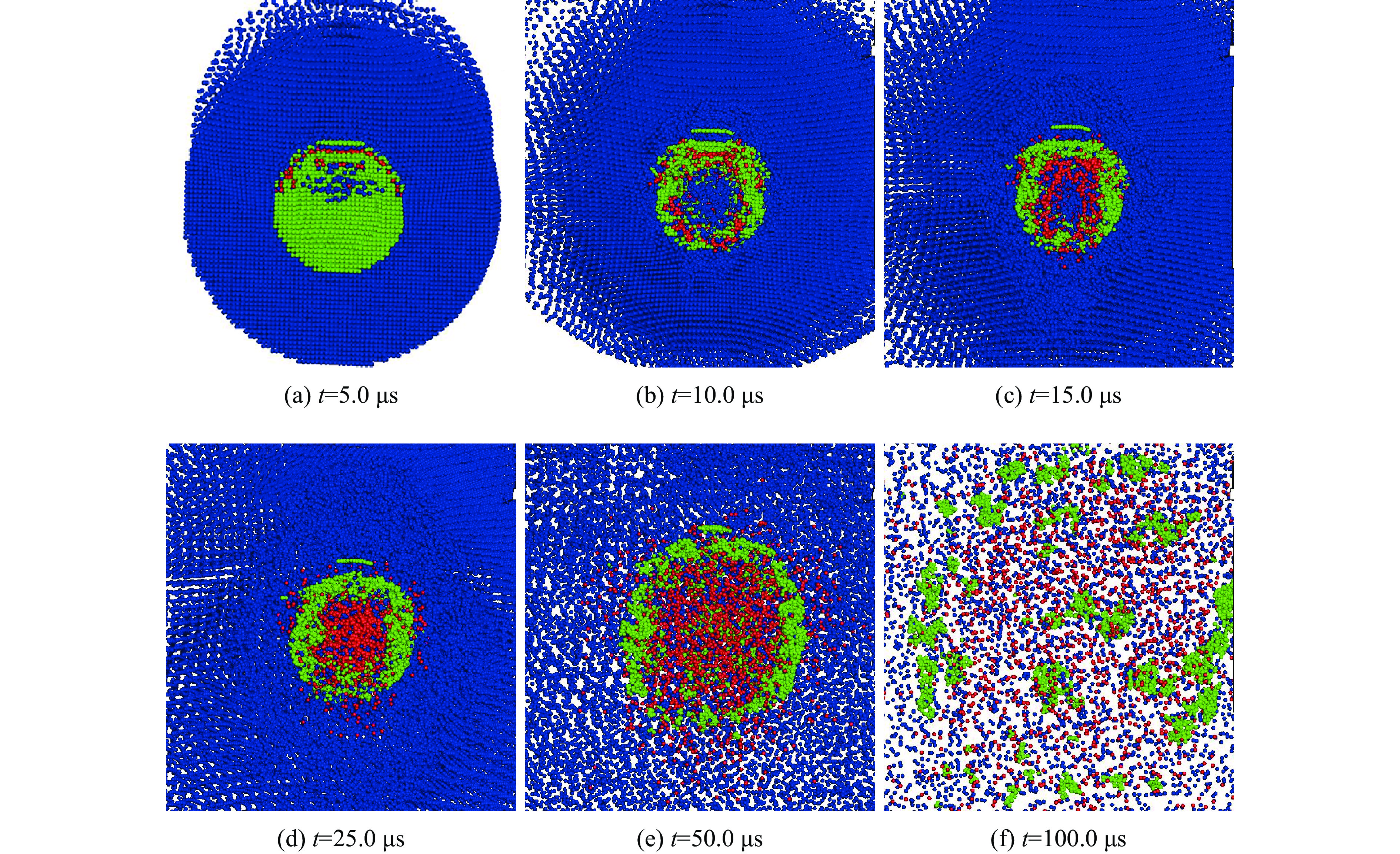
炸药材料发生爆轰对铀材料的压缩、推动过程如图3所示，蓝色粒子代表炸药材料，绿色粒子代表铀材料。图3反映出了球壳装药中爆轰波对材料的压缩破坏特点。当t=5.0 μs时，铀材料在爆轰波作用下开始运动；当t=10.0 μs时，在“三点钟”和“九点钟”方向上的铀球壳开始被爆轰波压缩；当t=15.0 μs左右，铀球壳模型的“三点钟”、“九点钟”和“六点钟”方向出现类似聚能射流的高速粒子现象，粒子能量值达到气化转变值，粒子转变为气体粒子，采用红色标注，这些粒子的速度明显高于其附近粒子的速度值。当t=10.0 μs时，爆轰波绕射传播结束，同时铀球壳向中心聚集的能量达到最大之后，铀球壳开始向外反弹，中心的气化粒子分布也变得更加均匀。

图 3 铀壳体受冲击挤压气化转变过程

Figure 3. Gasification transformation process of uranium shell under impact extrusion

下载: 全尺寸图片幻灯片

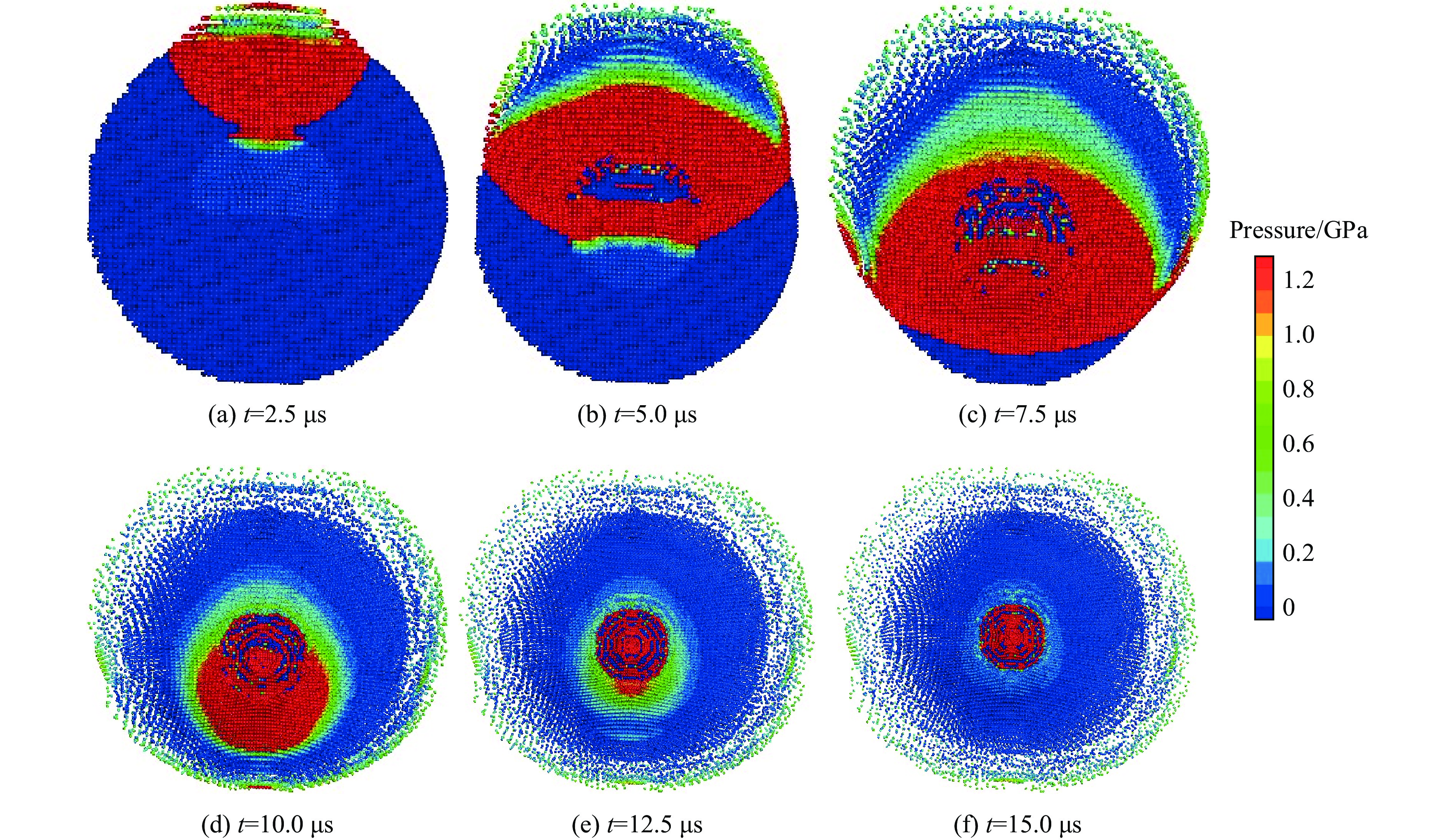
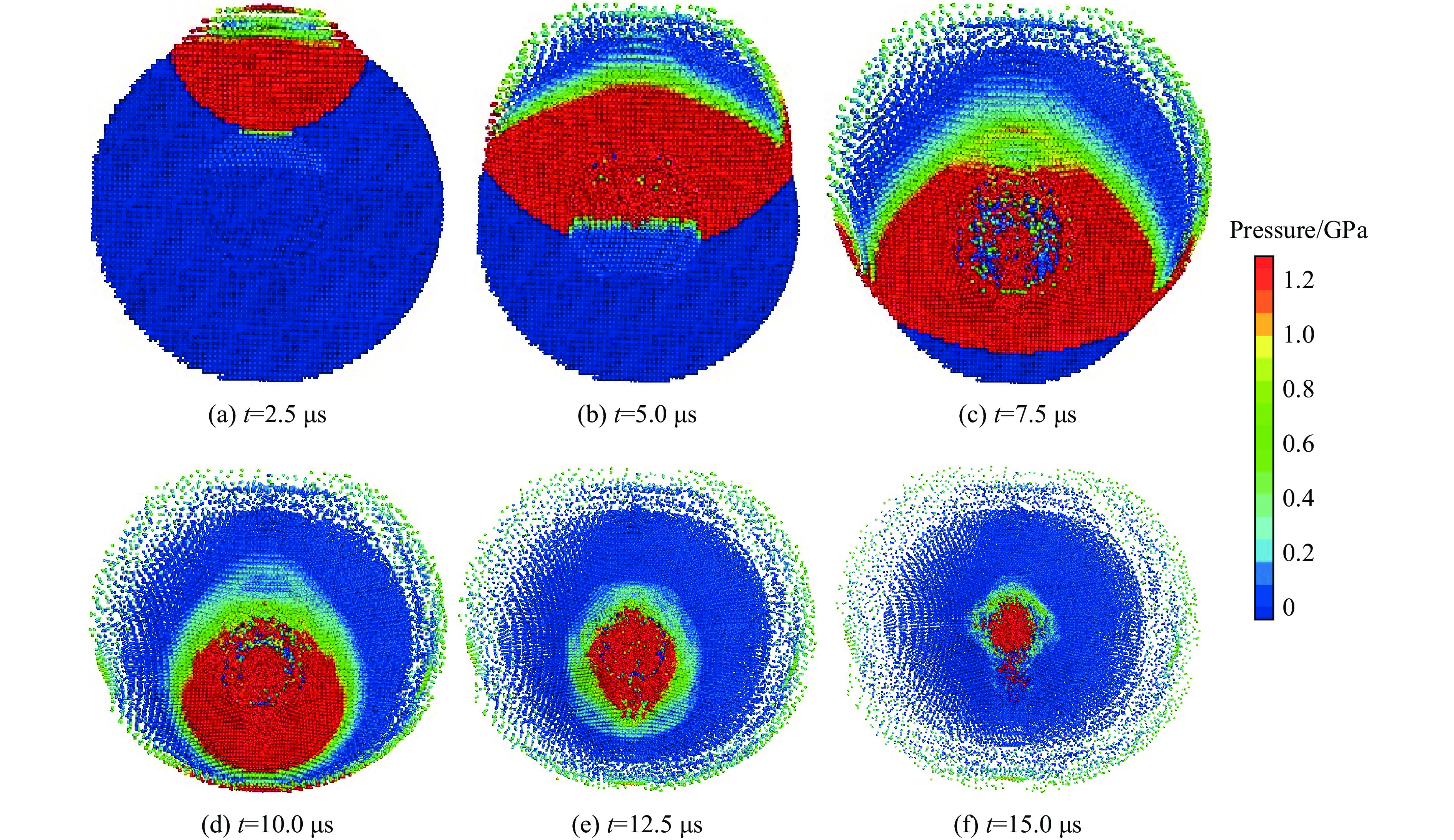
图4为压力传播的过程，可以看出高压区逐渐由起爆之后的扇形区向下方扩展，由2.5 μs的“一点钟”到5.0 μs的“两点钟”再到7.5 μs的“五点钟”，直到10.0 μs之后爆轰波绕流结束，最后高压区完全汇聚到中心铀球壳，实现了能量汇聚的结果，造成中心铀球壳结构的挤压破坏。

图 4 压力传播过程（铀壳内径16 mm）

Figure 4. Pressure propagation process (inner diameter: 16 mm)

下载: 全尺寸图片幻灯片

从16 mm内径球壳受冲击后的损伤破坏来看，其气化转化率较低，约为2.799%，同时气化转化的物质位于球壳内径边缘，因此在爆轰结束初期，整个球壳的损伤破坏不明显，整个球壳基本保持界面的连续性，随着爆轰波的传播与释放，球壳也伴随着收缩与扩展，整个球壳将解体为较大的块状体，气化的物质位于球壳的中心部位。

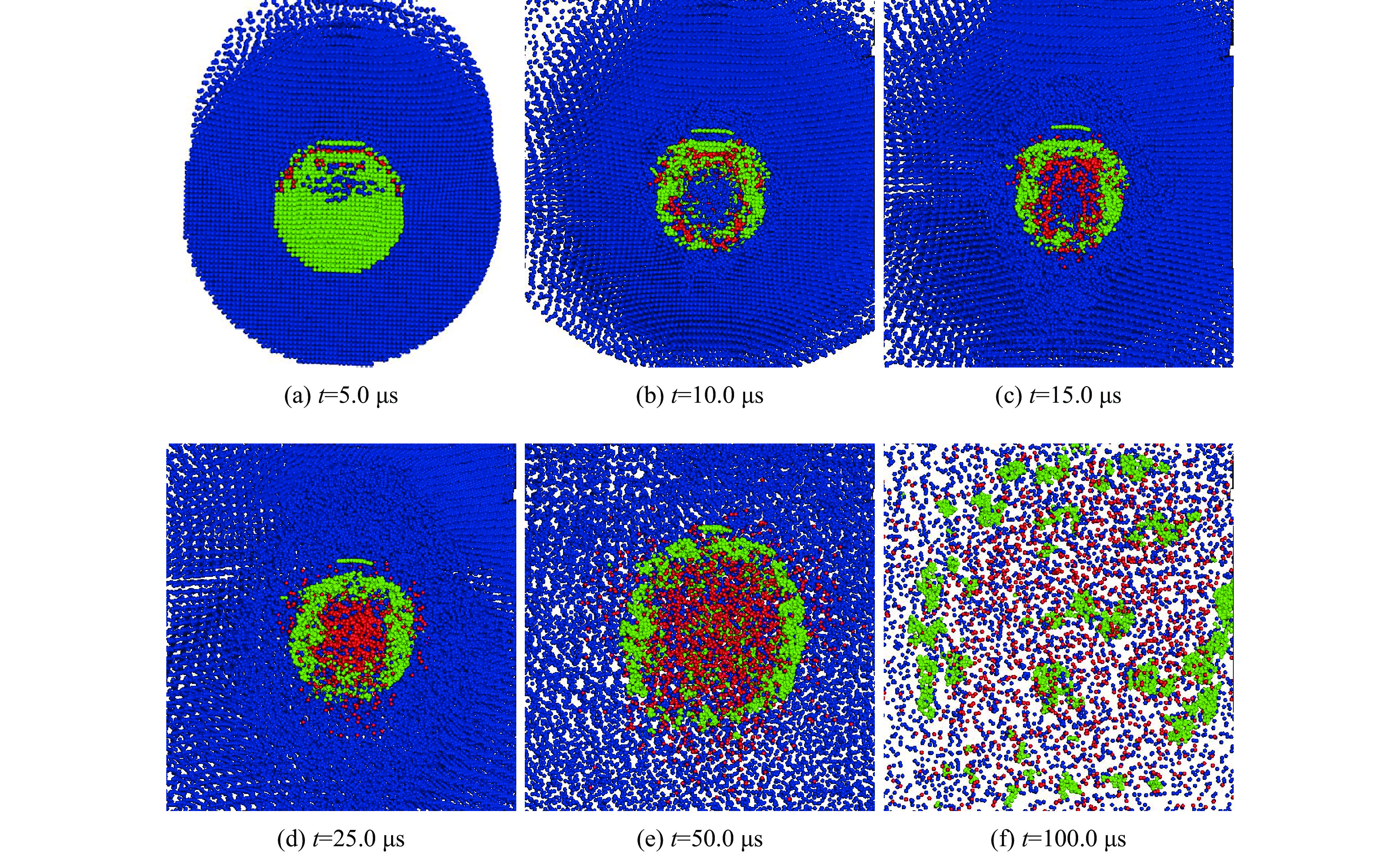
1.2.2 内径为26 mm铀金属壳破坏过程分析

对于较厚的铀壳体结构来说，其破坏过程呈现另一独特的现象，即气化质量达到一定程度后，球壳的界面也将发生破碎，由连续型界面演变为离散的碎片，中心气化后的粒子不受界面的制约而随着炸药的扩散而向空间扩散。图5为铀金属球壳的破坏细节展示：从5.0 μs开始，在球壳的外层首先有个别粒子达到气化能量发生相变；从10.0 μs开始，气化主要出现在球壳中心位置处，随着时间发展，气化物质增多；在25.0 μs基本达到峰值；而后在爆轰波扩散的条件下，中心球壳向四周扩散形成离散的碎片。

图 5 铀壳体气化转变及碎片运动过程

Figure 5. Uranium shell gasification transformation and fragment movement process

下载: 全尺寸图片幻灯片

图6为压力传播过程，可清晰地看到高压区逐渐由起爆点沿着环形球面向下绕流传播，在10.0 μs附近绕流传播结束，所有高压区逐渐向中心球壳汇聚，到15.0 μs时炸药上的高压区全部耗散转化完成，能量全部集中在中心球壳，达到了高能汇聚的目的。

图 6 压力传播过程（铀壳内径26 mm）

Figure 6. Pressure propagation process (inner diameter: 26 mm)

下载: 全尺寸图片幻灯片

2. 实验分析

为准确采集铀气溶胶的数量，同时防止放射性材料的泄露，实验在密闭爆炸容器中进行。爆炸容器由16MnR特种钢焊接而成，设计指标为爆炸当量不大于500 g TNT，瞬态载荷峰值不高于25 MPa。图7为爆炸容器结构示意图。

图 7 爆炸试验容器结构示意图

Figure 7. Structure of the explosive container

下载: 全尺寸图片幻灯片

2.1 实验装置设计

为研究不同爆炸载荷下铀金属气溶胶的转化效率，在爆炸威力不变的情况下，通过改变铀金属质量，达到改变铀吸收能量的目的。爆炸装置设计如图8所示，主体设计与数值计算模型一致，不同之处主要是增加了雷管、引爆药，并且在炸药球壳的外层包覆了一层示踪剂。加入示踪剂的主要目的是考核爆炸气溶胶分散均匀性，为了减小示踪剂的数据误差，采用了3种微米级的钨粉、银粉和铜粉示踪剂。由于示踪剂的颗粒直径为微米级，在爆炸时可以认为其全部转化为气溶胶，这样在进行气溶胶采集时，就可以对部分气溶胶样品进行采集分析，然后通过所采集的示踪剂质量占总质量的比值，换算出铀气溶胶的总质量。

图 8 爆炸装置结构

Figure 8. View of the explosive device

下载: 全尺寸图片幻灯片

2.2 实验数据分析

气溶胶采集装置为CXF-4B向心式粒度分级采样器；同时，为监测采集过程中是否有气溶胶泄漏，使用放射性气溶胶连续监测仪监测环境放射性；使用表面污染检测仪测量滤膜、容器内壁上铀气溶胶的放射性活度；运用化学分析方法分析滤膜上各种金属气溶胶的质量和成分。示踪剂用于校正采集过程中由于气溶胶分布均匀性等因素带来的误差。

首先通过测量滤膜上α粒子的计数反演吸附的铀气溶胶质量，并根据向心式粒度分级采样器的粒度分级特性，求出放射中位空气动力学直径（记为D_m）和相应的几何标准偏差。经过计算可得，装置起爆后形成的铀气溶胶D_m的分布范围为3.5～7.0 μm，几何标准偏差为3～4。可见，铀材料在爆炸载荷下形成的是一种以大粒径为主并且粒度分布范围较广的气溶胶。

对于装置上附着的其他金属示踪剂所形成的气溶胶，通过化学方法将其制成硝酸盐溶液，使用等离子体原子发射光谱仪对溶液进行测量分析，可以获取相应金属的含量。

通过采集和分析，得出爆炸容器内铀气溶胶的质量和转化率，结果如表4所示。可以看到，在爆炸载荷不变的情况下，随着铀材料质量逐渐降低，铀气溶胶的转化率逐渐增大，当铀材料质量为225.7 g时，气溶胶转化率仅为2.71%，仅有少量的铀材料转化为了气溶胶；而当铀材料的质量为49.3 g时，气溶胶转化率达到了92.8%。这表明，铀材料在爆炸载荷作用下，单位质量材料吸收的能量越多，气溶胶转化效率越高，即当铀材料在受到爆炸作用后，其比内能越大，气溶胶转化率越高。

表 4 不同实验工况下铀气溶胶的质量和转化率

Table 4. Mass and conversion ratio of uranium aerosol among different experiments

试验编号	铀气溶胶质量/g	铀壳质量/g	转化率/%
1	6.2	225.7	2.75
2	34.8	208.6	16.68
3	43.3	187.9	23.03
4	86.3	160.2	43.87
5	67.2	128.5	52.29
6	53.6	91.8	58.38
7	55.2	80.6	68.48
8	48.2	75.6	83.75
9	53.8	60.6	88.91
10	45.7	49.3	92.80

下载: 导出CSV

| 显示表格

3. 实验与数值模拟对比分析

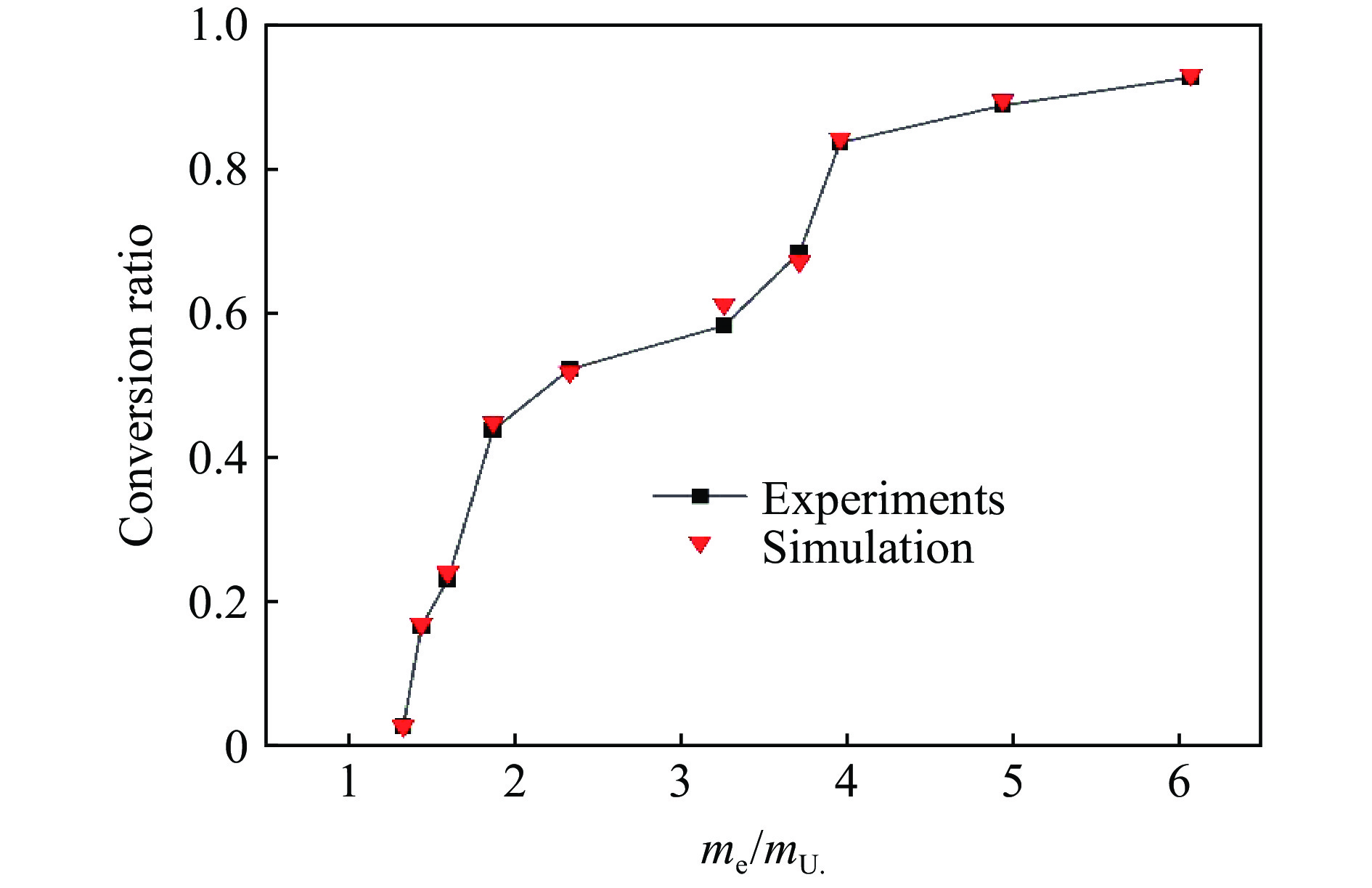
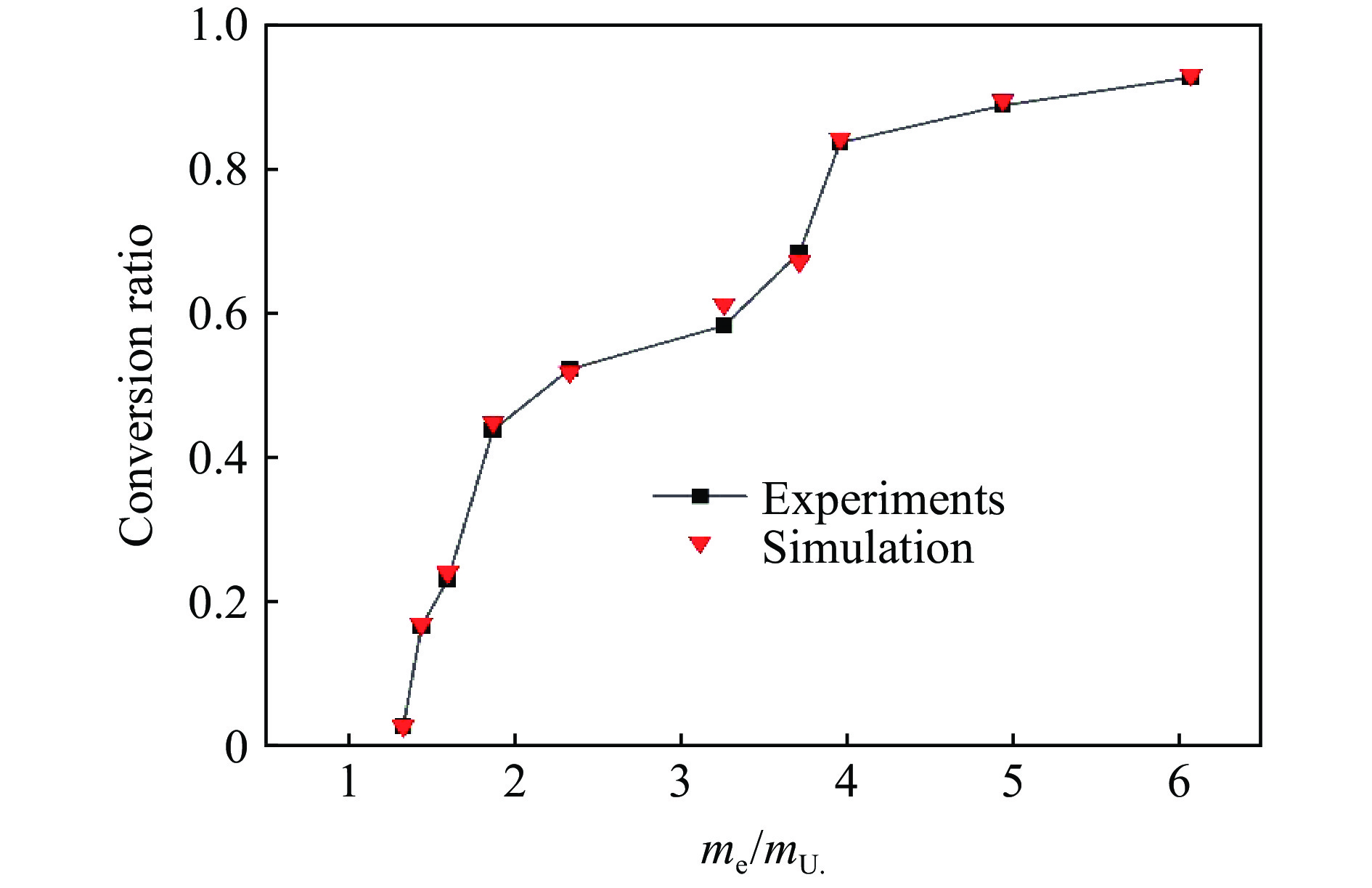
根据试验数据和仿真结果，进一步分析转化率的规律。由于在所有试验和模拟工况中，炸药质量不变，而铀壳质量一直在变，为更好地分析二者之间的关系，本文定义药壳质量比为炸药质量（m_e）与铀球壳质量（m_U）之比，药壳质量比与转化率之间的关系如图9所示，这一关系的物理意义更加明确，可以看到，当炸药质量与球壳质量接近时，铀材料的气溶胶转化率很低，但当炸药质量为铀质量的2倍时，转化率已接近50%，可见，在药壳质量比较低时，对转化率影响较大。当药壳质量比从2继续增大时，转化率的增幅逐渐降低，药壳质量比增大至6以上时，转化率已超过90%。

图 9 药壳质量比与转化率的关系

Figure 9. The relationship between explosive uranium mass ratio and conversion ratio

下载: 全尺寸图片幻灯片

通过以上分析可以看到，铀材料从固体金属向气溶胶的转化主要取决于铀材料在爆炸载荷作用下的比内能达到1.9 MJ/kg这一临界值的数量，在这一判据条件下，对于本文所设计的爆炸装置而言，药壳质量比是一个较好的能够反映气溶胶转化率的变量。本文基于SPH方法的物理算法所设计的模拟程序，计算结果与实验吻合较好，能够较好地反映铀材料在爆炸载荷作用下转变为气溶胶的物理过程，同时获取比实验更加丰富的数据。

4. 结　论

本文建立了基于SPH方法的铀球壳在爆炸载荷作用下的数值模型。在SPH理论框架下，对爆炸与冲击作用模型进行了研究，设计开发了相应的计算程序，对10种爆炸装置模型进行了计算，分析了在爆炸载荷作用下铀材料的运动过程，得到了在炸药球壳质量为350 g TNT当量情况下，铀气溶胶转化率随铀材料质量和药壳质量比之间的变化规律。

根据数值仿真计算结果，设计开展了铀球壳在爆炸载荷作用下气溶胶的采集和分析实验。通过一系列设计验证，开展了铀材料放射性气溶胶的真品试验和样品测试，获取了大量的实验数据。结果显示，气溶胶转化效率与数值仿真吻合较好，验证了数值模型的科学可靠，表明基于SPH方法的数值模拟程序可以用于金属材料在爆炸载荷条件下气溶胶转化过程的研究。

图 1 爆炸装置数值模拟模型

Figure 1. Numerical simulation model of the explosive device

下载: 全尺寸图片幻灯片

图 2 不同比内能截断值时气溶胶转化率计算结果

Figure 2. Calculation results of aerosol conversion ratio at different specific internal energy cut-off values

下载: 全尺寸图片幻灯片

图 3 铀壳体受冲击挤压气化转变过程

Figure 3. Gasification transformation process of uranium shell under impact extrusion

下载: 全尺寸图片幻灯片

图 4 压力传播过程（铀壳内径16 mm）

Figure 4. Pressure propagation process (inner diameter: 16 mm)

下载: 全尺寸图片幻灯片

图 5 铀壳体气化转变及碎片运动过程

Figure 5. Uranium shell gasification transformation and fragment movement process

下载: 全尺寸图片幻灯片

图 6 压力传播过程（铀壳内径26 mm）

Figure 6. Pressure propagation process (inner diameter: 26 mm)

下载: 全尺寸图片幻灯片

图 7 爆炸试验容器结构示意图

Figure 7. Structure of the explosive container

下载: 全尺寸图片幻灯片

图 8 爆炸装置结构

Figure 8. View of the explosive device

下载: 全尺寸图片幻灯片

图 9 药壳质量比与转化率的关系

Figure 9. The relationship between explosive uranium mass ratio and conversion ratio

下载: 全尺寸图片幻灯片

表 1 Project Roller Coaster实验状态

Table 1. The status of Project Roller Coaster

试验代号	炸药质量/kg	环境	爆炸烟云高度/m
DT	53.5	钢板上	220
CS1	481.7	混凝土上	710
CS2	1016.9	掩体内	440
CS3	1016.9	掩体内	520

下载: 导出CSV

表 2 铀金属的材料参数

Table 2. Material parameters of uranium metal

弹性模量/GPa	泊松比	密度/（kg·m⁻³）	A/MPa	B/MPa	n	C	D_C	ε_d	D₁	D₂	D₃	D₄	D₅
132.6	0.3	19 050	800	684	0.083	0.012	0.3	0	0.0705	1.732	−0.54	−0.0123	0

下载: 导出CSV

表 3 转化率的实验与模拟结果对比(%)

Table 3. Comparison of experimental and simulational results of conversion ratio (%)

实验编号	实验结果	计算结果	绝对误差	实验编号	试验结果	计算结果	绝对误差
1	2.75	2.80	−0.05	6	58.38	61.30	−2.92
2	16.68	16.92	−0.24	7	68.48	67.26	1.22
3	23.03	24.12	−1.09	8	83.75	84.33	−0.58
4	43.87	45.03	−1.16	9	88.91	89.64	−0.73
5	52.29	51.91	0.38	10	92.80	92.85	−0.05

下载: 导出CSV

表 4 不同实验工况下铀气溶胶的质量和转化率

Table 4. Mass and conversion ratio of uranium aerosol among different experiments

试验编号	铀气溶胶质量/g	铀壳质量/g	转化率/%
1	6.2	225.7	2.75
2	34.8	208.6	16.68
3	43.3	187.9	23.03
4	86.3	160.2	43.87
5	67.2	128.5	52.29
6	53.6	91.8	58.38
7	55.2	80.6	68.48
8	48.2	75.6	83.75
9	53.8	60.6	88.91
10	45.7	49.3	92.80

下载: 导出CSV

参考文献(31)

[1]	BARKER R B. DoD 5100.52-M nuclear weapon accident response procedures (NARP) [S]. Washington: Assistant to the Secretary of Defense, 1990.
[2]	CONDIT R H. Plutonium dispersal in fires: summary of what is known [R]. Livermore: Lawrence Livermore National Laboratory, 1993.
[3]	周建辉, 孙新利, 聂虎, 等. 基于修正光滑粒子流体动力学的爆轰波对金属球壳的压强数值模拟 [J]. 弹箭与制导学报, 2009, 29(4): 116–118. DOI: 10.3969/j.issn.1673-9728.2009.04.033. ZHOU J H, SUN X L, NIE H, et al. Numerical simulation of metallic sphere-shell’s pressure caused by detonation wave based on modified SPH [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2009, 29(4): 116–118. DOI: 10.3969/j.issn.1673-9728.2009.04.033.
[4]	王凯民, 于宪峰, 蔡瑞娇, 等. 传爆序列飞片起爆理论研究及应用 [J]. 探测与控制学报, 2007, 29(4): 57–60, 64. DOI: 10.3969/j.issn.1008-1194.2007.04.015. WANG K M, YU X F, CAI R J, et al. Shock-initiation theory and computations of flyer plate impact [J]. Journal of Detection & Control, 2007, 29(4): 57–60, 64. DOI: 10.3969/j.issn.1008-1194.2007.04.015.
[5]	STEELE C M, WALD T L, CHANIN D I. Plutonium explosive dispersal modeling using the MACCS2 computer code [R]. Lawrence: Lawrence Livermore National Laboratory, 1998.
[6]	TITUS R W. Operation roller coaster: project officers report-project, 2.4 POR-2504 [R]. Las Vegas: United States Weather Bureau Research Station, 1965.
[7]	HASCHKE J M. Evaluation of source-term data for plutonium aerosolization [R]. Los Alamos: Los Alamos National Laboratory, 1992.
[8]	HASCHKE J M, MARTZ J C. Oxidation kinetics of plutonium in air from 500 to 3 500 °C: application to source terms for dispersal [J]. Journal of Alloys and Compounds, 1998, 266(1−2): 81–89. DOI: 10.1016/S0925-8388(97)00499-4.
[9]	MARTZ J C, HASCHKE J M. A mechanism for combustive heating and explosive dispersal of plutonium [J]. Journal of Alloys and Compounds, 1998, 266(1−2): 90–103. DOI: 10.1016/S0925-8388(97)00498-2.
[10]	郝樊华, 刘晓亚, 肖成建, 等. 爆轰条件下的气溶胶粒径分布及Ag质量分数与粒径的关系 [J]. 核技术, 2009, 32(5): 343–346. DOI: 10.3321/j.issn: 0253-3219.2009.05.005. HAO F H, LIU X Y, XIAO C J, et al. Size distributing of explosion-generated particles in a sealed container [J]. Nuclear Techniques, 2009, 32(5): 343–346. DOI: 10.3321/j.issn: 0253-3219.2009.05.005.
[11]	刘文杰, 胡八一, 李庆忠. 核事故条件下钚气溶胶源项研究综述 [J]. 安全与环境学报, 2011, 11(5): 259–263. DOI: 10.3969/j.issn.1009-6094.2011.05.058. LIU W J, HU B Y, LI Q Z. Review of plutonium aerosol source-term in nuclear accident [J]. Journal of Safety and Environment, 2011, 11(5): 259–263. DOI: 10.3969/j.issn.1009-6094.2011.05.058.
[12]	唐秀欢, 杨宁, 包利红, 等. 核武器运输炸药爆炸事故气溶胶扩散数值模拟 [J]. 安全与环境学报, 2014, 14(1): 142–148. DOI: 10.13637/j.issn.1009-6094.2014.01.034. TANG X H, YANG N, BAO L H, et al. Numerical simulation of aerosol dispersion with the explosive’s detonation in the process of nuclear weapon transportation [J]. Journal of Safety and Environment, 2014, 14(1): 142–148. DOI: 10.13637/j.issn.1009-6094.2014.01.034.
[13]	SEO S, MIN O, LEE J. Application of an improved contact algorithm for penetration analysis in SPH [J]. International Journal of Impact Engineering, 2008, 35(6): 578–588. DOI: 10.1016/j.ijimpeng.2007.04.009.
[14]	董晃晃. SPH的粒子生成方法及其在弹体侵彻金属靶中的应用[D]. 南昌: 华东交通大学, 2017: 24−38.
[15]	FAN H F, BERGEL G L, LI S F. A hybrid peridynamics-SPH simulation of soil fragmentation by blast loads of buried explosive [J]. International Journal of Impact Engineering, 2016, 87: 14–27. DOI: 10.1016/j.ijimpeng.2015.08.006.
[16]	王志远, 王凤英, 刘天生, 等. 基于FEM/SPH算法弹丸侵彻多孔陶瓷板的数值模拟 [J]. 高压物理学报, 2017, 31(1): 35–41. DOI: 10.11858/gywlxb.2017.01.006. WANG Z Y, WANG F Y, LIU T S, et al. Numerical simulation of projectile penetration into porous ceramic plates based on FEM/SPH algorithm [J]. Chinese Journal of High Pressure Physics, 2017, 31(1): 35–41. DOI: 10.11858/gywlxb.2017.01.006.
[17]	赵德, 谈乐斌, 林志宸, 等. 基于SPH的弹丸侵彻土壤回收箱的数值模拟 [J]. 兵器装备工程学报, 2020, 41(4): 10–14. DOI: 10.11809/bqzbgcxb2020.04.003. ZHAO D, TAN L B, LIN Z C, et al. Numerical simulation of projectile penetrating soil receiving tank based on SPH method [J]. Journal of Ordnance Equipment Engineering, 2020, 41(4): 10–14. DOI: 10.11809/bqzbgcxb2020.04.003.
[18]	LIU M B, ZHANG Z L, FENG D L. A density-adaptive SPH method with kernel gradient correction for modeling explosive welding [J]. Computational Mechanics, 2017, 60(3): 513–529. DOI: 10.1007/s00466-017-1420-5.
[19]	强洪夫, 张国星, 王广, 等. SPH方法在宽速域岩石侵彻问题中的应用 [J]. 高压物理学报, 2019, 33(5): 055105. DOI: 10.11858/gywlxb.20180621. QIANG H F, ZHANG G X, WANG G, et al. Application of SPH method for problem of rock penetration within the wide-ranged velocity [J]. Chinese Journal of High Pressure Physics, 2019, 33(5): 055105. DOI: 10.11858/gywlxb.20180621.
[20]	强洪夫, 孙新亚, 王广, 等. 基于SPH的分层钢板抗半球头弹侵彻的数值模拟 [J]. 高压物理学报, 2018, 32(5): 055102. DOI: 10.11858/gywlxb.20170664. QIANG H F, SUN X Y, WANG G, et al. Numerical simulation of anti-penetration of laminated steel plate by hemispherical-nosed projectile using SPH [J]. Chinese Journal of High Pressure Physics, 2018, 32(5): 055102. DOI: 10.11858/gywlxb.20170664.
[21]	FAN H F, LI S F. A Peridynamics-SPH modeling and simulation of blast fragmentation of soil under buried explosive loads [J]. Computer Methods in Applied Mechanics and Engineering, 2017, 318: 349–381. DOI: 10.1016/j.cma.2017.01.026.
[22]	高本兵, 尹建平, 陈杰, 等. 基于SPH方法的不同材质射流毁伤性能研究 [J]. 北京理工大学学报, 2018, 38(4): 353–358. DOI: 10.15918/j.tbit 1001-0645.2018.04.004. GAO B B, YIN J P, CHEN J, et al. Study on damage characteristics of different jets based on SPH method [J]. Transactions of Beijing Institute of Technology, 2018, 38(4): 353–358. DOI: 10.15918/j.tbit 1001-0645.2018.04.004.
[23]	顾鑫, 章青. 爆炸荷载作用下大坝破坏分析的数值模拟研究进展 [J]. 河海大学学报 (自然科学版), 2017, 45(1): 45–55. DOI: 10.3876/j.issn.1000-1980.2017.01.007. GU X, ZHANG Q. Progress in numerical simulation of dam failure under blast loading [J]. Journal of Hehai University (Natural Sciences), 2017, 45(1): 45–55. DOI: 10.3876/j.issn.1000-1980.2017.01.007.
[24]	强洪夫, 范树佳, 陈福振, 等. 基于SPH方法的聚能射流侵彻混凝土靶板数值模拟 [J]. 爆炸与冲击, 2016, 36(4): 516–524. DOI: 10.11883/1001-1455(2016)04-0516-09. QIANG H F, FAN S J, CHEN F Z, et al. Numerical simulation on penetration of concrete target by shaped charge jet with SPH method [J]. Explosion and Shock Waves, 2016, 36(4): 516–524. DOI: 10.11883/1001-1455(2016)04-0516-09.
[25]	Los Alamos National Laboratory. Selected hugoniots [R]. Los Alamos: Los Alamos National Laboratory, 1969.
[26]	AMINI Y, EMDAD H, FARID M. A new model to solve fluid-hypo-elastic solid interaction using the smoothed particle hydrodynamics (SPH) method [J]. European Journal of Mechanics-B/Fluids, 2011, 30(2): 184–194. DOI: 10.1016/j.euromechflu.2010.09.010.
[27]	李运禄, 尹建平, 王志军, 等. 基于SPH算法对混凝土裂纹扩展研究 [J]. 兵器材料科学与工程, 2015, 38(5): 19–22. DOI: 10.14024/j.cnki.1004-244x.20150831.003. LI Y L, YIN J P, WANG Z J, et al. Crack propagation of concrete based on SPH algorithm [J]. Ordnance Material Science and Engineering, 2015, 38(5): 19–22. DOI: 10.14024/j.cnki.1004-244x.20150831.003.
[28]	胡德安, 孙占华, 朱婷. 三维自适应FE-SPH耦合算法在多层间隔金属靶侵彻问题中的应用 [J]. 爆炸与冲击, 2015, 35(3): 416–422. DOI: 10.11883/1001-1455-(2015)03-0416-07. HU D A, SUN Z H, ZHU T. Application of 3D FE-SPH adaptive coupling algorithm to penetration analysis of spaced multi-layered metallic targets [J]. Explosion and Shock Waves, 2015, 35(3): 416–422. DOI: 10.11883/1001-1455-(2015)03-0416-07.
[29]	杨刚, 傅奕轲, 郑建民, 等. 基于SPH方法对不同药型罩线性聚能射流形成及后效侵彻过程的模拟 [J]. 振动与冲击, 2016, 35(4): 56–61. DOI: 10.13465/j.cnki.jvs.2016.04.009. YANG G, FU Y K, ZHENG J M, et al. Simulation of formation and subsequent penetration process of linear shaped charge jets with different liners based on SPH method [J]. Journal of Vibration and Shock, 2016, 35(4): 56–61. DOI: 10.13465/j.cnki.jvs.2016.04.009.
[30]	高艳. 基于SPH方法的弹丸侵彻问题的数值模拟[D]. 西安: 长安大学, 2014: 34−41.
[31]	尹冠生, 高艳, 赵庭. 基于SPH算法的刚性弹丸侵彻的数值模拟 [J]. 长安大学学报 (自然科学版), 2014, 34(3): 74–79. DOI: 10.19721/j.cnki.1671-8879.2014.03.012. YIN G S, GAO Y, ZHAO T. Numerical simulation for penetration of rigid projectile based on SPH method [J]. Journal of Chang’an University (Natural Science Edition), 2014, 34(3): 74–79. DOI: 10.19721/j.cnki.1671-8879.2014.03.012.

施引文献

期刊类型引用(1)
1. 朱勇，周辉，赵海洋，李彦恒，杨凡杰. 复杂地下硐室群核事故钚气溶胶扩散行为研究. 安全与环境工程. 2023(06): 96-105 . 百度学术
其他类型引用(0)

资源附件(0)

访问统计

图(9) / 表(4)

计量

文章访问数: 476
HTML全文浏览量: 295
PDF下载量: 122
被引次数: 1

1. 铀气溶胶形成过程的数值仿真
1.1 数值仿真模型
1.2 数值模拟分析
2. 实验分析
2.1 实验装置设计
2.2 实验数据分析
3. 实验与数值模拟对比分析
4. 结　论

爆炸载荷作用下铀气溶胶形成机理研究

doi: 10.11883/bzycj-2021-0075

作者简介: 刘志勇（1982－ ），男，博士研究生，副研究员，ageleil@163.com

通讯作者: 王金涛（1985－ ），男，博士，讲师，451128257@qq.com

计量

出版历程