Study on the scaling law of geometrically-distorted thin-walled cylindrical shells subjected to axial impact
-
摘要: 对于受轴向冲击载荷作用的薄壁圆管动态响应的相似律问题,由于圆管的薄壁特性导致厚度无法与高度和半径按相同的比例进行结构缩放,从而产生模型的几何畸变,此时传统的相似律已无法描述原型与畸变模型之间的动态响应规律。基于薄壁圆管轴向冲击问题的控制方程,通过能量守恒和量纲分析,推导了考虑几何畸变条件下轴向冲击载荷作用的理想弹塑性薄壁圆管动态响应的相似律。通过在给定应变与应变率区间上建立比例模型预测的流动屈服应力与原型流动屈服应力的最佳逼近关系,将几何畸变相似律进一步推广至包含应变率和应变硬化的材料。通过数值方法验证了提出的几何畸变模型相似律的适用性。分析结果表明,提出的考虑厚度畸变的受轴向冲击薄壁圆管的相似律可用于预测原型结构的冲击动态响应,并显著降低比例模型与原型结构平均载荷和能量的偏差。Abstract: In scaling the dynamic responses of thin-walled cylindrical shells subjected to axial impact loading, the thickness cannot be adjusted according to the same scale as the radius and height due to the thin wall characteristics. Hence, geometrically-distorted models would be used, and the traditional scaling law cannot describe the relationship between the dynamic responses of the prototype and the geometrically-distorted model. In this paper, the scaling law for this case was derived for elastic-ideal plastic thin-walled cylindrical shells under axial impact loading. For strain hardening and strain-rate hardening material, based on the average load, deformation energy, and displacement of the shell in the axisymmetric deformation mode, the dimensionless numbers of three key design parameters, namely the stress, mass, and displacement, were obtained through the law of energy conservation. Then, the optimal approximation of the flow stress predicted by the distorted scaled model to the flow stress of the prototype was established on a given strain and strain rate interval. In this way, the derived scaling law can be applied to the case considering the coupling effects of geometric distortion, strain-rate sensitivity, and strain hardening. Finally, several finite element models of thin-walled cylindrical shell models subject to axial mass impact were established. These models use the elastic-ideal plastic material model and the general material model with strain-rate hardening and strain hardening effects. The modified impact velocity and impact mass were obtained by the present method using the geometrically-distorted model, which verified the effectiveness and correctness of the proposed scaling law. The results show that the geometrically distorted model corrected by the method proposed in this article can quite accurately predict the dynamic responses of the prototype, and significantly reduce the errors in the dynamic responses of the thin-walled cylindrical shell subjected to axial impact loading, especially the average load and deformation energy.
-
薄壁圆管凭借其自身结构简单,变形、破坏模式稳定,轴压峰值力与平均力的可设计性以及能量吸收可控等优点,被广泛地应用于抗冲击领域,如飞机坠撞防护、缓冲器以及其他的工程领域等[1-2]。薄壁圆管在轴向冲击压缩载荷作用下的屈曲问题长期以来备受关注。Alexander等[3]针对理想刚塑性材料制成的薄壁圆管,应用具有塑性铰的简化轴对称变形模式,推导出了轴对称条件下的轴压载荷下薄壁圆管的平均力。该变形模型获得了学者的广泛认可并得到了大量的推广应用。Abramowicz等[4]基于Alexander等[3]的研究结果,对薄壁圆管轴对称变形时的折皱长度公式进行了修正,试验结果表明,修正后的公式可以较好地预测轴对称变形以及金刚石变形模式下的薄壁圆管的折皱长度。Karagiozova等[5-6]对受轴向冲击的弹塑性材料薄壁圆管的峰值载荷进行了研究,分别获得了Mises屈服条件和Tresca屈服条件下的峰值载荷。Lu等[7]在Alexander等[3]的基础上,提出了一种新的运动位移场,求解了更加合理的薄壁圆管折皱长度公式,并改进了轴对称变形下的载荷位移曲线。这些重要的研究为理解轴向冲击圆管的变形行为奠定了理论基础。
结构的冲击承载安全性是结构设计的重要组成部分,但是大结构的全尺寸冲击试验极其耗时耗力,需要付出大量的人力物力和财力,因此一般使用比例模型试验来替代结构全尺寸原型[2, 8-9]。Jones[1]通过量纲分析,建立了结构冲击问题的经典相似理论,由于该理论基于简单的几何相似关系,因而广受重视。Oshiro等[10-11] 采用新的量纲分析体系克服经典相似律解决材料相似时的理论困难,提出一种通过修正冲击速度或密度的方式,来适应应变率敏感性以及比例模型与原型使用不同材料时的畸变问题的相似方案。王帅等[12-13]通过建立应变率区间上比例模型预测的流动屈服应力与原型流动屈服应力的最佳逼近关系,获得了速度比例因子的直接相似关系,从而扩展了相似律的应用技术。李肖成等[14]通过调整薄板结构的厚度,修正了比例模型与原型采用不同材料时引起的畸变问题。秦健等[15]基于VSG量纲体系,研究得到了加筋板结构在水下爆炸冲击波作用下模型和原型的动态响应相似关系。
然而,在实际工程应用中还存在加工制造等难题,通常,薄壁圆管的厚度不一定能够和半径以及长度按照相同的比例因子进行缩放,这就会产生畸变模型,从而导致原有的相似律失效。Alves等[16]提出单独考虑几何畸变以及应变率效应,并通过经验方法不断迭代几何畸变对缩比相似影响所占的比重来修正速度比例因子。Mazzariol等[17]通过塑性弯矩的无量纲数改进了经验法,提出了一种解决薄板和梁结构的几何畸变问题的新方法。Wang等[18-19]基于定向量纲的分析方法,从理论上克服了几何畸变的相似律难题,针对薄板冲击模型系统表达了几何畸变的相似体系。尽管这些研究在解决几何畸变相似律的问题上取得了重要的理论进步,但是针对薄壁圆管的几何畸变修正方法的研究分析并未开展,而且在实际的受轴向冲击载荷作用的薄壁圆管缩比相似分析中厚度畸变几乎不可避免。
本文中针对受轴向冲击载荷作用的薄壁圆管,基于方程分析与量纲分析,以载荷、位移和能量为关键设计参数,推导薄壁圆管几何畸变的动态响应相似律,此方法可推广至考虑材料应变率以及应变硬化效应和几何畸变问题耦合时的比例模型与原型物理量对应关系的描述。
1. 薄壁圆管相似律分析
在结构冲击相似分析中,一般使用基于一组无量纲数的比例因子来预测比例模型的力学行为,如VSG量纲体系[10]或DLV量纲体系[18],其比例因子如表1所示。其中,比例模型和原型相关的物理量分别用下标m和p表示,βK=Km/Kp表示比例模型和原型相关物理量K的比值,例如βv=vm/vp表示比例模型和原型速度的比值,比例模型和原型的几何比例因子β=Lm/Lp。
基于表1中的相似关系,目前针对受轴向冲击载荷作用的薄壁圆管的相似律研究对象均为纯几何缩放的比例模型,也就是说薄壁圆管的厚度h、长度L和半径R服从同一个几何比例因子,亦即βh = βR = βL = β。
但对于薄壁圆管而言,由于其自身几何尺寸的特性,厚度方向的尺寸与半径和长度方向的尺寸完全不在一个量级,厚度方向若要按照长度和半径的几何比例因子进行缩放,会导致比例模型加工的成本远远超过加工一个原型试验件的费用。并且,受到加工限制,其厚度方向的尺寸h并不一定能够与长度L和半径R按照同一个几何比例因子进行缩放,这时就会产生几何畸变模型,这时,βh = βR = βL = β这一基础条件会遭到破坏,将会导致表1中的相似律不能全部满足,这就需要重新推导薄壁圆管的相似律。
1.1 受轴向冲击的薄壁圆管塑性响应相似律分析
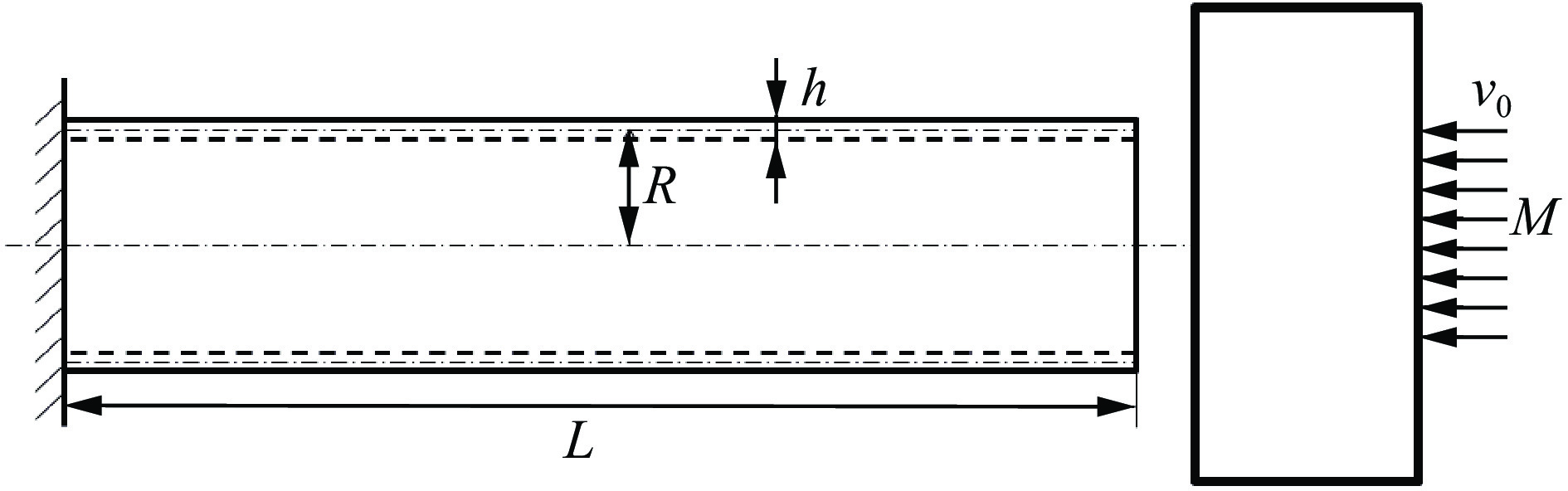
考虑到薄壁圆管的结构响应特性,以薄壁圆管压溃过程中的平均载荷、位移和能量这3个响应变量作为相似设计的关键参数[20]。影响薄壁圆管压溃特性的参量有:厚度h、半径R、长度L、冲击速度v、冲击质量M、材料的密度ρ、流动屈服应力σd。
根据薄壁圆管理论,在推导过程中做如下假设:
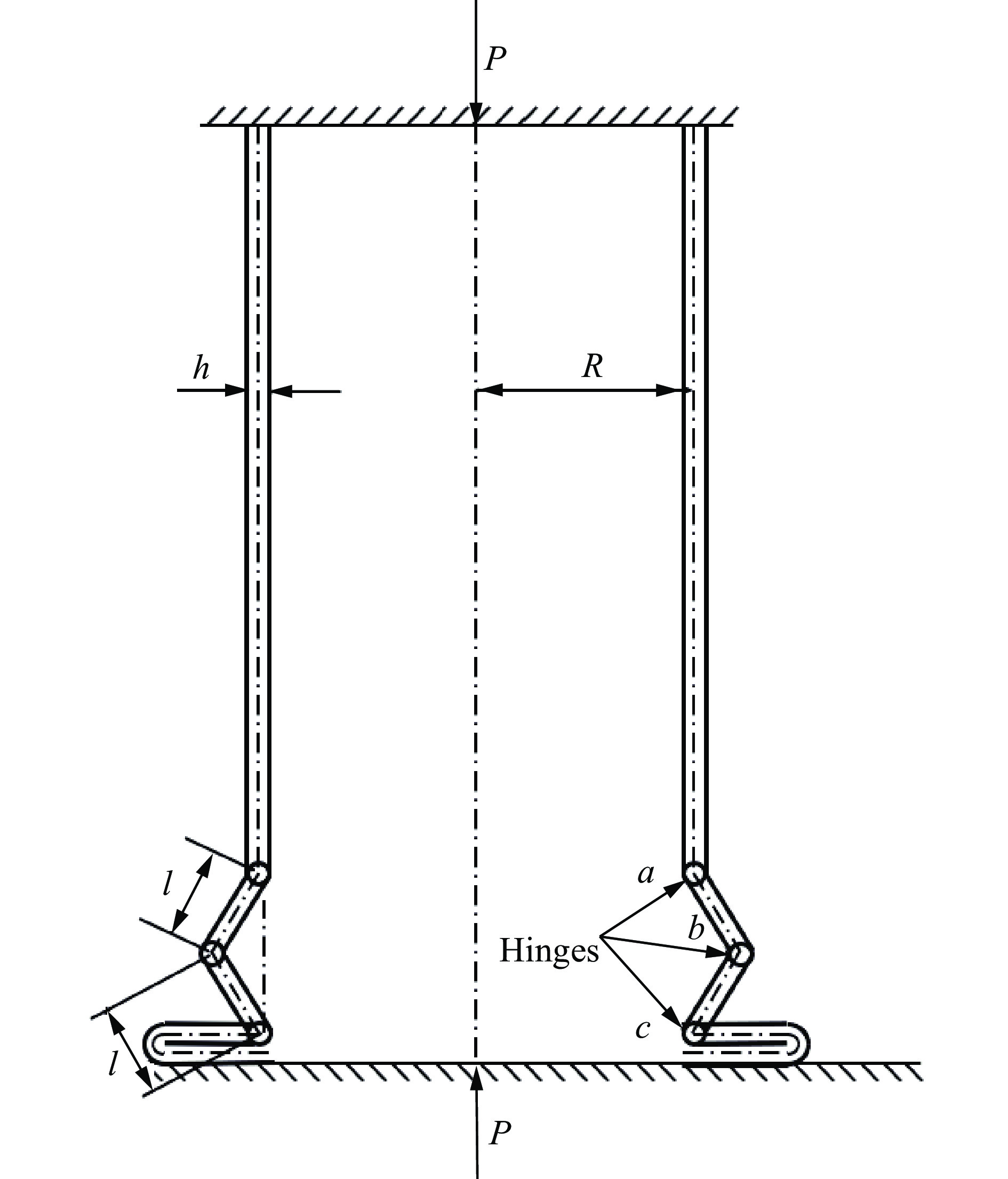
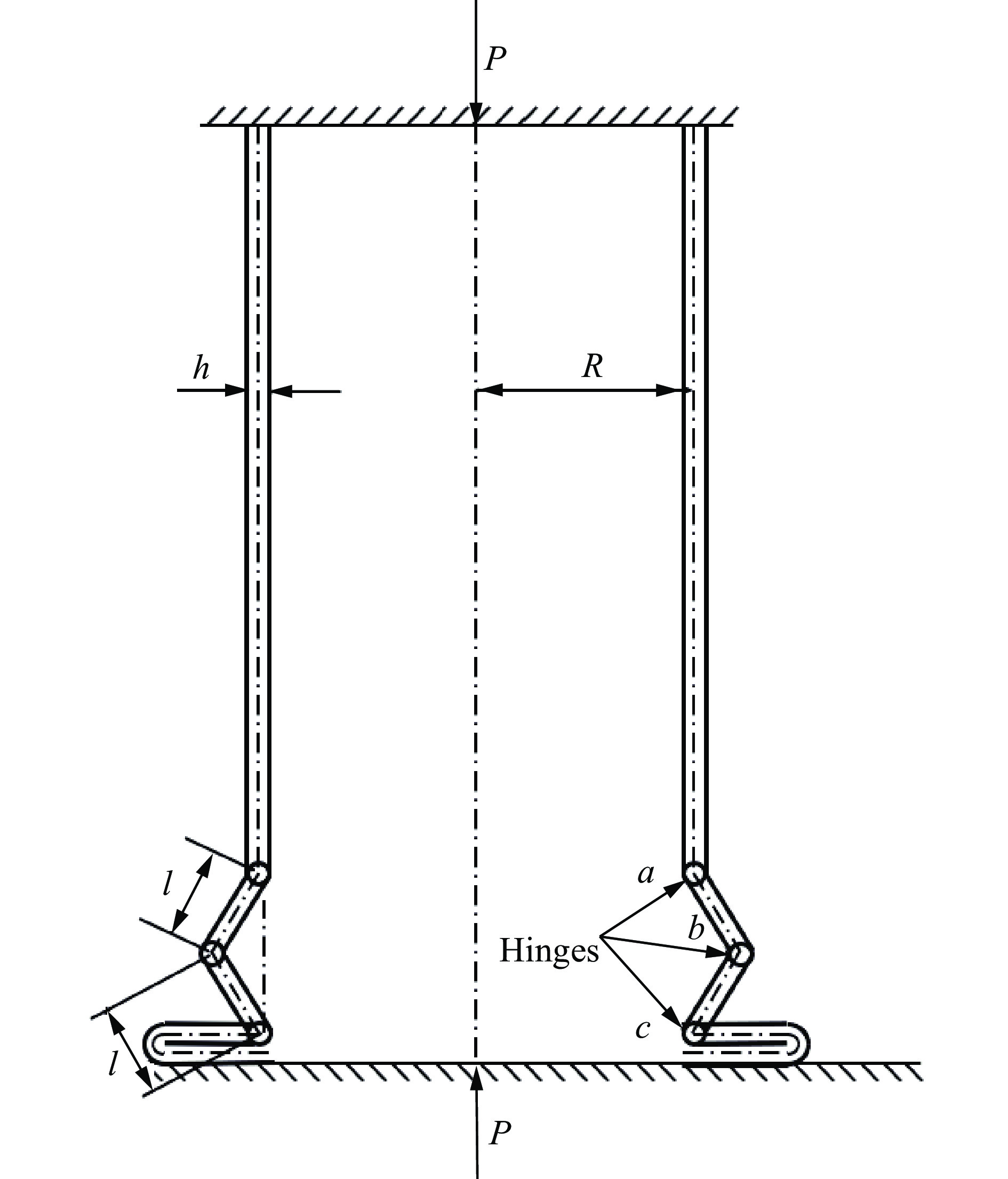
(1)受轴向冲击载荷作用的薄壁圆管的变形模式服从Alexander所给出的轴对称变形模式,如图1所示;
(2)薄壁圆管壁厚与半径及长度相比为小量,服从薄壳理论;
(3)与弹性变形相比,塑性变形占据主导地位,弹性变形可以忽略不计;
(4)薄壁圆管使用理想弹塑性材料,材料的流动屈服应力为一定值;
(5)薄壁圆管受到重撞击物的低速冲击;
(6)定义基本几何比例因子:β = βR = βL。
当薄壁圆管发生图1所示的准静态压溃模式时,Alexander[3]给出了该模式下的薄壁圆管每产生一个完整折皱时的平均压溃力P和折皱长度l的表达式:
{P=2(πh)3/2R1/2σd/31/4l=(πRh/√3)1/2 (1) 式中:σd为材料的流动屈服应力,对于理想弹塑性材料,其为一常值。
当薄壁圆管受重撞击物的低速冲击时,可忽略惯性力的影响,薄壁圆管的动态压溃过程可近似视为准静态,此时式(1)能够适用于动态载荷下的薄壁圆管的轴对称压溃问题[1, 4, 21]。
薄壁圆管的质量m为:
m=2ρπRhL (2) 当薄壁圆管受到轴向冲击载荷作用时,忽略摩擦以及温度软化导致的能量损失,那么,在整个薄壁圆管压溃过程中的能量转化就是结构的动能与塑性耗散能的相互转化。根据能量守恒定律,塑性耗散能可等效视为外力P在整个薄壁圆管的压缩行程δ中所做的功,也就是说外力P所做的功WP应等于薄壁圆管所受到的冲击能量Ek [22]:
WP=Ek⇒Pδ=12Mv02 (3) 式中:M为冲击块的质量,v0为撞击物的初始速度。
将式(2)代入式(3)可得:
Pδ=πρRhLv20Mm (4) 将式(1)代入式(4),则式(4)进一步可变形为:
δL=31/42√πρv20σd(Rh)0.5Mm (5) 通过式(5)可以得到,发生轴对称变形的薄壁圆管受轴向冲击载荷作用时的无量纲位移、质量和应力分别为:
Πδ=δL,Πm=Mm,Πσd=ρv2σd(Rh)0.5 (6) 为了使比例模型可以准确预测原型的动态响应,原型与比例模型的无量纲数应相等,也就是说:
(Πδ)m(Πδ)p=βδβ=1→βδ=β (7) (Πm)m(Πm)p=βMβm=1→βM=βm=βρβ2βh (8) (Πσd)m(Πσd)p=βρβ2vβσd(ββh)1/2=1→βv=√βρβσd(ββh)1/2 (9) 对于遵循图1所示的压溃模式的薄壁圆管来说,在折皱的轴对称部分ab段,距离a点径向距离为x的微元伸长量为:
ds=2π(R+x)−2πR=2πx (10) 对应该微元处的环向应变为:
εθ=2πx2πR=xR (11) 不难发现,图1中ab段各点处的环向应变与x成正比,那么对于一个完整折皱,x∈[0, l],其平均环向应变为:
εθ=120+lR=l2R (12) 平均环向应变率为:
˙εθ=εθt=l2Rt (13) 对发生轴对称变形的薄壁圆管,与环向应变相比,其轴向应变十分小,而薄壁圆管壁厚很薄,可近似认为其径向应变为零,因此可以用其环向的平均应变
εθ 和平均应变率˙εθ 替代等效应变ε 与等效应变率˙ε ,即:εθ≈ε,˙εθ≈˙ε (14) 将式(1)代入式(12)~(13),可得应变和应变率的比例因子为:
{βε=(εθ)m/(εθ)p=βlβ=√βhββ=√βh/ββ˙ε=(˙εθ)m/(˙εθ)p=βlββt=βlββvβ=(βhβ)1/2βvβ2=βvβ√βhβ (15) 综上所述,得到受轴向冲击载荷的薄壁圆管的缩放比例因子,见表2,当比例模型与原型满足表2中的比例因子时,比例模型可以准确地预测原型的动态响应。
表 2 受轴向冲击的理想弹塑性薄壁圆管比例因子Table 2. Scaling factors of the elastic-ideal plastic thin-walled cylindrical shell under axial impact loading变量 比例因子 变量 比例因子 长度L β=Lm/Lp 位移δ βδ=β 密度ρ βρ=ρm/ρp 应力σd βσd=βρβ2v√β/βh 速度v βv=vm/vp 应变ε βε=√βh/β 质量m βm=βρβ2βh 应变率˙ε β˙ε=(βv/β)√βL/βh 时间t βt=β/βv 载荷P βP=βρββhβ2v 加速度 a βa=β2v/β 动能Ek βEk=βρβhβ2β2v 1.2 考虑应变率效应和应变硬化效应的相似律分析
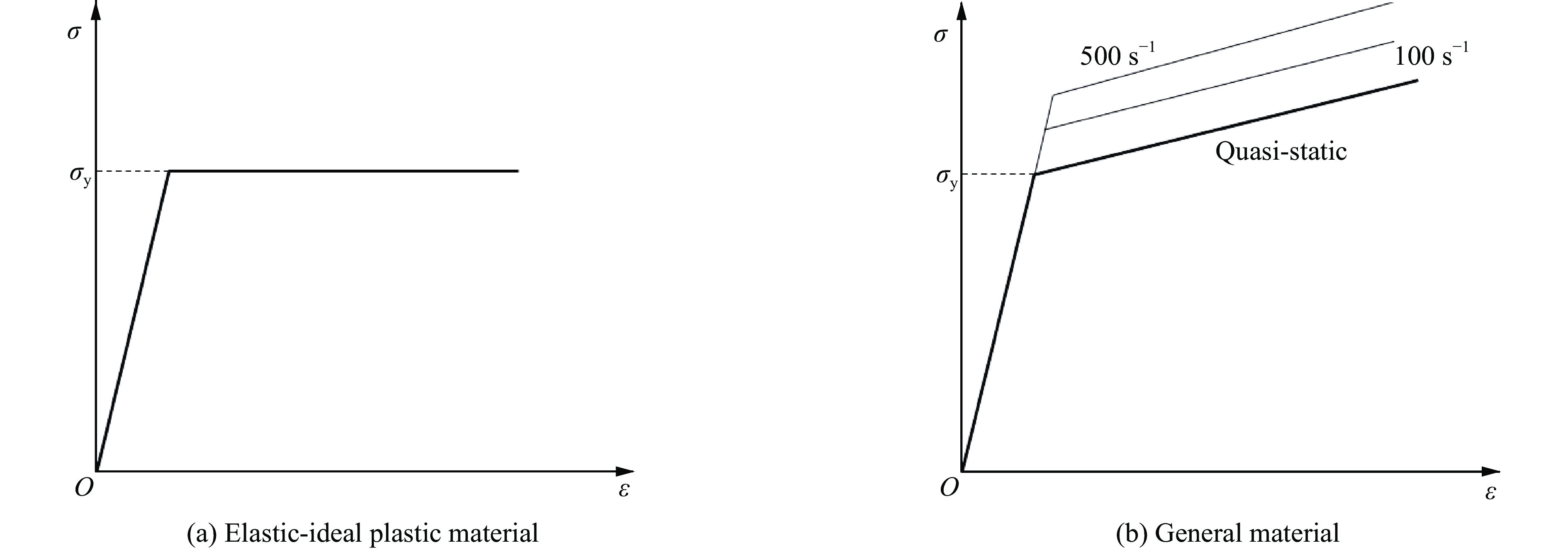
实际工程中,理想弹塑性的材料是几乎不存在的,绝大多数金属材料的塑性阶段都存在着应变率效应和应变硬化效应[23],如图2所示,材料的流动屈服应力是应变和应变率的函数而非一固定值,即:
σd=f(ε,˙ε) (16) 将式(15)代入式(16),得到流动屈服应力的比例因子为:
βσd=(σd)m(σd)p=f(εm,˙εm)f(εp,˙εp)=f(εpβε,˙εpβ˙ε)f(εp,˙εp)=f(εp√βh/β,˙εpβv√βh/β3)f(εp,˙εp) (17) 可见,式(17)所得到的
βσd 在整个薄壁圆管的压溃过程中是一个不断变化的值。但是若要使比例模型准确地预测原型的动态响应,原型与比例模型的流动屈服应力在整个冲击过程中的应变区间[εa,εb] 与应变率区间[˙εa,˙εb] 中应始终满足(σd)m=(σd)p 。而由于材料的应变率和应变硬化效应引起的缩比相似的畸变问题,使这一条件几乎无法满足。对此,可在应变率区间
[˙εa,˙εb] 和应变区间[εa,εb] 上,通过(σd)m 和(σd)p 的误差优化来减小两者的误差,从而减小应变率和应变硬化引起的误差[12]。如果原型的应变区间为
[εa,εb] ,应变率˙εp 在[˙εa,˙εb] 上变化,那么比例模型预测的流动屈服应力(σd)′m 与原型流动屈服应力(σd)p 存在一个最小误差,即:‖(σd)∗m−(σd)p‖2=minβv∈R‖(σd)′m−(σd)p‖2 成立。那么就有:‖fm(εp√βhβ,˙εpβ∗vβ√βhβ)1βρβ∗v2√βhβ−fp(εp,˙εp)‖2=minβv∈R‖fm(εp√βhβ,˙εpβvβ√βhβ)1βρβv2√βhβ−fp(εp,˙εp)‖2 (18) 式中:
β∗v 为比例模型所预测的流动屈服应力(σd)′m 与原型的流动屈服应力(σd)p 在整个应变区间和应变率区间上误差最小时的速度比例因子,(σd)∗m 为速度比例因子选取为β∗v 时的比例模型预测的原型的流动屈服应力。‖·‖ 为2范数,其中应变区间[εa,εb] 与应变率区间[˙εa,˙εb] 可根据试验确定,当难以进行试验时可根据经验粗略选取。至此,我们将理想弹塑性假设下的薄壁圆管缩比相似分析拓展到了同时伴有应变率效应和应变硬化效应的一般材料薄壁圆管缩比相似分析的应用中。
2. 数值验证
2.1 有限元模型
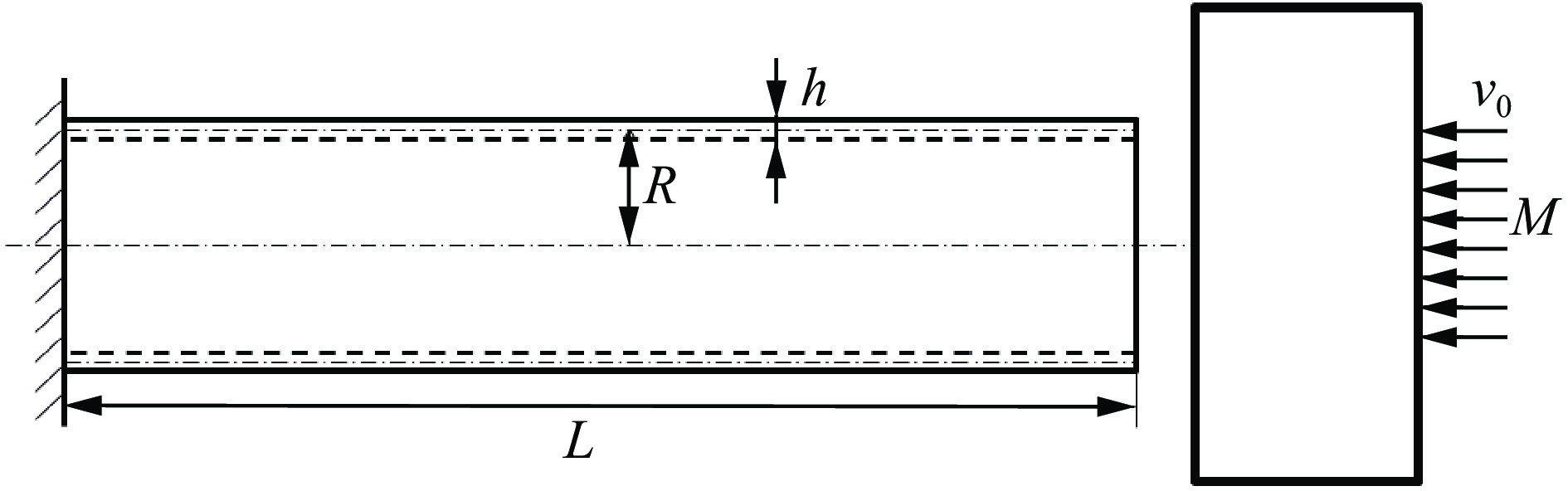
为了验证本文所推导的相似律对于结构缩比相似分析中的应变率和应变硬化效应畸变问题以及几何畸变问题的修正能力,在ABAQUS中建立了如图3所示的薄壁圆管模型进行验证分析。薄壁圆管原型的长度L=240 mm,中性面半径R=60 mm,壁厚h=1.8 mm。薄壁圆管材料选取应变率较为敏感的1006 钢,采用Johnson-Cook本构模型进行数值模拟,材料参数见表3[24]。
薄壁圆管采用CAX4R轴对称单元进行仿真,锤头和地面则使用刚体单元。薄壁圆管自由放置于刚性地面上,顶部受到质量M=128 kg、速度v0 =12 m/s的质量块冲击。薄壁圆管与地面之间的摩擦因数为0.2,圆管内壁、外壁之间设置为无摩擦。
分别建立了基本几何比例因子β=1/10,厚度方向的比例因子βh 分别为 0.12、0.15、0.18和0.20的比例模型。并按照表2所给出的相似律获得速度修正比例因子,定义几何畸变度η = βh /β。由表2可得理想弹塑性材料的薄壁圆管几何畸变比例模型的各动态变量的比例因子,见表4。同时为了验证本文对于应变率和应变硬化效应与几何畸变效应耦合后的修正方法,建立了考虑应变率效应和应变硬化效应后的薄壁圆管几何畸变比例模型,并按照式(18)所给出的方法获得应变率区间和应变硬化区间上的最佳速度比例因子,通过表2即可获得比例模型与原型各动态变量的比例因子,见表5。其中,应变率区间和应变区间在大量数值模拟经验的基础上分别选取为[0, 500]和[0, 0.6]。
表 4 理想弹塑性模型几何畸变比例因子Table 4. Scaling factors of geometrically-distorted models of elastic-ideal plastic materialβ η βh βM βv βt βP βδ βε 0.1 1.2 0.12 0.12 1.0466 0.0955 0.0131 0.1 1.0954 0.1 1.5 0.15 0.15 1.1067 0.0904 0.0184 0.1 1.2247 0.1 1.8 0.18 0.18 1.1583 0.0863 0.0241 0.1 1.3416 0.1 2.0 0.20 0.20 1.1892 0.0841 0.0283 0.1 1.4142 表 5 考虑应变率效应和应变硬化效应几何畸变模型比例因子Table 5. Scaling factors of geometrically-distorted models considering strain-rate sensitivity and strain hardeningβ η βh βM βv βt βP βδ βε 0.1 1.2 0.12 0.12 1.0756 0.0930 0.0139 0.1 1.0954 0.1 1.5 0.15 0.15 1.1442 0.0874 0.0196 0.1 1.2247 0.1 1.8 0.18 0.18 1.2038 0.0831 0.0261 0.1 1.3416 0.1 2.0 0.20 0.20 1.2396 0.0807 0.0306 0.1 1.4142 2.2 结果分析
2.2.1 理想弹塑性几何畸变模型结果分析
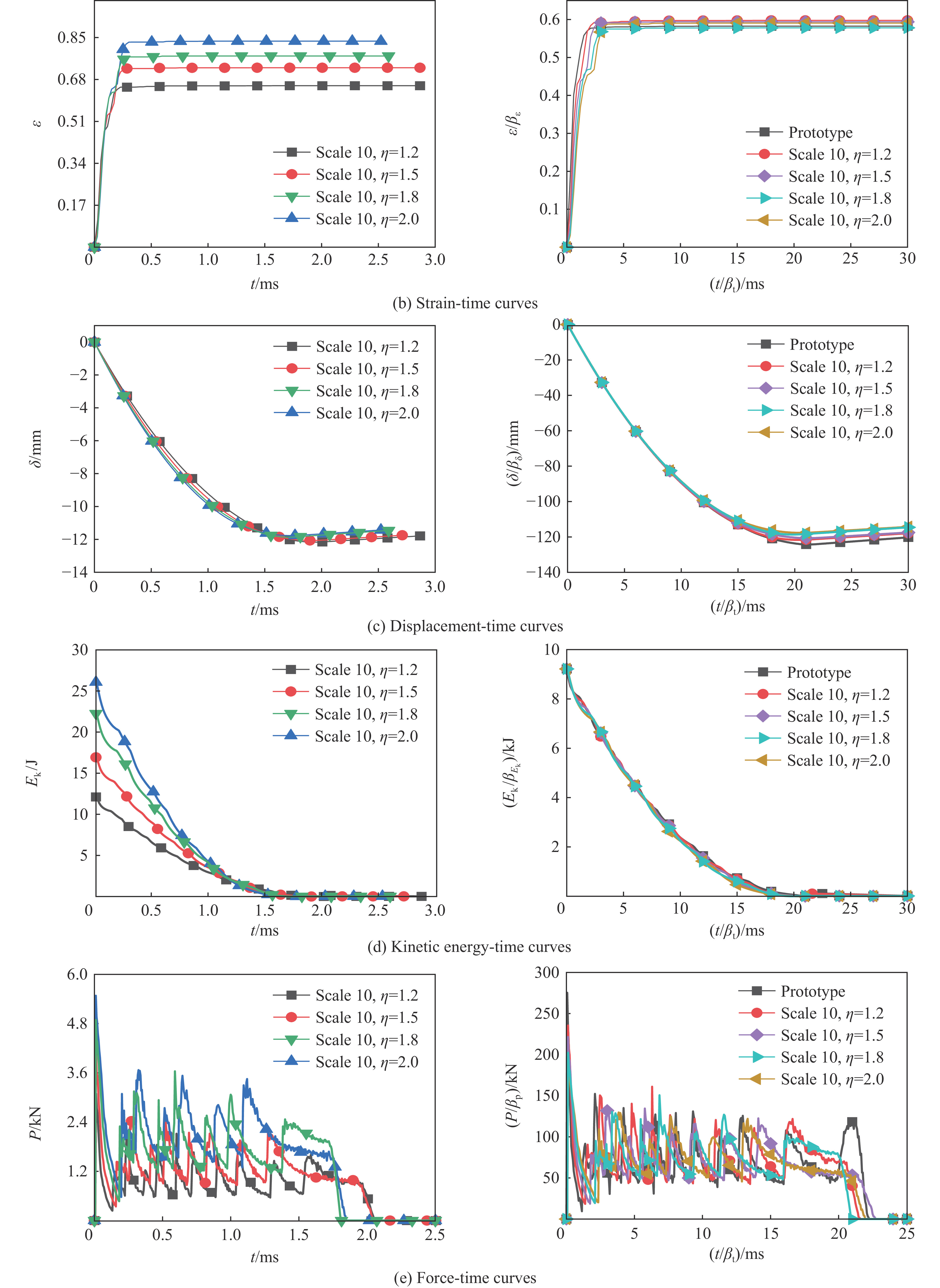
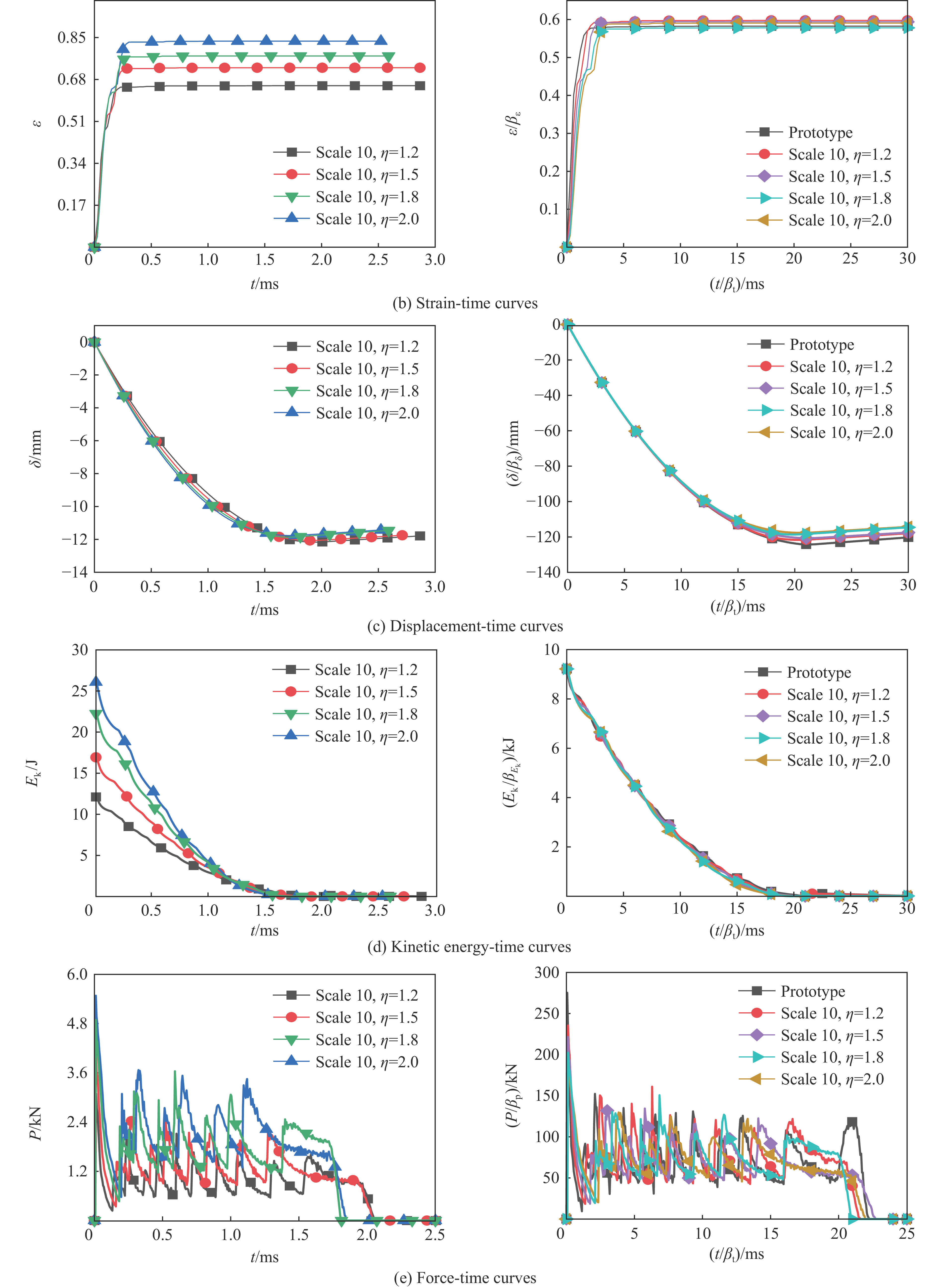
图4为使用本文提出的受轴向冲击的薄壁圆管几何畸变修正方法后,获得的修正后理想弹塑性薄壁圆管的几何畸变比例模型与原型结构动态响应时间曲线的对比。图4(a)为锤头的速度-时间响应曲线,图4(b)为薄壁圆管首个折皱处塑性铰位置的等效塑性应变-时间响应,图4(c)、(d)分别为锤头的位移和能量-时间响应曲线,图4(e)为薄壁圆管几何畸变比例模型与原型载荷时间-曲线的对比。表6为修正后的薄壁圆管几何畸变模型所预测原型的峰值位移和平均载荷与原型响应之间的相对误差。
表 6 比例模型与原型的峰值位移和平均载荷相对误差Table 6. Relative errors in the peak displacement and average force between the scale models and prototype模型 (δ/βδ)/mm 相对误差/% (P/βP)/kN 相对误差/% 原型 124.258 − 71.95 − β=1/10, η=1.2 121.669 2.084 73.43 2.057 β=1/10, η=1.5 120.651 2.903 69.48 3.433 β=1/10, η=1.8 118.642 4.520 75.85 5.420 β=1/10, η=2.0 117.678 5.295 72.25 0.417 从图4可以看出,修正前的不同几何畸变度的理想弹塑性薄壁圆管比例模型的响应曲线基本无法重合,各条曲线上的相应点所对应的时间与值均不相等,而修正后的理想弹塑性薄壁圆管几何畸变比例模型与原型的速度、能量、位移、等效塑性应变和载荷-时间曲线重合性明显改善,修正后的比例模型的速度和应变-时间曲线与原型的响应曲线完全重合。对于本文推导过程中的3个关键设计参数:能量Ek、位移δ和平均载荷P,修正后的比例模型的位移和能量曲线与原型的响应曲线重合性也非常好,但是修正后的比例模型与原型的载荷-时间曲线重合度不是很高。这是因为本文推导的相似律以能量Ek、位移δ和平均载荷P作为关键相似设计参数,薄壁圆管的折皱长度l与位移δ并不服从同一个比例因子,因此几何畸变比例模型与原型的折皱数量并不一致,导致修正后的比例模型和原型的载荷-时间曲线无法完全重合,但是相较修正前,其误差已经大大减小,且其整体的时间趋势一致性比较良好。因此我们又分析了比例模型与原型的峰值位移和平均载荷的误差,从表6可以看到,即使几何畸变度达到2,比例模型所预测的结果与原型的峰值位移和平均载荷的相对误差均不超过6%。
综上所述,使用本文所推导的相似律,修正后的理想弹塑性薄壁圆管几何畸变比例模型的动态响应可以准确地预测原型的动态响应。
2.2.2 考虑应变率效应和应变硬化效应几何畸变模型结果分析
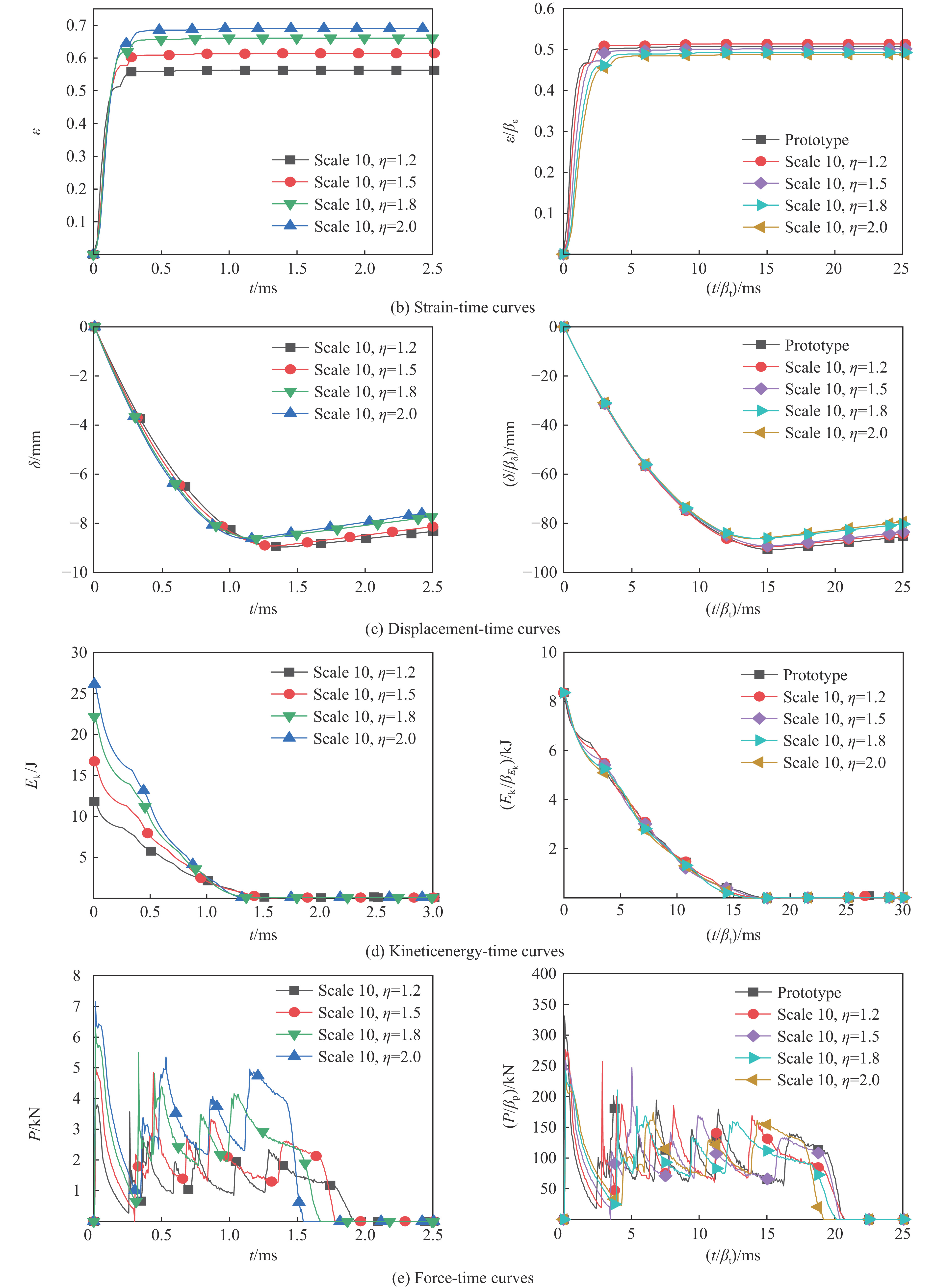
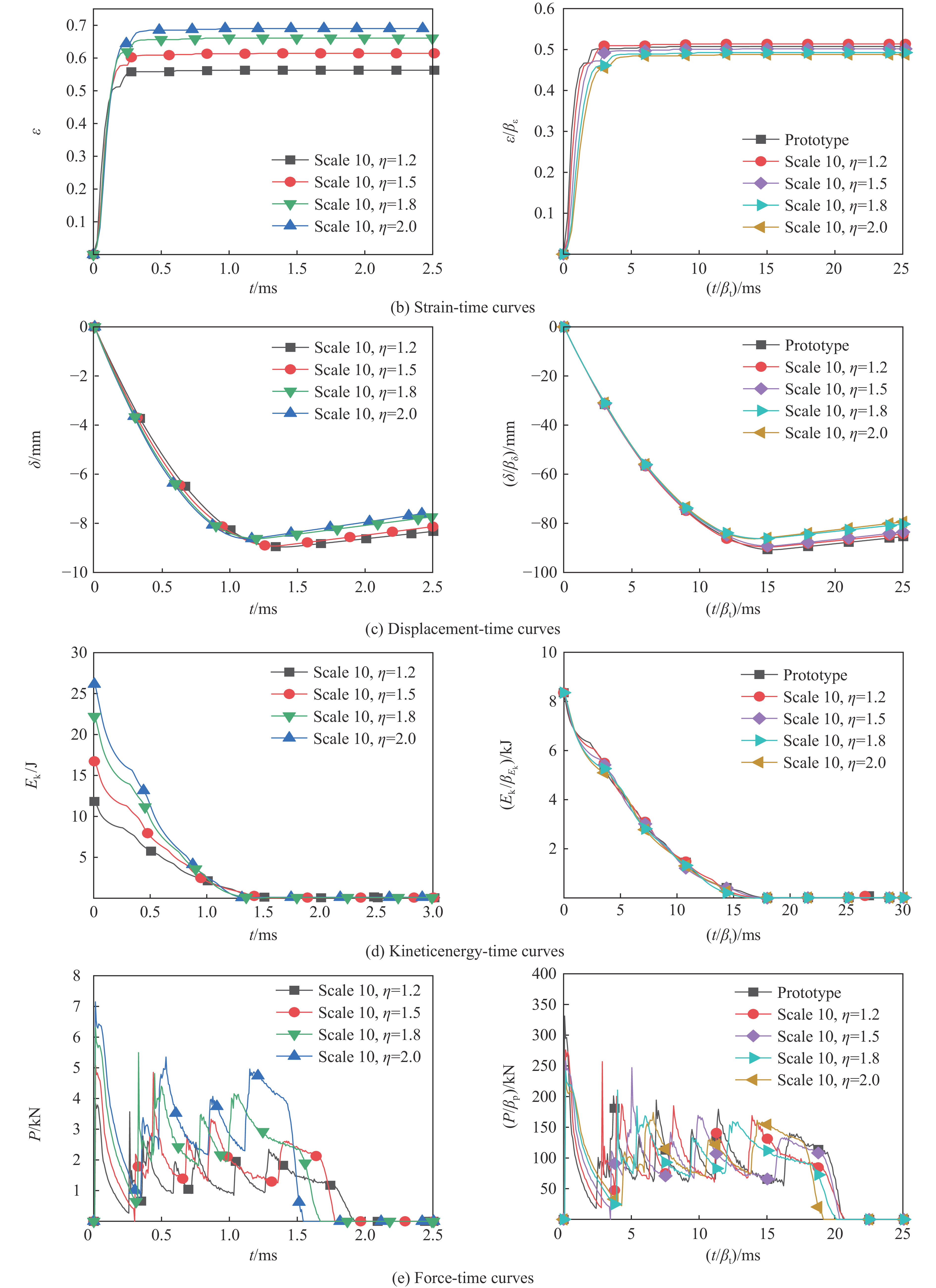
使用本文提出的受轴向冲击的一般材料薄壁圆管几何畸变修正方法,同时考虑应变率效应和应变硬化效应与几何畸变问题,获得的修正后比例模型预测结果与原型动态响应时间曲线的对比如图5所示。图5(a)为锤头的速度-时间响应曲线,图5(b)为薄壁圆管首个折皱处塑性铰位置的等效塑性应变-时间响应,图5(c)、(d)分别为锤头的位移和能量-时间响应曲线,图5(e)为修正前后的薄壁圆管几何畸变比例模型与原型载荷时间-曲线的对比。表7为考虑应变率效应和应变硬化效应,修正后的薄壁圆管几何畸变模型所预测原型的峰值位移与平均载荷与原型响应之间的相对误差。
表 7 考虑应变率与应变硬化效应的比例模型位移与平均载荷相对误差Table 7. Relative errors in the peak displacement and average force of the scaled models considering strain-rate sensitivity and strain hardening模型 (δ/βδ) /mm 相对误差/% (P/βP)/kN 相对误差/% 原型 90.740 − 97.79 − β=1/10, η=1.2 89.619 1.235 98.42 0.644 β=1/10, η=1.5 89.222 1.673 99.47 1.718 β=1/10, η=1.8 86.201 5.002 101.06 3.344 β=1/10, η=2.0 86.232 4.968 105.14 7.516 从图5可以看出,修正前的不同几何畸变度的一般材料薄壁圆管比例模型的响应曲线基本无法重合,各条曲线上的相应点所对应的时间与值均不相等,而修正后的一般材料薄壁圆管几何畸变比例模型与原型的速度、能量、位移、等效塑性应变和载荷-时间曲线重合度明显提高。修正后的比例模型的速度和应变-时间曲线与原型的响应曲线基本完全重合。对于本文推导过程中的3个关键设计参数:能量Ek、位移δ和平均载荷P,修正后的比例模型的位移和能量曲线与原型的响应曲线重合度非常高,但修正后的几何畸变比例模型的载荷-时间曲线与原型重合度不是很高,原因如2.2.1节所述。然而随着几何畸变度的增大,修正后的比例模型的动态响应变量曲线与原型的动态变量响应曲线的重合程度逐渐降低。对比修正后的比例模型与原型的峰值位移和平均载荷,从表7中可以看到,即便几何畸变度增大至2,比例模型所预测的结果与原型的峰值位移相对误差依然不超过6%,平均载荷的误差亦不超过8%。
可见,通过使用本文所推导出的薄壁圆管相似律,对考虑应变率效应和应变硬化效应的一般材料薄壁圆管几何畸变比例模型进行修正后,比例模型可以准确地预测原型的动态响应。
3. 结 论
以受轴向冲击载荷的薄壁圆管为研究对象,基于量纲分析与方程分析,推导了薄壁圆管结构的几何畸变相似律。
(1)通过能量守恒定律,直接建立了结构几何特征与冲击速度和冲击质量的关系,解决了理想弹塑性材料薄壁圆管的壁厚h、半径R和长度L非等比例缩放的不相似问题。
(2)建立应变区间和应变率区间上比例模型预测的流动屈服应力与原型流动屈服应力的最佳逼近关系,解决了考虑材料应变率和应变硬化效应与薄壁圆管几何畸变问题耦合时的不相似问题。
(3)通过数值模拟验证了该方法的有效性,研究表明,使用该方法可大幅降低几何畸变模型预测结果与原结构实际响应的误差,特别是轴向冲击问题备受关注的载荷和能量,相似误差最大不超过8%。
但该相似律只适用于几何畸变比例模型满足薄壳假设且弹性变形能占比可忽略时的薄壁圆管几何畸变相似性分析,对于不满足该条件的薄壁圆管相似性分析还需进一步研究。
-
表 2 受轴向冲击的理想弹塑性薄壁圆管比例因子
Table 2. Scaling factors of the elastic-ideal plastic thin-walled cylindrical shell under axial impact loading
变量 比例因子 变量 比例因子 长度L β=Lm/Lp 位移δ βδ=β 密度ρ βρ=ρm/ρp 应力σd βσd=βρβ2v√β/βh 速度v βv=vm/vp 应变ε βε=√βh/β 质量m βm=βρβ2βh 应变率˙ε β˙ε=(βv/β)√βL/βh 时间t βt=β/βv 载荷P βP=βρββhβ2v 加速度 a βa=β2v/β 动能Ek βEk=βρβhβ2β2v ρ/(g∙cm−3) E/GPa μ A/MPa B/MPa C n ˙ε0/s−1 7.89 207 0.3 350 275 0.022 0.36 1 表 4 理想弹塑性模型几何畸变比例因子
Table 4. Scaling factors of geometrically-distorted models of elastic-ideal plastic material
β η βh βM βv βt βP βδ βε 0.1 1.2 0.12 0.12 1.0466 0.0955 0.0131 0.1 1.0954 0.1 1.5 0.15 0.15 1.1067 0.0904 0.0184 0.1 1.2247 0.1 1.8 0.18 0.18 1.1583 0.0863 0.0241 0.1 1.3416 0.1 2.0 0.20 0.20 1.1892 0.0841 0.0283 0.1 1.4142 表 5 考虑应变率效应和应变硬化效应几何畸变模型比例因子
Table 5. Scaling factors of geometrically-distorted models considering strain-rate sensitivity and strain hardening
β η βh βM βv βt βP βδ βε 0.1 1.2 0.12 0.12 1.0756 0.0930 0.0139 0.1 1.0954 0.1 1.5 0.15 0.15 1.1442 0.0874 0.0196 0.1 1.2247 0.1 1.8 0.18 0.18 1.2038 0.0831 0.0261 0.1 1.3416 0.1 2.0 0.20 0.20 1.2396 0.0807 0.0306 0.1 1.4142 表 6 比例模型与原型的峰值位移和平均载荷相对误差
Table 6. Relative errors in the peak displacement and average force between the scale models and prototype
模型 (δ/βδ)/mm 相对误差/% (P/βP)/kN 相对误差/% 原型 124.258 − 71.95 − β=1/10, η=1.2 121.669 2.084 73.43 2.057 β=1/10, η=1.5 120.651 2.903 69.48 3.433 β=1/10, η=1.8 118.642 4.520 75.85 5.420 β=1/10, η=2.0 117.678 5.295 72.25 0.417 表 7 考虑应变率与应变硬化效应的比例模型位移与平均载荷相对误差
Table 7. Relative errors in the peak displacement and average force of the scaled models considering strain-rate sensitivity and strain hardening
模型 (δ/βδ) /mm 相对误差/% (P/βP)/kN 相对误差/% 原型 90.740 − 97.79 − β=1/10, η=1.2 89.619 1.235 98.42 0.644 β=1/10, η=1.5 89.222 1.673 99.47 1.718 β=1/10, η=1.8 86.201 5.002 101.06 3.344 β=1/10, η=2.0 86.232 4.968 105.14 7.516 -
[1] JONES N. Structural impact [M]. 2nd ed. New York: Cambridge University Press, 2012. [2] 徐海斌, 张德志, 谭书舜, 等. 轴向压缩的金属薄壁圆管相似律的实验研究 [C] // 第20届全国结构工程学术会议论文集. 浙江宁波: 中国力学学会工程力学编辑部, 2011: 554–559. [3] ALEXANDER J M. An approximate analysis of the collapse of thin cylindrical shells under axial loading [J]. The Quarterly Journal of Mechanics and Applied Mathematics, 1960, 13(1): 10–15. DOI: 10.1093/qjmam/13.1.10. [4] ABRAMOWICZ W, JONES N. Dynamic axial crushing of circular tubes [J]. International Journal of Impact Engineering, 1984, 2(3): 263–281. DOI: 10.1016/0734-743X(84)90010-1. [5] KARAGIOZOVA D, JONES N. Influence of stress waves on the dynamic progressive and dynamic plastic buckling of cylindrical shells [J]. International Journal of Solids and Structures, 2001, 38(38/39): 6723–6749. DOI: 10.1016/S0020-7683(01)00111-1. [6] KARAGIOZOVA D, NURICK G N, YUEN S C K. Energy absorption of aluminium alloy circular and square tubes under an axial explosive load [J]. Thin-Walled Structures, 2005, 43(6): 956–982. DOI: 10.1016/j.tws.2004.11.002. [7] LU G, YU J L, ZHANG J J, et al. Alexander revisited: upper- and lower-bound approaches for axial crushing of a circular tube [J]. International Journal of Mechanical Sciences, 2021, 206: 106610. DOI: 10.1016/j.ijmecsci.2021.106610. [8] CASABURO A, PETRONE G, FRANCO F, et al. A review of similitude methods for structural engineering [J]. Applied Mechanics Reviews, 2019, 71(3): 030802. DOI: 10.1115/1.4043787. [9] COUTINHO C P, BAPTISTA A J, RODRIGUES J D. Reduced scale models based on similitude theory: a review up to 2015 [J]. Engineering Structures, 2016, 119: 81–94. DOI: 10.1016/j.engstruct.2016.04.016. [10] OSHIRO R E, ALVES M. Scaling impacted structures [J]. Archive of Applied Mechanics, 2004, 74(1/2): 130–145. DOI: 10.1007/BF02637214. [11] OSHIRO R E, ALVES M. Scaling of cylindrical shells under axial impact [J]. International Journal of Impact Engineering, 2007, 34(1): 89–103. DOI: 10.1016/j.ijimpeng.2006.02.003. [12] 王帅, 徐绯, 代震, 等. 结构冲击畸变问题的直接相似方法研究 [J]. 力学学报, 2020, 52(3): 774–786. DOI: 10.6052/0459-1879-19-327.WANG S, XU F, DAI Z, et al. A direct scaling method for the distortion problems of structural impact [J]. Chinese Journal of Theoretical and Applied Mechanics, 2020, 52(3): 774–786. DOI: 10.6052/0459-1879-19-327. [13] WANG S, XU F, ZHANG X Y, et al. Material similarity of scaled models [J]. International Journal of Impact Engineering, 2021, 156: 103951. DOI: 10.1016/j.ijimpeng.2021.103951. [14] 李肖成, 徐绯, 杨磊峰, 等. 薄板在冲击载荷下线弹性理想塑性响应的相似性研究 [J]. 爆炸与冲击, 2021, 41(11): 113103. DOI: 10.11883/bzycj-2020-0374.LI X C, XU F, YANG L F, et al. Study on the similarity of elasticity and ideal plasticity response of thin plate under impact loading [J]. Explosion and Shock Waves, 2021, 41(11): 113103. DOI: 10.11883/bzycj-2020-0374. [15] 秦健, 张振华. 原型和模型不同材料时加筋板冲击动态响应的相似预报方法 [J]. 爆炸与冲击, 2010, 30(5): 511–516. DOI: 10.11883/1001-1455(2010)05-0511-06.QIN J, ZHANG Z H. A scaling method for predicting dynamic responses of stiffened plates made of materials different from experimental models [J]. Explosion and Shock Waves, 2010, 30(5): 511–516. DOI: 10.11883/1001-1455(2010)05-0511-06. [16] ALVES M, OSHIRO R E, CALLE M A G, et al. Scaling and structural impact [J]. Procedia Engineering, 2017, 173: 391–396. DOI: 10.1016/j.proeng.2016.12.036. [17] MAZZARIOL L M, ALVES M. Similarity laws of structures under impact load: geometric and material distortion [J]. International Journal of Mechanical Sciences, 2019, 157/158: 633–647. DOI: 10.1016/j.ijmecsci.2019.05.011. [18] WANG S, XU F, DAI Z. Suggestion of the DLV dimensionless number system to represent the scaled behavior of structures under impact loads [J]. Archive of Applied Mechanics, 2020, 90(4): 707–719. DOI: 10.1007/s00419-019-01635-9. [19] WANG S, XU F, ZHANG X Y, et al. A directional framework of similarity laws for geometrically distorted structures subjected to impact loads [J]. International Journal of Impact Engineering, 2022, 161: 104092. DOI: 10.1016/j.ijimpeng.2021.104092. [20] 李志斌, 虞吉林, 郑志军, 等. 薄壁管及其泡沫金属填充结构耐撞性的实验研究 [J]. 实验力学, 2012, 27(1): 77–86.LI Z B, YU J L, ZHENG Z J, et al. An experimental study on the crashworthiness of thin-walled tubes and their metallic foam-filled structures [J]. Journal of Experimental Mechanics, 2012, 27(1): 77–86. [21] 朱文波, 杨黎明, 余同希. 薄壁圆管轴向冲击下的动态特性研究 [J]. 宁波大学学报(理工版), 2014, 27(2): 92–96.ZHU W B, YANG L M, YU T X. Study on dynamic properties of thin-walled circular tubes under axial compression [J]. Journal of Ningbo University (Natural Science & Engineering Edition), 2014, 27(2): 92–96. [22] 余同希, 卢国兴, 张雄. 能量吸收: 结构与材料的力学行为和塑性分析 [M]. 北京: 科学出版社, 2019. [23] 白以龙, 黄筑平, 虞吉林, 等. 材料和结构的动态响应 [M]. 合肥: 中国科学技术大学出版社, 2005. [24] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures [C] // Proceedings of the 7th International Symposium on Ballistics. Hague, Netherlands, 1983: 541–547. 期刊类型引用(0)
其他类型引用(6)
-







 下载:
下载:



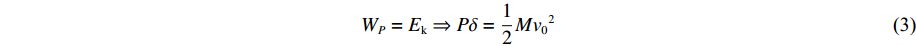

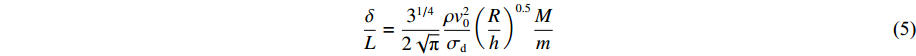
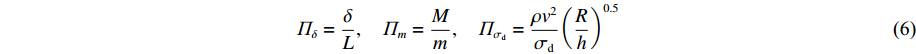



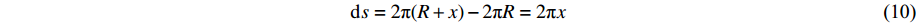
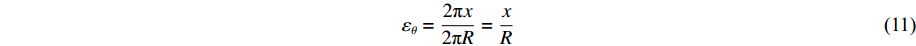
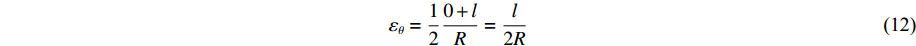






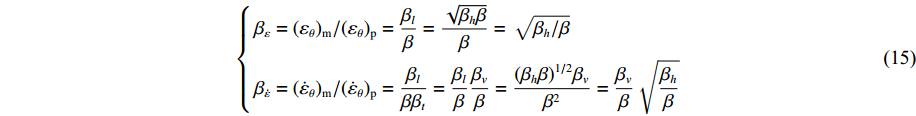





























 下载:
下载: