On dynamic behaviors and failure of bedding coal rock subjected to cyclic impact
-
摘要: 为研究复杂地况下含特征层理煤岩的动态力学行为，采用
∅ 50 mm分离式霍普金森压杆实验系统，对含层理 (0°、30°、45°、60°、90°)煤岩进行动态三轴循环冲击实验研究，并结合3D轮廓扫描仪量化其断裂界面，分析层理效应和围压效应对煤岩动态力学特性及其损伤破坏规律的影响。研究表明：围压的施加使煤岩应力-应变曲线出现弹性后效现象；较无围压状态，抗压强度提高3.9~4.2倍，失效应变增大2.59~3.05倍。随着层理角度的增大，煤岩的动态抗压强度、弹性模量和能量透射率均呈现先降低后升高的U形分布，在层理角为45°时均达到最小值；能量吸收率和断面粗糙度呈现先增大后减小的∩形分布，损伤变量呈现N形分布，在层理角为45°时达到最大值。煤岩的损伤破坏特征随层理角度的变化可概括为张拉破坏（0°）-剪切破坏（30°、45°和60°）-劈裂破坏（90°）的演变过程，所得特征规律可为实际复杂环境下煤层气资源安全高效开采提供理论支持。Abstract: Dynamic triaxial cyclic impact experiments on the coal rock samples with the bedding angles of 0°, 30°, 45°, 60°, and 90°, respectively, were conducted using a 50-mm split Hopkinson pressure bar (SHPB) system to study the dynamic mechanical behaviors of the coal rock with characteristic bedding under complex ground conditions. A 3D profile scanner was utilized to quantify the fracture interface roughness and to investigate the bedding effect on the dynamic fracture process of the coal rock. The bedding angle effect and confining pressure effect on the dynamic properties of the coal rock were explored by combining dynamic parameters such as compressive strength, elastic modulus, energy distribution evolution with the fracture surface roughness variation. The research shows that when confining pressure is applied, the stress-strain curve of the coal rock has an elastic aftereffect. The dynamic compressive strength and failure strain of the bedding coal rock with confining pressure are respectively 3.9−4.2 and 2.59−3.05 times higher than those without confining pressure. As the bedding angle increases, the dynamic compressive strength, elastic modulus, and energy transmitted ratio of the coal rock display the U-shaped distribution, which decreases first and then increases, reaching the minimum at the bedding angle of 45°. Meanwhile, the energy absorbed ratio and fracture surface roughness show the ∩-shaped distribution, first increasing and then decreasing, and the damage variable shows the N-shaped distribution, reaching the maximum at the bedding angle of 45°. The failure of the coal rock with 45° bedding is the most serious, which is more prone to intergranular and spalling fractures. However, the 90° bedding coal rock is more likely to absorb energy and to form transgranular fractures, resulting in a large number of mesoscopic fractures. Variation of the damage characteristics of the coal rocks with bedding angle can be summarized as a tensile damage (0°)-shear damage (30°, 45°, 60°)-splitting damage (90°) evolution process. The relevant characteristic results obtained from the experiments can provide a theoretical support for the safe and efficient exploitation of coalbed methane resources in the complex environment under practical working conditions. -
煤层气资源能源是世界范围内储量巨大的重要非常规能源之一，煤-气共采技术不仅可以预防煤矿瓦斯灾害，还可以增加可利用瓦斯资源，提高煤矿经济开采。目前，使用最有效的地表钻孔开采技术机理是排出煤层承压水，降低煤储层压力，促进吸附甲烷解吸成大量游离甲烷，在地层压力和井筒压差的作用下向井口运移[1-6]。而水力压裂作为目前的主要手段，因向井中倾倒大量的水、沙子和化学物质，涉及污染环境并引发地震等相关问题，已经被越来越多的国家禁止。由于资源开发的迫切需求，越来越多的学者开始关注爆燃压裂方法[7-8]。然而，动态压裂技术相对较新，其机理较少受到重视，随着煤炭资源开采深度的增加，爆燃压裂法在煤层气开采中面临的挑战也越来越大。首先，煤在深部环境中受到地应力，这导致了动态特性的显著变化；其次，无论是钻孔爆破法还是爆燃压裂法，单次冲击并不一定能使煤层破碎或达到预期效果。在实际应用中，它经常受到多次加载，循环效应下的动态力学特性表现出明显的变化[9-11]；最后，煤岩作为典型的沉积岩，其内部存在许多薄弱结构面，如微裂缝和层理，导致其力学性能复杂[12-14]。随着开采深度的不断深入，深部煤岩地层情况愈加复杂，相关资源的开采难度也与之俱增。因此，研究含层理角度煤岩的动态力学响应成为当前煤层气高效开采的关键科学问题之一。
众多学者已对煤岩的力学性质进行了大量的研究。如Zhao等[15]、Kong等[16]、Hao等[17]和Liu等[18]研究了煤岩的静态和动态力学行为，虽然前者已对煤岩的静、动态力学的做了大量的研究工作，然而对含层理煤岩的研究仍比较少，已逐渐成为相关领域的难点和热点问题之一。Chen等[19]研究了不同层理对煤岩的力学性质和渗透性的影响，发现随着层理角度的增大，通道数量随着初始渗透率的增加而增加，抗压强度呈现先降低后升高的趋势。Zhao等[20]利用分离式霍普金森压杆（split Hopkinson pressure bar, SHPB）实验系统研究了不同层理角度对煤岩断裂韧性的影响，发现层理引起的各向异性对煤岩的动态启动断裂韧性起着重要作用。Wang等[21]采用SHPB实验系统和高速摄影技术发现层理影响裂纹的起裂方向，裂纹扩展路径由最大主应力方向和层理共同决定；当层理角度方向垂直于冲击方向时，动态断裂韧性较大；随着层理角度的增大，试件的损伤逐渐由拉伸损伤向拉剪耦合损伤转变。Fan等[22]利用SHPB实验系统和3D轮廓扫描仪研究了不同层理角度和温度对页岩的动态影响，发现不同层理角度的页岩临界温度不同；动态抗压强度随层理角度的增大呈现先降低后升高的趋势，其趋势与断面粗糙度呈正相关。王卫华等[23]研究了应力波在等效节理处的传播规律，并建立了层理非线性位移不连续模型，研究了层理初始刚度、层理合合度对层理透射反射系数的影响。李业学等[24]引入分形损伤理论研究应力波穿透节理时的透、反射效应，建立了分形损伤本构模型。
综上所述，相关研究均对含特征层理岩石的静、动态力学特性方面取得了一定的研究成果，其中一方面，上述研究仅聚焦单轴压缩或劈裂实验；另一方面，当煤层被钻爆法、燃爆法致裂时，单次冲击并未能使煤层破裂充分或达到预期效果。然而实际煤层气资源开发中煤岩在深部地层条件下会受到多次燃爆冲击，形成成熟、贯通的裂缝网络，从而最大效率地实现单井煤层气开采，因此仅在单轴冲击条件下研究不同层理角度煤岩的动态力学性能并不能完全揭示真实的破坏特征规律。故此本文研究了动态三轴压缩作用下含特征层理煤岩在循环冲击下断裂的动态力学行为及损伤破坏演化特征，深入研究了层理角度对煤岩力学特性和能量演变的影响规律，以期待高效压裂深部含特征层理煤岩提供关键力学基础科学数据支撑。
1. 动态冲击实验
1.1 试样加工过程
煤岩均产自陕西省赋谷县张明沟煤矿，为避免煤岩强度离散性对实验结果的影响，选取同一煤岩加工含不同层理 （0°、30°、45°、60°、90°）的标准煤岩试样，如图1所示。
所有试样层理角度清晰可见，加工精度满足GB/T 50266—2013《工程岩体试验方法标准》的要求。将用于SHPB (split Hopkinson pressure bar)实验的煤岩全部加工成直径50 mm、高25 mm的标准圆柱体试样，并对煤岩试样进行抛光处理，保证2个加载面的表面光洁度和平整度均小于0.02 mm，并在冲击测试前用保鲜膜包裹保护，以防止碰撞损坏和空气湿度影响。采用分辨率为0.5～10 μm的NanoVoxel3502E CT装置对冲击前具有代表性的0°层理煤岩进行扫描分析，如图2所示，CT扫描煤岩具有明显的贯通层理面，且分布均匀。
1.2 SHPB实验系统及理论基础
实验在
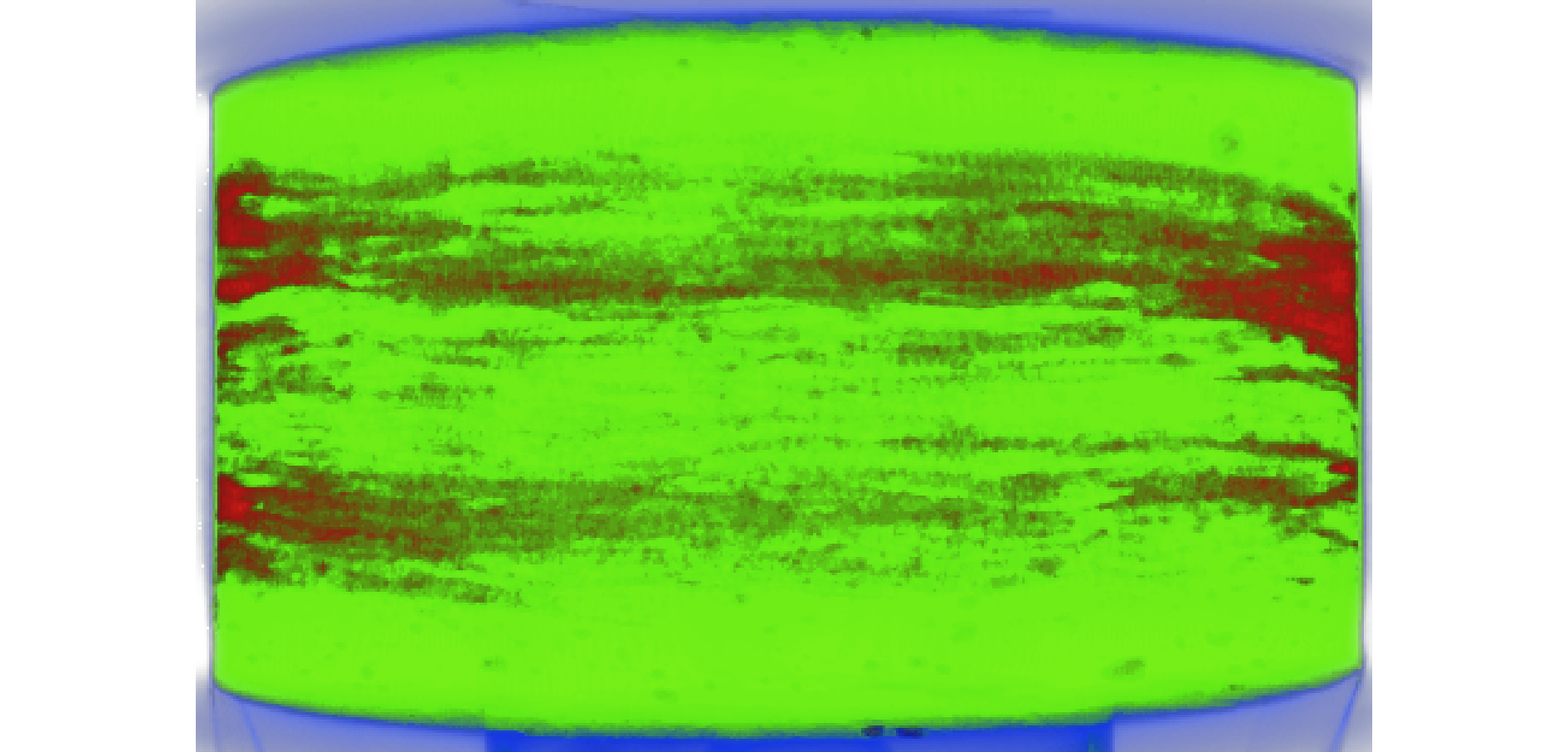
∅ 50 mm SHPB系统上完成，系统装置如图3所示，整个实验系统包括发射操作装置、冲击杆、入射杆、透射杆、缓冲杆、围压加载设备、轴向加载设备、激光测速仪和动态实验分析器。冲击杆、入射杆、透射杆和缓冲杆的长度分别为500、3000、3000和1200 mm。杆件材料采用硅锰弹簧钢，具有高强度、弹性和稳定性等特点，弹性模量为210 GPa，泊松比为0.29，纵波波速为5188 m/s，密度为7800 kg/m3。在入射杆撞击煤岩时，入射波会产生反射波和透射波，由于杆件与煤岩的波阻抗失配，应力波在煤岩中来回反、透射，最终实现应力平衡。为了验证煤岩试件的应力均匀性假设，采用下式计算试件两端的受力：
F1=AbEb(εi+εr),F2=AbEbεt (1) 式中：F1为煤岩试样靠近入射杆的界面所受的力；F2为煤岩试样靠近透射杆的界面所受的力；
εi 、εr 和εt 分别为入射应变脉冲、反射应变脉冲和透射应变脉冲；Ab 和As 为杆件和煤岩试样的横截面积；Eb 为杆件弹性模量。实验结果如图4所示，煤岩加载面两端应力曲线基本重合，即p1=p2，说明在整个动态实验过程中，试件内部各部位的应力应变几乎都处于均匀状态，即εi+εr=εt [25-26]。因此，可以用二波法代替三波法，即用下式：σs=AbAsEbεt,˙εs(t)=−2c0Lsεr,εs=−2c0Ls∫t0εrdτ (2) 代替下式：
σs=Ab2AsEb(εi+εr+εt),˙εs(t)=c0Ls(εi−εr−εt),εs=∫t0˙εs(τ)dτ (3) 计算煤岩冲击损伤后的应力-应变曲线[27-28]。式中：
σs 、˙εs(t) 和εs 分别为煤岩试样的应力、应变率和应变，c0 为杆的弹性纵波速度，Ls 为煤岩试样的初始长度。1.3 SHPB压杆的能量分析
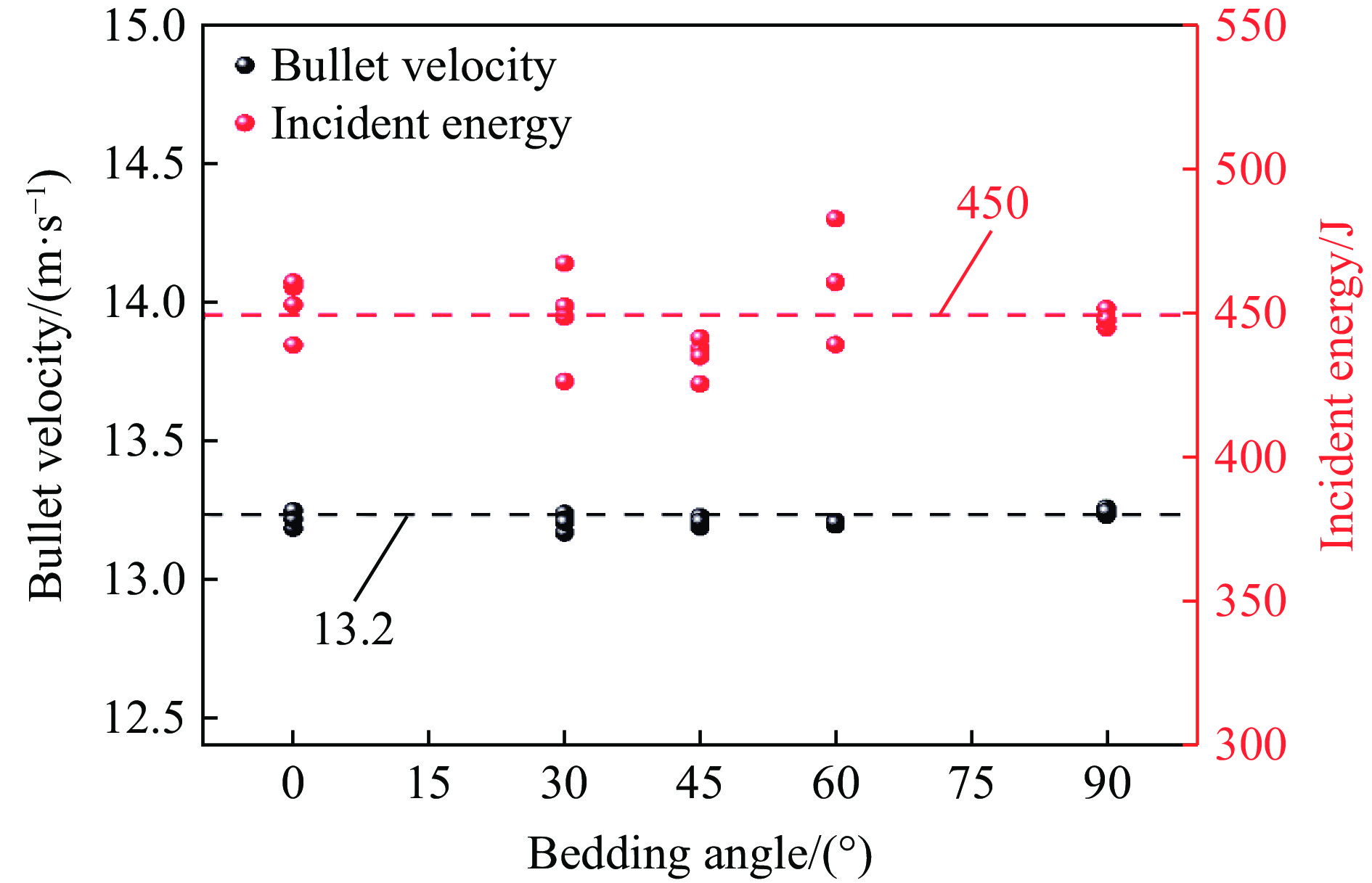
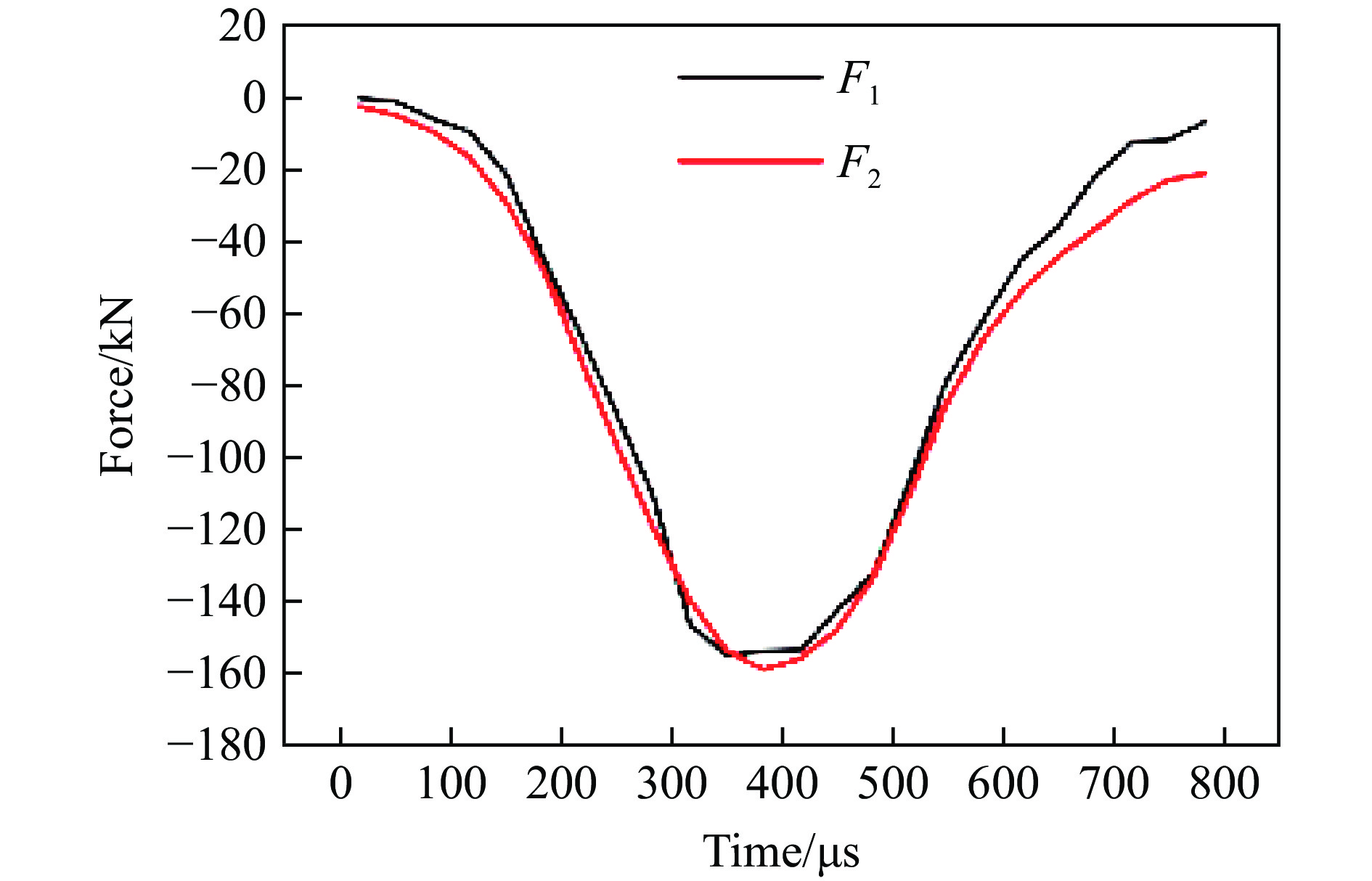
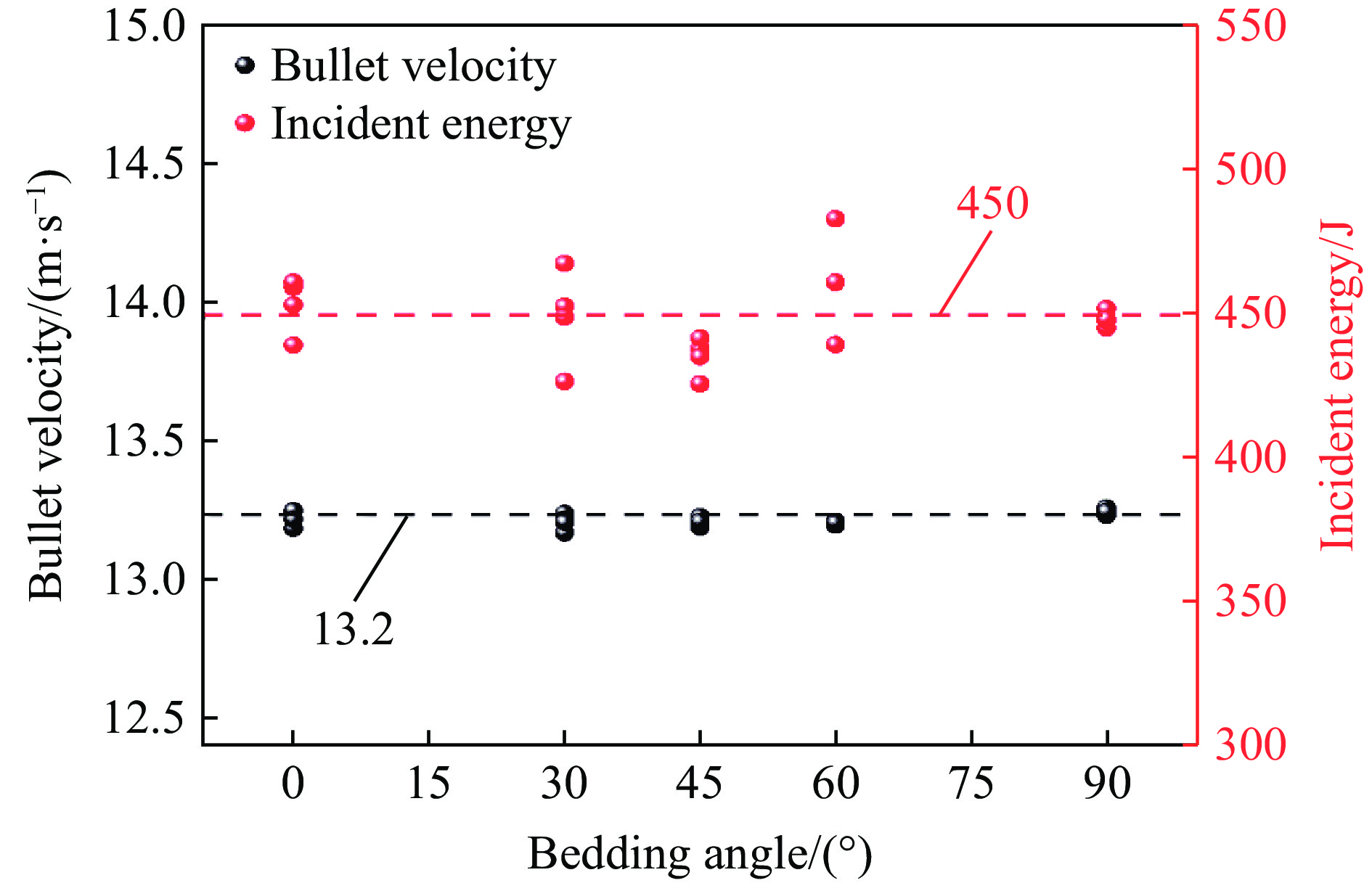
含特征层理煤岩的动态测试过程分为单轴压缩冲击实验和动态三轴循环冲击实验，在单轴压缩冲击实验采用0.3 MPa冲击压力单次冲击煤岩，并以单轴破坏结果数据为基础研究围压效应对煤岩的影响，在动态三轴循环冲击实验中使用1.0 MPa冲击压力进行循环三次冲击，研究动态三轴压缩下层理效应对煤岩的影响。如图5所示，动态三轴循环冲击实验过程中，子弹速度均可控制在(13.216±0.025) m/s。然而，在计算入射能量时发现，设备在冲击实验过程中或多或少存储或消耗了一些能量，导致入射能量略有偏差。为避免实验装置的影响，采用反射能、透射能和吸收能占入射能的百分比，来研究能量分配的变化：
图 5 含不同特征层理煤岩动态测试中的子弹速度和入射能量Figure 5. Velocity and incident energy of the bullets in dynamical tests on coal rock samples with different bedding anglesWx=Abc0Eb∫t0σ2x(t)dt=Abc0Eb∫t0ε2x(t)dt,Wa=Wi−(Wr+Wt),λy=WyWi (4) 式中：x=i, r, t；
Wi 、Wr 和Wt 分别为入射、反射和透射应力波的能量；σi 、σr 和σt 分别为入射、反射和透射应力波；Wa 为吸收应力波能量；y=r, t, a；λr 、λt 和λa 分别为反射能、透射能和吸收能与入射能的比。2. 应力-应变曲线的变化特征
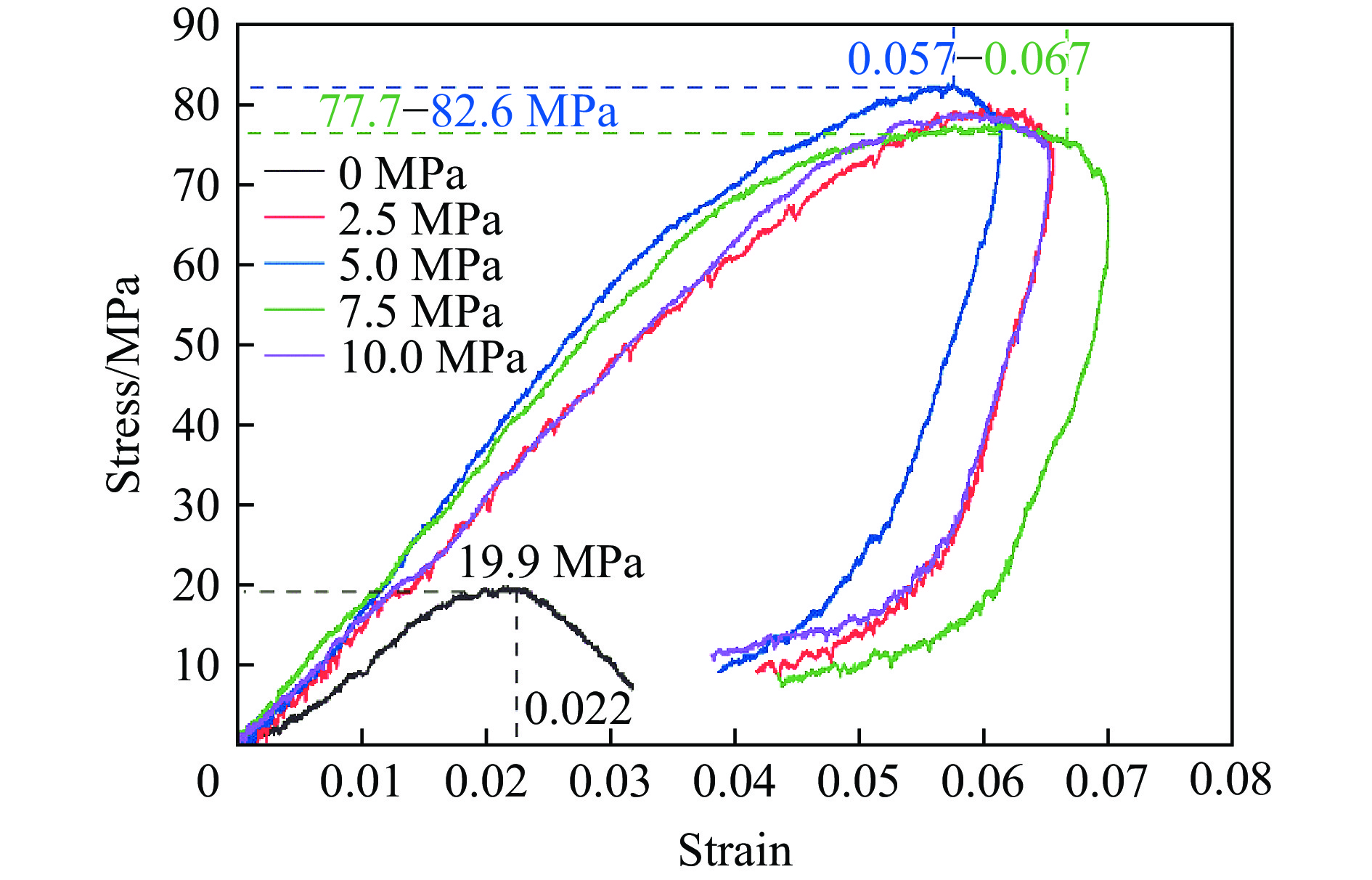
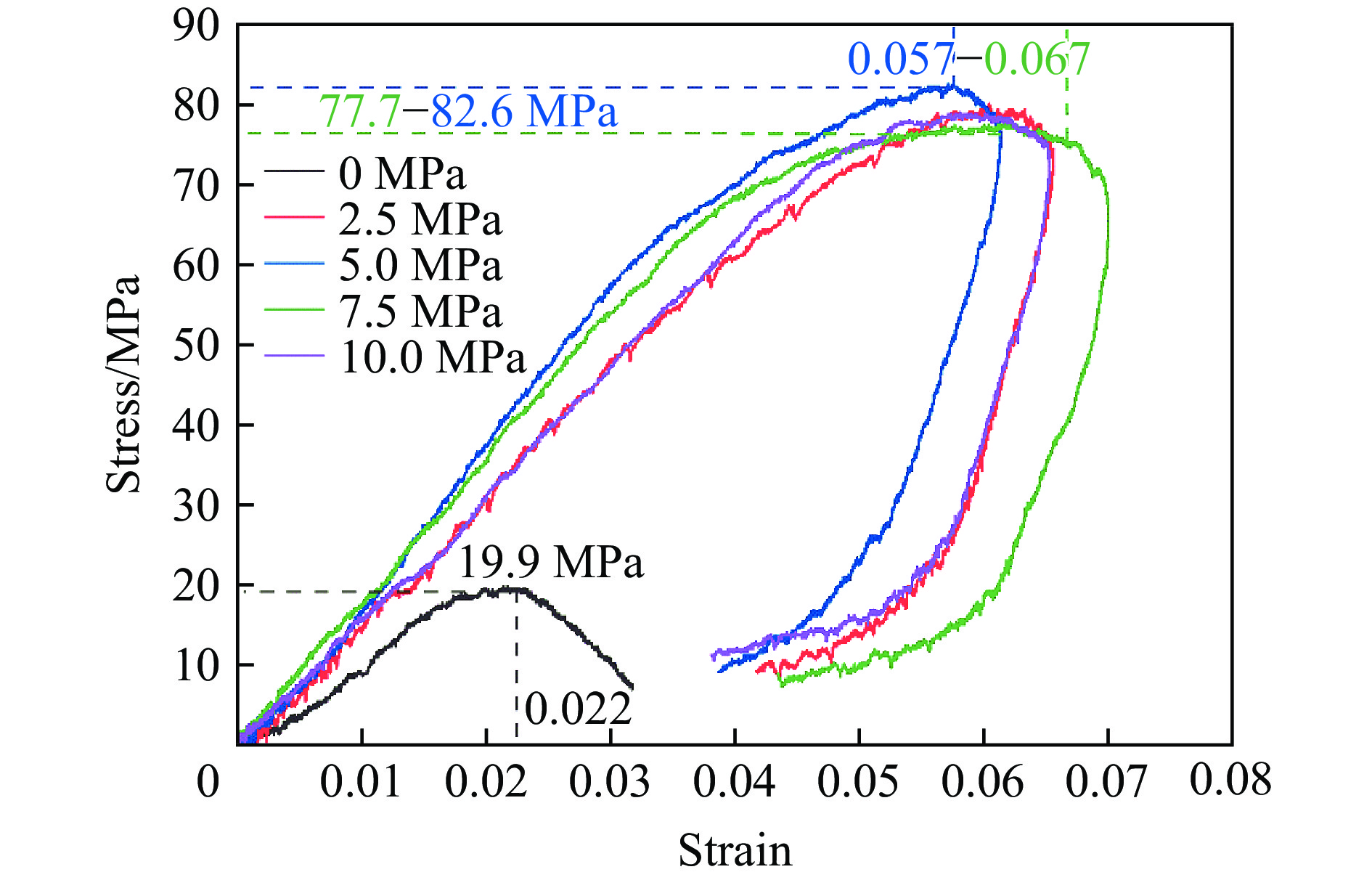
选取不同围压下45°层理煤岩的应力-应变曲线进行分析，发现含层理煤岩对有无围压的敏感性显著。如图6所示，45°层理煤岩的应力-应变曲线在围压状态下均出现弹性后效现象，围压促使煤岩的力学性质从脆性向延性转变。单轴冲击压缩下，煤岩的动态抗压强度和失效应变分别为19.9 MPa和0.022；当施加围压后，层理煤岩的动态抗压强度和失效应变明显增大，分别增大3.9～4.2和2.59～3.05倍。围压的施加增强了煤岩内质点的内聚力，使晶格不易破坏，增大了煤岩的极限强度和韧性[29-30]。
图 6 不同围压下45°层理煤岩的应力-应变曲线Figure 6. Stress-strain curves of 45° bedding coal rock under different confining pressures3. 煤岩动态力学特性的层理效应
3.1 动态抗压强度的变化特征
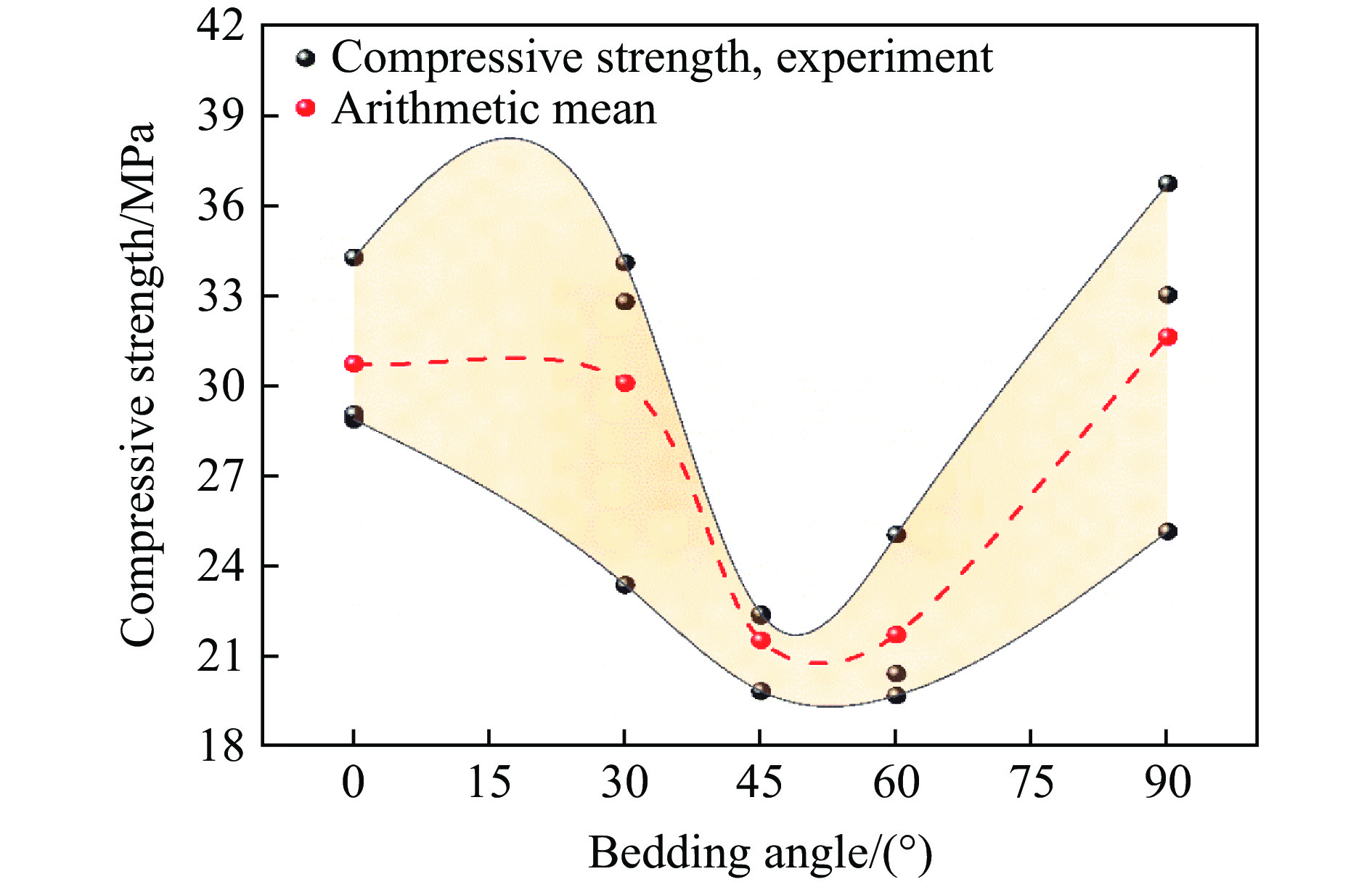
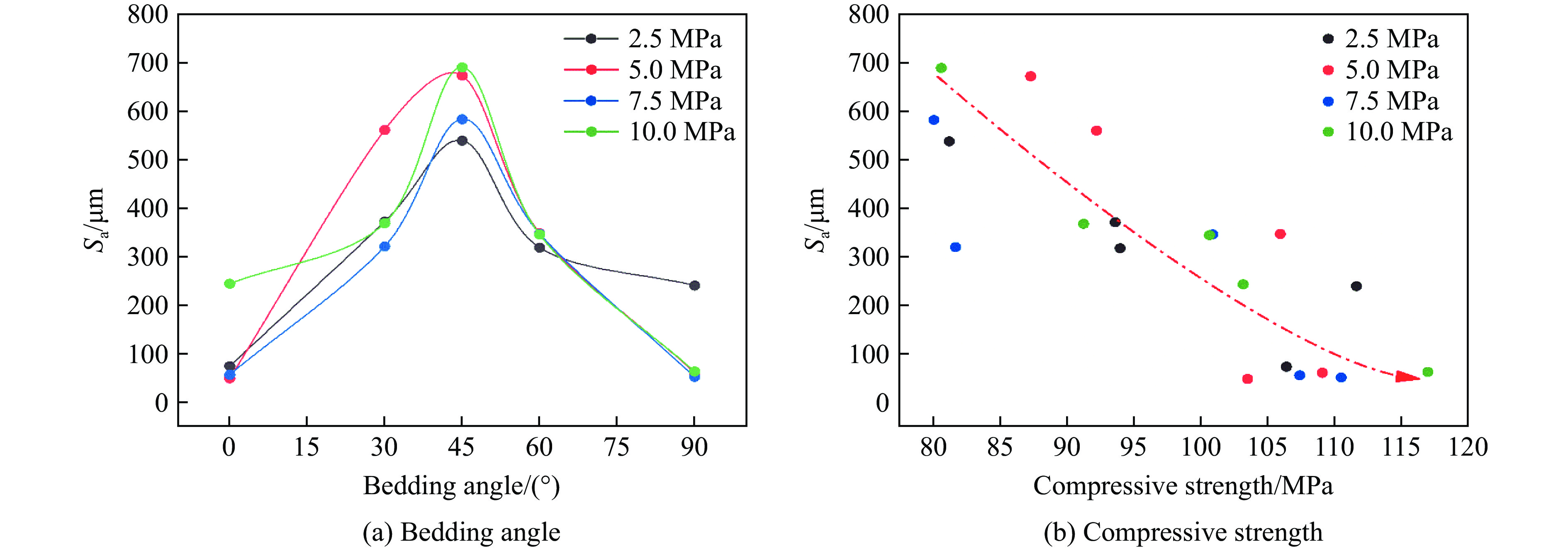
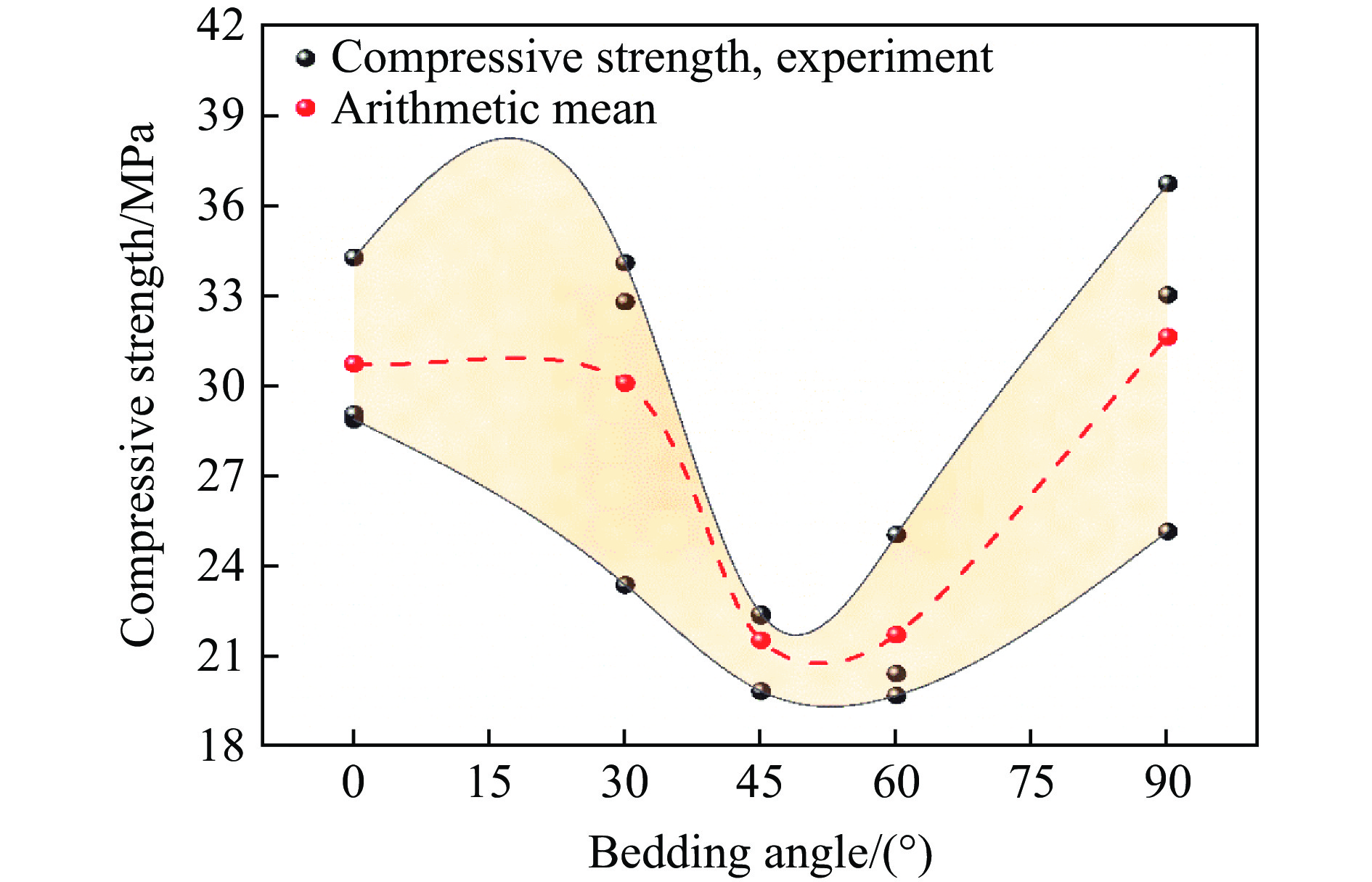
单轴压缩冲击和动态三轴压缩冲击状态下，煤岩的动态力学性能差异明显。在研究围压效应时，基于单轴冲击压缩破坏数据，如图7所示，将实验所得数据采用样条曲线拟合连接（以下数据结果若无明确标注则均为此方式连接），发现单轴压缩冲击下的动态抗压强度随层理角的增大呈U形分布，其中0°和90°层理煤岩的强度最高。
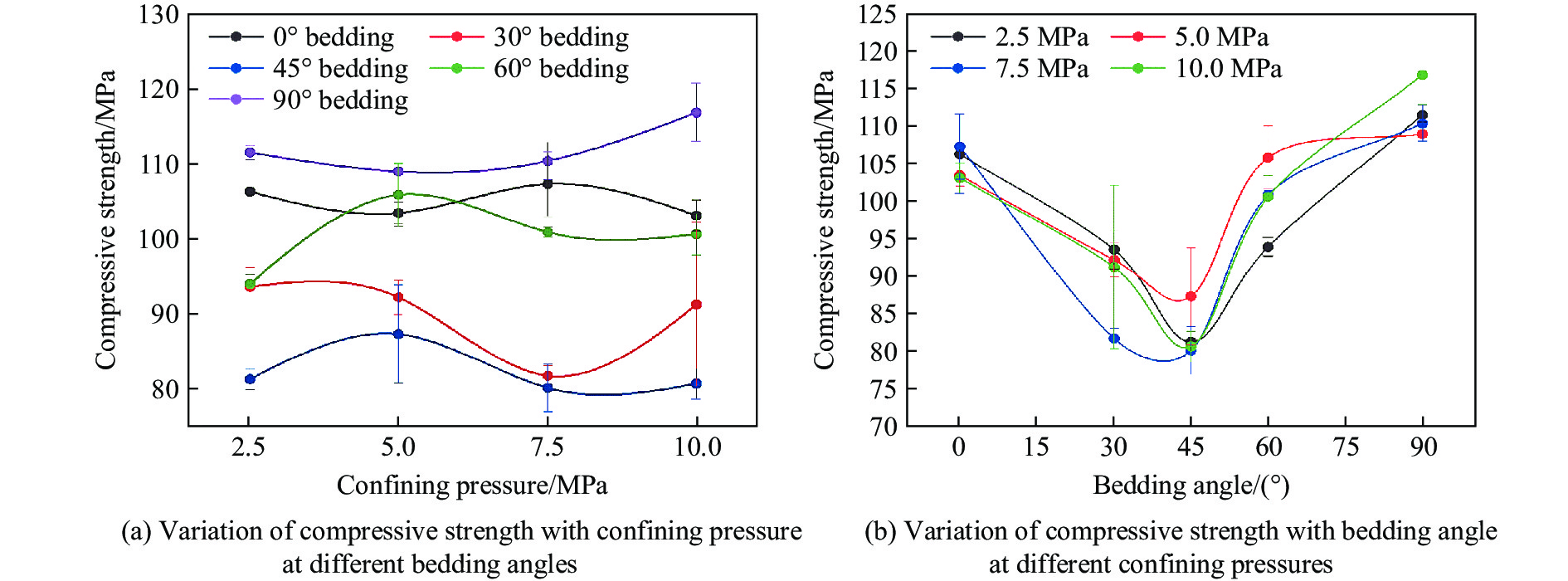
图 7 无围压条件下含不同特征层理煤岩试样的动态抗压强度Figure 7. Dynamic compressive strengths of coal rock samples with different bedding angles without confining pressure如图8(a)所示，不同层理角度煤岩的动态抗压强度并没有随围压的增大而呈现单一的增大或减小的变化趋势。图8(b)显示，煤岩的动态抗压强度则随层理角度的增大呈现明显的U形变化趋势，90°层理煤岩的抗压强度最高，0°层理煤岩的抗压强度次之，45°层理煤岩的动态抗压强度最低。因为90°层理方向与围压作用方向垂直，能较好地压密煤岩内部的孔隙和裂隙，对其动态抗压强度的提高最显著。当层理方向与围压方向存在夹角（如30°、45°、60°）时，层理面之间会发生摩擦或滑移，对其抗压强度有增强效果，但明显低于90°层理煤岩。
图 8 抗压强度随围压和层理角度的变化Figure 8. Variation of compressive strength with confining pressure and bedding angle3.2 动态弹性模量的变化特征
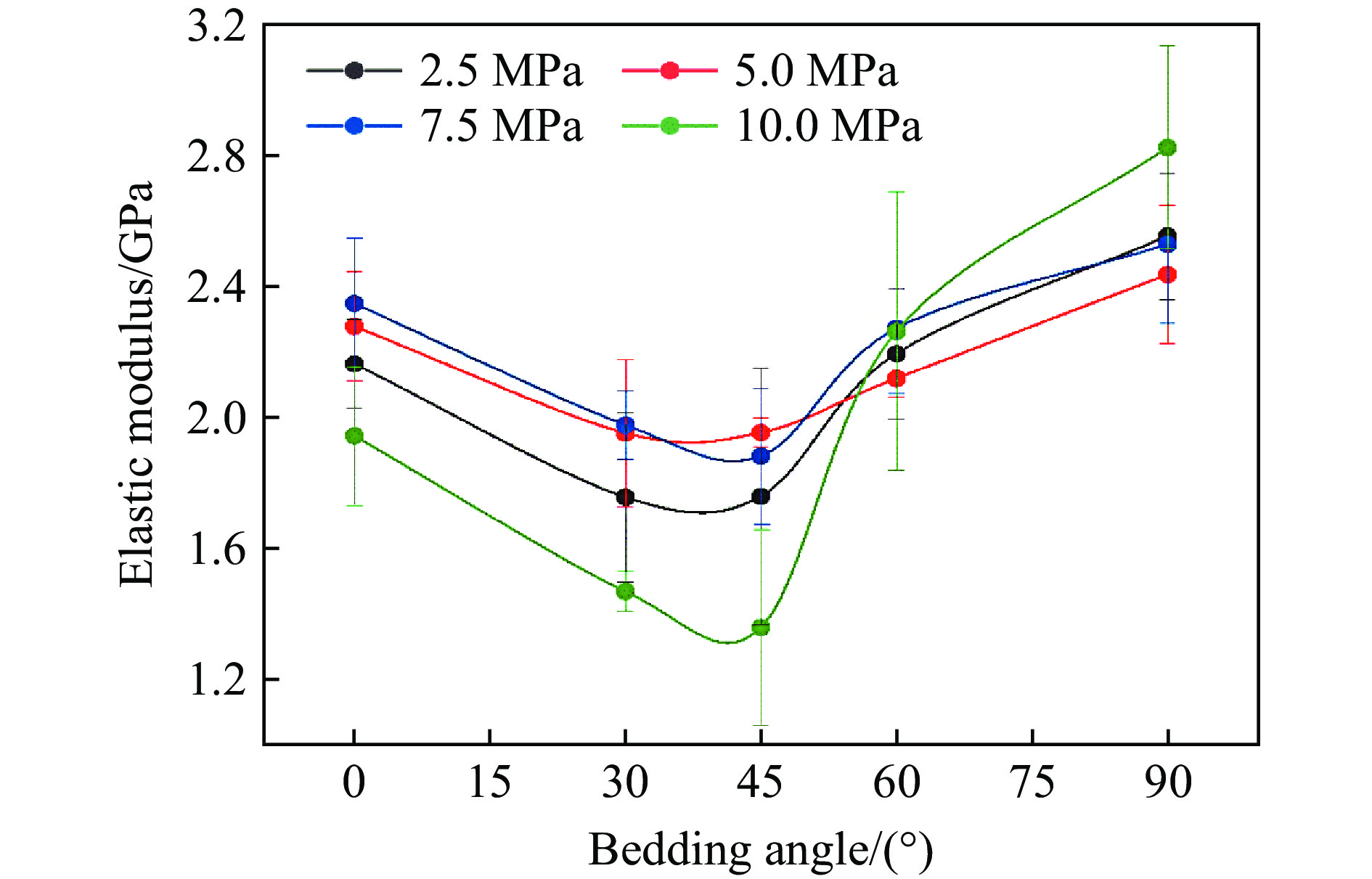
弹性模量是物体抵抗弹性变形能力的量度。采用峰值应力的0.4倍和0.6倍的应力差与应变差之比计算煤岩的动态弹性模量：
E=σ2−σ1ε2−ε1 (5) 式中：
ε1 和σ1 分别为0.4倍峰值应力时的应变和应力，ε2 和σ2 分别为0.6倍峰值应力时的应变和应力。如图9所示，弹性模量随层理角度的增大呈现先减小后增大的趋势。在90°层理时最大，45°层理时最小。因为45°层理煤岩的抗压强度最低，更易发生破坏变形，细裂纹的发展降低了煤结构传递荷载的能力和比例，降低了材料性能，导致弹性模量降低，此规律与抗压强度相似，因此认为含层理煤岩的动态力学参数的变化并不是孤立存在，而是相互影响。
图 9 不同围压下煤岩弹性模量随层理角度的变化Figure 9. Variation of elastic modulus of coal rock with bedding angle at different confining pressures4. 煤岩能量演变的层理效应
4.1 能量分配率的变化特征
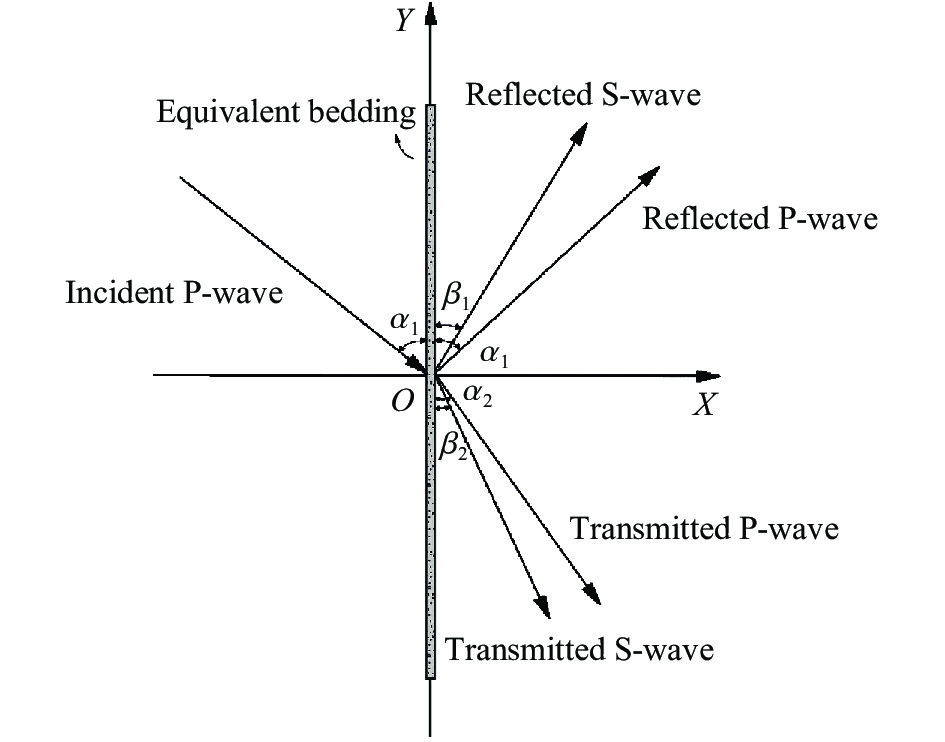
如图10所示，入射纵波以α1的角度通过层理面时，透射与反射效应同时发生，产生反射横波、反射纵波、透射横波、透射纵波[23-24]。不同层理面的α1不同，能量的透射和反射也会有很大的不同，因此，研究不同层理煤岩的能量变化率有非常重要的理论意义。
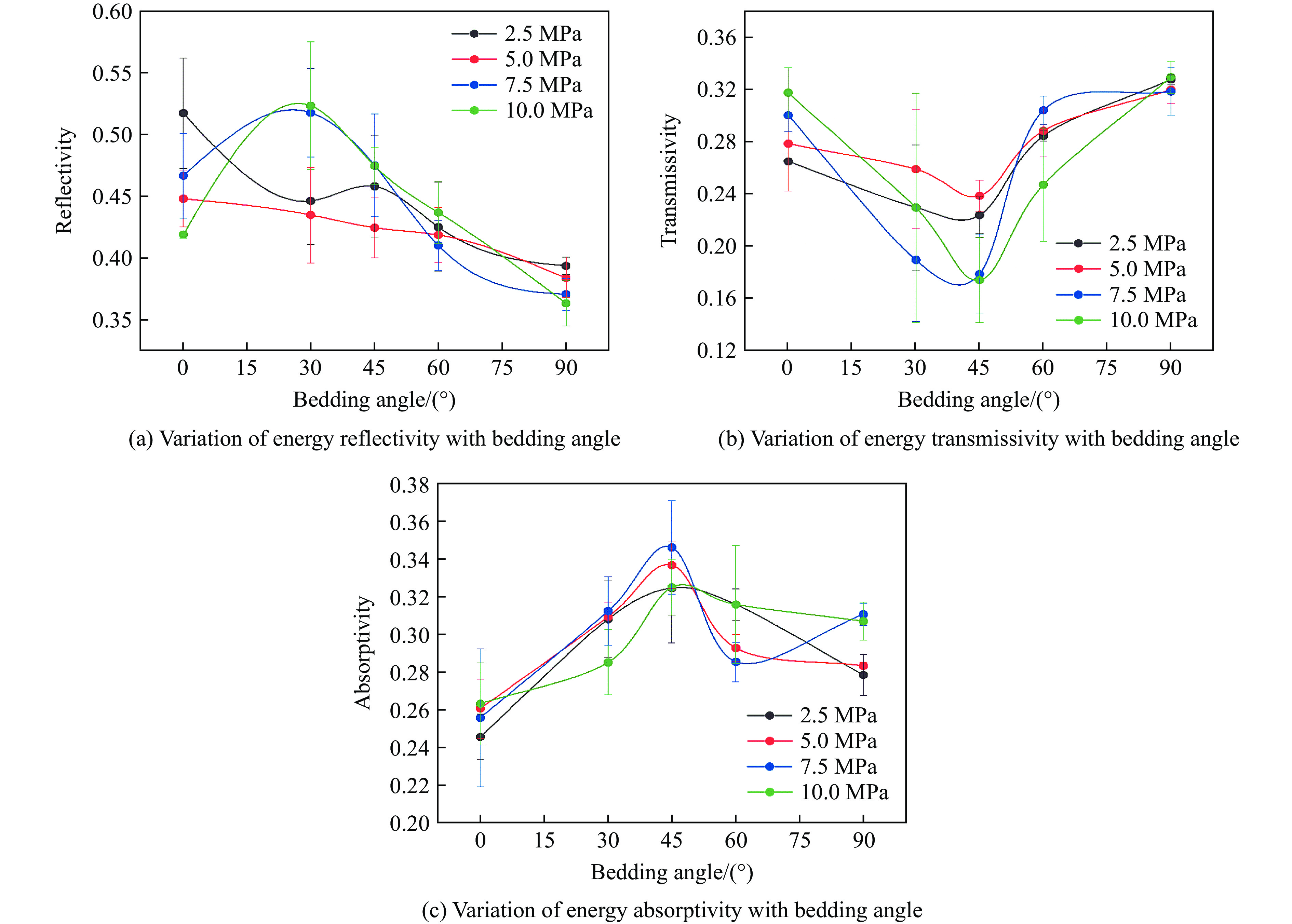
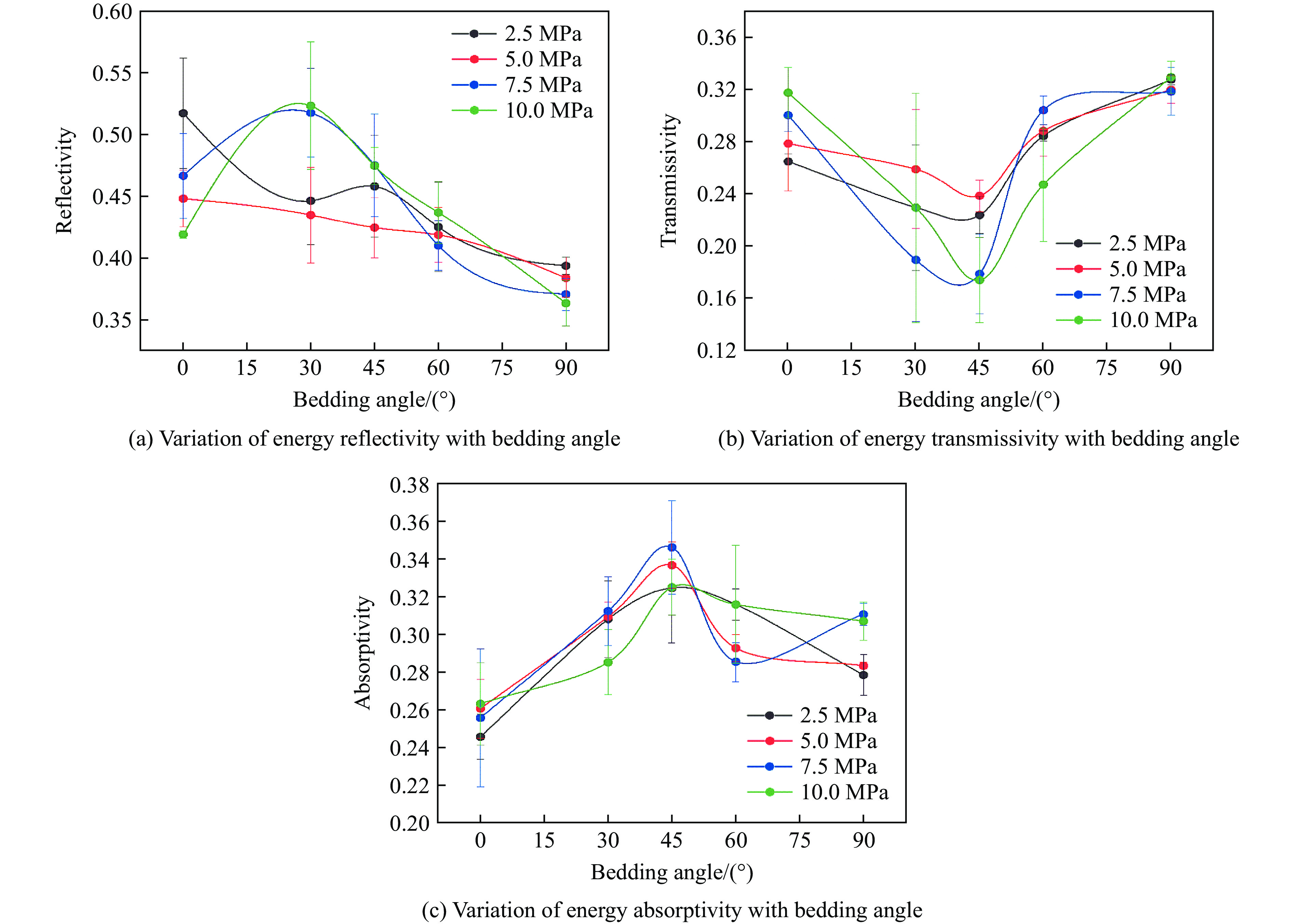
图 10 P波在等效层理面上的反射和透射Figure 10. Reflection and transmission of P-waves at the equivalent bedding plane如图11(a)所示，当研究煤岩的能量反射率时，发现2.5和5.0 MPa围压下能量反射率随层理角度呈现下降趋势，90°层理煤岩的能量反射率最小，0°层理煤岩的反射率最大，7.5 和11.0 MPa围压下的能量反射率呈现先增大后减小的趋势，在30°层理角度处达到最大。因为过高的围压施加在煤岩上，层理因为围压的施加而进一步发育，导致层理角度有所改变，能量的演化受围压和层理共同影响。
图 11 不同围压下煤岩能量分配率随层理角度的变化Figure 11. Variation of energy distribution ratio of coal rock with bedding angle at different confining pressures如图11(b)所示，能量透射率与层理角度呈现U形趋势，这一趋势与抗压强度的趋势相似，抗压强度越高，能量透射程度越高，其中90°层理煤岩的透射率最高，其次是0°层理煤岩，0°和90°层理煤岩能更好地保证能量的穿透和传递，45°层理煤岩的透射率最低，说明角度层理（30°，45°，60°）煤岩更能降低能量的透射。
如图11(c)所示，能量吸收率与层理角度呈现先增后减的∩形趋势，与抗压强度和弹性模量呈负相关，与峰值应变呈正相关，45°层理煤岩的能量吸收率最大，0°层理煤岩吸收率最低。因为在应力波作用0°和90°层理面时，应力波以反射和透射效应为主，当作用45°层理面时，更多的应力波能量被用于破坏断裂层理面，能量吸收率达到最大。
4.2 损伤变量的变化特征
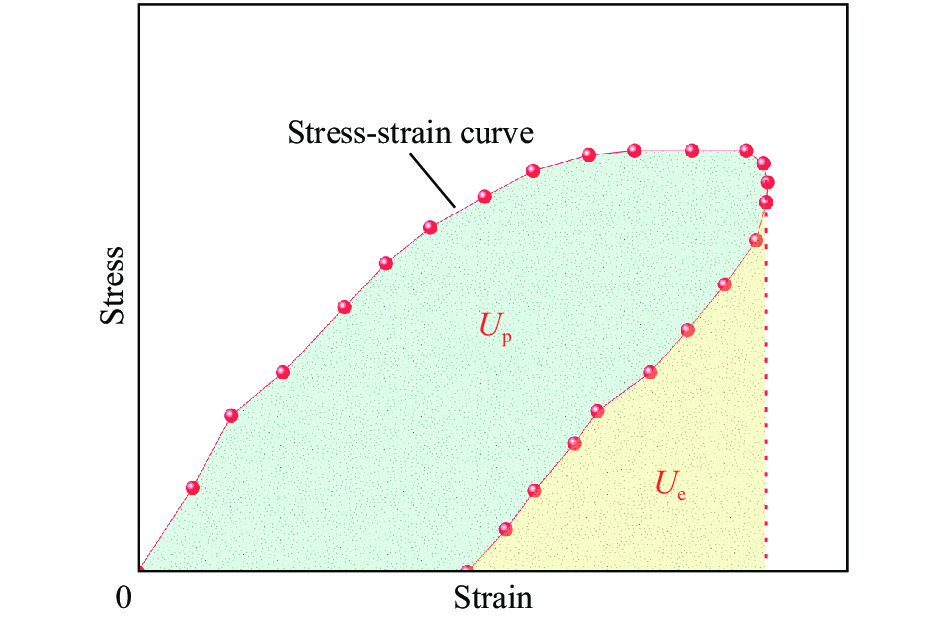
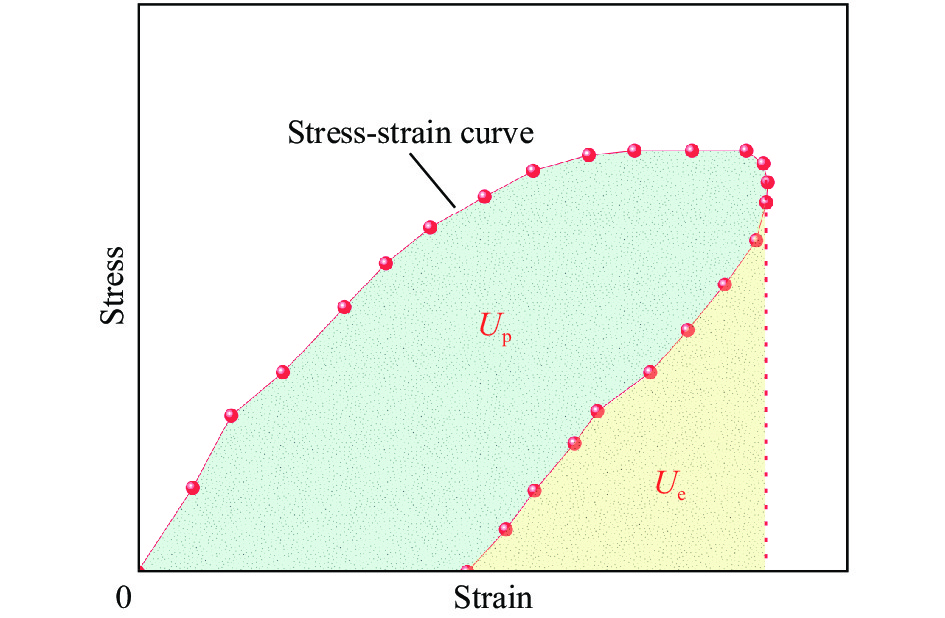
谢和平等[31]指出岩石失稳和破坏损伤的本质是能量释放和耗散的演化过程。煤岩作为典型沉积岩，采用基于能量耗散理论的损伤变量可以更好地揭示其内部损伤的本质变化，因此按照耗散结构理论，将损伤变量定义为吸收能量密度与本构能的比值。如图12所示，本构能Up由煤岩应力（
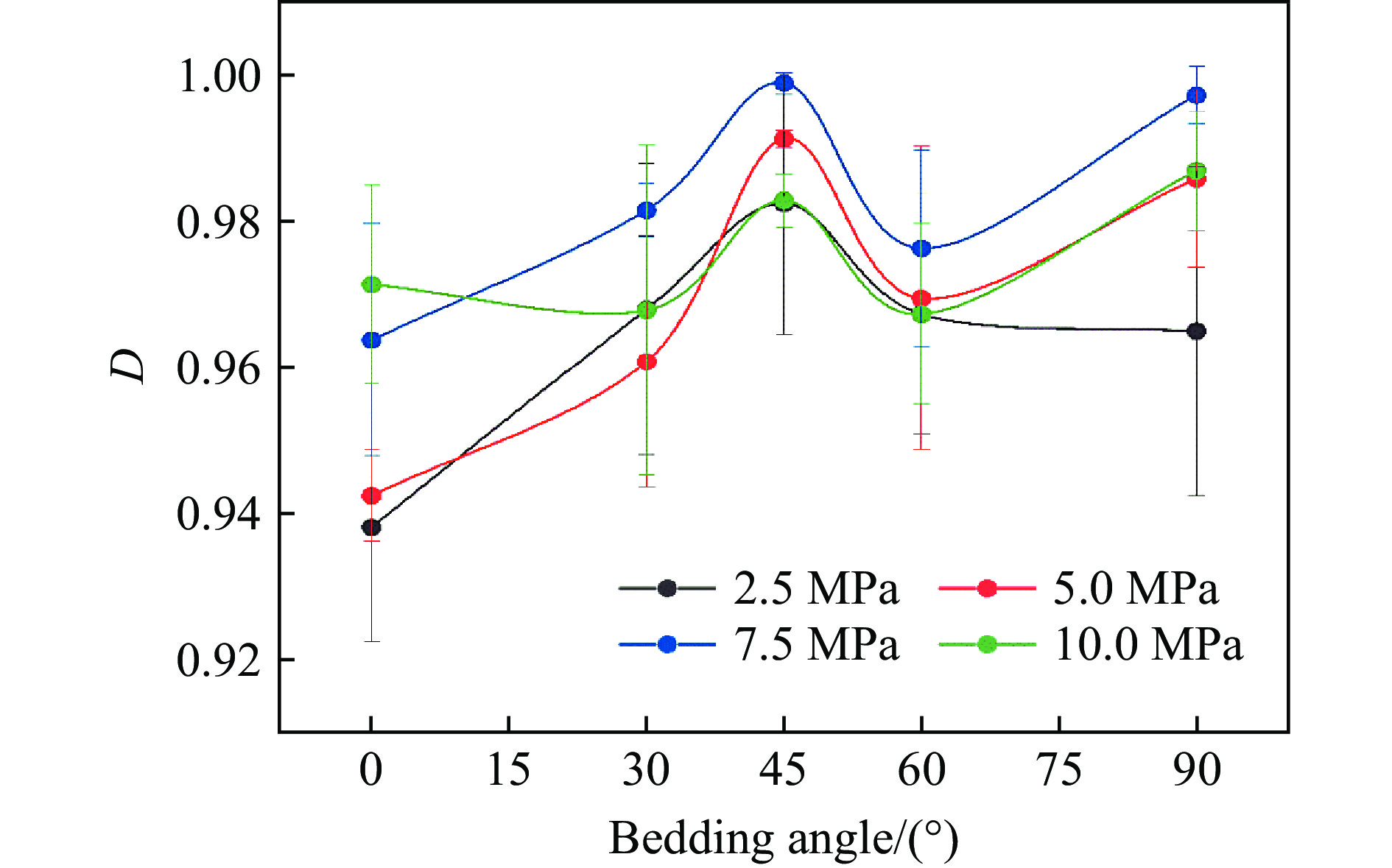
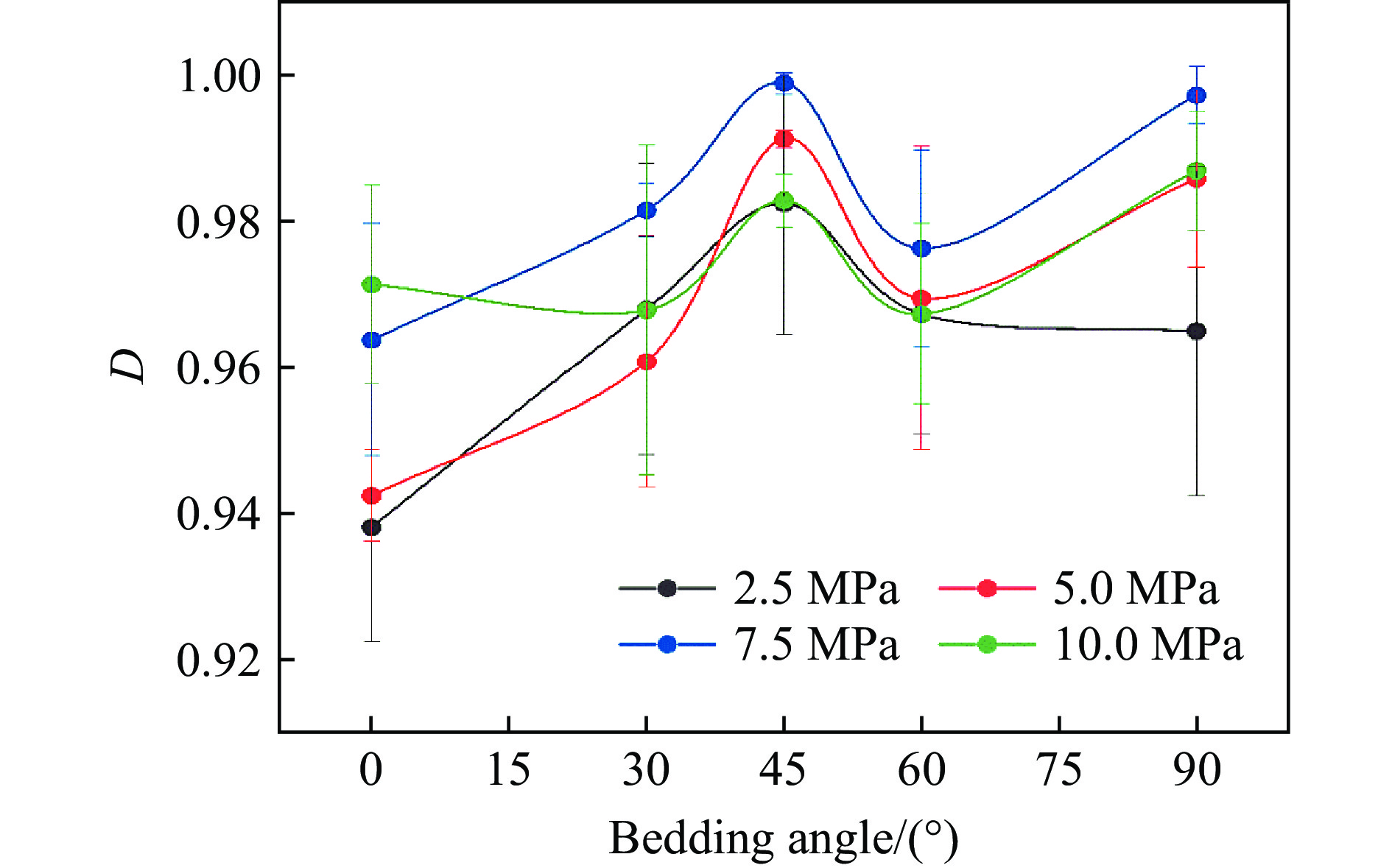
σ ）-应变（ε ）曲线进行积分得到，即Up=∫σdε ，表示单元发生损伤和塑性变形时所消耗的能量；Ue为单元内存储的可释放应变能，为岩石单元卸载后释放的弹性应变能。此外，引入吸收能量密度Uc=Wc/Vc （Wc为煤岩的吸收能，Vc为煤岩的体积），表示煤岩单位体积吸收能量[32-33]；将D=Uc/Up 定义为损伤变量[34]。
图 12 体积能量耗散和可释放应变能Figure 12. Energy dissipation and releasable strain energy per unit volume当D=0时，煤岩单元体无损伤，无能量耗散；当D=1时，煤岩单元体能够承受所有的能量耗散，煤岩被完全破坏。如图13所示，D与层理角度呈N形分布，层理角度为45°或90°时，D达到最大值。煤岩吸收能量用于裂纹的发育、扩展或贯通：在45°层理煤岩中，裂纹容易形成宏观裂缝，发生断裂；而90°层理煤岩更易吸收能量，形成未贯通的大量细观裂纹。基于能量法的D有效地反映了煤岩体破坏的真实情况和本质变化。
5. 层理煤岩表观破坏形态及致裂机理
5.1 层理煤岩断面粗糙度的变化特征
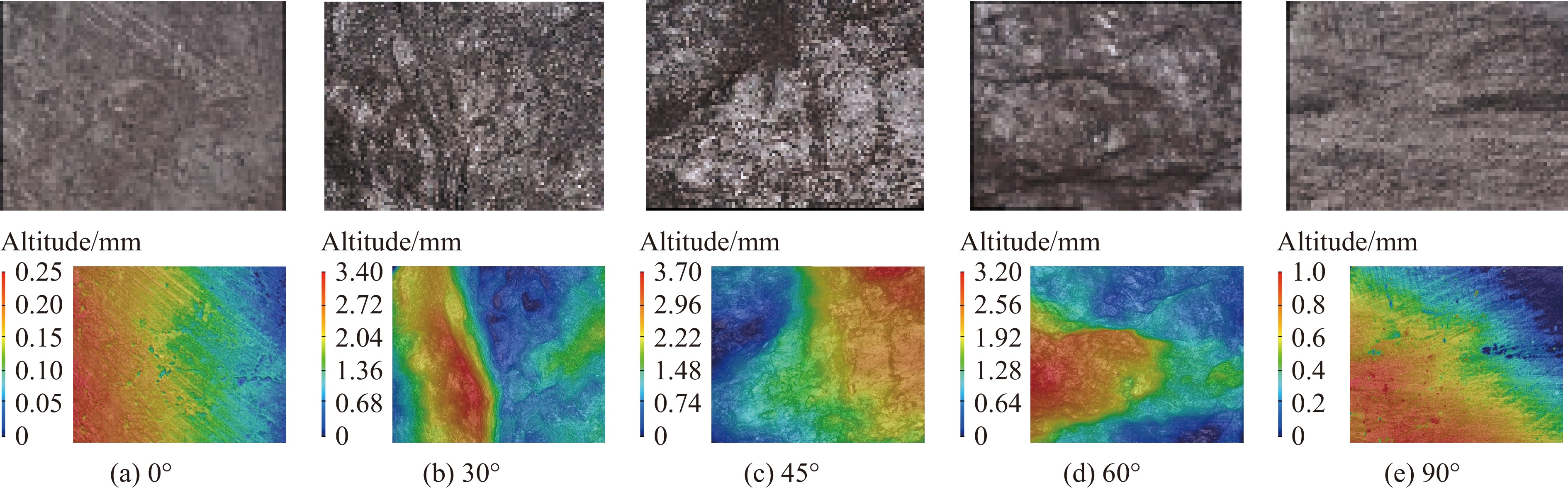
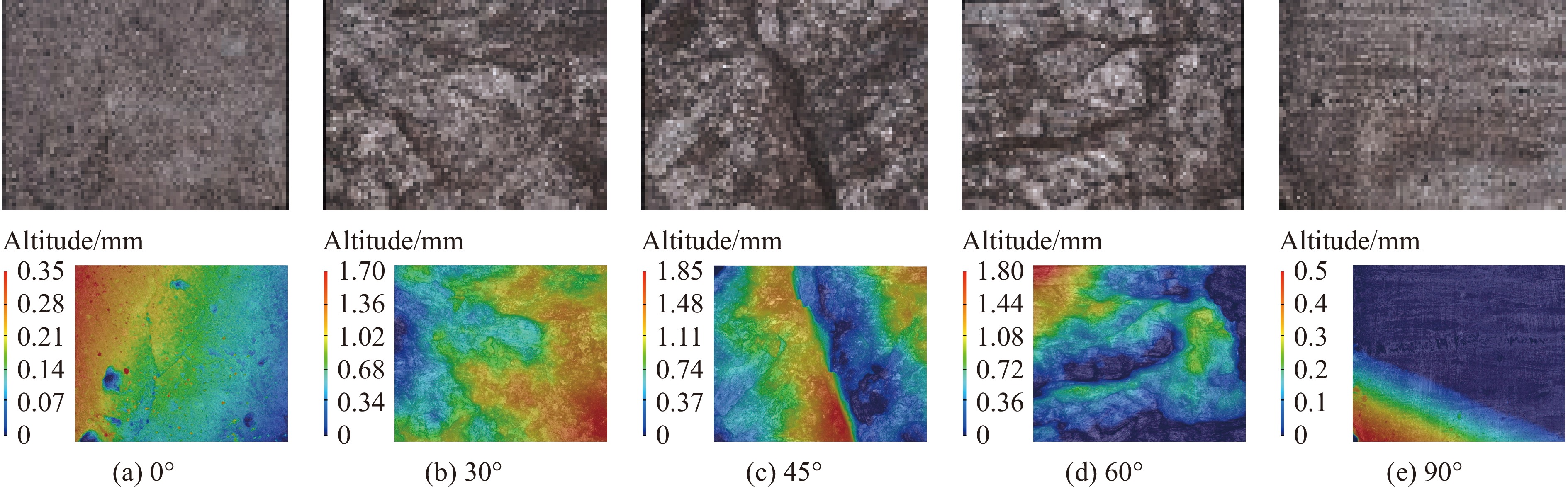
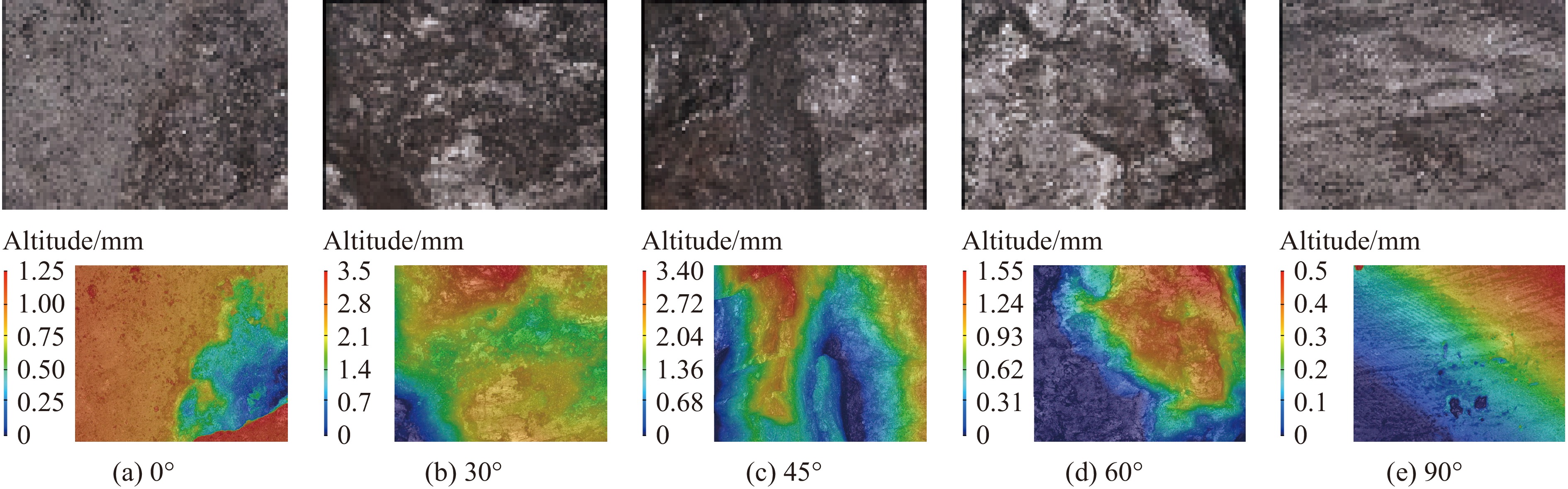
岩石断裂面的形态对揭示岩石动态断裂机制有重大意义，断裂面的粗糙度也常被用于分析岩石裂缝中的剪切和流动行为。为此，选取3D轮廓扫描仪（KEYENCE VR-5000），测量层理煤岩的断裂表面形态特征，以分析动态三轴循环压缩冲击下层理效应对煤岩断裂特性的影响。并结合粗糙度分析模块，采用下式计算三维表面粗糙度Sa，定量分析层理煤岩断裂面粗糙度的变化特征[22]：
Sa=1NMN∑i=1M∑j=1|Zij| (6) 式中：Z为煤岩表面轮廓点到基准平面的距离，N和M分别为测量区域中相互垂直的2个方向上的采集点数。
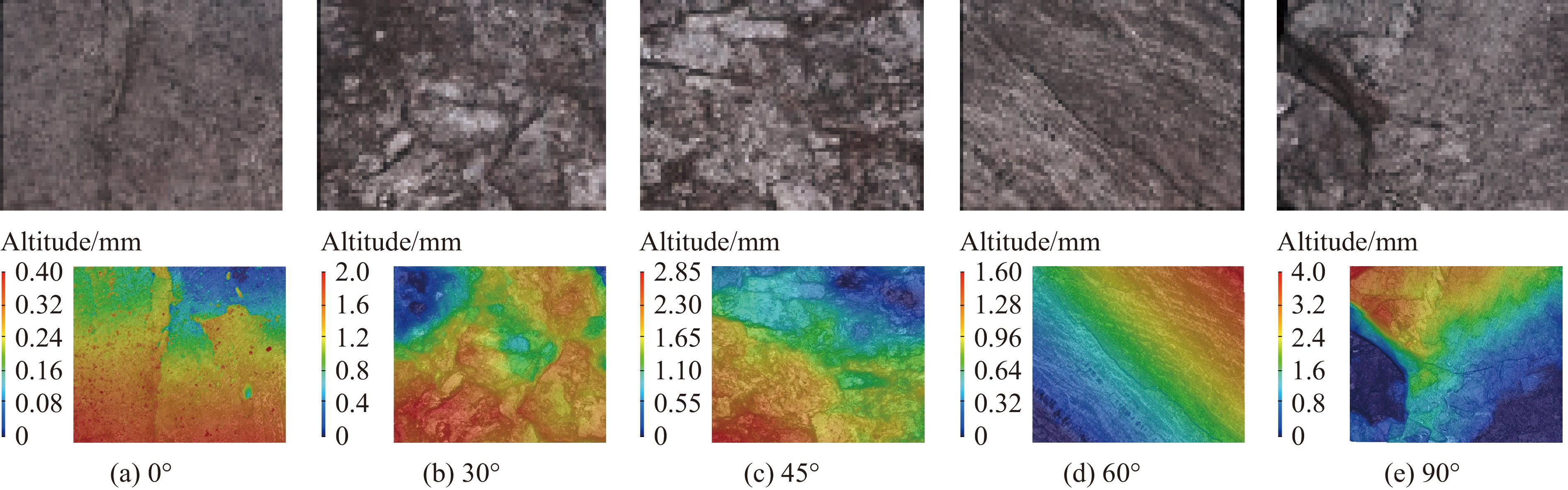
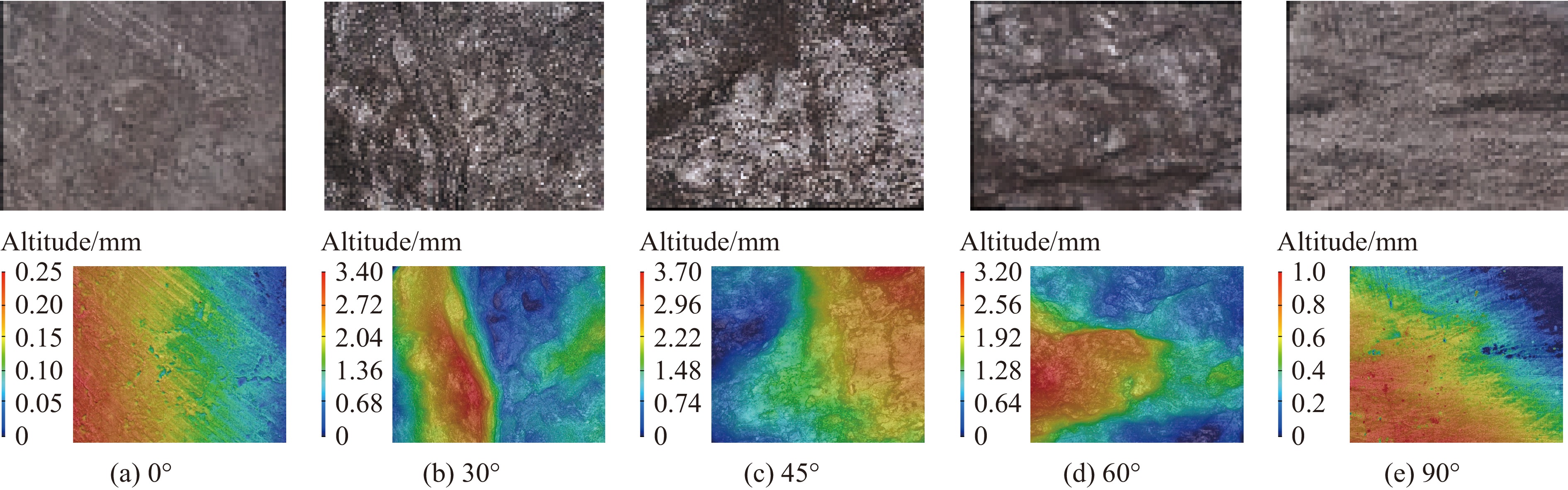
如图14～17所示，具有代表性的煤岩断裂面的初始照片和三维扫描图被分析展示，通过式(7)量化断裂表面得到断面粗糙度Sa。
图 14 在2.5 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图Figure 14. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 2.5 MPa
图 15 在5.0 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图Figure 15. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 5.0 MPa
图 16 在7.5 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图Figure 16. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 7.5 MPa
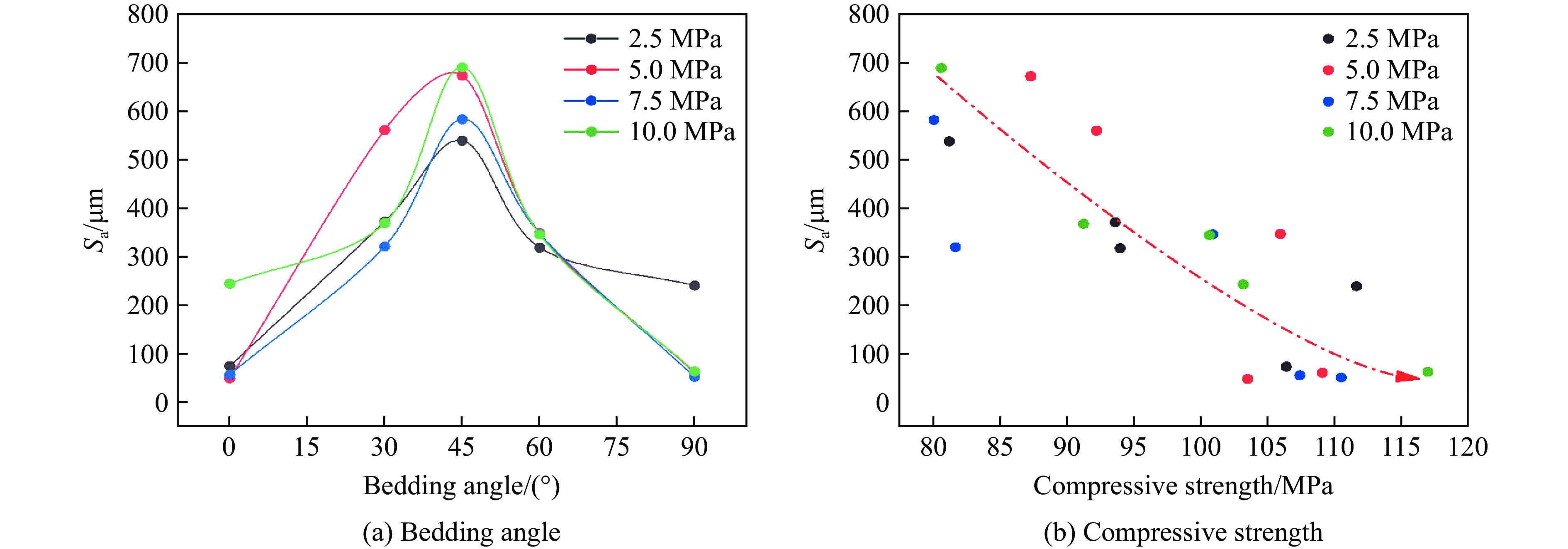
图 17 在10.0 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图Figure 17. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 10.0 MPa如图18(a)所示，Sa与层理角度呈∩形分布，在0°和90°最小，在45°层理角度达到最大。因为煤岩是由大量微米～厘米级晶粒组成的多晶体脆性材料，煤岩易沿着脆性层理面发生沿晶或穿晶断裂，较0°和90°，45°层理面接触薄弱，更易发生沿晶断裂，破坏形式表现为压剪层裂破坏。内部晶粒在动载荷作用下会承受正应力和切应力而在层理断裂面产生冲蚀坑和划痕，0°层理方向与冲击方向垂直，层理表面切削作用最小，正向的冲击作用导致晶粒产生的冲蚀坑最深；90°层理方向与冲击方向平行，更易发生穿晶断裂[35]；45°层理面上的晶粒更易因冲击而反弹，冲蚀坑加深并在层理断裂面产生切削划痕，使断面粗糙度最高。如图18(b)所示，断面粗糙度与抗压强度展现良好的负相关，即抗压强度越高，断面粗糙度越低。
图 18 煤岩断裂面的粗糙度随层理角度和抗压强度的变化Figure 18. Roughness of fracture interface of coal rock varied with bedding angle and compressive strength5.2 煤岩表观破坏形态
如图19所示，0°层理煤岩在循环冲击后表面出现剥落现象，在10 MPa围压时出现张拉破坏。当围压为2.5 MPa时，90°层理煤岩表现出劈裂损伤，但随着围压的增大，煤岩表面则并无明显裂纹，因为2.5 MPa围压不足以压实90°层理煤岩的内部裂隙结构，导致损伤较严重，然而对于90°层理煤岩，围压作用方向垂直于层理角度，围压能更好地保证煤岩内部孔隙的闭合，因此当围压升高时，损伤开始减小，导致90°层理角度煤岩抗压强度最高，破坏程度最低。层理角度为30°、45°、60°的煤岩均沿层理角的角度方向发生压剪破坏，其中，45°层理煤岩破坏形式最严重，出现层裂现象，30°和60°层理煤岩分别在10.0和7.5 MPa围压情况下也有层裂现象出现，煤岩损伤对层理角的响应更敏感，损伤形式主要为沿脆性节理面断裂[36]。
图 19 煤岩在不同围压和层理角度作用下的破坏模式Figure 19. Failure modes of coal rocks with different bedding angles at different confining pressures5.3 致裂机理分析
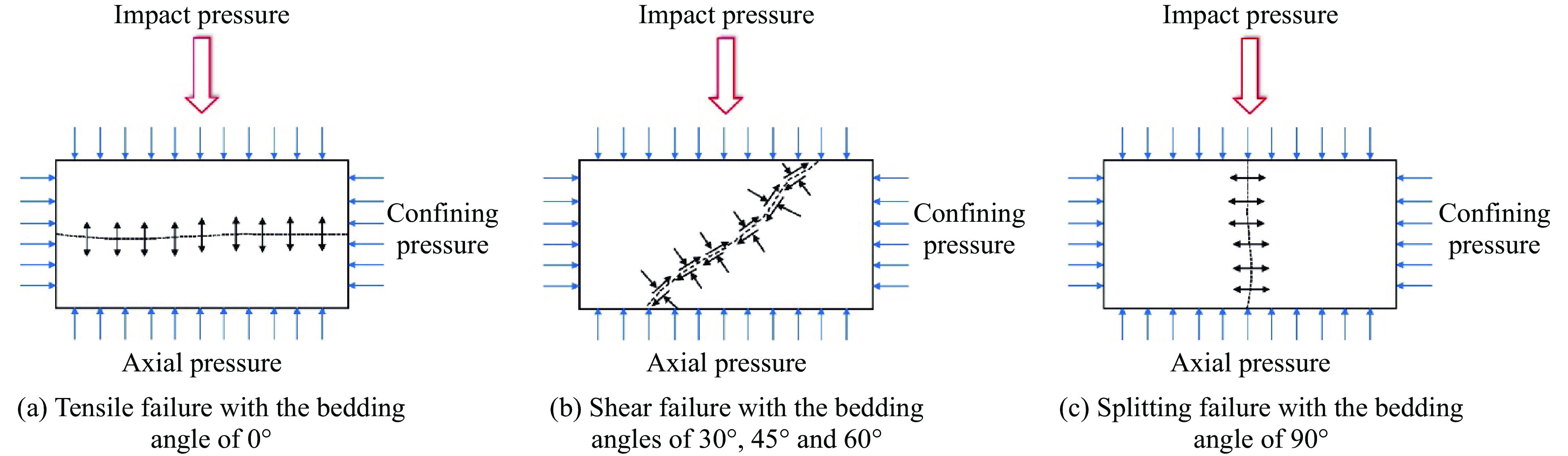
如图20所示，沿不同层理角度的各种损伤形式可归纳为3种，分别为垂直于冲击方向的拉伸损伤、与冲击方向呈夹角的压剪损伤、平行于冲击方向的劈裂损伤。对于0°层理煤岩，图20(a)所示的破坏形式倾向于在高围压状况下发生，这是由于围压足够大的时候，煤岩表面会产生环形裂纹，在围压作用下的弯曲应力导致脆性表面拉伸形成损伤；图20(b)所示的破坏形式最易发生，因为主层理角度极易改变煤岩内部的应力状态，导致破裂面上的摩擦承载能力下降，裂纹扩展并发生扩张和位移，对岩石造成剪切破坏，45°层理煤岩以压剪层裂的破坏形式为主，30°和60°层理煤岩在7.5和10.0 MPa围压下，层裂现象也会出现；对于90°层理煤岩，图20(c)所示的破坏形式倾向于在低围压下发生，这是由于煤岩在低围压下发生轴压变形和侧向膨胀变形，脆性层理面在拉伸作用下被激活开裂，最终沿着冲击方向形成劈裂损伤。因此，煤岩的损伤破坏特征随层理角度的变化可总结为张拉破坏(0°)-剪切破坏（30°、45°、60°）-劈裂破坏（90°）的演变过程。
图 20 煤岩在不同层理角度下的损伤形式Figure 20. Damage modes of coal rocks with different bedding angles6. 结 论
采用
∅ 50 mm SHPB测试系统，对含特征层理（0°、30°、45°、60°、90°）的煤岩进行了动态三轴循环冲击实验，探讨了层理效应对煤岩动态力学参数和能量演化的影响，并结合3D轮廓扫描仪测量断裂表面以分析层理效应对煤岩断裂特性的影响，获得的主要结论如下。(1) 围压的施加促使了煤岩由脆性向延性的转变，应力-应变曲线出现弹性后效现象，抗压强度和失效应变较无围压状态增大3.9～4.2和2.59～3.05倍。施加围压后，煤岩对层理角度的响应更明显，呈现一致的变化趋势。45°层理煤岩的动态抗压强度和弹性模量最低，90°层理煤岩的动态抗压强度和弹性模量最高，0°层理煤岩的动态抗压强度和弹性模量次之
(2) 45°层理能有效地抑制能量的透射，并加速能量的吸收用于裂隙发育，直至发生断裂，导致该煤岩破坏最严重；90°层理更利于能量的透射，并抑制能量的反射。在5 MPa围压之前，0°层理煤岩能量的反射率最高；之后，30°层理煤岩能量的反射率最高。这说明，层理煤岩的能量分配受围压和层理的相互影响，但层理作用仍占主导地位。
(3) 采用3D轮廓扫描仪，对动态三轴循环冲击煤的岩层理面特性进行了表征量化。含特征层理煤岩的断面粗糙度与层理角度呈现∩形分布，在45°层理角度，断面粗糙度达到最大；且断面粗糙度与抗压强度展现良好的负相关，即抗压强度越高，断面粗糙度越低。
(4) 0°层理煤岩在10 MPa围压时出现张拉破坏，90°层理煤岩在2.5 MPa围压时出现劈裂损伤。层理角度为30°、45°、60°的煤岩均沿脆性节理面发生压剪破坏，破坏倾角主要受层理角度的影响，其中45°层理煤岩的破坏最严重，出现压剪层裂破坏。
-
图 5 含不同特征层理煤岩动态测试中的子弹速度和入射能量
Figure 5. Velocity and incident energy of the bullets in dynamical tests on coal rock samples with different bedding angles
图 6 不同围压下45°层理煤岩的应力-应变曲线
Figure 6. Stress-strain curves of 45° bedding coal rock under different confining pressures
图 7 无围压条件下含不同特征层理煤岩试样的动态抗压强度
Figure 7. Dynamic compressive strengths of coal rock samples with different bedding angles without confining pressure
图 8 抗压强度随围压和层理角度的变化
Figure 8. Variation of compressive strength with confining pressure and bedding angle
图 9 不同围压下煤岩弹性模量随层理角度的变化
Figure 9. Variation of elastic modulus of coal rock with bedding angle at different confining pressures
图 10 P波在等效层理面上的反射和透射
Figure 10. Reflection and transmission of P-waves at the equivalent bedding plane
图 11 不同围压下煤岩能量分配率随层理角度的变化
Figure 11. Variation of energy distribution ratio of coal rock with bedding angle at different confining pressures
图 12 体积能量耗散和可释放应变能
Figure 12. Energy dissipation and releasable strain energy per unit volume
图 14 在2.5 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图
Figure 14. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 2.5 MPa
图 15 在5.0 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图
Figure 15. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 5.0 MPa
图 16 在7.5 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图
Figure 16. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 7.5 MPa
图 17 在10.0 MPa围压下不同层理角度煤岩断裂面的初始照片和三维扫描图
Figure 17. Original photographs and 3-D scans of fracture interfaces of coal rocks with bedding angles at the confining pressure of 10.0 MPa
图 18 煤岩断裂面的粗糙度随层理角度和抗压强度的变化
Figure 18. Roughness of fracture interface of coal rock varied with bedding angle and compressive strength
图 19 煤岩在不同围压和层理角度作用下的破坏模式
Figure 19. Failure modes of coal rocks with different bedding angles at different confining pressures
-
[1] 叶建平, 史保生, 张春才. 中国煤储层渗透性及其主要影响因素 [J]. 煤炭学报, 1999, 24(2): 8–12. DOI: 10.3321/j.issn:0253-9993.1999.02.002.YE J P, SHI B S, ZHANG C C. Coal reservoir permeability and its controlled factors in China [J]. Journal of China Coal Society, 1999, 24(2): 8–12. DOI: 10.3321/j.issn:0253-9993.1999.02.002. [2] MOORE T A. Coalbed methane: a review [J]. International Journal of Coal Geology, 2012, 101: 36–81. DOI: 10.1016/j.coal.2012.05.011. [3] HAMAWAND I, YUSAF T, HAMAWAND S G. Coal seam gas and associated water: a review paper [J]. Renewable and Sustainable Energy Reviews, 2013, 22: 550–560. DOI: 10.1016/j.rser.2013.02.030. [4] WANG D K, LV R H, WEI J P, et al. An experimental study of the anisotropic permeability rule of coal containing gas [J]. Journal of Natural Gas Science and Engineering, 2018, 53: 67–73. DOI: 10.1016/j.jngse.2018.02.026. [5] 傅雪海, 秦勇, 张万红, 等. 基于煤层气运移的煤孔隙分形分类及自然分类研究 [J]. 科学通报, 2005, 50(S1): 66–71. DOI: 10.3321/j.issn:0023-074X.2005.z1.009.FU X H, QIN Y, ZHANG W H, et al. Fractal classification and natural classification of coal pore structure based on migration of coal bed methane [J]. Chinese Science Bulletin, 2005, 50(S1): 66–71. DOI: 10.3321/j.issn:0023-074X.2005.z1.009. [6] LI B B, YANG K, XU P, et al. An experimental study on permeability characteristics of coal with slippage and temperature effects [J]. Journal of Petroleum Science and Engineering, 2019, 175: 294–302. DOI: 10.1016/j.petrol.2018.12.048. [7] 吴飞鹏, 刘洪志, 任杨, 等. 燃爆冲击作用下岩石初始破坏区形成机制与主控因素 [J]. 爆炸与冲击, 2016, 36(5): 663–669. DOI: 10.11883/1001-1455(2016)05-0663-07.WU F P, LIU Z H, REN Y, et al. Formation mechanism and main controlling factors of rock’s initial damaged zone under explosive impact effect [J]. Explosion and Shock Waves, 2016, 36(5): 663–669. DOI: 10.11883/1001-1455(2016)05-0663-07. [8] 牟恭雨, 罗宁, 申涛, 等. 聚能射流侵彻页岩储层损伤裂隙形成机制[J]. 爆炸与冲击, 2023, 43(3): 033301. DOI: 10.11883/bzycj-2022-0182.MU G Y, LUO N, SHEN T, et al. Mechanism of damage-induced fracture formation in shale reservoir penetrated by shaped charge jet [J]. Explosion and Shock Waves, 2023, 43(3): 033301. DOI: 10.11883/bzycj-2022-0182. [9] RAMULU M, CHAKRABORTY A K, SITHARAM T G. Damage assessment of basaltic rock mass due to repeated blasting in a railway tunnelling project-A case study [J]. Tunnelling and Underground Space Technology, 2009, 24(2): 208–221. DOI: 10.1016/j.tust.2008.08.002. [10] LI H B, XIA X, LI J C, et al. Rock damage control in bedrock blasting excavation for a nuclear power plant [J]. International Journal of Rock Mechanics and Mining Sciences, 2011, 48(2): 210–218. DOI: 10.1016/j.ijrmms.2010.11.016. [11] 谢和平. 深部岩体力学与开采理论研究进展 [J]. 煤炭学报, 2019, 44(5): 1283–1305. DOI: 10.13225/j.cnki.jccs.2019.6038.XIE H P. Research review of the state key research development program of China: deep rock mechanics and mining theory [J]. Journal of China Coal Society, 2019, 44(5): 1283–1305. DOI: 10.13225/j.cnki.jccs.2019.6038. [12] 鞠杨, 李业学, 谢和平, 等. 节理岩石的应力波动与能量耗散 [J]. 岩石力学与工程学报, 2006, 25(12): 2426–2434. DOI: 10.3321/j.issn:1000-6915.2006.12.007.JU Y, LI Y X, XIE H P, et al. Stress wave propagation and energy dissipation in jointed rocks [J]. Chinese Journal of Rock Mechanics and Engineering, 2006, 25(12): 2426–2434. DOI: 10.3321/j.issn:1000-6915.2006.12.007. [13] MA Y, PAN Z J, ZHONG N N, et al. Experimental study of anisotropic gas permeability and its relationship with fracture structure of Longmaxi Shales, Sichuan Basin, China [J]. Fuel, 2016, 180: 106–115. DOI: 10.1016/j.fuel.2016.04.029. [14] TAN Y L, PAN Z J, LIU J S, et al. Experimental study of permeability and its anisotropy for shale fracture supported with proppant [J]. Journal of Natural Gas Science and Engineering, 2017, 44: 250–264. DOI: 10.1016/j.jngse.2017.04.020. [15] ZHAO Y X, ZHAO G F, JIANG Y D, et al. Effects of bedding on the dynamic indirect tensile strength of coal: laboratory experiments and numerical simulation [J]. International Journal of Coal Geology, 2014, 132: 81–93. DOI: 10.1016/j.coal.2014.08.007. [16] KONG X G, WANG E Y, LI S G, et al. Dynamic mechanical characteristics and fracture mechanism of gas-bearing coal based on SHPB experiments [J]. Theoretical and Applied Fracture Mechanics, 2020, 105: 102395. DOI: 10.1016/j.tafmec.2019.102395. [17] HAO X J, DU W S, ZHAO Y X, et al. Dynamic tensile behaviour and crack propagation of coal under coupled static-dynamic loading [J]. International Journal of Mining Science and Technology, 2020, 30(5): 659–668. DOI: 10.1016/j.ijmst.2020.06.007. [18] LIU X H, DAI F, ZHANG R, et al. Static and dynamic uniaxial compression tests on coal rock considering the bedding directivity [J]. Environmental Earth Sciences, 2015, 73(10): 5933–5949. DOI: 10.1007/s12665-015-4106-3. [19] CHEN Y L, ZHANG Y N, LI X L. Experimental study on influence of bedding angle on gas permeability in coal [J]. Journal of Petroleum Science and Engineering, 2019, 179: 173–179. DOI: 10.1016/j.petrol.2019.04.010. [20] ZHAO Y X, GONG S, HAO X J, et al. Effects of loading rate and bedding on the dynamic fracture toughness of coal: laboratory experiments [J]. Engineering Fracture Mechanics, 2017, 178: 375–391. DOI: 10.1016/j.engfracmech.2017.03.011. [21] WANG W, ZHAO Y X, TENG T, et al. Influence of bedding planes on mode Ⅰ and mixed-mode (Ⅰ-Ⅱ) dynamic fracture toughness of coal: analysis of experiments [J]. Rock Mechanics and Rock Engineering, 2021, 54(1): 173–189. DOI: 10.1007/s00603-020-02250-9. [22] FAN X R, LUO N, LIANG H L, et al. Dynamic breakage characteristics of shale with different bedding angles under the different ambient temperatures [J]. Rock Mechanics and Rock Engineering, 2021, 54(6): 3245–3261. DOI: 10.1007/s00603-021-02463-6. [23] 王卫华, 李夕兵, 左宇军. 非线性法向变形节理对弹性纵波传播的影响 [J]. 岩石力学与工程学报, 2006, 25(6): 1218–1225. DOI: 10.3321/j.issn:1000-6915.2006.06.020.WANG W H, LI X B, ZUO Y J. Effects of single joint with nonlinear normal deformation on P-wave propagation [J]. Chinese Journal of Rock Mechanics and Engineering, 2006, 25(6): 1218–1225. DOI: 10.3321/j.issn:1000-6915.2006.06.020. [24] 李业学, 谢和平, 朱哲明, 等. 应力波穿越分形节理时的透反射规律研究 [J]. 岩石力学与工程学报, 2009, 28(1): 120–129. DOI: 10.3321/j.issn:1000-6915.2009.01.016.LI Y X, XIE H P, ZHU Z M, et al. Study on rules of transmission and reflection of stress wave across fractal joint [J]. Chinese Journal of Rock Mechanics and Engineering, 2009, 28(1): 120–129. DOI: 10.3321/j.issn:1000-6915.2009.01.016. [25] 许金余, 范建设, 吕晓聪. 围压条件下岩石的动态力学特性 [M]. 西安: 西北工业大学出版社, 2012: 21–25.XU J Y, FAN J S, LYU X C. Dynamic mechanical properties of rock with the confining pressure [M]. Xi’an: Northwestern Polytechnical University Press, 2012: 21–25. [26] LEWANDOWSKI C M, COINVESTIGATOR N. Split Hopkinson (Kolsky) bar: design, testing and applications [M]. Springer, 2015: 6–12. [27] FAKHIMI A, AZHDARI P, KIMBERLEY J. Physical and numerical evaluation of rock strength in Split Hopkinson Pressure Bar testing [J]. Computers and Geotechnics, 2018, 102: 1–11. DOI: 10.1016/j.compgeo.2018.05.009. [28] FENG J J, WANG E Y, CHEN X, et al. Energy dissipation rate: an indicator of coal deformation and failure under static and dynamic compressive loads [J]. International Journal of Mining Science and Technology, 2018, 28(3): 397–406. DOI: 10.1016/j.ijmst.2017.11.006. [29] LUO N, SUO Y C, FAN X R, et al. Research on confining pressure effect of pore structure of coal-rich in coalbed methane under cyclic impact [J]. Energy Reports, 2022, 8: 7336–7348. DOI: 10.1016/j.egyr.2022.05.238. [30] SUO Y C, LUO N, CHAI Y B, et al. Experimental investigation of dynamic mechanical characteristics of inhomogeneous composite coal-sandstone combination for coalbed methane development [J]. Heliyon, 2022, 8: e11628. DOI: 10.1016/j.heliyon.2022.e11628. [31] 谢和平, 彭瑞东, 鞠杨, 等. 岩石破坏的能量分析初探 [J]. 岩石力学与工程学报, 2005, 24(15): 2603–2608. DOI: 10.3321/j.issn:1000-6915.2005.15.001.XIE H P, PENG R D, JU Y, et al. On energy analysis of rock failure [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(15): 2603–2608. DOI: 10.3321/j.issn:1000-6915.2005.15.001. [32] 谢和平, 鞠杨, 黎立云. 基于能量耗散与释放原理的岩石强度与整体破坏准则 [J]. 岩石力学与工程学报, 2005, 24(17): 3003–3010. DOI: 10.3321/j.issn:1000-6915.2005.17.001.XIE H P, JU, Y, LI L Y. Criteria for strength and structural failure of rocks based on energy dissipation and energy release principles [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(17): 3003–3010. DOI: 10.3321/j.issn:1000-6915.2005.17.001. [33] 黎立云, 徐志强, 谢和平, 等. 不同冲击速度下岩石破坏能量规律的实验研究 [J]. 煤炭学报, 2011, 36(12): 2007–2011. DOI: 10.13225/j.cnki.jccs.2011.12.012.LI L Y, XU Z Q, XIE H P, et al. Failure experimental study on energy laws of rock under differential dynamic impact velocities [J]. Journal of China Coal Society, 2011, 36(12): 2007–2011. DOI: 10.13225/j.cnki.jccs.2011.12.012. [34] 刘晓辉, 薛洋, 周济芳, 等. 层理煤岩动态破坏能量变化规律及损伤特征 [J]. 地下空间与工程学报, 2021, 17(4): 1052–1062.LIU X H, XUE Y, ZHOU J F, et al. Dynamic failure energy change and damage characteristics of bedding coal rock [J]. Chinese Journal of Underground Space and Engineering, 2021, 17(4): 1052–1062. [35] 周磊, 姜亚成, 朱哲明, 等. 动载荷作用下裂隙岩体的止裂机理分析 [J]. 爆炸与冲击, 2021, 41(5): 053102. DOI: 10.11883/bzycj-2020-0125.ZHOU L, JIANG Y C, ZHU Z M, et al. Mechanism study of preventing crack propagation of fractured rock under dynamic loads [J]. Explosion and Shock Waves, 2021, 41(5): 053102. DOI: 10.11883/bzycj-2020-0125. [36] 杨国梁, 毕京九, 郭伟民, 等. 加载角度对层理页岩裂纹扩展影响的实验研究 [J]. 爆炸与冲击, 2021, 41(9): 093101. DOI: 10.11883/bzycj-2021-0097.YANG G L, BI J J, GUO W M, et al. Experimental study on the effect of loading angle on crack propagation in bedding shale [J]. Explosion and Shock Waves, 2021, 41(9): 093101. DOI: 10.11883/bzycj-2021-0097. 期刊类型引用(11)
1. 马衍坤，黄勤豪，刘泽伟，柴虎峰，殷志强，王超，吴昌，潘科举. 基于NMR震动载荷作用下含层理煤样损伤演化特征. 采矿与安全工程学报. 2025(01): 126-136 . 
百度学术2. 马衍坤，黄勤豪，孔祥国，冯俊军，殷志强，王超. 震动载荷多次作用下烟煤孔裂隙结构演化特征试验研究. 煤炭学报. 2024(04): 1882-1893 . 百度学术3. 王汉鹏，王伟，张冰，邱廷麟，侯夫斌. 循环冲击下预加静载岩石损伤破坏机制. 煤炭学报. 2024(04): 1860-1872 . 百度学术4. 马智会，马智勇，潘荣锟，朱长远，张春光，张露. 三维静载与循环冲击共同作用下组合煤岩体力学特性试验研究. 中国安全生产科学技术. 2024(08): 58-67 . 百度学术5. 张蓉蓉，沈永辉，马冬冬，平琦，杨毅. 循环冲击作用下冻融红砂岩动力学特性与损伤机理. 爆炸与冲击. 2024(08): 133-148 . 
本站查看6. 葛兆龙，马世榃，周哲，张旭，常文旭. 真三轴应力下水射流冲击不同层理面倾角煤的破坏机制. 振动与冲击. 2024(22): 10-19 . 百度学术7. 李鹏龙，罗宁，索云琛，柴亚博，孙锐. 力-热耦合作用下煤岩动力学特性和破碎机理研究. 爆破. 2024(04): 8-17+60 . 百度学术8. 陶瑞律，薛生，李炜煜，李光明. 三维静载作用下含层理煤动态力学特性研究. 安全. 2024(12): 73-81 . 百度学术9. 叶海旺，钱正昆，雷涛，温颖，李睿. 冲击载荷下石墨矿石动力学特性的层理效应及宏微观破坏机理. 爆炸与冲击. 2023(12): 27-39 . 本站查看10. 宋战平，刘洪珂，郑方，程昀，孙引浩，宋婉雪. 考虑层理倾角的硬质砂岩力学行为及破裂响应特征. 煤田地质与勘探. 2023(12): 167-175 . 百度学术11. 余旭，石克龙，王宇，刘厅，唐伟. 燃爆载荷冲击下五峰—龙马溪组页岩断裂韧性及破坏模式. 煤炭学报. 2023(12): 4322-4335 . 百度学术其他类型引用(5)
-
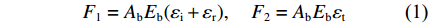
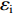
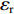
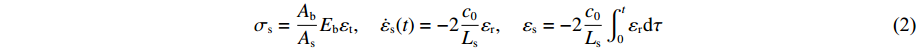
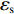
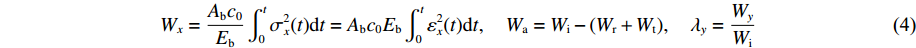
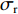
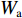
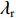
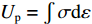
下载:
下载:
百度学术
计量
- 文章访问数: 646
- HTML全文浏览量: 160
- PDF下载量: 124
- 被引次数: 16