基于分数阶模型的牡蛎壳动力学特性研究

袁良柱; 陆建华; 苗春贺; 王鹏飞; 徐松林

doi:10.11883/bzycj-2022-0318

基于分数阶模型的牡蛎壳动力学特性研究

doi: 10.11883/bzycj-2022-0318

袁良柱^1,,
陆建华¹,
苗春贺¹,
王鹏飞¹,
徐松林^{1, 2, ,}

1.
中国科学技术大学中国科学院材料力学行为和设计重点实验室，安徽合肥 230027
2.
中国地震局地震预测研究所高压物理与地震科技联合实验室，北京 100036

基金项目: 国家自然科学基金（11672286, 11872361）；中央高校基本科研业务费专项资金（WK2480000008）；中石油与中科院重大战略合作项目（2015A-4812）；高压物理与地震科技联合实验室室开放基金（2019HPPES01）

详细信息

作者简介:
袁良柱（1998－　），男，博士研究生，ylzustcedu@mail.ustc.edu.cn

通讯作者:
徐松林（1971－　），男，博士，研究员，博士生导师，slxu99@ustc.edu.cn

中图分类号: O347
计量
- 文章访问数: 548
- HTML全文浏览量: 178
- PDF下载量: 98
- 被引次数: 3
出版历程
- 收稿日期: 2022-07-21
- 修回日期: 2022-10-11
- 网络出版日期: 2022-11-21
- 刊出日期: 2023-01-05

Dynamic properties of oyster shells based on a fractional-order model

1.
CAS Key Laboratory for Mechanical Behavior and Design of Materials, University of Science and Technology of China, Hefei 230027, Anhui, China
2.
United Laboratory of High-Pressure Physics and Earthquake Science, Institute of Earthquake Forecasting, China Earthquake Administration, Beijing 100036, China

摘要

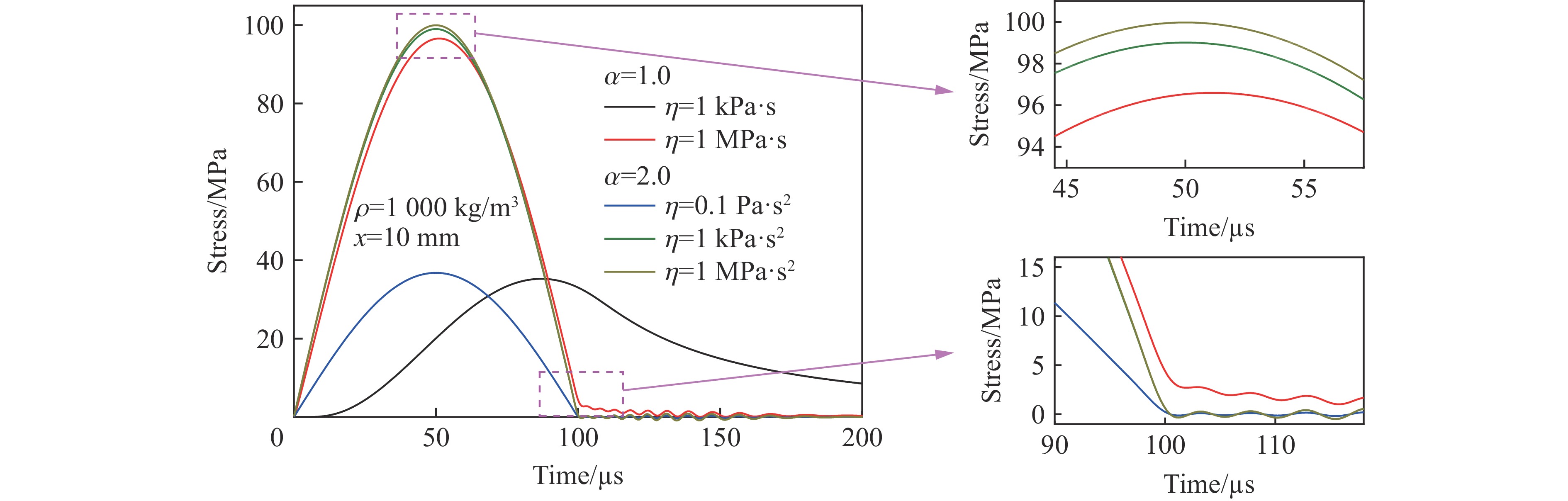
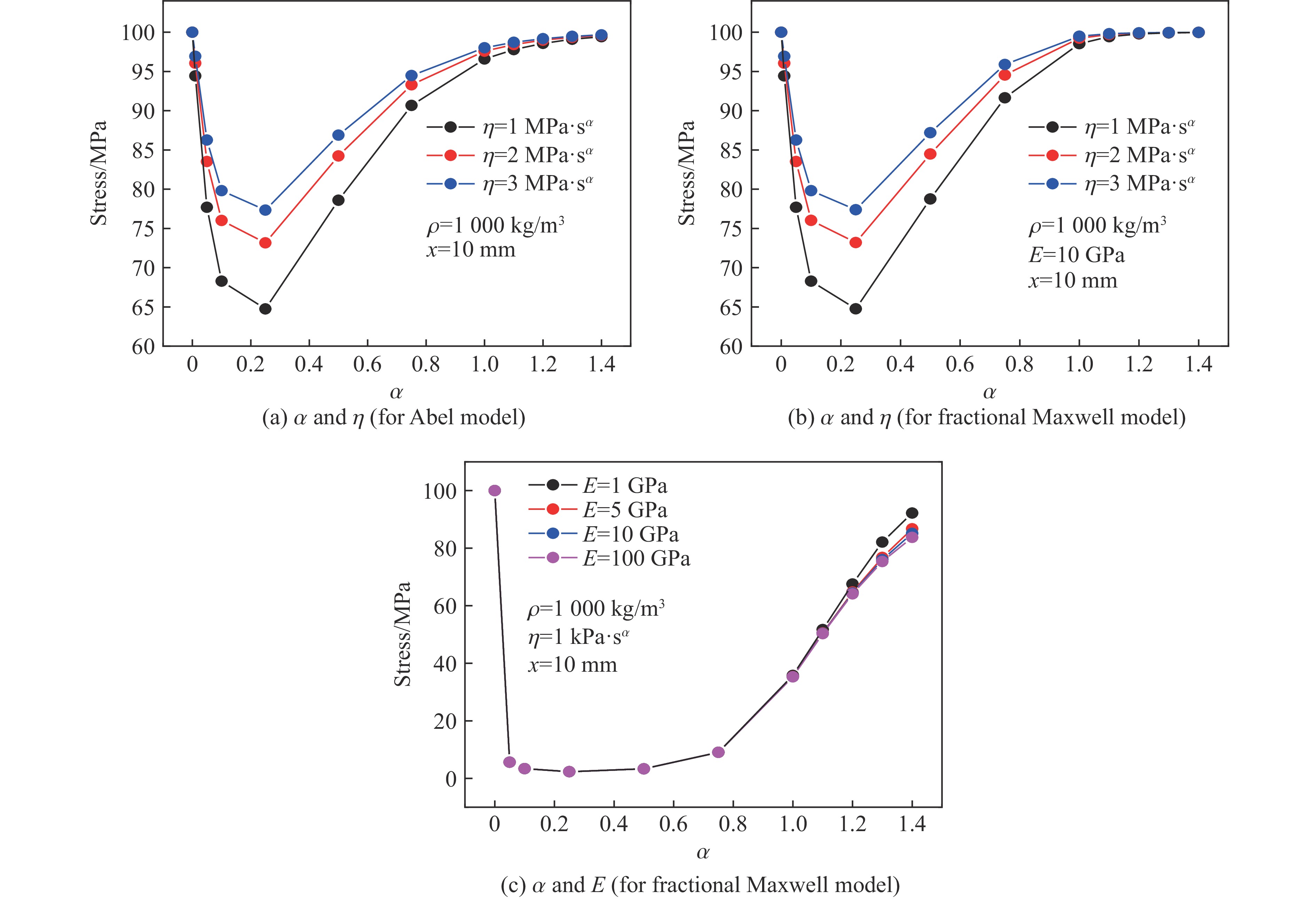
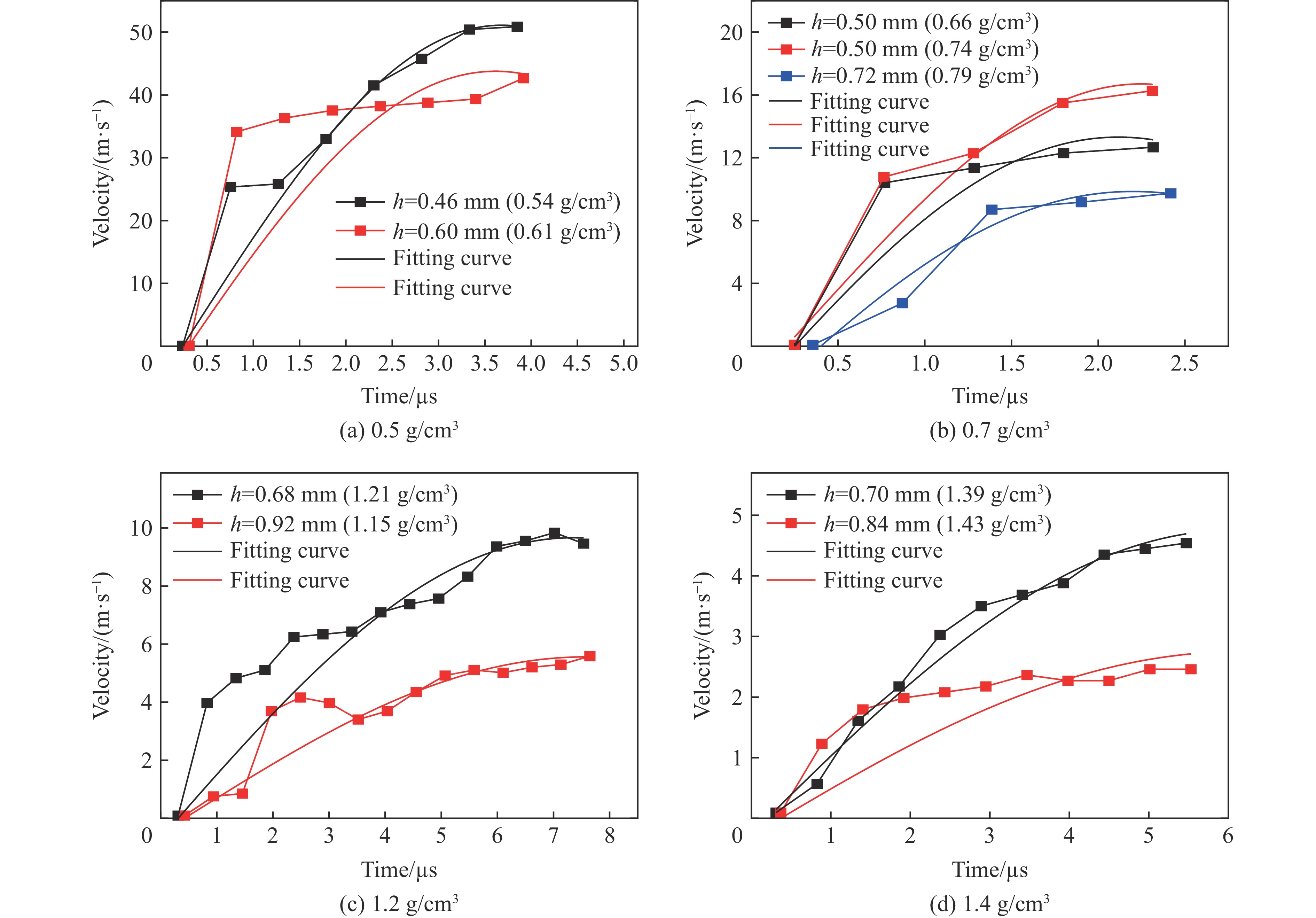
摘要: 贝壳、牡蛎等天然材料因其轻质高强的力学特性在材料设计等领域受到了广泛的关注，但由于材料本身结构的复杂性，对其力学行为的研究十分困难。近年来，分数阶模型在研究材料的力学特性上取得了成功，相比传统模型，分数阶模型可以更好地表征复杂介质的应力或应变与时间的关系。因此，本文从波传播理论出发，以分数阶模型作为材料本构，得到了复杂介质的波传播控制方程。通过Laplace变换得到了控制方程的解析解，并通过Laplace数值逆变换分析了波的衰减对分数阶模型中参量的敏感性，讨论了不同于材料弹性、黏性的材料“惯性”特性。接着，基于解析解和多种实验测试信号，给出了得到分数阶模型参数的拟合式子。以牡蛎材料作为研究对象，利用CO₂脉冲激光器进行小试样的冲击加载、应用两点激光干涉测速系统（laser interferometer velocimetry system, VISAR）对表面粒子的速度进行测量，得到了4种密度下不同厚度的牡蛎壳试样的粒子速度时程曲线，再结合上述理论方法分析得到了牡蛎壳试样的Abel模型和分数阶Maxwell模型的参数，模型参数反映了牡蛎壳试样的细微观结构特征。结果发现：牡蛎壳试样的密度越大，即在细微观上具有砖泥结构的珍珠层的占比越高，速度衰减越大，试样的黏性越大；这是由于CO₂激光脉冲器发射的激光波长与牡蛎壳试样珍珠层的砖泥结构间的缝隙尺寸相近，使得激光在冲击牡蛎壳试样中的珍珠层时发生较大的散射。
- 分数阶导数 /
- 本构模型 /
- 牡蛎壳 /
- 脉冲激光 /
- 动力学特性
Abstract: Natural materials such as shells and oysters have attracted extensive attention in the field of material design due to their lightweight and high-strength mechanical properties. However, due to the complex structure of shells, it is very difficult to study their mechanical behavior. In recent years, fractional-order models have been successful in studying the mechanical properties of materials. Compared with the traditional constitutive model, the fractional model can better characterize the relationship between the complex media’s stress or strain and time. Therefore, based on wave propagation theory and by using the time-dependent fractional-order model as the material constitutive model, the complex medium is simplified to the uniform medium, and its governing equation is obtained by then. The analytic solution of the governing equation which is a function of space coordinate x and Laplace variable s is obtained by the Laplace transform. It is hard to obtain the analytical solution of space coordinate x and time t directly through the inverse Laplace transform, so the numerical inverse Laplace transform is used to obtain the numerical solution of the governing equation in the time domain. The sensitivity of wave attenuation to parameters in the fractional model is analyzed. The inertial properties, which are different from the elastic and viscous properties of materials, are also discussed by analyzing the attenuation characteristics of stress waves when the order α is 0, 1.0, and 2.0 respectively. Then, based on the analytical solution of the governing equation and a variety of experimental test signals, a fitting formula is given to obtain the parameters of the fractional model. Oyster material with layered structure is taken as the research object. To obtain the local dynamic mechanical properties of oyster samples, the CO₂ pulse laser was used to carry out the impact loading of the small sample due to the high variability of the density distribution of oyster samples, and the two-point laser interferometer velocimetry system (VISAR) was used to measure the surface particle velocity. The particle velocity time history curve of the oyster sample with different densities and thicknesses was obtained. Combined with the above fitting formula, the parameters of the Abel model and Maxwell fractional differential model of oyster samples were obtained by fixing and unfixing the values of fractional order α, and the model parameters reflected the fine microstructure characteristics of oyster samples. The results show that the higher the density of the oyster sample is, the higher the proportion of nacre with brick and mortar structure in fine and micro, the greater the velocity attenuation, and the greater the viscosity of the oyster sample. The laser wavelength emitted by the CO₂ laser pulse is similar to the size of the gap between brick and mortar structures in the nacre of the oyster sample, so the laser has a large scattering when it impacts the nacre of the oyster sample, which causes the velocity attenuation. This study has a good reference significance for the study of the dynamic properties of meso-isomeric and macro-continuous complex media.
- fractional derivative /
- constitutive model /
- oyster shell /
- pulse laser /
- dynamic properties

HTML全文

CL-20炸药爆速快，爆压高，但爆轰反应时间短，这给观测其爆轰波结构带来困难。采用激光干涉法观测炸药与窗口的界面粒子速度，是分析炸药爆轰波结构的主要方法之一。该方法把界面粒子速度随时间的变化与ZND爆轰模型中的压力分布假设相对应，将速度曲线中出现的速率变化折点看作爆轰波结构中的CJ点，从而得出爆轰反应结束时间，确定出爆轰反应区宽度。W.L.Seitz等^[1]采用Fabry-perot激光速度干涉仪，分别测量TATB炸药与氟化锂和有机玻璃界面的粒子速度，结合数值模拟分析TATB爆轰波结构。S.A.Sheffield等^[2]采用光学记录速度干涉仪获得了炸药与水界面粒子速度在500 ns之内的变化。韩勇等^[3]采用激光位移干涉仪，测量了PETN和TNT炸药与有机玻璃界面粒子速度，通过计算爆轰产物等熵线与有机玻璃雨贡纽曲线交点的粒子速度，找到实验测量粒子速度曲线上的对应值，确定炸药到达CJ点的时间。

目前，在激光干涉测量中，数据点的采样时间间隔大多在2 ns到10 ns之间，可以满足绝大部分炸药的测量需求。如果依赖于直接观察法^[4]，即从曲线上直接读取速度变化拐点，并定义为CJ状态点，可分析炸药的爆轰波特性。然而，对于反应时间小于100 ns的高能炸药，如CL-20、HMX等，从上述采样频率下获得的粒子速度曲线上直接判断爆轰CJ点会有一定困难。B.G.Loboiko等^[5]提出了先对速度-时间曲线求导，再对获得的速度导数曲线进行分段线性拟合的方法，将粒子速度变化趋势通过2个不同的函数表示，认为2个函数的交点就是CJ点。

本文中，通过爆轰数值模拟，分析CL-20混合炸药的爆轰反应特征，设计炸药与窗口的界面粒子速度测量实验装置。采用激光位移干涉仪，测量C-1炸药(CL-20/粘合剂/94/6)与窗口的界面粒子速度随时间的变化。用先求导、再分段拟合的方法，确定界面粒子速度-时间曲线上CJ点对应的时间位置；基于ZND爆轰模型，分析C-1炸药的爆轰反应区宽度和爆轰压力。

1. 实验装置设计

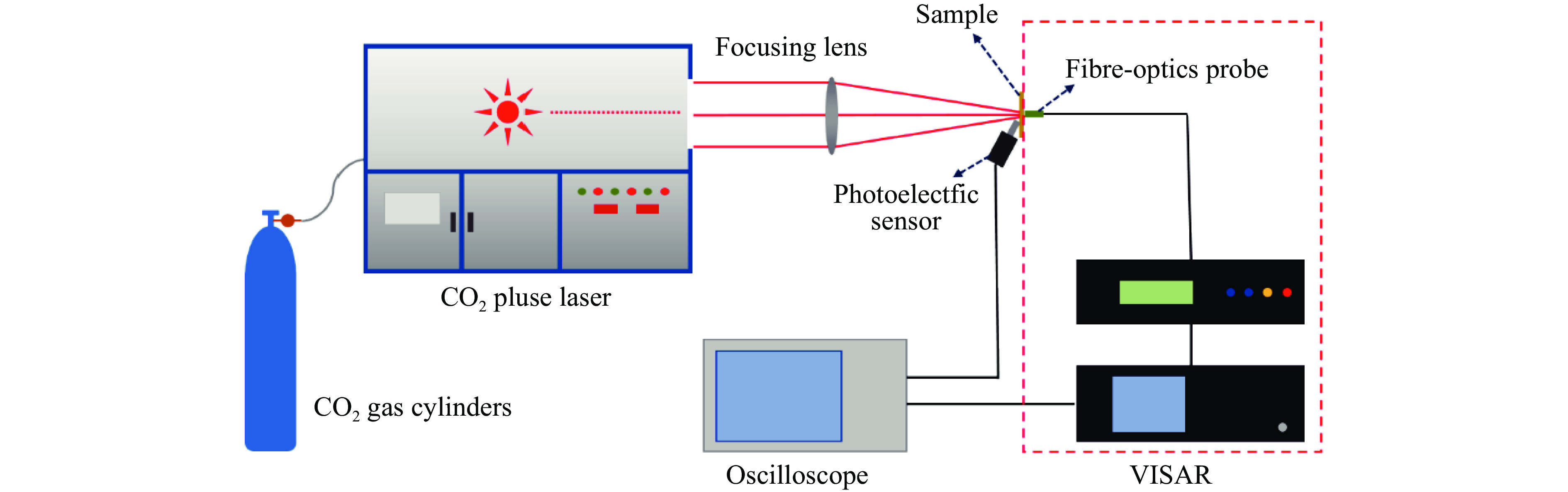
测量炸药与窗口界面粒子速度的实验装置如图 1所示，由雷管、加载炸药、被测炸药和透明窗口等组成。透明窗口与炸药的接触面镀有一层金属薄膜，用于反射激光信号。实验中，首先由雷管起爆加载炸药，加载炸药爆炸引爆被测炸药。采用激光位移干涉仪，记录被测炸药与测试窗口的界面粒子速度。激光探头发出激光束，透过透明窗口照射于炸药与窗口界面的中心位置。通过接收金属薄膜反射的激光，获得金属薄膜速度变化信息。由于金属薄膜很薄，可以认为金属薄膜速度与炸药粒子速度一致。实验时在加载炸药和被测炸药之间放置一个电离探针，用于给出激光位移干涉仪启动信号。

图 1 实验装置示意图

Figure 1. Schematic diagram of experimental setup

下载: 全尺寸图片幻灯片

在实验中需要选择合适的炸药尺寸，确保爆轰波在到达测量点时已成长为稳定爆轰波，并且不受稀疏波影响。为此，采用LS-DYNA有限元分析软件对炸药爆轰进行数值模拟，对实验装置中的炸药尺寸进行设计。根据实验装置的结构特征，建立了二维轴对称计算模型。模型由加载炸药柱、被测炸药柱及透明窗口组成。把加载炸药上表面中心点设为起爆点，代替雷管。采用高能炸药材料模型和JWL状态方程描述加载炸药，采用弹塑性流体力学材料模型和Grüneisen状态方程描述窗口材料^[6]。采用点火增长反应速率方程和JWL状态方程描述CL-20混合炸药。点火增长反应速率方程为^[7]：

$\frac{{{\rm{d}}\lambda }}{{{\rm{d}}t}} = I{(1 - \lambda )^b}{\left( {\frac{\rho }{{{\rho _0}}} - 1 - a} \right)^x} + {G_1}{(1 - \lambda )^c}{\lambda ^d}{p^y} + {G_2}{(1 - \lambda )^e}{\lambda ^g}{p^z}\$

(1)

式中:λ为炸药反应度，t为时间，ρ为密度，ρ₀为初始密度，p为压力，I、G₁、G₂、a、b、x、c、d、y、e、g和z为常数。CL-20混合炸药的方程参数选用LX-19炸药(CL-20/Estane/95.8/4.2)的方程参数^[8]。

当加载炸药为JO-9159(HMX/粘合剂/95/5)，其药柱尺寸为Ø20 mm×20 mm，被测炸药柱尺寸为Ø20 mm×20 mm，窗口材料尺寸为Ø20 mm×10 mm时，图 2为计算得到的距离起爆面不同距离下LX-19炸药内部的压力变化。从图 2可看出，炸药内部在距离起爆面3 mm处已成长为稳定爆轰波。图 3为计算得到的在爆轰波到达与窗口界面时炸药轴向剖面的压力分布。由图 3可看出，爆轰波在以中心测量点为圆心、半径为4 mm的圆面范围内未受到稀疏波的影响，而照射到测量点的激光束直径小于0.5 mm。因此，在上述炸药尺寸条件下，实验装置能够满足实验要求。

图 2 炸药内部压力历史随轴向距离的变化

Figure 2. Internal pressure history of explosive varied with axial distances

下载: 全尺寸图片幻灯片

图 3 炸药轴向剖面的压力分布

Figure 3. Pressure distribution of explosive on axial section

下载: 全尺寸图片幻灯片

根据计算结果开展实验，其中1发实验的照片如图 4所示。实验中，加载炸药JO-9159的密度为1.78 g/cm³；被测炸药为C-1炸药(CL-20/粘合剂/94/6)，共进行了2发实验，第1发实验中被测炸药密度为1.943 g/cm³，第2发实验中被测炸药密度为1.940 g/cm³；测试窗口材料为LiF(氟化锂)，密度为2.63 g/cm³；金属薄膜为铝膜，厚度为0.6 μm。激光位移干涉仪的激光波长为1 550 nm。实验结果经处理后，时间分辨率为5 ns。

图 4 实验装置照片

Figure 4. Photo of experimental setup

下载: 全尺寸图片幻灯片

2. 实验结果与分析

在爆炸冲击波作用下，LiF窗口的折射率发生改变，使得激光速度干涉仪测得的界面粒子速度u_a与界面粒子速度的真实值u_p存在一定差异^[9]，但在一定压力范围内，可认为两者是线性关系，在激光波长为1 550 nm时, 该关系式为^[10]：

${u_{\rm{p}}} = {u_{\rm{a}}}/1.2678$

(2)

图 5为经过式(2)修正及数据平滑处理后的C-1炸药与LiF窗口的界面粒子速度曲线。从图 5可以看出，第2发实验仅记录到200 ns的有效信号，但在记录的时间内，2条曲线的数值和变化趋势几乎相同，说明实验有较好的重复性。本文中主要对第1发实验结果进行分析，从图中可以看出，炸药爆轰波到达LiF窗口界面时，粒子速度先是瞬间达到最大值，然后在很短时间内快速下降，最后在较长时间内以相对平缓的速度下降。在ZND模型中，爆轰波由前导冲击波和紧跟在后面的化学反应区构成：由于前导冲击波的作用，粒子速度会在瞬间达到最大值；之后，炸药发生化学反应，粒子速度快速下降；当炸药反应结束时，到达爆轰CJ点，进入爆轰产物等熵膨胀阶段，粒子速度相对缓慢下降。因此，在分析炸药与窗口界面粒子速度曲线时，确定CJ点位置是分析炸药爆轰波结构的关键。

图 5 C-1炸药与窗口的界面粒子速度

Figure 5. Particle velocity at C-1-LiF interface varying with time

下载: 全尺寸图片幻灯片

从图 5中的炸药粒子速度曲线，直接判断CJ点的位置有一定困难。因此，基于ZND爆轰模型假设，采用G.B.Loboiko等^[5]提出的对曲线求导的计算方法，确定爆轰CJ点的位置。

在半对数坐标系下，对速度-时间曲线取导数，粒子速度曲线的变化规律就体现成了可以近似成2条相交的直线，并且对应爆轰产物等熵膨胀区的直线斜率几乎为零。2条直线的交点即为CJ点，从而可以得出炸药的爆轰反应时间t_CJ。按照上述方法，对第1发实验C-1炸药的粒子速度曲线进行处理，结果如图 6所示。

图 6 C-1炸药粒子速度导数-时间曲线

Figure 6. Derivative of particle velocity at C-1-LiF interface varying with time

下载: 全尺寸图片幻灯片

对图 6中拟合出的2条直线积分，可知在CJ点前后界面粒子速度u_p随时间的变化规律为：

${\mathit{u}_{\rm{p}}} = \left\{ \begin{array}{l} {u_{11}} + {u_{12}}\exp \left( { - t/\mathit{\tau }} \right){\rm{\;\;\;\;\;\;\;\;\;\;\;\;}}\mathit{t < }{\mathit{t}_{{\rm{CJ}}}}\\ {\mathit{u}_{{\rm{p0}}}} + \mathit{u't}{\rm{\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;}}\mathit{t} \ge {\mathit{t}_{{\rm{CJ}}}} \end{array} \right.$

(3)

式中：u₁₁、u₁₂、u_p0、u′、τ为拟合过程中形成的系数。

已知在ZND爆轰模型中，前沿冲击波与爆轰反应区按爆速D沿炸药传播，则炸药的反应区宽度x₀可以近似为^[11]：

${x_0} = \int_0^{{t_{{\rm{CJ}}}}} {\left( {D - {u_{\rm{p}}}} \right)} {\rm{d}}t$

(4)

根据Goranson公式和LiF晶体的Hugoniot关系可知压力p与界面粒子速度u_p的关系为^[12]：

$p = \frac{1}{2}{u_{\rm{p}}}\left[ {{\rho _{{\rm{m}}0}}\left( {5.15 + 1.35{u_{\rm{p}}}} \right) + {\rho _0}D} \right]\$

(5)

式中：ρ₀为被测炸药的初始密度，g/cm³；ρ_m0为窗口材料的初始密度，g/cm³；界面粒子速度u_p和爆速D的单位均为km/s，压力p的单位为GPa。

通过电探针法测得密度为1.943 g/cm³的C-1炸药的爆速为9 100 m/s。由式(3)~(5)得到的C-1炸药的爆轰反应区参数如表 1所示。考虑到实验数据的离散性，CJ时刻的粒子速度u_{p, CJ}取式(3)2条拟合曲线的交点，为1 817 m/s。将C-1的实验结果与LX-19的模拟计算结果相对比，结果如表 1及图 7所示。计算中，将炸药反应度λ从0到1的时间定义为反应时间，粒子速度从峰值经反应时间后达到的速度为CJ速度。

图 7 C-1炸药与LX-19炸药窗口界面粒子速度曲线对比

Figure 7. Comparison of particle velocity curves at explosive-LiF interfaces between C-1 and LX-19

下载: 全尺寸图片幻灯片

表 1 炸药爆轰反应区参数

Table 1. Parameters for reaction zones of explosivess

炸药	ρ/(g·cm^-3)	方法	t_CJ/ns	x₀/mm	p_CJ/GPa
C-1	1.943	实验	38	0.27	34.2
LX-19	1.942	计算	40	0.28	35.2

下载: 导出CSV

| 显示表格

从表 1可以看出，C-1炸药的爆轰反应时间很短，只有38 ns，而实验中的时间分辨率为5 ns，这使测量到的炸药反应区内的数据点很少，给判断CJ点的位置造成了困难，而由于反应区内粒子速度变化很快，实验中很可能没有测量到粒子速度的最大值，因此，还需要进一步提高测量系统的时间分辨率。从表 1和图 7可以看到：C-1炸药的爆轰反应区参数与LX-19炸药的接近，2种炸药粒子速度随时间的变化规律也基本一致；在炸药爆轰反应区，C-1炸药的粒子速度略低于LX-19炸药的粒子速度，C-1炸药的粒子速度峰值为2 050 m/s，低于LX-19炸药的2 388 m/s，在CJ点后2种炸药反应产物的粒子速度基本相同。

3. 小结

(1) 采用点火增长模型对CL-20混合炸药爆轰过程进行数值模拟，设计了CL-20混合炸药与窗口的界面粒子速度测量实验。

(2) 根据计算设计的实验装置，采用激光干涉法获得了C-1炸药与LiF窗口的界面粒子速度随时间的变化曲线。数据处理结果显示，密度为1.943 g/cm³的C-1炸药的爆轰反应时间为38 ns，反应区宽度为0.27 mm，CJ压力为34.2 GPa。

(3) 由于CL-20混合炸药爆轰反应时间很短，还需要进一步提高测量系统的时间分辨率，从而提高其测量精度。

图 1 牡蛎壳材料、圆形实验试样、试样纵剖面电镜照片与局部放大

Figure 1. Oyster shell material, circular sample, longitudinal section electron microscopy (SEM) and local magnification

下载: 全尺寸图片幻灯片

图 2 CO₂脉冲加载与激光干涉测速系统(VISAR)

Figure 2. CO₂ pulse loading and laser interferometer velocimetry system (VISAR)

下载: 全尺寸图片幻灯片

图 3 几种分数阶本构模型

Figure 3. Several fractional differential constitutive models

下载: 全尺寸图片幻灯片

图 4 单个半脉宽正弦函数

Figure 4. Single half pulse width sine function

下载: 全尺寸图片幻灯片

图 5 正弦波在Abel黏壶模型介质的衰减

Figure 5. Attenuation of a single half pulse width sine wave in Abel model media

下载: 全尺寸图片幻灯片

图 6 当α分别为1.0和2.0时的波传播特性

Figure 6. The property of wave propagation when the order α is 1.0 and 2.0 , respectively

下载: 全尺寸图片幻灯片

图 7 参数α、η和E对幅值衰减的影响

Figure 7. Influence of parameters α, η and E on amplitude attenuation

下载: 全尺寸图片幻灯片

图 8 各个密度下不同厚度牡蛎试样的速度信号及拟合曲线

Figure 8. Velocity signals and fitting curves of oyster samples with different thicknesses and densities

下载: 全尺寸图片幻灯片

图 9 牡蛎试样分数阶模型的拟合曲线及参数与牡蛎试样密度的关系

Figure 9. The fitting curve of the fractional model of the oyster sample and relationship between parameters and oyster sample density

下载: 全尺寸图片幻灯片

表 1 牡蛎壳试样分数阶模型的拟合参数

Table 1. Fitting parameters of the fractional model of the oyster sample

材料本构	密度/(g·cm⁻³)	α	E/GPa	η/(Pa·s^α)
Abel模型	0.5	0.631	-	9.09×10⁴
	0.7	0.811	-	5.65×10³
	0.7	1.025	-	192.12
	1.2	1.181	-	17.84
	1.4	1.297	-	2.19
分数阶Maxwell模型	0.5	0.690	2.21	6.71×10⁴
	0.7	0.851	7.34	3.55×10³
	0.7	0.897	7.67	1.45×10³
	1.2	1.033	12.88	170.01
	1.4	1.224	15.40	6.76

下载: 导出CSV

表 2 固定分数阶阶数情况下，牡蛎壳试样分数阶Maxwell模型的拟合参数

Table 2. Fitting parameters of fractional Maxwell model for oyster shell samples in the case of fixed fractional order

材料本构	密度/(g·cm⁻³)	α	E/GPa	η/(Pa·s^α)
分数阶Maxwell模型	0.5	0.94	2.44	1518.00
	0.7		7.67	929.35
	0.7		7.82	751.88
	1.2		13.15	687.85
	1.4		16.46	471.30

下载: 导出CSV

参考文献(33)

[1]	杨挺青. 黏弹性力学 [M]. 武汉: 华中理工大学出版社, 1992: 1–28.
[2]	JI B H, GAO H J. Mechanical properties of nanostructure of biological materials [J]. Journal of the Mechanics and Physics of Solids, 2004, 52: 1963–1990. DOI: 10.1016/j.jmps.2004.03.006.
[3]	MAO L B, GAO H L, YAO H B, et al. Synthetic nacre by predesigned matrix-directed mineralization [J]. Science, 2016, 354: 107–110. DOI: 10.1126/science.aaf8991.
[4]	GAO H L, CHEN S M, MAO L B, et al. Mass production of bulk artificial nacre with excellent mechanical properties [J]. Nature Communications, 2017(8): 287. DOI: 10.1038/s41467-017-00392-z.
[5]	WU K J, SONG Z Q, ZHANG S S, et al. Discontinuous fibrous Bouligand architecture enabling formidable fracture resistance with crack orientation insensitivity [J]. Proceedings of the National Academy of Sciences, 2020, 117(27): 15465–15472. DOI: 10.1073/pnas.2000639117.
[6]	HUANG Z W, LI H Z, PAN Z L, et al. Uncovering high-strain rate protection mechanism in nacre [J]. Scientific Reports, 2011(1): 148. DOI: 10.1038/srep00148.
[7]	徐松林, 刘永贵, 席道瑛等. 弹性波在含双裂纹岩体中的传播分析 [J]. 地球物理学报, 2012, 55(3): 944–952. DOI: 10.6038/j.issn.0001-5733.2012.03.024. XU S L, LIU Y G, XI D Y, et al. Analysis of propagation of elastic wave in rocks with double-crack model [J]. Chinese Journal of Geophysics, 2012, 55(3): 944–952. DOI: 10.6038/j.issn.0001-5733.2012.03.024.
[8]	谭子翰, 徐松林, 刘永贵, 等. 含多种尺寸缺陷岩体中的弹性波散射 [J]. 应用数学和力学, 2013, 34(1): 38–48. DOI: 10.3879/j.issn.1000-0887.2013.01.005. TAN Z H, XU S L, LIU Y G, et al. Scattering of elastic waves by multi-size defects in rock mass [J]. Applied Mathematics and Mechanics, 2013, 34(1): 38–48. DOI: 10.3879/j.issn.1000-0887.2013.01.005.
[9]	WANG P F, XU S L, LI Z B, et al. Experimental investigation on the strain-rate effect and inertia effect of closed-cell aluminum foam subjected to dynamic loading [J]. Materials Science and Engineering A, 2014, 620: 253–261. DOI: 10.1016/j.msea.2014.10.026.
[10]	MIAO C H, XU S L, SONG Y P, et al. Influence of stress state on dynamic breakage of quartz glass spheres subjected to lower velocity impacting [J]. Powder Technology, 2022, 397: 117081. DOI: 10.1016/j.powtec.2021.117081.
[11]	HUANG J Y, HU S S, XU S L, et al. Fractal crushing of granular materials under confined compression at different strain rates [J]. International Journal of Impact Engineering, 2017, 106: 259–265. DOI: 10.1016/j.ijimpeng.2017.04.021.
[12]	TING T C T, MUKUNOKI I. A theory of viscoelastic analogy for wave propagation normal to the layering of a layered medium [J]. Journal of Applied Mechanics, 1979, 46(2): 329–336. DOI: 10.1115/1.3424550.
[13]	张鸣, 王道荣, 单俊芳, 等. 石英纤维布叠层材料冲击性能研究 [J]. 实验力学, 2018, 33(2): 183–193. DOI: 10.7520/1001-4888-17-201. ZHANG M, WANG D R, SHAN J F, et al. Investigation on impact properties of quartz fiber cloth laminated material [J]. Journal of Experimental Mechanics, 2018, 33(2): 183–193. DOI: 10.7520/1001-4888-17-201.
[14]	李毅, 苗春贺, 徐松林, 等. 梯度密度黏弹性材料的波传播研究 [J]. 爆炸与冲击, 2021, 41(1): 013202. DOI: 10.11883/bzycj-2020-0313. LI Y, MIAO C H, XU S L, et al. Wave propagation in density-graded viscoelastic material [J]. Explosion and Shock Waves, 2021, 41(1): 013202. DOI: 10.11883/bzycj-2020-0313.
[15]	李毅. 梯度密度黏弹性材料的冲击响应研究 [D]. 合肥: 中国科学技术大学, 2020: 17–74. DOI: 10.27517/d.cnki.gzkju.2021.001407. LI Y. Investigation of dynamic response in density-graded viscoelastic material [D]. Hefei, Anhui, China: University of Science and Technology of China, 2021: 17–74. DOI: 10.27517/d.cnki.gzkju.2021.001407.
[16]	陈文, 孙洪广, 李西成, 等. 力学与工程问题的分数阶导数建模 [M]. 北京: 科学出版社, 2010: 12–56.
[17]	BAGLEY R L. Power law and fractional calculus model of viscoelasticity [J]. AIAA Journal, 1989, 27(10): 1412–1417. DOI: 10.2514/3.10279.
[18]	雷经发, 许孟, 刘涛, 等. 聚氯乙烯弹性体静动态力学性能及本构模型 [J]. 爆炸与冲击, 2020, 40(10): 103103. DOI: 10.11883/bzycj-2019-0249. LEI J F, XU M, LIU T, et al. Static/dynamic mechanical properties and a constitutive model of a polyvinyl chloride elastomer [J]. Explosion and Shock Waves, 2020, 40(10): 103103. DOI: 10.11883/bzycj-2019-0249.
[19]	段宇星, 杨强, 赵苗苗, 等. 弹性体材料应变率相关力学行为模型 [J]. 橡胶工业, 2020, 67(12): 899–903. DOI: 10.12136/j.issn.1000-890X.2020.12.0899. DUAN Y X, YANG Q, ZHAO M M, et al. Strain rate-related mechanical behavior model of elastomer material [J]. China Rubber Industry, 2020, 67(12): 899–903. DOI: 10.12136/j.issn.1000-890X.2020.12.0899.
[20]	WANG P F, YANG J L, SUN G Z, et al. Twist induced plasticity and failure mechanism of helical carbon nanotube fibers under different strain rates [J]. International Journal of Plasticity, 2018, 110: 74–94. DOI: 10.1016/j.ijplas.2018.06.007.
[21]	WANG P F, YANG J L, ZHANG X, et al. Dynamic behavior of carbon nanofiber-modified epoxy with the effect of polydopamine-coated interface [J]. Mechanics of Advanced Materials and Structures, 2018: 1827–1839. DOI: 10.1080/15376494.2018.1529843.
[22]	薛晓. 碳纳米管纤维的动静态力学性能研究 [D]. 合肥: 中国科学技术大学, 2020: 53–61. DOI: 10.27517/d.cnki.gzkju.2020.000565. XUE X. Investigation of dynamic and quasi-static mechanical properties of carbon nanotube fibers [D]. Hefei, Anhui, China: University of Science and Technology of China, 2020: 53–61. 10.27517/d.cnki.gzkju.2020.000565.
[23]	XUE X, WANG P F, GONG M, et al. Time-dependent microstructural evolution mechanisms of twisted carbon nanotube fibers under tension and relaxation [J]. International Journal of Plasticity, 2021, 136: 102866. DOI: 10.1016/j.ijplas.2020.102866.
[24]	赵永玲, 侯之超. 基于分数导数的橡胶材料两种粘弹性本构模型 [J]. 清华大学学报(自然科学版), 2013, 53(3): 378–383. DOI: 10.16511/j.cnki.qhdxxb.2013.03.004. ZHAO Y L, HOU Z C. Two viscoelastic constitutive models of rubber materials using fractional derivations [J]. Journal of Tsinghua University (Science and Technology), 2013, 53(3): 378–383. DOI: 10.16511/j.cnki.qhdxxb.2013.03.004.
[25]	BAGLEY R L, TORRVIK P J. A theoretical basis for the application of fractional calculus to viscoelasticity [J]. Journal of Rheology, 1983, 27(3): 201–210. DOI: 10.1122/1.549724CODEN:JORHD2.
[26]	寇磊. 分数阶微分型双参数黏弹性地基矩形板受荷响应 [J]. 力学季刊, 2013, 34(1): 154–160. DOI: 10.3969/j.issn.0254-0053.2013.01.020. KOU L. Response of rectangular plate on fractional derivative two-parameter viscoelastic foundation [J]. Chinese Quarterly of Mechanics, 2013, 34(1): 154–160. DOI: 10.3969/j.issn.0254-0053.2013.01.020.
[27]	尹耀得, 赵德敏, 刘建林, 等. 丙烯酸弹性体的率相关分数阶黏弹性模型研究 [J]. 力学学报, 2022, 54(1): 154–162. DOI: 10.6052/0459-1879-21-445. YIN Y D, ZHAO D M, LIU J L, et al. Study on the rate dependency of acrylic elastomer-based fractional viscoelastic model [J]. Chinese Journal of Theoretical and Applied Mechanics, 2022, 54(1): 154–162. DOI: 10.6052/0459-1879-21-445.
[28]	ZHAO D M, YIN Y D, LIU J L. A fractional finite strain viscoelastic model of dielectric elastomer [J]. Applied Mathematical Modelling, 2021, 100: 564–579. DOI: 10.1016/j.apm.2021.08.023.
[29]	ROSSIKHIN Y A, SHITIKOVA M V. Application of fractional calculus for dynamic problems of solid mechanics: novel trends and recent results [J]. Applied Mechanics Reviews, 2009, 63: 010801. DOI: 10.1115/1.4000563.
[30]	冯卓意, 陈雪梅. 贝壳资源的深加工利用 [J]. 材料科学与工程学报, 2022, 40(1): 123–128. DOI: 10.14136/j.cnki.issn1673-2812.2022.01.020. FENG Z Y, CHEN X M. Research on the processing technology of seashell resource [J]. Journal of Materials Sciense and Engineering, 2022, 40(1): 123–128. DOI: 10.14136/j.cnki.issn1673-2812.2022.01.020.
[31]	孙晋美, 郭万林. 贝壳珍珠母多级结构的化学-力学稳定性 [J]. 中国科学:物理学力学天文学, 2009, 39(11): 1654–1663. SUN J M, GUO W L. Chemical-mechanical stability of the hierarchical structure of shell nacre [J]. Scientia Sinica: Physica, Mechanica and Astronomica, 2009, 39(11): 1654–1663.
[32]	CONNOLLY J A. The numerical solution of fractional and distributed order differential equations [D]. Liverpool, UK: University of Liverpool, 2004: 13–48.
[33]	张元林. 工程数学(积分变换) [M]. 4版. 北京: 高等教育出版社, 2010: 85–88.