Determination and application of the HJC constitutive model parameters for ultra-high performance concrete
-
摘要: 在超高性能混凝土的数值模拟中,合理地确定其本构模型参数是提高计算精度和设计可靠度的基础。基于超高性能混凝土单轴压缩试验、霍普金森压杆试验和已有的三轴围压试验等,确定了超高性能混凝土的Holmquist-Johnson-Cook (HJC)本构模型参数。利用LS_DYNA软件模拟单向板爆炸试验,通过与试验中单向板的损伤程度和最大挠度进行对比,验证了已确定参数的有效性。为了进一步了解超高性能混凝土构件的抗爆机理,采用已确定的参数对单向板爆炸工况进行数值模拟,分析配筋和尺寸变化对爆炸结果的影响。结果表明,在爆炸过程中,提高纵筋配筋率可以减小单向板的跨中最大挠度,适当加密箍筋可以减小单向板侧面的斜裂缝长度。超高性能混凝土单向板具有明显的尺寸效应,其中厚度和长度变化对爆炸结果的影响最突出。Abstract: The parameters of the Holmquist-Johnson-Cook (HJC) constitutive model for ultra-high performance concrete (UHPC) were determined based on uniaxial compression test, split Hopkinson pressure bar (SHPB) test and existing tri-axial compression test and so on, in order to improve the calculation accuracy and design reliability. In the determination process of parameters, the parameters of the HJC constitutive model were divided into five categories. The yield-surface parameters were determined by the static failure surface equation, the parameters of state equation were determined by the p-μ relation, the damage parameters were determined according to relevant literature, the basic physical parameters were determined according to the test, and so on. LS_DYNA was used to simulate the explosion test of the one-way slab. Firstly, the finite element model of the one-way slab was established. The HJC constitutive model was used for the UHPC, and the linear reinforcement model was used for the reinforcement material. The reinforcement and UHPC were connected by common joints. The air and explosive models were established, and the fluid-solid coupling method was used for calculation. The effectiveness of the determined parameters was verified by comparing the simulation results with the damage degree and the maximum deflection of the one-way slab in the test. In order to further understand the anti-blast mechanism of the UHPC members, the determined parameters were used to conduct numerical simulation on the one-way slab explosion condition, and the influences of reinforcement and size effect on the explosion result were analyzed. Results show that during the explosion process, the maximum mid-span deflection of the one-way slab can be reduced by increasing the longitudinal reinforcement ratio, and the length of oblique cracks on the side of the one-way slab can be reduced by properly encrypted stirrups. The UHPC one-way slab has an obvious size effect, and the variation of its thickness and length has the greatest influence on the explosion result.
-
超高性能混凝土(ultra-high performance concrete, UHPC)是近年来发展起来的一种新型水泥基材料,具有较高的强度、韧性、抗冲击性以及耐久性[1-3]。杜忠等[4]对高延性混凝土的抗爆性能开展了研究,发现在爆炸冲击作用下,混凝土的强度和韧性越高,对冲击波冲量的吸收能力越强。若将UHPC应用于防护工程,必将显著提高防护结构的抗力水平。目前,我国对于UHPC材料的研究和应用尚处于起步阶段,其抗爆性能的研究成果并不丰富,这严重阻碍了UHPC在国防工程中的应用。开展UHPC抗爆性能研究,通常有试验、理论分析和数值模拟3种方法。由于试验费用较高,存在一定的危险性和环境影响[5],并且UHPC爆炸试验处于高应变率状态下,持续时间极短,普通的传感器或应变片很难精确记录基体在抗爆破坏过程中的变化情况。而理论分析简化过多,难以得到可靠的方程解。因此,数值模拟成为开展UHPC抗爆性能研究的重要方法。
然而,大量实践表明:数值模拟结果的准确性取决于动态本构模型的选择及参数的确定。因此,开展UHPC动态本构模型的研究非常必要,这对于了解UHPC结构抗爆破坏机理,建立UHPC结构的抗爆性能设计方法,推广其在国防工程中的应用都具有重要意义。目前,在爆炸数值模拟中常用的混凝土动态本构模型有Riedel-Hiermaier-Thoma (RHT)本构模型[6]、K&C本构模型[7]和Holmquist-Johnson-Cook(HJC)本构模型[8]。其中RHT本构模型对混凝土损伤裂纹的描述较成功,但其参数较多,标定过程复杂,并且UHPC相关试验数据较少,很难采用岩石材料参数的确定方法去标定UHPC材料参数。K&C本构模型可以自动生成大量参数,为研究人员提供了极大的便利。但模拟试验表明,针对强度较高的混凝土,自动生成的参数往往达不到精度要求,而关于K&C参数标定的相关研究内容较少。在损伤表达方面,K&C本构模型考虑较全面,但模拟效果表明,其损伤程度也往往偏大[9]。HJC本构模型能够很好地描述材料在强动载作用下的力学行为,且该模型参数数量相对较少、物理意义明确,其中大多数参数可以由试验及公式获得[10],使该本构模型在高压、大应变、高应变率工况的数值模拟计算中,实现了简单性和准确性的良好折中。因此,HJC本构模型在混凝土爆炸数值模拟中被广泛使用[11]。如Liu等[12]利用HJC模型对钢筋混凝土材料进行了爆炸数值模拟,分析了混凝土的动态力学响应规律。张志刚等[13]在数值模拟中,采用HJC模型建立了混凝土板构件,研究了碳纤维布加固混凝土板的抗爆能力。张志华等[14]利用HJC模型建立了不同强度的混凝土块,分析了爆炸工况下混凝土内部的应力波传递规律。然而,在HJC本构模型的参数确定方面,由于缺少相关试验,大多数研究人员套用已有文献相似材料的计算参数,导致计算结果与试验结果差距较大。近年来,针对该本构模型参数的确定,国内外学者开展了大量研究,并取得了一定成果。如任根茂等[15]基于普通混凝土相关力学试验,确定了适用于不同强度的普通混凝土HJC模型参数,并通过模拟侵彻试验进行了验证。陈睿等[16]基于霍普金森压杆(split Hopkinson pressure bar, SHPB)试验,提出了一种混凝土材料动态模型参数的分阶段反求法。张社荣等[17]基于动、静态力学试验对HJC原始参数进行了修正,并通过模拟SHPB试验验证了参数的有效性。熊益波等[18]利用三轴围压数据,在不考虑损伤和应变率的基础上给出了一套确定混凝土强度参数的方法。李鹏[19]通过对强度参数进行敏感性分析并结合试验调整参数,确定出了适用于爆炸荷载的混凝土HJC本构模型参数。但以上研究大多是对普通混凝土HJC本构模型参数进行研究确定,针对超高性能混凝土HJC本构模型参数的研究未见报道。
本文中,以HJC本构模型为基础,通过相关力学试验和现有文献结论确定一组适用于UHPC的动态本构模型参数。以UHPC构件抗爆试验数据为依据,验证参数的有效性。在此基础上,开展UHPC单向板抗爆性能研究,分析配筋和尺寸效应对UHPC单向板爆炸结果的影响。
1. 参数确定
1.1 HJC模型简介
HJC本构模型包括屈服面方程、状态方程和损伤方程。屈服面方程通过无量纲等效应力描述,用于控制材料单元形状的改变。状态方程表示材料承受的静水压力与体积应变之间的关系,由弹性区、破碎区和压实区3部分组成,用来描述材料单元在高压下的体积改变。损伤方程以等效塑性应变和塑性体积应变的累积来表示。该本构模型共包含21项参数,本文中将这些参数分为基础物理参数、屈服面参数、状态方程参数、损伤参数和软件参数,并结合相关试验及文献结论进行参数确定。
1.2 基础物理参数
基础物理参数主要通过UHPC基本物理试验获得,其中最大拉伸静压力T=7.12 MPa,单轴抗压强度σc=105 MPa,剪切模量G=20.37 GPa,混凝土密度ρ=2.67 g/cm3。
1.3 屈服面参数
当不考虑应变率影响时,屈服面方程转化为静态失效面方程:
¯σ=A(1−D)+B¯pN (1) 式中:
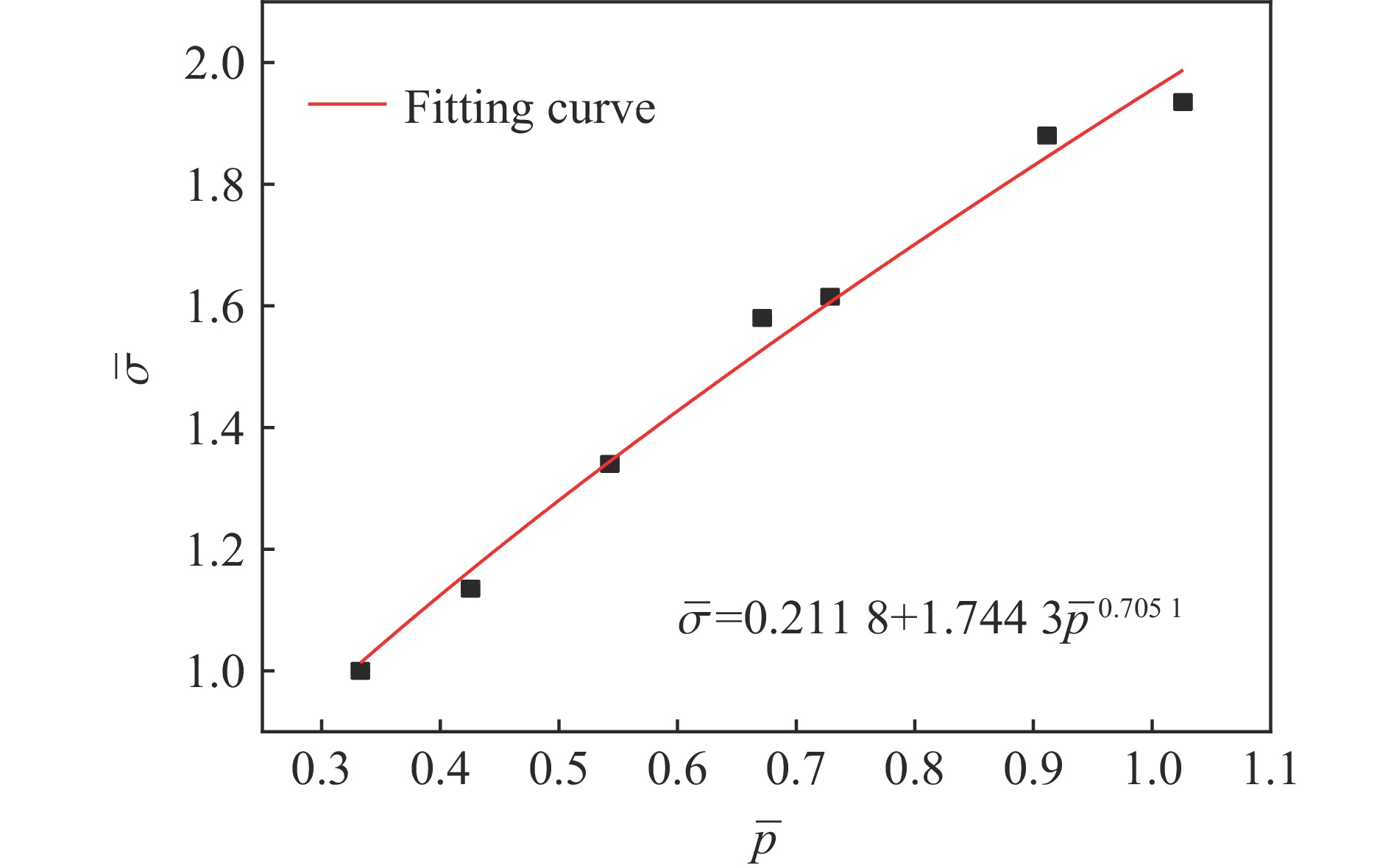
¯σ 为无量纲等效应力,A为归一化内聚力强度,B为归一化压力硬化因子,¯p 为无量纲静水压力,D为混凝土损伤程度参数,N为压力硬化指数。在静载过程中混凝土达到最大强度,材料经历了弹性阶段和屈服阶段,由于内部孔洞被压缩,混凝土已产生部分损伤。张若棋等[20]通过混凝土单轴压缩失效强度得到失效时的损伤度D=0.659 4,并推广到一般情况。但该结果是由48 MPa混凝土的A、B、N值推导而来,不适用于UHPC。试验测得UHPC的峰值应变约为0.004,与普通混凝土相近,但UHPC密度大,孔隙率较低,因而达到峰值应力时的损伤程度较普通混凝土低。根据文献[21]中的UHPC循环压缩试验得出超高性能混凝土的失效应变为0.018 1,屈服时的等效塑性应变约为0.001 6,进而推算出屈服时混凝土材料的损伤度D=0.090 0。通过三轴等拉强度[22]fttt=0.9ft、单轴抗压强度和文献[23]给出的一组等强度UHPC三轴围压数据,计算得出相应的归一化等效应力和归一化静水压力。将得到的3组数据和已确定的D值代入式(1)中,得到方程组:{0.91A−0.0613NB=00.91A+0.3333NB=10.91A+0.6511NB=1.5369 (2) 求解方程组,得到A=0.232 8。为了使确定的屈服面方程满足一般情况,将A、D的取值代入式(1),根据三轴围压数据[23]进行曲线拟合,如图1所示,得到参数B=1.744 3,N=0.705 1。
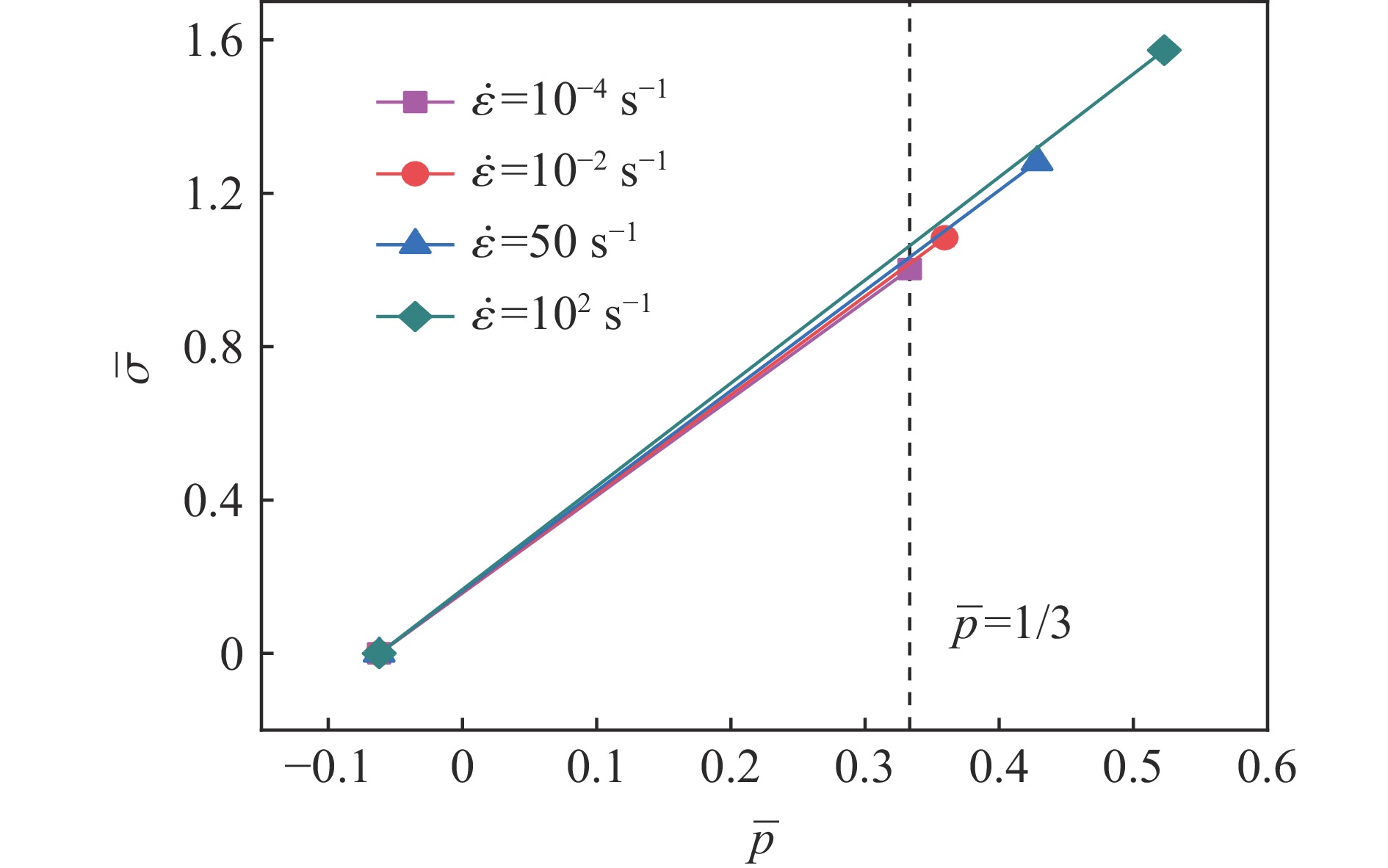
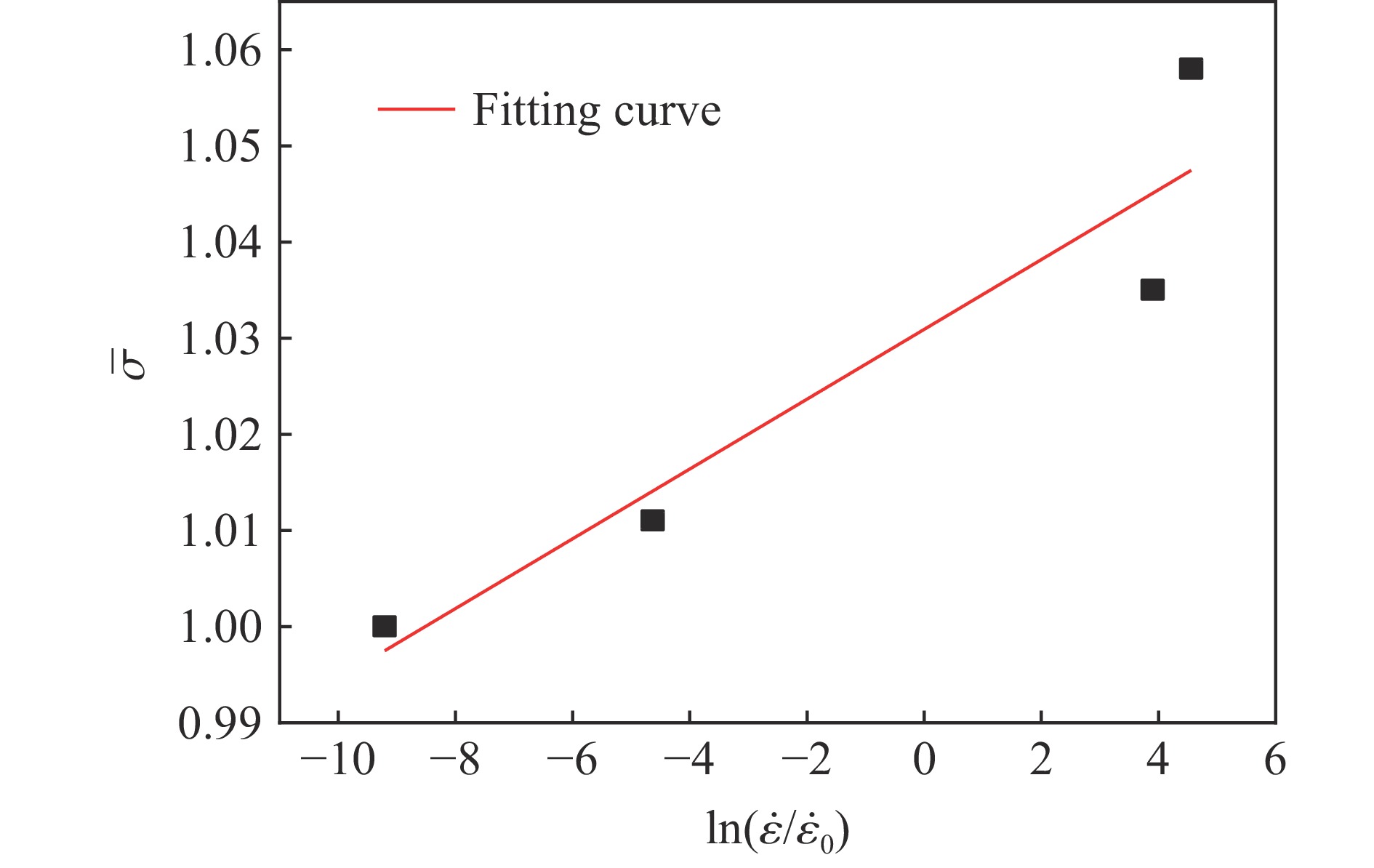
应变率系数C由静态抗压强度和动态抗压强度计算得到。通过SHPB试验得到不同应变率下C120UHPC的抗压强度,如表1所示。在动态荷载作用下,材料强度的提高受应变率效应和静水压力共同影响,在确定参数C时,首先要消除静水压力的影响。如图2所示, 从
−¯T(1−D) 出发,与表1中的数据点相连,分别作不同应变率下的直线,其中¯T 为无量纲最大拉伸压力。过¯p =1/3处作垂直于横轴的直线并与不同应变率下的直线相交于4个点。取各交点的归一化等效应力和相应的应变率数据进行线性拟合,并作¯σ 与ln(˙ε/˙ε0) 的关系曲线,其中˙ε 为材料真实应变率,˙ε0 为参考应变率。如图3所示,所拟合直线的斜率为应变率系数C,即C=0.003 6。表 1 不同应变率下的UHPC力学参数Table 1. UHPC mechanical parameters under different strain rates应变率/s−1 抗压强度/MPa ¯σ ¯p 10−4 105.0 1.000 0 0.333 3 10−2 113.3 1.079 0 0.359 7 50 134.6 1.281 9 0.427 3 102 164.7 1.568 6 0.522 9 Sfmax表示归一化等效应力所能达到的最大值。在大多数的参数敏感性分析中[24-27],该参数为不敏感参数,因此对该参数延用原始数据7.0。
1.4 状态方程参数
状态方程中弹性区和破碎区之间的过渡点静水压力表示为:
pc=σc3 (3) 式中:pc为状态方程中弹性区和破碎区之间的过渡点静水压力,根据式(3)计算得出pc=35 MPa。
通过物理试验得到混凝土弹性模量E=50 GPa,泊松比ν=0.227。根据弹性理论计算出体积模量Ke=30.525 GPa,图4给出了状态方程的p-μ关系曲线,由p-μ曲线关系可以计算出弹性阶段的曲线斜率,即μc=0.001 1。
μl为混凝土内部空隙被压实时的体积应变,在混凝土材料中该值与材料孔隙率相同。胡志豪[28]通过压汞试验得到相似配比UHPC的孔隙率为3.83%,即μl=0.038 3。
K1、K2、K3为状态方程中压实段参数。相应的表达式为:
p=K1(μ−μl1+μl)+K2(μ−μl1+μl)2+K3(μ−μl1+μl)3 (4) 在大多数模拟中,对压力常数采用原始数据,但原始参数值过高地估计了混凝土压实后的抗体积变形能力[29-30],需要重新确定。状态方程参数主要根据Hugoniot试验数据[31-34]确定,目前UHPC在这方面的试验较少,蒋国平等[35]通过气炮试验给出了C100混凝土状态方程的压力参数:K1=46.4 GPa,K2=−195.0 GPa,K3=416.6 GPa。相比48 MPa混凝土,C100高强混凝土的抗体积变形能力与C120UHPC更接近,这里采用以上参数作为C120UHPC的状态方程参数。
从图4看出,pl是破碎段曲线与压实段曲线交点处的纵坐标。由于压实段函数已经确定,下面只需求出破碎段曲线方程,通过求解方程组可以得到交点处坐标(μp, pl),其中μp为pl对应的体积应变。在状态方程破碎段中,混凝土体积应变满足μc≤μ≤μp。基于静水压力实验[23],获取上述体积应变范围内的静水压力数据,拟合第2阶段函数表达式。在拟合过程中严格要求曲线过起始点(μc, pc),以确保三段式函数的连续性,拟合结果为:
p=4.6983μ+0.0298 (5) 将已确定的K1、K2、K3、μl代入式(4),得到第3阶段表达式。联立式(5)和第3阶段表达式,求解方程组,得到μp=0.043 7,pl=235.0 MPa。
1.5 损伤参数
损伤常数D1、D2与混凝土强度无关[8],在缺少试验数值的情况下可以取原始数据,即D1=0.04,D2=1。εefmin为最小断裂应变,根据文献[21]的结论,取εefmin=0.018 1。
1.6 软件参数
参数
˙ε 0用于消除应变率的量纲,其原始数值取为1 s−1,在模拟中取1~105 s−1发现各项指标的模拟结果差异不大,因此对该参数延用原始数据1 s−1。fs用于控制混凝土的失效模式,根据孙其然等[36]提出的假设,fs满足:
fs=D1(¯pmax+¯T)D2 (6) 式中:
¯pmax 为混凝土达到极限密度ρmax时的归一化静水压力,¯T 为无量纲最大拉伸压力。林琛等[37]假设极限密度为压实密度的1.01倍,据此推算出ρmax=2.80 g/cm3,与之对应的体积应变μmax=0.048 7。将μmax和已确定的参数代入式(4),计算得出¯pmax =445.6 MPa,结合式(6)得到fs=0.1725。如表2所示,超高性能混凝土HJC本构模型参数全部确定。表 2 超高性能混凝土HJC模型参数Table 2. HJC model parametrs of UHPCA B N C T/MPa Sfmax εefmin D1 D2 fs ˙ε0/s−1 0.232 8 1.744 3 0.705 1 0.003 6 7.12 7.0 0.018 1 0.04 1.0 0.1725 1 pc/MPa pl/MPa μc μl K1/GPa K2/GPa K3/GPa σc/MPa G/GPa ρ/(g·cm−3) 35.0 235.0 0.0011 0.0383 46.4 −195.0 416.6 105.0 20.37 2.67 2. 模拟验证
确定了UHPC的HJC本构模型参数,即可通过模拟UHPC单向板抗爆试验来验证参数的合理性。
2.1 试验工况
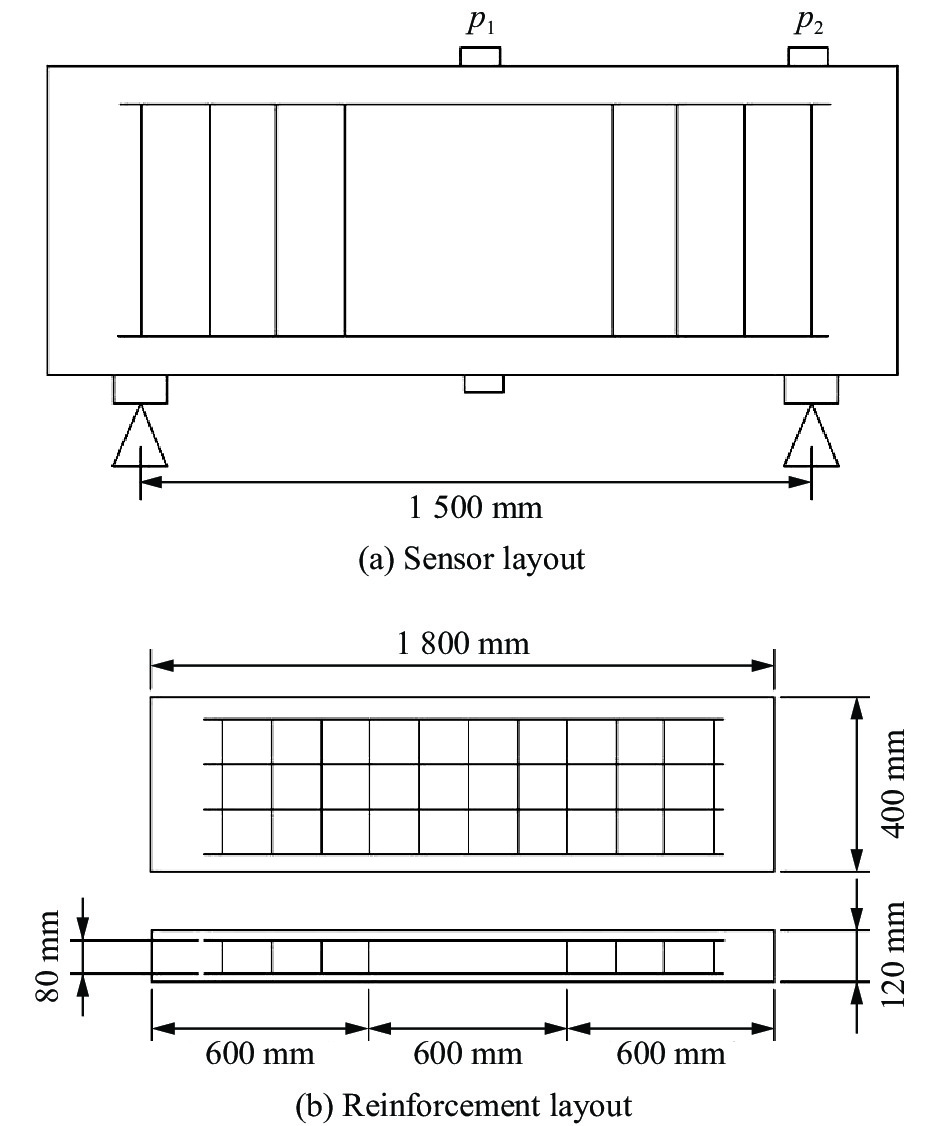
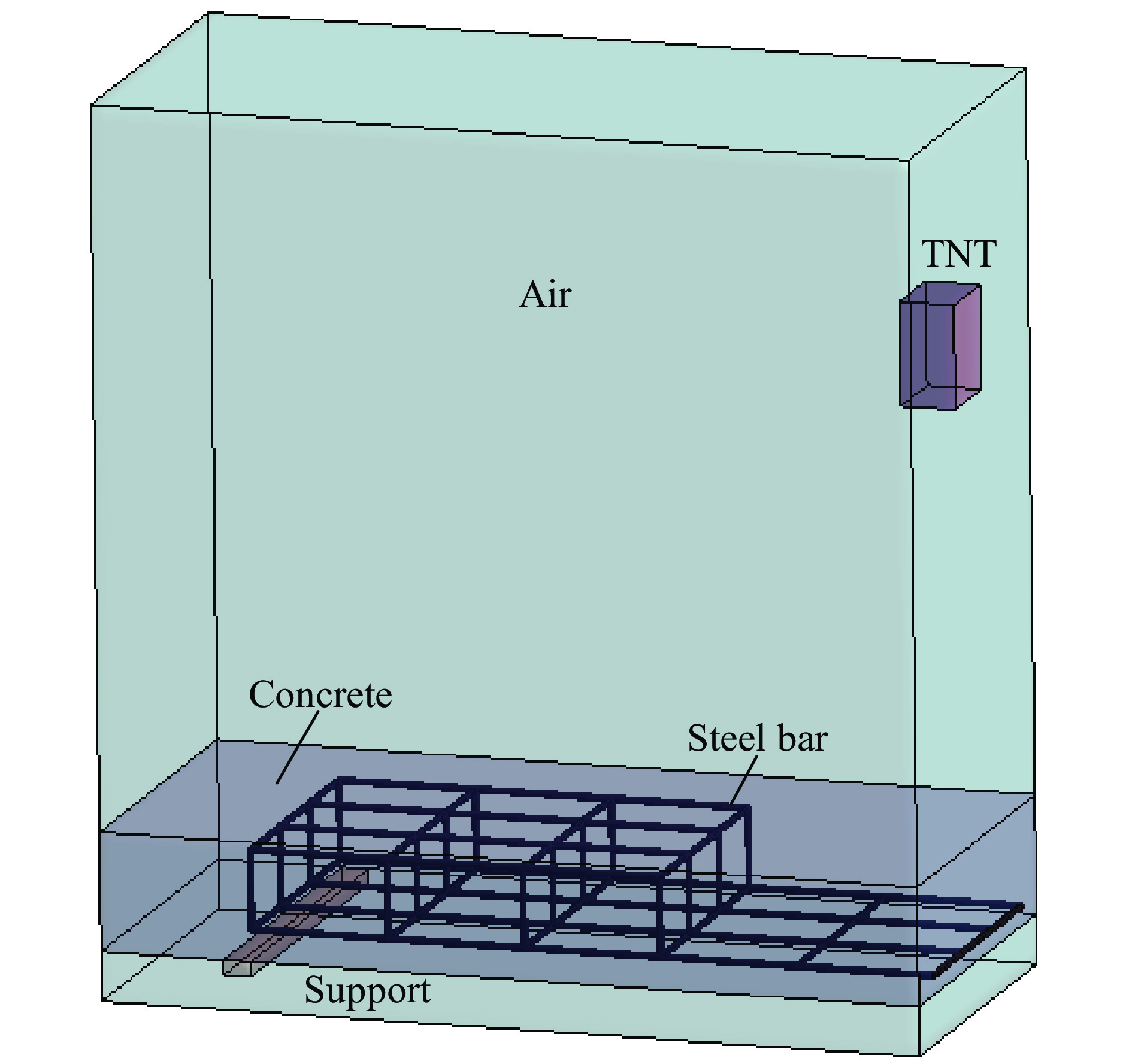
试验中采用临空板爆炸的形式,UHPC单向板试件尺寸为1 800 mm×400 mm×120 mm,炸药采用TNT,当量为4.9 kg。将炸药尽量捆绑为立方体形状,置于试件中心正上方。起爆点为炸药中心,距离板的上表面跨中1.5 m处,起爆方式为中心起爆。试验传感器布置如图5(a)所示,在UHPC单向板跨中和支点上表面分别固定2个压力传感器,用来测量所在位置的反射超压p1和p2。在跨中位置下表面两侧布置位移传感器,用来测量单向板跨中挠度。
单向板试件采用4根直径为8 mm的受拉钢筋,钢筋级别为HRB500,保护层厚度为20 mm,受拉纵筋配筋率为0.52%。试件中箍筋采用HRB400级钢筋,单向板的配筋见图5(b)。
2.2 模型建立
利用LS_DYNA软件对爆炸工况进行数值模拟。如图6所示,建立1/2模型,在对称面上施加法向约束,空气四周设置透射边界。在混凝土的一侧建立刚性、全约束支撑,混凝土材料选择HJC模型,材料取值见表2。钢筋材料选择线性强化模型MAT_PLASTIC_KINEMATIC_TITLE,该模型对于爆炸荷载作用下的金属材料非常适用[38]。材料参数由钢筋拉伸试验测得,取值如表3所示。在爆炸作用下,构件中的应力波作用时间极短,钢筋和混凝土之间可以按变形一致处理[39]。因此,对钢筋和混凝土采用共节点方式建立联系。分析使用流固耦合算法,炸药和空气采用Euler网格,钢筋混凝土板采用Lagrange网格,其中总单元数为243 000,节点数为261 000。
表 3 钢筋本构模型参数Table 3. Parameters of reinforcement constitutive models材料 密度/(g·cm−3) 弹性模量/GPa 泊松比 屈服应力/MPa 切线模量/GPa 失效应变 受拉钢筋 7.85 200 0.25 524 1.61 0.10 箍筋 7.85 200 0.25 423 1.91 0.08 2.3 结果分析
试验中,由于药量较大,未能测得跨中反射超压p1,仅测量了支座处的反射压力。p2的平均测试结果为7.20 MPa,模拟值为7.40 MPa,误差为2.7%,表明模拟加载过程相对可靠。从毁伤结果可以看出,试件完整性较好,顶部未见严重破坏,其破坏主要体现为单向板底部开裂和侧面的裂缝开展。这是因为,爆炸产生的冲击波在靶板迎爆面形成超压破坏,当应力波传递到板下表面并反射形成拉伸波对靶板造成拉伸破坏,而UHPC的拉压抗性的不同造成背爆面产生更严重的损伤。在数值模拟中,仅对原始参数[8]中的基本物理参数(抗压强度、最大拉伸静压力、剪切模量和密度)进行修改,以此作为修正前的原始参数,如表4所示。将已确定的HJC模型参数作为修正后的UHPC参数,并将模拟结果与试验结果进行比较。当计算到7 ms时,单向板变形达到最大并开始出现回弹现象,这时累积塑性损伤阶段基本结束,因此计算时间采用7 ms。
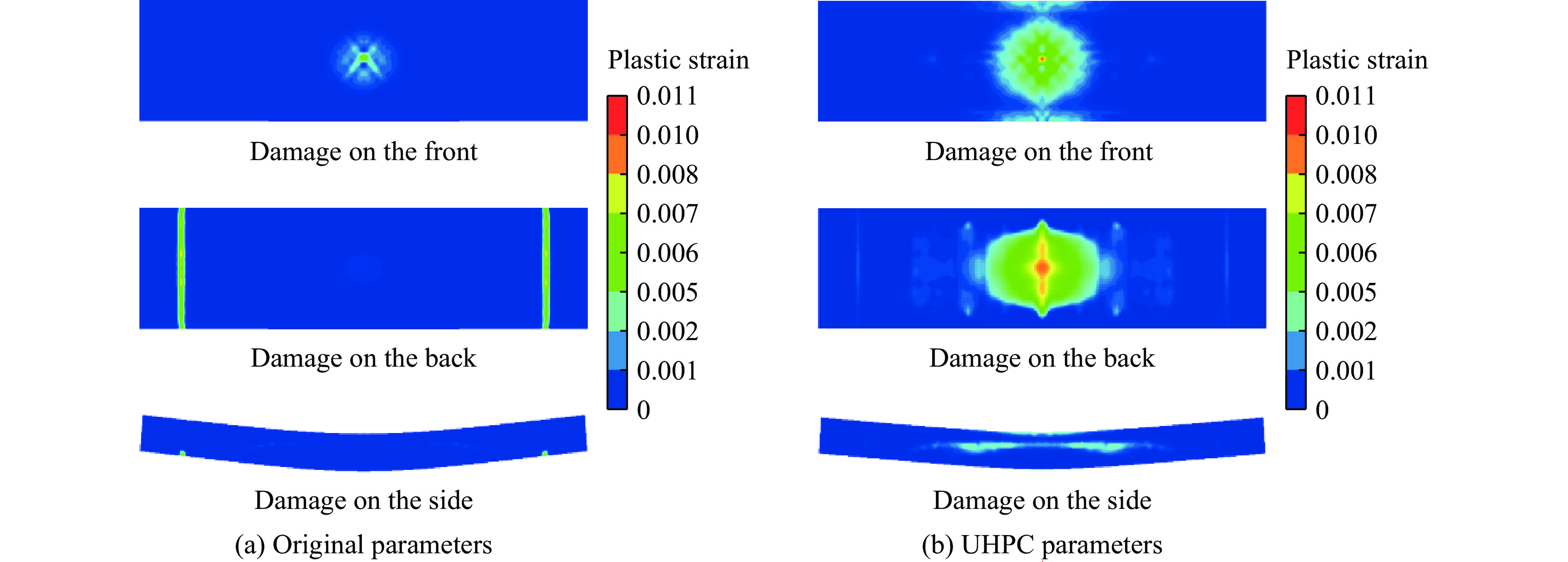
表 4 修正前的原始参数Table 4. Original parameters before correctionA B N C T/MPa Sfmax εefmin D1 D2 fs ˙ε0/s−1 0.76 1.6 0.61 0.007 7.12 7.0 0.01 0.04 1.0 − 1 pc/MPa pl/MPa μc μl K1/GPa K2/GPa K3/GPa σc/MPa G/GPa ρ/(g·cm−3) 16.0 800.0 0.001 0.1 85.0 −171.0 208.0 105.0 20.37 2.67 等效塑性应变可以直接、定量地反映材料的变形毁伤程度[40]。图7给出了不同参数下的等效塑性应变云图。从图7可以看出,在修正前的原始参数模拟结果中,单向板的迎爆面在中心出现少量损伤,而在背爆面中部没有出现损伤,其背爆面的损伤程度远小于迎爆面,与试验结果相悖。在修正后的UHPC参数模拟结果中,单向板迎爆面出现轻微塑性破坏,在背爆面中部出现较大范围的塑性损伤并向板两侧延伸,模拟出了拉伸应力波对板的破坏效果。其背爆面的破坏程度大于迎爆面,符合实际情况。观察单向板侧面,参数修正前没有出现破坏现象。参数修正后,呈现出了由中部向两侧斜向延伸的损伤带,类似于斜拉破坏特征。由此可知,修正后的UHPC参数对试件侧面斜裂缝的描述也与试验基本接近。
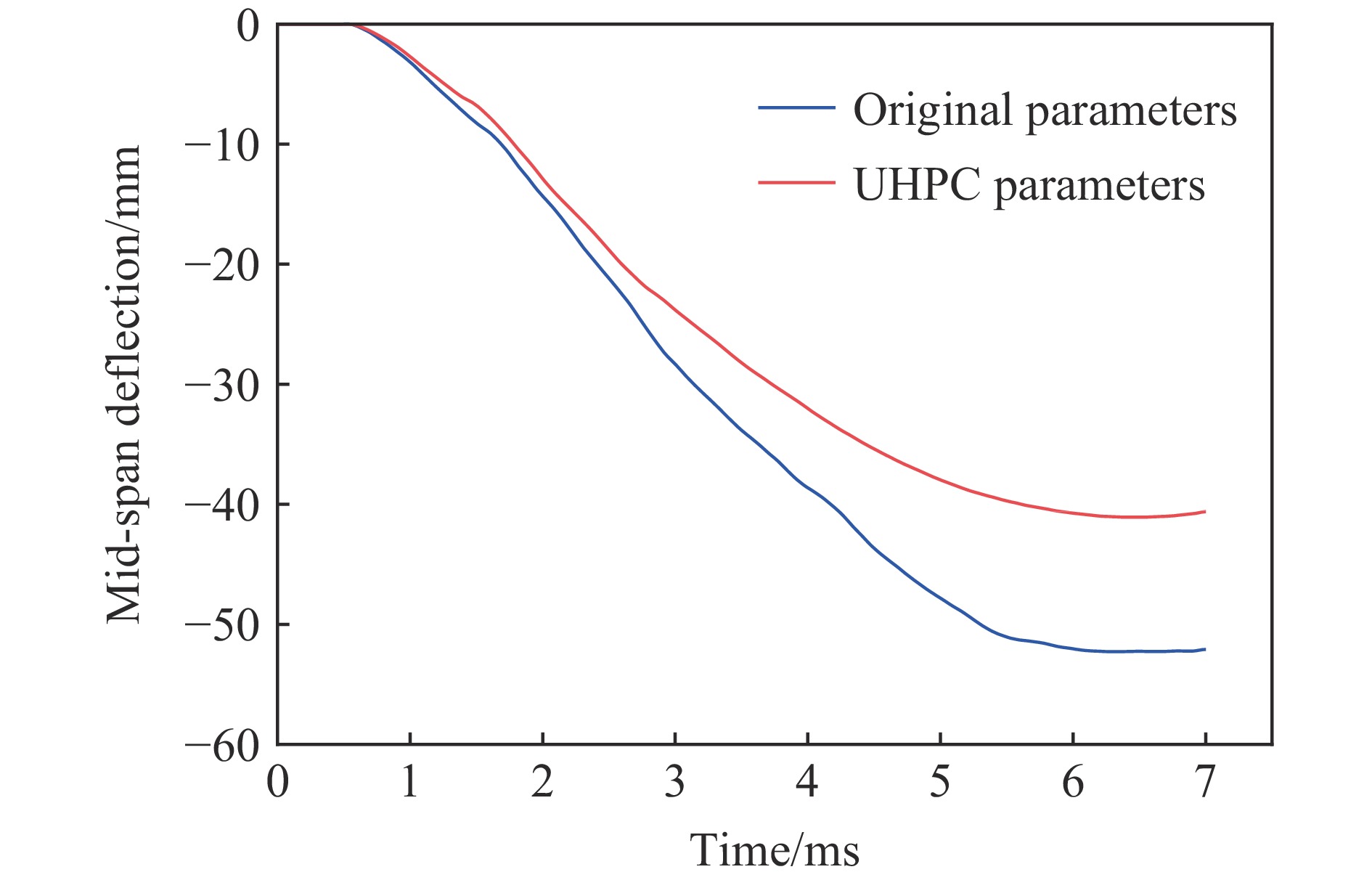
通过位移传感器,测得单向板的最大挠度为42.80 mm。图8为修正前的原始参数和修正后的UHPC参数的位移时程曲线。参数修正前的计算结果显示,板的最大挠度为53.86 mm,误差为25.8%。参数修正后的计算结果显示,板的最大挠度为41.14 mm,误差为3.9%,与试验基本一致。
综上,在爆炸工况下, 修正后的UHPC参数对单向板的跨中最大挠度和毁伤程度的描述更接近试验结果。表2给出的本构模型参数可以初步作为超高性能混凝土的HJC本构模型参数。
3. 超高性能混凝土HJC本构模型应用
3.1 配筋对UHPC单向板抗爆性能的影响
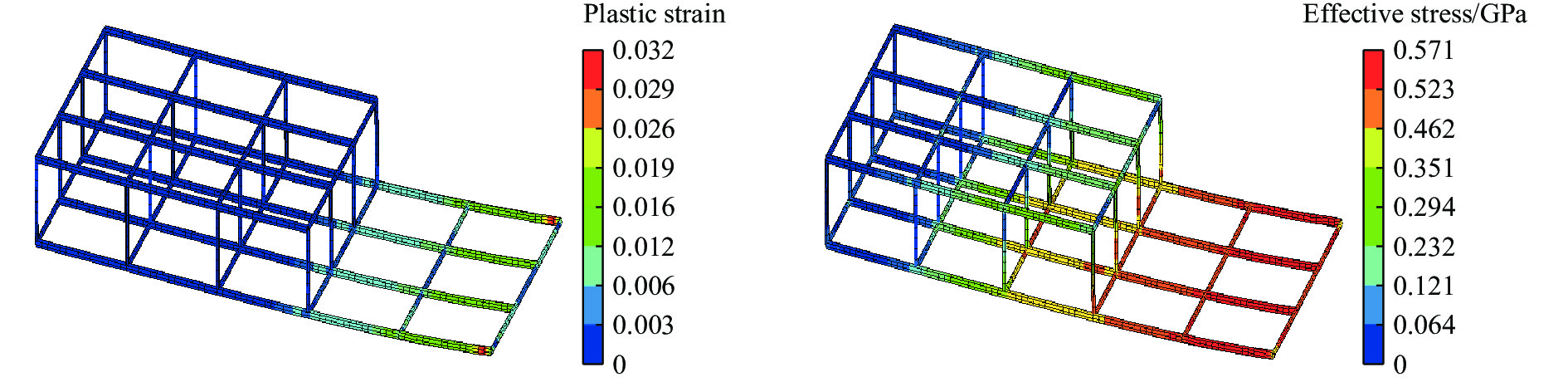
图5的试验工况中,当混凝土板达到最大挠度时,钢筋的等效塑性应变和等效应力分布如图9所示。从纵向钢筋的受力情况可以看出,底部中央的纵筋应力最大且出现塑性应变,端部上表面纵向钢筋的应力比下表面低且整体应力水平较低,基本没有产生塑性应变。说明构件在底部中央受到较大拉伸变形,其纵筋在受拉过程中达到屈服,起到了很好的耗能作用。构件端部受爆炸影响相对较小,其纵筋未达到屈服且上部钢筋的利用率更低。观察箍筋受力情况,发现底部中央的横向钢筋受力最大并出现轻微塑性变形,垂直于混凝土上下表面的钢筋也承受一定荷载,但并未发生屈服。说明在构件底部中央的横向拉伸变形较小,在构件两端有剪切破坏的趋势,因此箍筋中产生的拉应力起到了一定的作用。
为了提高钢筋的利用率,应对底部受拉纵筋采用较高级别的钢筋,上部纵向钢筋和箍筋可以采用低级别的钢筋。为了进一步了解钢筋对UHPC单向板抗爆性能的影响规律,通过数值模拟来对比不同箍筋间距、纵筋配筋率下的单向板跨中最大挠度和板侧面斜裂缝长度。模型工况为在图5基础上采用上下双层配筋,对箍筋和上部纵筋使用HRB400级钢筋,对底部纵筋使用HRB500级钢筋。其中纵筋配筋率r分别为0.26%、0.52%、0.92%,箍筋间距d分别为75、150、300 mm。
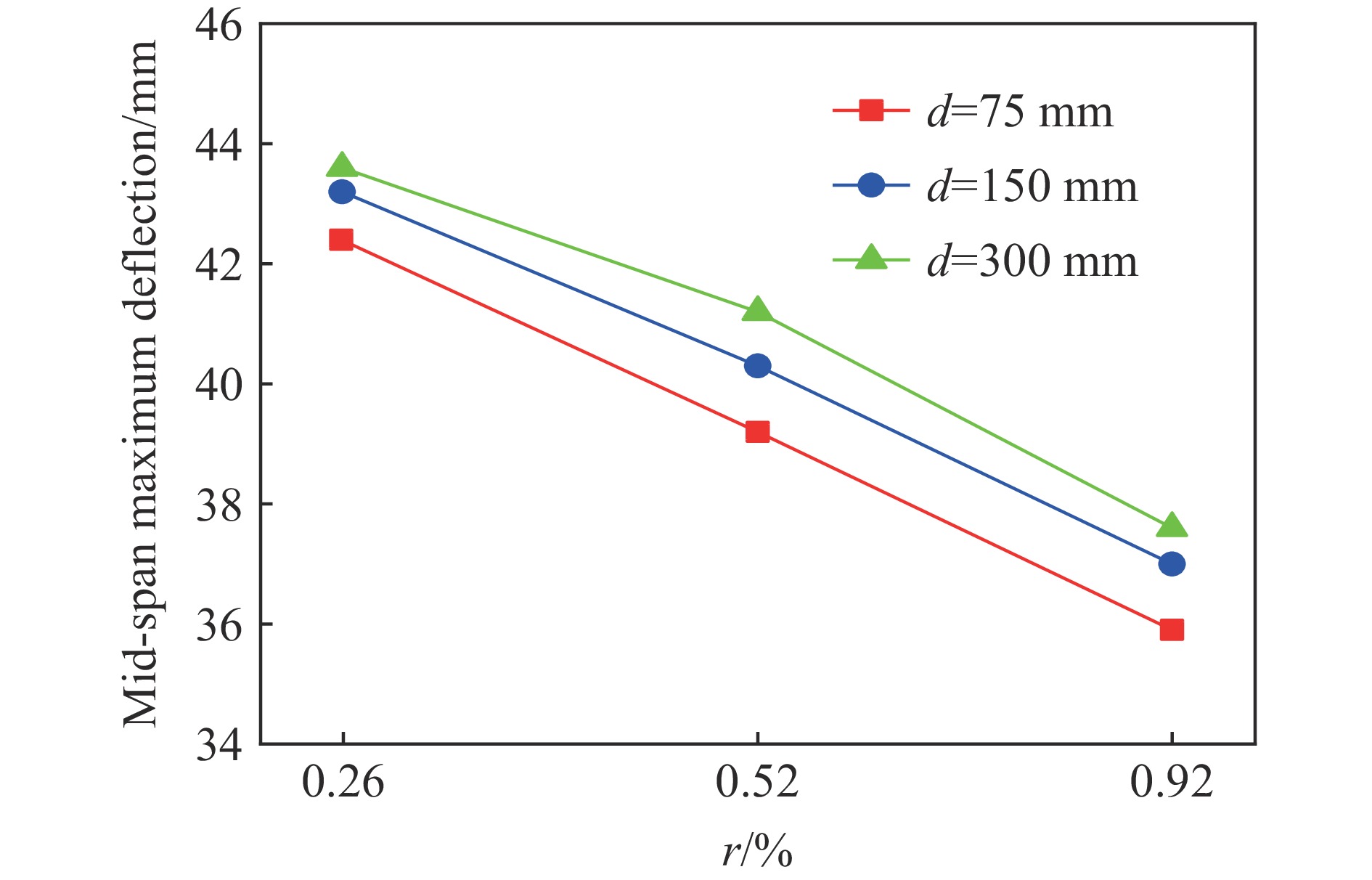
图10为不同箍筋间距下跨中最大挠度与配筋率的关系,可以看出,单向板跨中最大挠度随主筋配筋率的增加而减小,减小幅度近似呈线性。当主筋配筋率不变,减小箍筋的间距时,板的挠度最大值变化小于2 mm,变化不大。表明主筋的配筋率对跨中最大挠度的变化更敏感,提高配筋率可以提高单向板底部的受拉承载力,而加密箍筋对板跨中最大挠度影响较小。
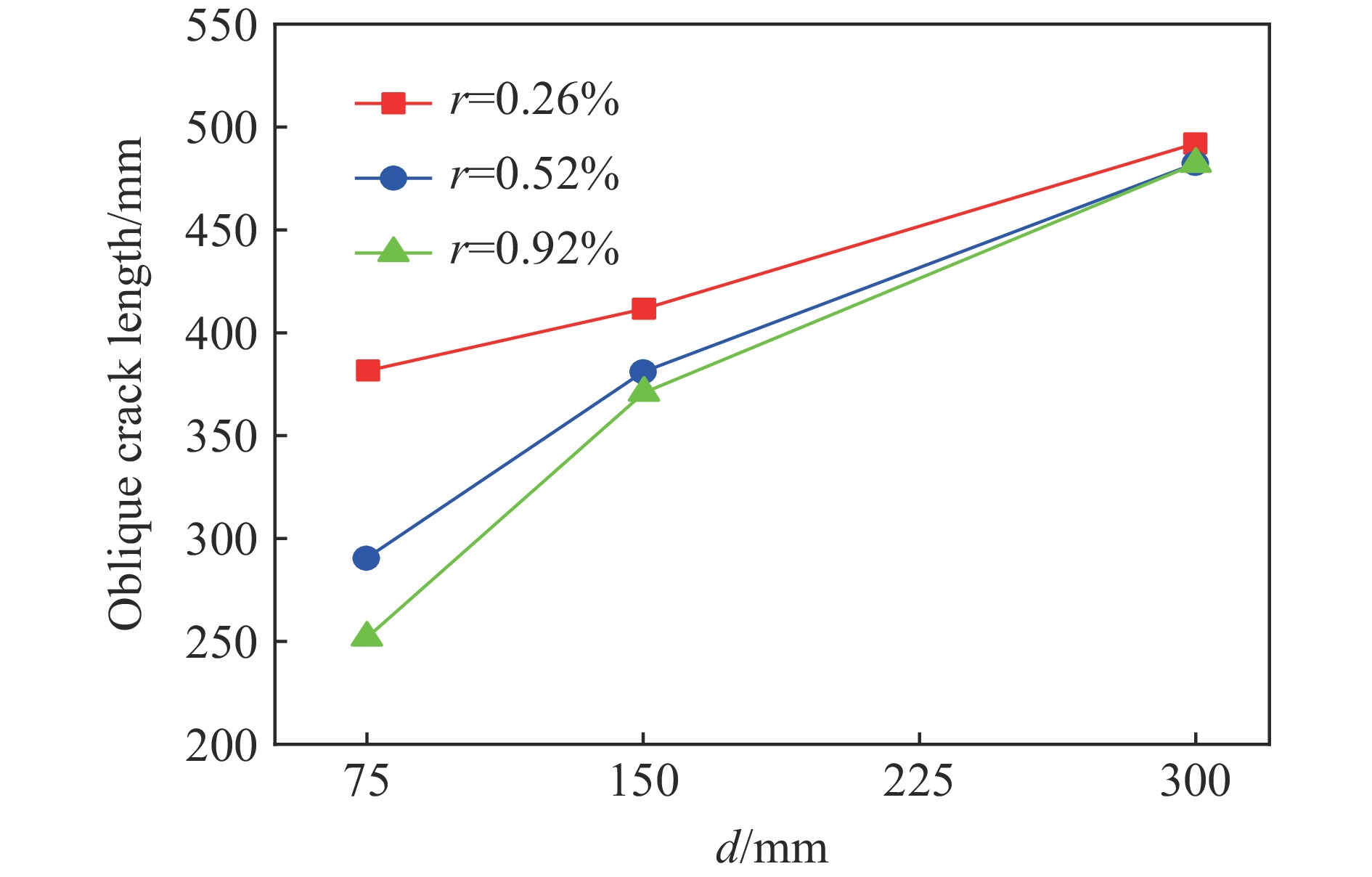
图11为不同配筋率下斜裂缝长度与箍筋间距的关系,可以看出,单向板侧面斜裂缝长度随箍筋间距减小而减小,当箍筋间距处于150~300 mm时,箍筋的作用效果减弱。主筋配筋率的提高也会在一定程度上抑制斜裂缝的增长,并且增强箍筋的作用效果。当箍筋间距为300 mm时,主筋对斜裂缝的扩展几乎没有影响,随着箍筋的不断加密,主筋的影响效果也在增强。
以上现象表明,加密箍筋可以有效抑制斜裂缝的扩展,提高UHPC单向板的抗剪承载力,但提高效率会出现下降拐点。主筋配筋率的提高也会对箍筋的作用效果起到辅助作用。
3.2 单向板尺寸对UHPC抗爆性能的影响
根据单向板的设计要求,在试验板的基础上分别对板的长、宽、厚度尺寸进行调整。变化某一方向尺寸时,保持另外2个方向尺寸不变,对单向板的长、宽、厚度各取3个尺寸进行模拟计算,尺寸变化如表5所示。通过比较不同尺寸下的单向板跨中最大挠度、斜裂缝长度及迎爆面和背爆面的毁伤面积,得到尺寸变化对UHPC单向板抗爆性能的影响规律。爆炸工况采用图5试验工况,由于只考虑混凝土影响,不建立钢筋模型。毁伤面积根据塑性应变值确定,根据UHPC单轴循环试验结果[21],分析当塑性应变累积到0.002时,UHPC材料出现明显的破裂现象,因此以该塑性应变作为毁伤界限。
表 5 单向板各方向尺寸变化Table 5. Dimension change of one-way plate in each direction长度/mm 宽度/mm 厚度/mm 1 200 400 120 1 500 500 180 1 800 600 240 图12给出了爆炸工况下UHPC单向板尺寸变化的影响规律。从图12(a)可以看出,单向板长度增加会引起迎爆面和背爆面的毁伤范围增大,当单向板长度为1 500~1 800 mm时,迎爆面的毁伤范围不再变化,而背爆面损伤范围仍在增加。单向板的裂缝长度以及跨中最大挠度也随着长度的增加而增加,这表明,UHPC单向板长度变化与构件破坏程度成正相关。从图12(b)可以看出,单向板的宽度增加同样引起迎爆面和背爆面的毁伤面积增加,当宽度为500~600 mm时,迎爆面和背爆面损伤出现相反的变化趋势,其中背爆面损伤面积的增长速率呈下降趋势。随着宽度的增加,斜裂缝长度和跨中最大挠度均出现下降,且当宽度为500~600 mm时,斜裂缝不再变化,跨中最大挠度的下降速率有所降低。这是因为,板宽度的增大使横截面惯性矩成倍增大,从而导致截面抗弯性能增强,但在此宽度范围内,板出现纵向的轻微弯曲,对横向弯曲变形起到了抑制作用。单向板宽度的增大使靶板的受力面积增大,从而导致迎爆面和背爆面的破坏范围也有所增大,这不足以说明板的毁伤程度增大。综合考虑,UHPC单向板的宽度变化与构件破坏程度成负相关。由图12(c)可知,当单向板厚度增大时,各项破坏指标均大幅度降低。当厚度为180~240 mm时,最大挠度的下降速率变缓,斜裂缝长度的下降速率变大。可以看出,截面的惯性矩呈指数形式增长,大幅度提高了横截面的抗弯刚度,从而减小跨中挠度。而增大UHPC单向板厚度可以有效抑制爆炸工况下的斜裂缝扩展现象。对3种尺寸效应进行横向对比可以发现,相比迎爆面,单向板的背爆面毁伤面积对尺寸变化更敏感,这从侧面反映了UHPC抗拉、压能力的不同。在3种尺寸效应中,通过各指标变化范围与尺寸变化之比来衡量尺寸效应的影响程度,容易看出,计算结果对厚度变化的敏感程度最高,对长度次之,对宽度变化的敏感程度最低。
4. 结 论
基于超高性能混凝土力学试验及单向板数值模拟分析,得出以下主要结论。
(1) 得到一组超高性能混凝土的HJC本构模型参数。通过与超高性能混凝土单向板爆炸试验结果进行对比,验证了参数的有效性。
(2) 在临空板爆炸工况下,提高超高性能混凝土单向板的主筋配筋率,可以有效降低板的跨中最大挠度,并且可以促进箍筋的作用效果。加密箍筋可以提高单向板的抗剪承载力,抑制斜裂缝扩展,对跨中挠度的减小也有一定效果。在配筋时,单向板上部纵筋和箍筋可采用低级别钢筋,底部纵筋宜采用较高级钢筋。
(3) 在临空板爆炸工况下,超高性能混凝土单向板有明显的尺寸效应。其中毁伤程度随板长度增大而升高,随板宽度和厚度增大而降低。在不同尺寸效应中,爆炸计算结果对单向板厚度变化最敏感,对长度次之,对宽度变化的敏感程度最低。
-
表 1 不同应变率下的UHPC力学参数
Table 1. UHPC mechanical parameters under different strain rates
应变率/s−1 抗压强度/MPa ¯σ ¯p 10−4 105.0 1.000 0 0.333 3 10−2 113.3 1.079 0 0.359 7 50 134.6 1.281 9 0.427 3 102 164.7 1.568 6 0.522 9 表 2 超高性能混凝土HJC模型参数
Table 2. HJC model parametrs of UHPC
A B N C T/MPa Sfmax εefmin D1 D2 fs ˙ε0/s−1 0.232 8 1.744 3 0.705 1 0.003 6 7.12 7.0 0.018 1 0.04 1.0 0.1725 1 pc/MPa pl/MPa μc μl K1/GPa K2/GPa K3/GPa σc/MPa G/GPa ρ/(g·cm−3) 35.0 235.0 0.0011 0.0383 46.4 −195.0 416.6 105.0 20.37 2.67 表 3 钢筋本构模型参数
Table 3. Parameters of reinforcement constitutive models
材料 密度/(g·cm−3) 弹性模量/GPa 泊松比 屈服应力/MPa 切线模量/GPa 失效应变 受拉钢筋 7.85 200 0.25 524 1.61 0.10 箍筋 7.85 200 0.25 423 1.91 0.08 表 4 修正前的原始参数
Table 4. Original parameters before correction
A B N C T/MPa Sfmax εefmin D1 D2 fs ˙ε0/s−1 0.76 1.6 0.61 0.007 7.12 7.0 0.01 0.04 1.0 − 1 pc/MPa pl/MPa μc μl K1/GPa K2/GPa K3/GPa σc/MPa G/GPa ρ/(g·cm−3) 16.0 800.0 0.001 0.1 85.0 −171.0 208.0 105.0 20.37 2.67 表 5 单向板各方向尺寸变化
Table 5. Dimension change of one-way plate in each direction
长度/mm 宽度/mm 厚度/mm 1 200 400 120 1 500 500 180 1 800 600 240 -
[1] LI J, WU C Q, HAO H. An experimental and numerical study of reinforced ultra-high performance concrete slabs under blast loads [J]. Materials and Design, 2015, 82: 64–76. DOI: 10.1016/j.matdes.2015.05.045. [2] WANG Y Z, WANG Y B, ZHAO Y Z, et al. Experimental study on ultra-high performance concrete under triaxial compression [J]. Construction and Building Materials, 2020, 263: 120225. DOI: 10.1016/j.conbuildmat.2020.120225. [3] LI J, WU C Q, HAO H, et al. Experimental investigation of ultra-high performance concrete slabs under contact explosions [J]. International Journal of Impact Engineering, 2016, 93: 62–75. DOI: 10.1016/j.ijimpeng.2016.02.007. [4] 杜忠, 胡福. 高延性混凝土(HDC)在抗爆间室中的防护效果评估 [J]. 工程建设与设计, 2019(22): 9–10. DOI: 10.13616/j.cnki.gcjsysj.2019.11.204.DU Z, HU F. Evaluation of the protective effect of high-ductility concrete (HDC) in anti-explosion chamber [J]. Construction and Design for Engineering, 2019(22): 9–10. DOI: 10.13616/j.cnki.gcjsysj.2019.11.204. [5] 辛健. 爆炸作用下RHT模型参数敏感性分析 [J]. 舰船电子工程, 2019, 39(4): 111–113, 122. DOI: 10.3969/j.issn.1672-9730.2019.04.024.XIN J. Sensitivity analysis of RHT model parameters under explosive attack [J]. Ship Electronic Engineering, 2019, 39(4): 111–113, 122. DOI: 10.3969/j.issn.1672-9730.2019.04.024. [6] RIEDEL W, THOMA K, HIERMAIER S, et al. Penetration of reinforced concrete by BETA-B-500 numerical analysis using a new macroscopic concrete model for hydrocodes [C] // Proceeding of the 9th International Symposium, Interaction of the Effects of Munitions with Structures. Berlin: ISIEMS, 1999: 315–322. [7] MALVAR L J, CRAWFORD J E, WESEVICH J W, et al. A plasticity concrete material model for DYNA3D [J]. International Journal of Impact Engineering, 1997, 19(9/10): 847–873. DOI: 10.1016/S0734-743X(97)00023-7. [8] HOLMQUIST T J, JOHNSON G R, COOK W H. A computational constitutive model for concrete subjected to large strains, high strain rates and high pressures [C] // 14th International Symposium on Ballistics. Quebec: American Defense Preparedness Association, 1993: 591–600. [9] 杜闯, 宋帅, 张江鹏. 爆炸冲击作用下三种混凝土本构模型对比研究 [J]. 兵器装备工程学报, 2022, 43(11): 49–56. DOI: 10.11809/bqzbgcxb2022.11.007.DU C, SONG S, ZHANG J P. Comparative study on three concrete constitutive models under blast loading [J]. Journal of Ordnance Equipment Engineering, 2022, 43(11): 49–56. DOI: 10.11809/bqzbgcxb2022.11.007. [10] 张凤国, 李恩征. 混凝土撞击损伤模型参数的确定方法 [J]. 弹道学报, 2001, 13(4): 12–16, 23. DOI: 10.3969/j.issn.1004-499X.2001.04.003.ZHANG F G, LI E Z. A method to determine the parameters of the model for concrete impact and damage [J]. Journal of Ballistics, 2001, 13(4): 12–16, 23. DOI: 10.3969/j.issn.1004-499X.2001.04.003. [11] 韩莉, 吴萍. 动荷载作用下混凝土HJC本构模型 [J]. 绿色环保建材, 2018(11): 3–4. DOI: 10.16767/j.cnki.10-1213/tu.2018.11.002. [12] LIU Y, HUANG F L, MA A E. Numerical simulations of oblique penetration into reinforced concrete targets [J]. Computers and Mathematics with Applications, 2011, 61(8): 2168–2171. DOI: 10.1016/j.camwa.2010.09.006. [13] 张志刚, 李姝雅, 瘳红建. 爆炸荷载下碳纤维布加固混凝土板的抗弯性能研究 [J]. 应用力学学报, 2008, 25(1): 150–153.ZHANG Z G, LI S Y, LIAO H J. Flexural resistance of concrete plate strengthened with CFRP under explosive loading [J]. Chinese Journal of Applied Mechanics, 2008, 25(1): 150–153. [14] 张志华, 刘磊, 王亚, 等. 混凝土爆破试验及数值模拟 [J]. 矿冶, 2018, 27(6): 34–37. DOI: 10.3969/j.issn.1005-7854.2018.06.008.ZHANG Z H, LIU L, WANG Y, et al. Blasting experiment and numerical simulation of concrete [J]. Mining and Metallurgy, 2018, 27(6): 34–37. DOI: 10.3969/j.issn.1005-7854.2018.06.008. [15] 任根茂, 吴昊, 方秦, 等. 普通混凝土HJC本构模型参数确定 [J]. 振动与冲击, 2016, 35(18): 9–16. DOI: 10.13465/j.cnki.jvs.2016.18.002.REN G M, WU H, FANG Q, et al. Determinations of HJC constitutive model parameters for normal strength concrete [J]. Journal of Vibration and Shock, 2016, 35(18): 9–16. DOI: 10.13465/j.cnki.jvs.2016.18.002. [16] 陈睿, 刘杰, 韩旭, 等. 混凝土材料动态本构参数的分阶段计算反求技术 [J]. 爆炸与冲击, 2014, 34(3): 315–321. DOI: 10.11883/1001-1455(2014)03-0315-07.CHEN R, LIU J, HAN X, et al. A multi-stage computational inverse technique for identification of the dynamic constitutive parameters of concrete [J]. Explosion and Shock Waves, 2014, 34(3): 315–321. DOI: 10.11883/1001-1455(2014)03-0315-07. [17] 张社荣, 宋冉, 王超, 等. 碾压混凝土HJC动态本构模型修正及数值验证 [J]. 振动与冲击, 2019, 38(12): 25–31. DOI: 10.13465/j.cnki.jvs.2019.12.004.ZHANG S R, SONG R, WANG C, et al. Modification of a dynamic constitutive model—HJC model for roller-compacted concrete and numerical verification [J]. Journal of Vibration and Shock, 2019, 38(12): 25–31. DOI: 10.13465/j.cnki.jvs.2019.12.004. [18] 熊益波, 陈剑杰, 胡永乐, 等. 混凝土Johnson-Holmquist本构模型关键参数研究 [J]. 工程力学, 2012, 29(1): 121–127.XIONG Y B, CHEN J J, HU Y L, et al. Study on the key parameters of the Johnson-Holmquist constitutive model for concrete [J]. Engineering Mechanics, 2012, 29(1): 121–127. [19] 李鹏. 钢管混凝土柱爆炸作用下试验及动态本构模型研究 [D]. 广州: 广州大学, 2016: 64–71.LI P. Test and dynamic constitutive model study of CFST column under blast load [D]. Guangzhou, Guangdong, China: Guangzhou University, 2016: 64–71. [20] 张若棋, 丁育青, 汤文辉, 等. 混凝土HJC、RHT本构模型的失效强度参数 [J]. 高压物理学报, 2011, 25(1): 15–22. DOI: 10.11858/gywlxb.2011.01.003.ZHANG R Q, DING Y Q, TANG W H, et al. The failure strength parameters of HJC and RHT concrete constitutive models [J]. Chinese Journal of High Pressure Physics, 2011, 25(1): 15–22. DOI: 10.11858/gywlxb.2011.01.003. [21] DU Y X, WEI J, LIU K, et al. Research on dynamic constitutive model of ultra-high performance fiber-reinforced concrete [J]. Construction and Building Materials, 2020, 234: 117386. DOI: 10.1016/j.conbuildmat.2019.117386. [22] 过镇海, 王传志. 多轴应力下混凝土的强度和破坏准则研究 [J]. 土木工程学报, 1991, 24(3): 1–14. DOI: 10.15951/j.tmgcxb.1991.03.001.GUO Z H, WANG C Z. Investigation of strength and failure criterion of concrete under multi-axial stresses [J]. China Civil Engineering Journal, 1991, 24(3): 1–14. DOI: 10.15951/j.tmgcxb.1991.03.001. [23] 仵鹏涛. 三向应力状态下超高性能混凝土材料静态力学性能研究 [D]. 天津: 天津大学, 2019: 40–44. DOI: 10.27356/d.cnki.gtjdu.2019.004796.WU P T. Study of static mechanical properties of ultra-high performance concrete under triaxial stress states [D]. Tianjin, China: Tianjin University, 2019: 40–44. DOI: 10.27356/d.cnki.gtjdu.2019.004796. [24] 薛文. 落锤冲击作用下RC梁的动力响应研究 [D]. 武汉: 华中科技大学, 2016: 42–54.XUE W. Study on dynamic response of RC beams under drop hammer impact [D]. Wuhan, Hubei, China: Huazhong University of Science and Technology, 2016: 42–54. [25] 王志亮, 毕程程, 李鸿儒. 混凝土爆破损伤的SPH-FEM耦合法数值模拟 [J]. 爆炸与冲击, 2018, 38(6): 1419–1428. DOI: 10.11883/bzycj-2017-0209.WANG Z L, BI C C, LI H R. Numerical simulation of blasting damage in concrete using a coupled SPH-FEM algorithm [J]. Explosion and Shock Waves, 2018, 38(6): 1419–1428. DOI: 10.11883/bzycj-2017-0209. [26] 陈星明, 刘彤, 肖正学. 混凝土HJC模型抗侵彻参数敏感性数值模拟研究 [J]. 高压物理学报, 2012, 26(3): 313–318. DOI: 10.11858/gywlxb.2012.03.011.CHEN X M, LIU T, XIAO Z X. Numerical simulation study of parameter sensitivity analysis on concrete HJC model [J]. Chinese Journal of High Pressure Physics, 2012, 26(3): 313–318. DOI: 10.11858/gywlxb.2012.03.011. [27] 汪衡, 董静, 顾振中, 等. HJC模型参数对侵彻效应影响度的数值研究 [J]. 兵器装备工程学报, 2020, 41(3): 200–204. DOI: 10.11809/bqzbgcxb2020.03.040.WANG H, DONG J, GU Z Z, et al. Numerical study on the effect of HJC model parameters on penetration [J]. Journal of Ordnance Equipment Engineering, 2020, 41(3): 200–204. DOI: 10.11809/bqzbgcxb2020.03.040. [28] 胡志豪. 超高性能混凝土的气体渗透性能研究 [D]. 长沙: 湖南大学, 2021: 49–51. DOI: 10.27135/d.cnki.ghudu.2021.001461.HU Z H. Experimental study on gas permeability of ultra-high performance concrete [D]. Changsha, Hunan, China: Hunan University, 2021: 49–51. DOI: 10.27135/d.cnki.ghudu.2021.001461. [29] 王政. 弹靶侵彻动态响应的理论与数值分析 [D]. 上海: 复旦大学, 2005: 39–40. [30] BEISSEL S R, JOHNSON G R. An abrasion algorithm for projectile mass loss during penetration [J]. International Journal of Impact Engineering, 2000, 24(2): 103–116. DOI: 10.1016/S0734-743X(99)00146-3. [31] 严少华, 钱七虎, 周早生, 等. 高强混凝土及钢纤维高强混凝土高压状态方程的实验研究 [J]. 解放军理工大学学报, 2000, 1(6): 49–53. DOI: 10.7666/j.issn.1009-3443.20000610.YAN S H, QIAN Q H, ZHOU Z S, et al. Experimental study of equation of state for high-strength concrete and high-strength fiber concrete [J]. Journal of PLA University of Science and Technology, 2000, 1(6): 49–53. DOI: 10.7666/j.issn.1009-3443.20000610. [32] 孙玉祥, 王杰, 武海军, 等. 混凝土高压状态方程实验与数值模拟研究 [J]. 爆炸与冲击, 2020, 40(12): 121401. DOI: 10.11883/bzycj-2020-0002.SUN Y X, WANG J, WU H J, et al. Experiment and simulation on high-pressure equation of state for concrete [J]. Explosion and Shock Waves, 2020, 40(12): 121401. DOI: 10.11883/bzycj-2020-0002. [33] 高乐. 活性粉末混凝土高压状态方程研究 [D]. 广州: 广州大学, 2011: 51–53.GAO L. Research on high pressure equation of RPC [D]. Guangzhou, Guangdong, China: Guangzhou University, 2011: 51–53. [34] 唐鸽, 江少恩, 巫顺超, 等. 用Hugoniot数据计算高压状态方程 [J]. 强激光与粒子束, 2009, 21(11): 1737–1740.TANG G, JIANG S E, WU S C, et al. Deducing equation of state under high pressure from Hugoniot data [J]. High Power Laser and Particle Beams, 2009, 21(11): 1737–1740. [35] 蒋国平, 焦楚杰, 肖波齐. 高强混凝土气体炮试验与高压状态方程研究 [J]. 物理学报, 2012, 61(2): 026701. DOI: 10.7498/aps.61.026701.JIANG G P, JIAO C J, XIAO B Q. High-pressure state equation of high strength concrete investigated with the gas gun experiment [J]. Acta Physica Sinica, 2012, 61(2): 026701. DOI: 10.7498/aps.61.026701. [36] 孙其然, 李芮宇, 赵亚运, 等. HJC模型模拟钢筋混凝土侵彻实验的参数研究 [J]. 工程力学, 2016, 33(8): 248–256. DOI: 10.6052/j.issn.1000-4750.2014.12.1094.SUN Q R, LI R Y, ZHAO Y Y, et al. Investigation on parameters of HJC model applied to simulate perforation experiments of reinforced concrete [J]. Engineering Mechanics, 2016, 33(8): 248–256. DOI: 10.6052/j.issn.1000-4750.2014.12.1094. [37] 林琛, 徐建军, 杨晋伟, 等. 基于HJC模型的钢筋混凝土侵彻仿真失效准则与参数 [J]. 探测与控制学报, 2017, 39(2): 100–105.LIN C, XU J J, YANG J W, et al. The failure criterions and parameters of HJC model based perforation simulation [J]. Journal of Detection and Control, 2017, 39(2): 100–105. [38] 石少卿, 康建功, 汪敏, 等. ANSYS/LS-DYNA在爆炸与冲击领域内的工程应用 [M]. 北京: 中国建筑工业出版社, 2011. [39] 汪维, 张舵, 卢芳云, 等. 方形钢筋混凝土板的近场抗爆性能 [J]. 爆炸与冲击, 2012, 32(3): 251–258. DOI: 10.11883/1001-1455(2012)03-0251-08.WANG W, ZHANG D, LU F Y, et al. Anti-explosion performances of square reinforced concrete slabs under close-in explosions [J]. Explosion and Shock Waves, 2012, 32(3): 251–258. DOI: 10.11883/1001-1455(2012)03-0251-08. [40] LIN X S. Numerical simulation of blast responses of ultra-high performance fibre reinforced concrete panels with strain-rate effect [J]. Construction and Building Materials, 2018, 176: 371–382. DOI: 10.1016/j.conbuildmat.2018.05.066. 期刊类型引用(10)
1. 张鑫,刘泽功,常帅,陈响升,薛勇林,朱家亮,宋鑫. 爆破荷载作用下煤岩本构模型参数特性研究. 振动与冲击. 2025(05): 263-277 .  百度学术
百度学术2. 楼晓明,林杰,王新龙,刘怀宇,王广. 球面爆轰波下不同孔径耦合装药孔壁压力沿炮孔轴向分布特征. 哈尔滨工业大学学报. 2025(03): 148-159 .  百度学术
百度学术3. 张旭,刘晓辉,刘楚佳,罗盈. 高温大理岩的动态能量耗散机理及破坏特征. 爆炸与冲击. 2025(06): 68-80 .  本站查看
本站查看4. 王子琛. 白云石大理岩HJC本构参数确定及应用. 科技创新与应用. 2024(11): 72-75+80 .  百度学术
百度学术5. 谢全敏,刘文文,晏理想. 某大型深埋罐室围岩和混凝土试验及本构模型研究. 武汉理工大学学报. 2024(03): 48-55 .  百度学术
百度学术6. 骆柯,牛雷雷,朱万成,王聪,赵蔚. 不同冻结状态下含单裂隙岩石动态抗拉特性研究. 有色金属(矿山部分). 2024(04): 124-132+141 .  百度学术
百度学术7. 宁鹏博,刘军,赵硕,李瑶. 钢纤维增强混凝土动态力学性能及HJC本构模型参数标定. 中国建材科技. 2024(03): 20-25 .  百度学术
百度学术8. 漆涛,陶铁军,田兴朝,谢财进,万安桐,张厚英. 大断面隧道“楔形掏槽+高能孔”布设方法研究. 爆破. 2024(03): 95-103+120 .  百度学术
百度学术9. 李干,李杰,宋春明,李孝臣,王明洋. 花岗岩的动态力学性能、本构模型与状态方程研究. 力学与实践. 2023(05): 952-959 .  百度学术
百度学术10. 朱明,宫能平,穆朝民,蔡天宇,章雨. 高温后钢纤维自密实混凝土动、静态力学性能研究. 硅酸盐通报. 2023(11): 3895-3905 .  百度学术
百度学术其他类型引用(9)
-












 下载:
下载:


























 下载:
下载:


 百度学术
百度学术










