Size distribution characteristics of blast-induced rock fragmentation under decoupled charge structures
-
摘要: 通过不同装药结构下立方体红砂岩小型爆破实验,分析了岩石的损伤程度和破坏模式,同时引入三参数极值分布函数量化岩石爆破块体尺寸分布特征。此外,根据岩样R1的爆破实验结果进行了有限元数值模型验证,基于验证的模型展开了岩石单孔爆破损伤破裂行为的模拟,讨论了径向、轴向不耦合系数和耦合介质对岩石破碎效果的影响。结果表明,三参数极值分布函数可以较好地表征岩石爆破后破碎块体尺寸分布特征,块体平均尺寸随着不耦合系数的减小呈线性降低趋势,且破碎块度趋于均匀。通过比较不同耦合介质装药时岩石内部的能量分布特征和破坏体积发现,水作为耦合介质时,爆炸能量的传递效率最高,其次分别是湿砂和干砂,空气的能量传递效率最低。结合等效波阻法计算的理论应力透射系数可以很好地反映不耦合装药时岩石的破碎程度。Abstract: Decoupled charge structure is widely used in contour blasting for rock excavation engineering, and its efficacy in rock breaking is tied intricately to both the decoupling ratio and the transfer features of explosion energy. In this study, the analysis delves into the damage degree and failure patterns of cubic red sandstone samples through two groups of lab-scale blasting tests utilizing various charging modes. To precisely quantify the features of rock fragmentation size distribution (FSD) induced by blasting load, a three-parameter generalized extreme value (GEV) function was introduced. In addition, a three-dimensional finite element model was developed in ANSYS software. The numerical model was calibrated based on the tested results of sample R1 by comparing the fracture networks and FSD curves. This validated model was then deployed to model the rock fracture behavior under decoupled charge blasting, and the evolution of blasting cracks and explosion pressure inside the rock sample was reproduced. Moreover, the effects of axial and radial decoupled ratios and the choice of coupling medium on the rock fragmentation and fracture patterns were discussed. The results showed that the three-parameter GEV function can better characterize the rock fragmentation features resulting from blasting. Notably, the average size of the fragment decreases linearly with the decrease of the decoupling ratio, and the degree of fragmentation tends to be uniform. By comparing the energy distribution and damage levels of rock when using different coupling mediums, it was found that water as the coupling medium exhibits the highest efficiency in energy transfer, followed by wet sand and dry sand, and air has the lowest energy transfer efficiency. Furthermore, the theoretical stress transmission coefficient calculated by the equivalent wave impedance method can well reflect the rock fragmentation features and serve as a valuable reference for rock blasting in decoupled charge.
-
钻孔爆破作为岩体开挖的重要方法,被广泛应用于隧道掘进、水利建设和矿山爆破等众多工程领域[1-3]。轮廓控制爆破工程中常采用不耦合装药结构,在药卷和炮孔之间留有一定空隙,空隙中充填不同的耦合介质,以实现预期的爆破效果。轮廓控制爆破的关键是保护开挖面,因此,通常采用低爆速和低威力的炸药,以减弱对炮孔壁面的冲击压力[4-5]。生产实践中多依据经验进行轮廓爆破方案设计,不合理的装药结构常常会导致岩体超挖或欠挖,进而影响施工成本和施工进度。为了优化轮廓控制爆破方案,深入了解不耦合装药下岩石爆破块体的尺寸分布特征显得尤为重要。
对不耦合装药条件下岩石爆破的动态响应,已开展了大量理论、实验及数值模拟方面的研究。其中最早的研究可追溯至20世纪中期,Langefors等[6]开展的不耦合装药结构下岩体预裂爆破实验。20世纪60年代初期,Paine等[7]将不耦合装药爆破技术大规模运用到Niagara水电站建设中,通过该方法不仅提高了工作效率,还解决了岩体的超/欠挖问题。此后,不耦合装药控制爆破技术在英、美等发达国家得到了广泛的应用。与此同时,中国的水利建设部门及矿山企业也开展了一些不耦合装药爆破的实验研究,并于20世纪70年代初期,成功运用到葛洲坝水电站的建设工程中[8-9]。在随后的水利及矿业工程建设中,不耦合装药爆破技术得到了进一步的推广与应用。工程实际应用离不开基础理论的研究工作,近些年来对于不耦合装药爆破的探索也是日趋深入。王伟等[10]通过室外爆破实验研究了不耦合装药条件下爆炸应力波的传播规律,发现水介质不耦合装药结构可以减少爆炸能量的耗散,提高能量的传递效率,从而实现更好的爆破效果。李桐等[11]在考虑岩石应变率效应的基础上,针对不同耦合介质材料,从理论方面分析了岩石在爆破作用下的变形破坏特征,并得到爆炸能量传递效率的理论解。Chi等[12]利用应变片和高速摄影仪分析了岩石爆破过程中环向应变的变化规律,讨论了不同耦合介质对岩石爆破破碎效果的影响。Yang等[13]通过高速摄影仪并结合数字图像相关技术,研究了不耦合装药时爆炸应力波的衰减规律,发现爆炸应力波衰减指数随着不耦合系数的增大呈现先增大后减小的变化趋势。
岩石不耦合装药爆破是一个较复杂的动力学问题,材料表面的变形破坏过程可通过高速摄影仪监测,但其内部的损伤演化行为却很难通过现有的实验手段进行分析。近些年来,随着数字计算技术的飞速发展,数值模拟成为研究岩石动力学不可或缺的方法之一[14-15]。王志亮等[16]基于显式示动力有限元程序LS-DYNA中的Johnson-Holmquist-Concrete (JHC)模型,针对水不耦合装药条件下混凝土爆破进行数值计算,分析了不耦合系数对孔壁压力和损伤破坏范围的影响,并指出水不耦合系数取3.0时爆炸能量利用率更高。Zhu等[17]利用有限元差分程序AUTODYN对岩石在不同耦合介质装药下的破裂过程进行了模拟,通过改进的主应力失效准则实现了剪切、压缩和拉伸破坏类型的识别,进而揭示了岩体在动态荷载下的断裂和破碎机制。Yuan等[18]基于颗粒流离散元(particle flow code, PFC)程序中的平行黏结模型,模拟了岩石类材料水压爆破的破坏特征,结合能量守恒定律分析了爆炸应力波在界面处的传播规律,讨论了爆炸冲击波和爆生气体对爆破裂纹传播的影响。袁增森等[19]在考虑爆炸冲击波和爆生气体共同作用的基础上,通过动-静荷载混合施加的方法进行离散元爆破数值模拟,并建立了岩石爆破损伤程度随装药不耦合系数变化的预测模型。
上述研究为不耦合装药下岩石爆破提供了诸多思路,在前人研究的基础上,本文中将进一步展开不耦合装药下岩石爆破块体尺寸分布特征的研究;在2组红砂岩小型爆破实验后,收集并筛分岩石爆破后的块体、碎片、颗粒和粉末,进而获取岩石在不同装药结构下的损伤程度和破坏模式,引入三参数极值分布函数定量表征岩石爆破后破碎块体的尺寸分布(fragmentation size distribution, FSD)特征;此外,根据岩样R1的爆破实验结果,对有限元数值模型进行参数验证,基于验证的模型对不同装药结构下岩石内部的压力演化过程和损伤破裂行为进行数值模拟;将数值模拟获取的岩石损伤云图进行图像处理,以定量分析不同耦合介质、不耦合系数和不耦合结构下岩石的破碎效果,结合应力透射系数解释爆炸能量的传递规律,以期为不耦合装药岩石爆破提供新的认识。
1. 岩石小型爆破实验
1.1 实验设置
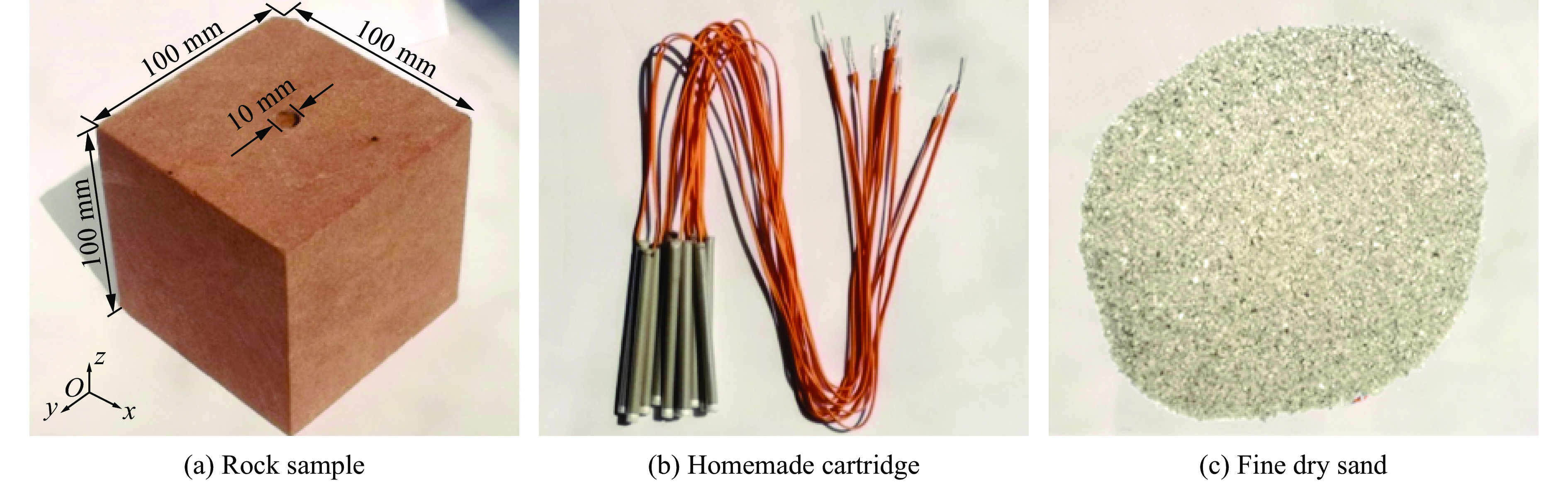
为了尽量减小岩样之间的性质差异,爆破实验中选用的红砂岩试样均取自同一块母岩。所选用的岩样具有相对均匀的粒度,且每个试样的表面均无明显裂缝或缺陷。从母岩中获取岩芯后,对其进行切割和抛光,加工成边长100 mm的立方体试样。红砂岩室内爆破实验准备如图1所示,在试样中心钻取不同直径的炮孔用于装填炸药,典型的试样如图1(a)所示。此外,使用波速仪对每个试样的纵波波速进行测量,若其纵波波速偏离平均值超过3%,则不选用该试样。通过室内实验,分别测得岩石的物理参数:密度ρr为2 360 kg/m3,横波速度cs为2 710 m/s,孔隙率α为0.12;力学参数:单轴抗压强度fc为21.6 MPa,抗拉强度ft为2.1 MPa,抗剪强度fs为9.7 MPa,杨氏模量E为12.53 GPa,泊松比μ为0.23。
考虑到红砂岩的强度较低,选用爆压和爆速均相对较低的黑火药作为爆源。在保证炸药性质与岩石力学特性匹配的同时,也有利于实验安全进行。首先将火药粉末放置在塑料管内并不断压实,然后将电子引线端头放置在塑料管顶端,最后进行密封处理。塑料管外径约为4 mm,其厚度较小,可忽略不计,如图1(b)所示。黑火药的密度为790 kg/m3,爆轰温度为2 500 °C,爆炸热量为3015 kJ/kg,爆轰气体体积为280 L/kg,爆速在300~450 m/s之间[20]。将自制的药卷放置在炮孔中心,在炮孔上下两端用细砂填塞,并用胶水封堵,细砂粒径在0.1~0.8 mm之间,如图1(c)所示。
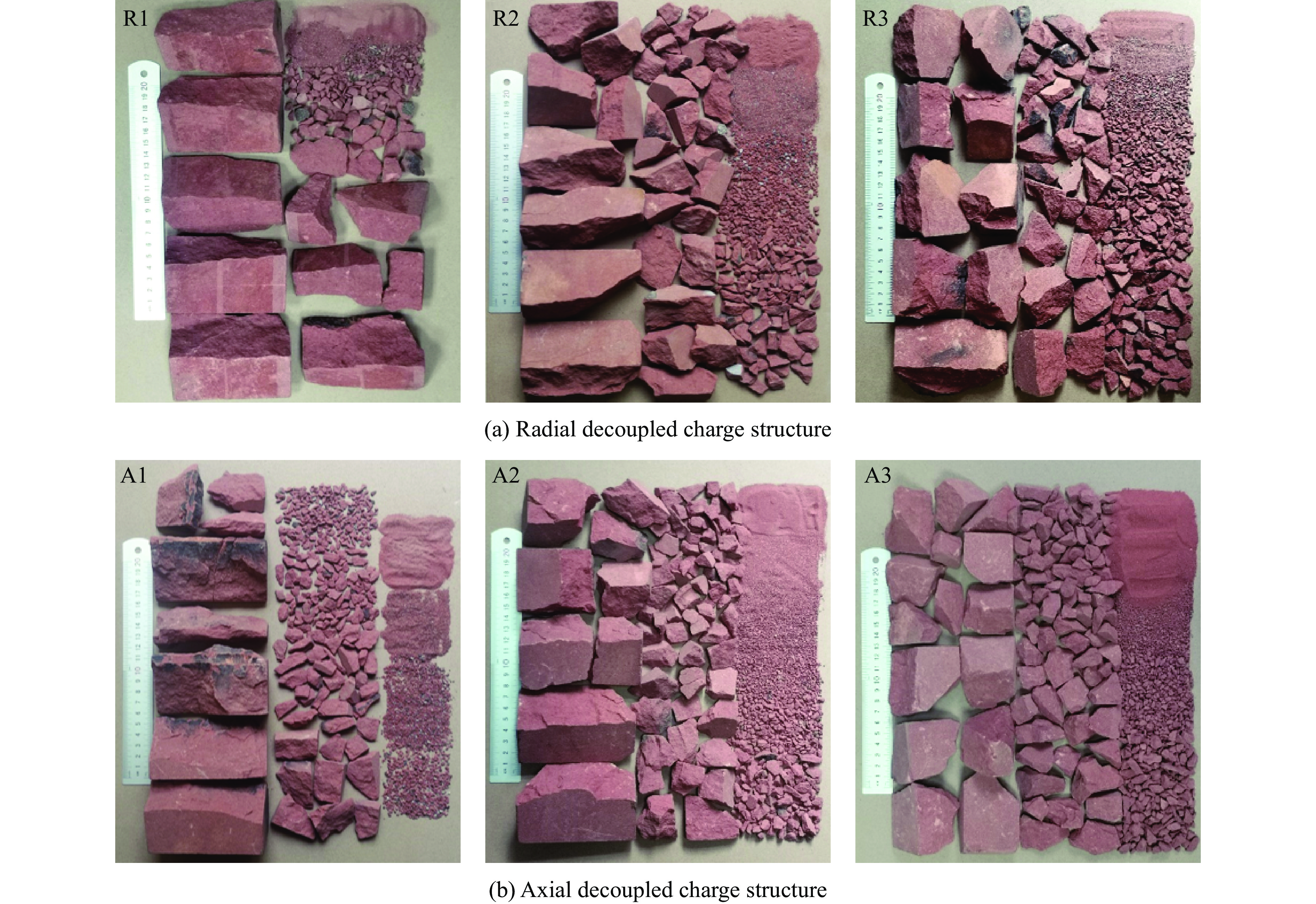
根据装药结构可将岩石小型爆破实验分为2组:径向不耦合装药组和轴向不耦合装药组。将径向不耦合系数Kr定义为:Kr = Db/De,其中De和Db分别为装药直径和炮孔直径。将轴向不耦合系数Ka定义为:Ka=Hair/He,其中Hair和He分别为空气层高度和装药高度。为比较装药结构对岩石爆破损伤的影响,在每个试样的炮孔内装有相同的药量。对每个试样进行编号分类后,测量和记录了各试样及炮孔的几何尺寸,如表1所示。
表 1 试样尺寸及装药结构Table 1. Samples dimensions and charge structures试样 试样长度/mm 试样宽度/mm 试样高度/mm 炮孔直径/mm 不耦合系数 装药结构 R1 100.29 100.34 100.37 12.00 3.0 径向不耦合 R2 100.38 99.81 99.73 10.00 2.5 R3 100.02 100.53 100.52 8.00 2.0 A1 99.81 100.14 100.12 10.00 3.0 轴向不耦合 A2 100.31 100.27 99.77 10.00 2.5 A3 99.63 99.86 100.11 10.00 2.0 1.2 实验结果
将爆破后的岩块整理收集起来,使用不同孔径的网筛进行筛分,筛孔直径在0.08~16.00 mm范围内,其中较大的岩块使用钢尺测量其尺寸。根据岩块的尺寸,从大到小依次排列摆放,最终获得不同装药条件下岩石的破坏模式,如图2所示。径向不耦合装药方式下岩石的破坏模式如图2(a)所示,试样R1爆破后主要破裂成6个大块,与4个中等尺寸的块体拼接后可以得到近乎完整的岩样,该试样用于后续的数值模型验证。试样R2爆破后破裂成4个大块和4个中等尺寸的块体,小块岩石相较于试样R1明显增多。试样R3爆破后仅有1个大块,主要以中等尺寸的块体为主,岩石颗粒和粉末相较于试样R1和R2显著增多。随着径向不耦合系数的减小,岩石的破碎程度逐渐加剧,且岩块的尺寸分布更加均匀。轴向不耦合装药条件下岩石的破坏模式如图2(b)所示,与径向不耦合装药类似,岩石大块数量随轴向不耦合系数的减小而减少,且中等尺寸与小块岩石的数量也有显著增加。相较于径向不耦合装药,轴向不耦合装药下岩石的破碎块度更均匀,在不耦合系数较小时最显著。
上述分析仅限于对岩石破坏程度的定性比较,为了量化表征岩石的爆破破碎效果,引入了三参数极值分布函数用于描述爆破块体的尺寸分布特征[21],其表达式如下:
F(d;ω,ψ,ξ)=exp{−[1+ξ(d−ωψ)]−1/−1ξξ} (1) 式中:d为岩石的爆破块体尺寸;ω为位置参数,通常被用作表征破碎块体的平均尺寸;ψ为尺度参数,该参数决定了破碎块体尺寸的分布范围;ξ为破碎块体的形状参数。
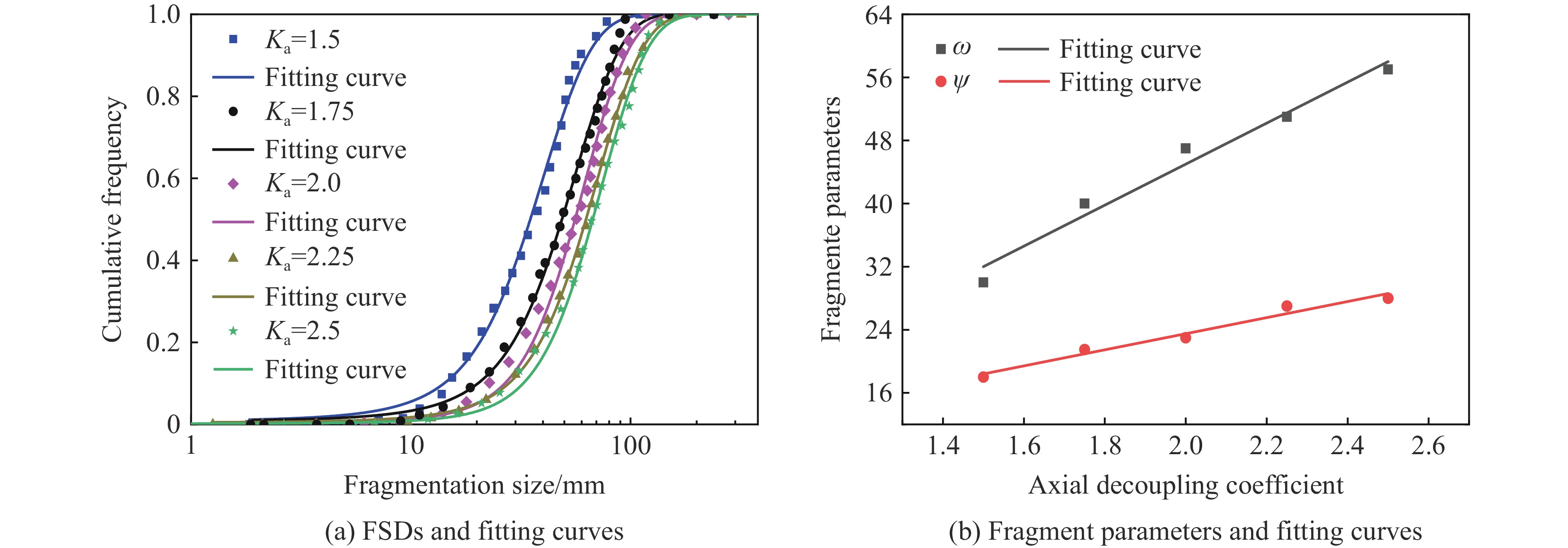
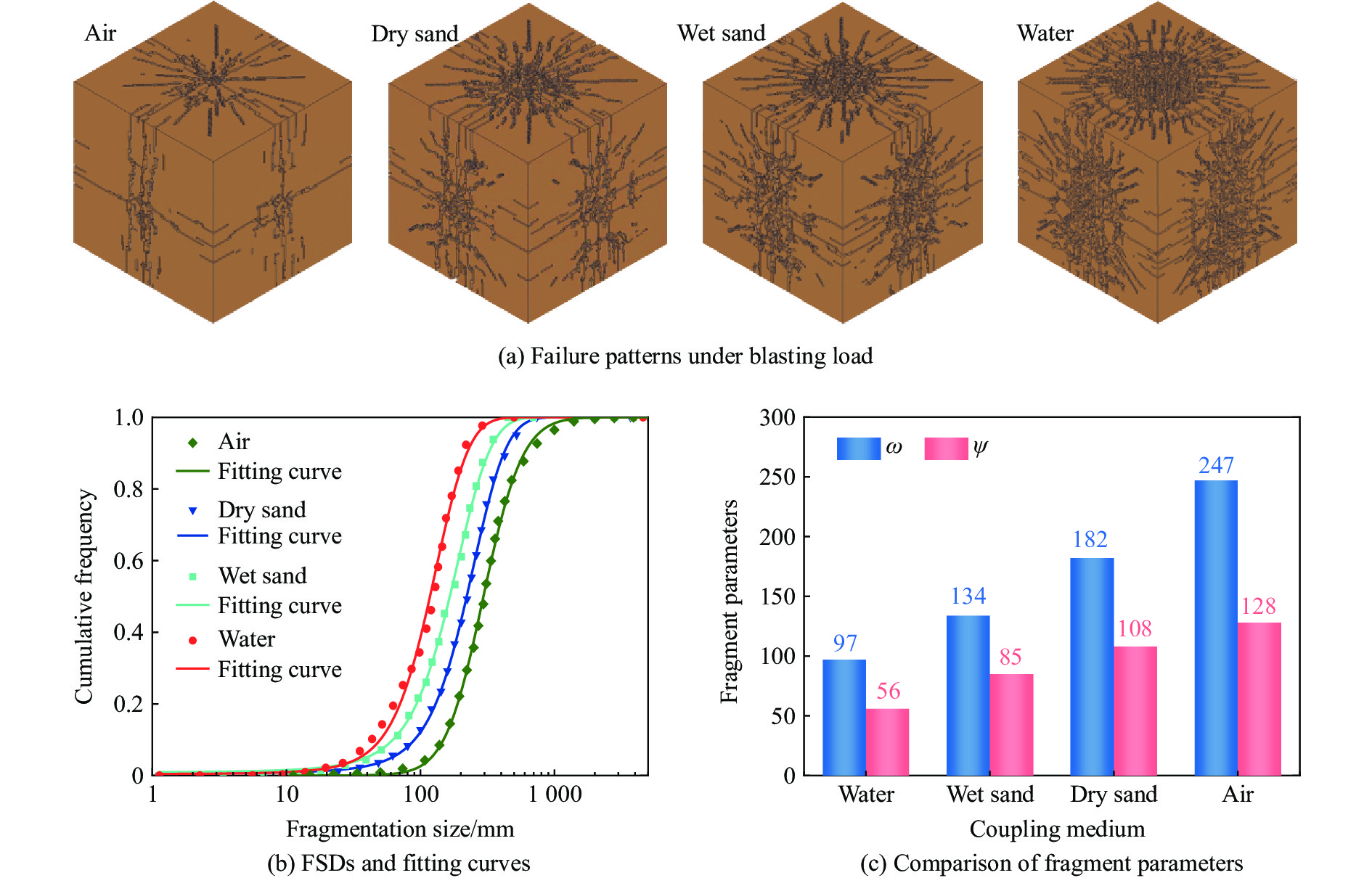
图3给出了不同装药条件下岩石爆破后的块体尺寸分布,散点表示实验结果,实线为三参数极值分布函数拟合结果,由拟合曲线的决定系数可知,该函数与实验结果匹配性较好。径向不耦合装药条件下块体的尺寸分布特征如图3(a)所示,参数ω和ψ随径向不耦合系数的增大逐渐增大,且呈线性正相关变化。轴向不耦合装药条件下,参数ω和ψ与径向不耦合装药条件下的变化规律类似,如图3(b)所示。即随着轴向不耦合系数的增大,破碎块体的平均尺寸和分布范围逐渐增大。结合参数ω、ψ的变化规律和实验结果发现,三参数极值分布函数可较好地描述岩石的破碎块体尺寸分布特征。
2. 数值模型验证
岩石爆破实验可控性较差,且岩石变形破坏过程难以捕捉,为深入分析不耦合装药下岩石的爆破损伤机理,有必要引入数值模拟方法。利用显式动力学有限元程序LS-DYNA对不同装药条件下岩石的破裂行为进行数值模拟,为岩石爆破块体尺寸分布规律研究提供了新的研究思路。
2.1 岩石材料参数
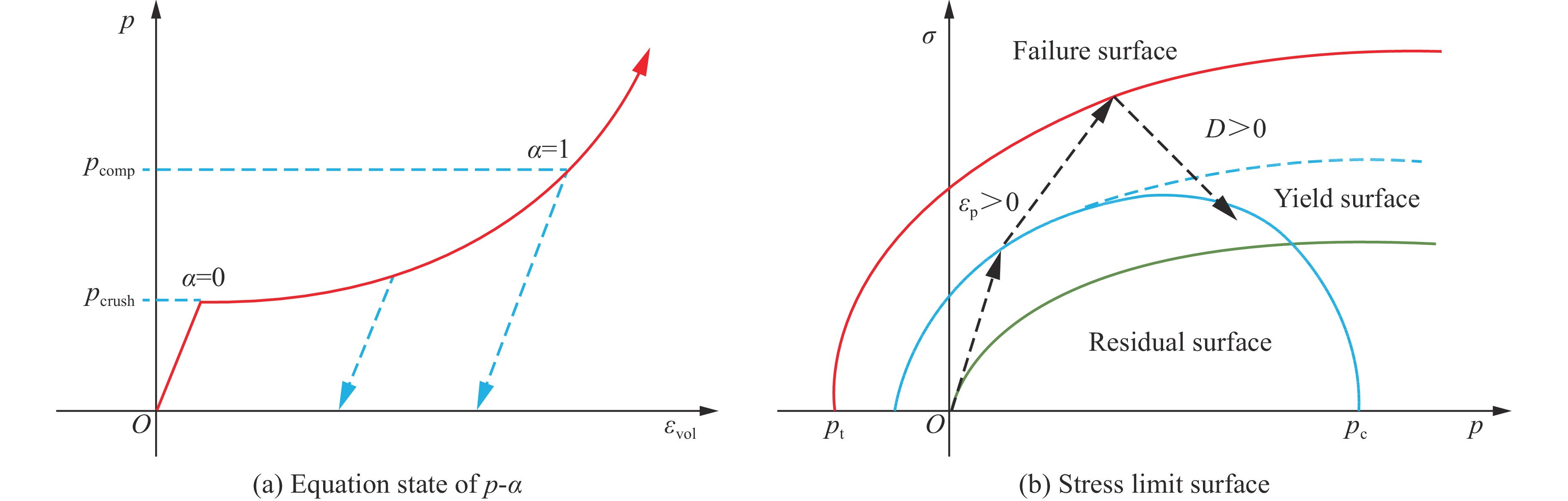
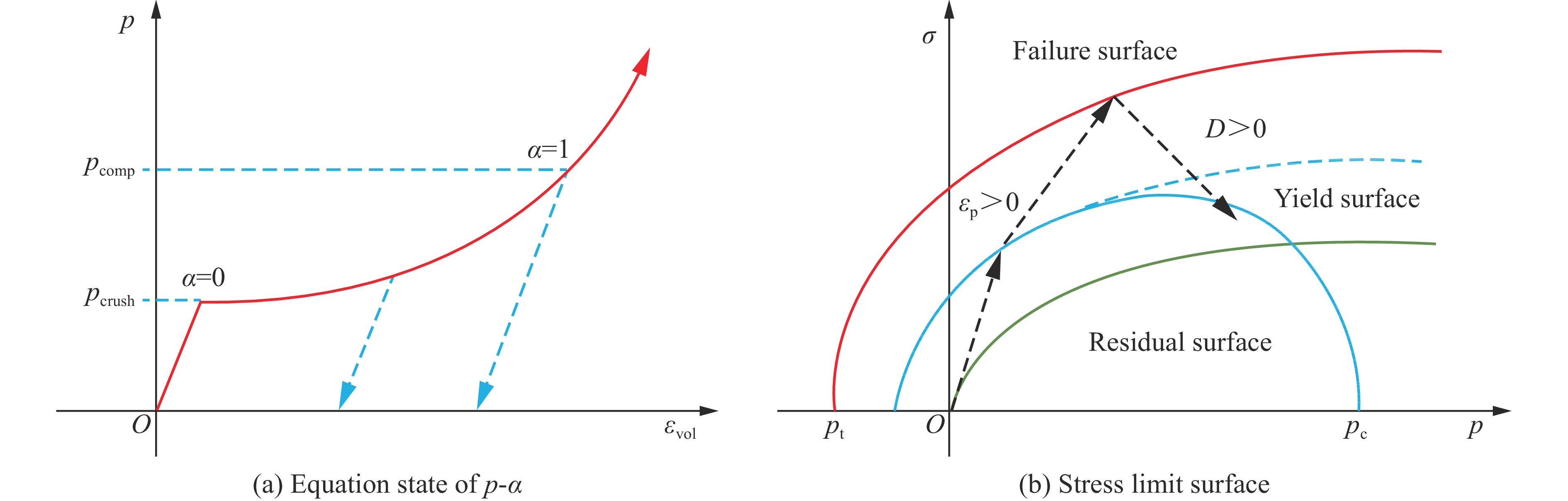
Riedel-Hiermaier-Thoma (RHT)拉压损伤模型中充分考虑了岩石材料的应变率效应,可以较好地反映岩石的动态力学响应特征[22-24],因此,该模型被广泛应用于模拟岩石的爆炸损伤破裂过程,如图4[25]所示。在孔隙压缩模型中,孔隙度α随压力p的增大而减小,在孔隙坍塌压力pcrush和孔隙压实压力pcomp处,α分别取最小值0和最大值1,如图4(a)所示。当p < pcrush时,模型为线弹性,而当p > pcrush时,孔隙压缩导致材料的体积刚度和有效体积模量降低,模型表现为非线性。若压力在pcrush和pcomp之间卸载时,卸载曲线将沿卸载点平行线弹性阶段发展,此时产生的体积应变εvol无法恢复。
不同加载阶段材料强度的变化特征可以通过3个应力极限面(弹性屈服面、极限破坏面和残余强度面)描述,典型的加载路径如图4(b)所示,其中pt和pc分别表示材料处于拉伸应力状态和压缩应力状态。材料在到达弹性屈服面前表现为线弹性变形,当材料所受压力超出弹性屈服面后,材料表现为塑性变形,塑性应变不断累积,即εp>0。当材料加载至极限破坏面时,塑性应变引起的损伤开始累积,即D>0。损伤累积到一定程度时材料发生软化,降低至残余强度面,持续加载后最终完全破坏,即D=1。材料的损伤演化方程定义为:
D=∑(Δεp/Δεpεfpεfp) (2) 式中:Δεp为累积塑性应变,
εfp 为材料破坏时的塑性应变。εfp 可由下式求得:εfp={D1[p∗−(1−D)p*t]D2p∗≥(1−D)p*t+(εmp/D1)1/D2εmpp∗<(1−D)p*t+(εmp/D1)1/D2 (3) 式中:D1和D2为损伤因子,分别取值0.04和1.0;p*为归一化压力,且p*=p/fc;
εmp 为最小损伤残余应变,p∗t 为破坏截止压力。RHT模型共包含38个参数,这些参数通常需要通过实验和理论公式进行确定,参数的标定方法可以参考文献[26],本文中使用的红砂岩RHT模型参数如表2所示。
表 2 岩石RHT模型的材料参数Table 2. Material parameters of the rock RHT model参数名称 符号 值 来源 参数名称 符号 值 来源 密度/(kg·m−3) ρr 2360 实验测定 残余面参数 Af 1.62
试错法初始孔隙度 αr0 1.12 残余面参数 Nf 0.61 抗压强度/MPa fc 21.6 压缩屈服面参数 G∗c 0.53 压缩应变率指数 βc 0.047 理论计算 拉伸屈服面参数 G∗t 0.70 拉伸应变率指数 βt 0.048 最小损伤残余应变 εmp 0.01 状态方程参数/GPa T1 17.33 孔隙压实压力/GPa pcomp 6.00 Hugoniot多项式系数/GPa A1 17.33 损伤因子 D1 0.04 默认取值 Hugoniot多项式系数/GPa A2 29.11 损伤因子 D2 1.00 Hugoniot多项式系数/GPa A3 17.79 孔隙度指数 NP 3.0 孔隙坍塌压力/MPa pcrush 14.4 参考压缩应变率/s−1 ˙εc0 3.0×10−5 洛德角相关因子 Q0 0.68 参考拉伸应变率/s−1 ˙εt0 3.0×10−6 洛德角相关因子 B 0.05 破坏压缩应变率/s−1 ˙εc 3.0×1022 破坏面参数 A 1.99 破坏拉伸应变率/s−1 ˙εt 3.0×1022 破坏面参数 N 0.59 Grüneisen系数 γ 0.0 相对抗剪强度 F∗s 0.45 理论计算 侵蚀塑性应变 εfs 2.00 默认取值 相对抗拉强度 F∗t 0.10 剪切模量减小因子 ξr 0.50 状态方程参数 B0 1.68 状态方程参数/GPa T2 0.00 状态方程参数 B1 1.68 拉伸体积塑性应变分数 pft 0.001 弹性剪切模量/GPa G 5.10 2.2 炸药材料参数
LS-DYNA程序中采用08号高能炸药材料模型*MAT_HIGH_EXPLOSIVE_BURN模拟黑火药,并结合Jones-Wilkens-Lee (JWL)状态方程描述炸药爆轰体积、压力与内能的关系:
pe=Ae(1−ωeR1Ve)e−R1Ve+Be(1−ωeR2Ve)e−R2Ve+ωeEe0Ve (4) 式中:pe为爆轰压力,Ve为相对体积,
Ee0 为炸药体积内能,参数Ae、Be、R1、R2和ωe为材料常数。详细的参数如表3所示,其中vOD为爆轰速度,pCJ为C-J爆炸压力。表 3 炸药材料参数Table 3. Material parameters of the explosiveρe/(kg·m−3) vOD/(m·s−1) pCJ/GPa Ae/GPa Be/GPa R1 R2 ωe Ee0/GPa 790 1530 16.0 371 3.23 4.15 0.95 0.33 2.0 2.3 空气及细砂材料参数
LS-DYNA程序中模拟空气介质常使用09号材料模型*MAT_NULL,该材料可以选择定义气体或流体的黏度,且无需计算偏应力[27]。通过线性多项式状态方程*EOS_LINEAR_POLYNOMIAL定义了空气压力、密度和内能之间的关系:
pa=C0+C1μa+C2μ2a+C3μ3a+(C4+C5μa+C6μ2a)Ea0 (5) 式中:pa为空气压力,
Ea0 为空气的体积内能;μa为动态黏度系数,μa=(ρa/ρa0 )−1,其中ρa和ρa0 分别为空气材料的当前密度和初始密度;通常C0=C1=C2=C3=0,C6=0,C4=C5=γa−1,γa为绝热指数。详细的空气材料模型参数如表4[26]所示,其中Va0 为空气的初始相对体积。显式有限元程序中模拟细砂使用05号材料模型*MAT_SOIL_AND_FOAM,该模型可较好地模拟土壤、泡沫等多孔介质类材料的大变形行为,模型相对简单且适用性较好,其参数如表5[28]所示,其中
ρds 为干砂密度,Gds 和Kds 分别为干砂材料的剪切模量和卸载体积模量,pds 为干砂材料的截止压力;ads0 、ads1 和ads2 为干砂材料的剪切屈服面系数。该模型通过10组数据对材料的压缩状态方程进行多段线性逼近,进而描述材料体积应变随压力变化的关系,其中体积应变用相对体积的自然对数ln(Vds /Vds0 )表示,Vds 和Vds0 分别表示干砂变形后的体积和初始体积,10组ln(Vds /Vds0 )分别为0、0.11、0.17、0.22、0.26、0.29、0.36、0.41、0.46和0.51,相应的压力参数分别为0、26.7、46.8、66.9、93.6、120.2、233.3、368.5、669.2和1100.0 MPa,以上数据均来自文献[28]。2.4 模拟结果与实验结果的比较
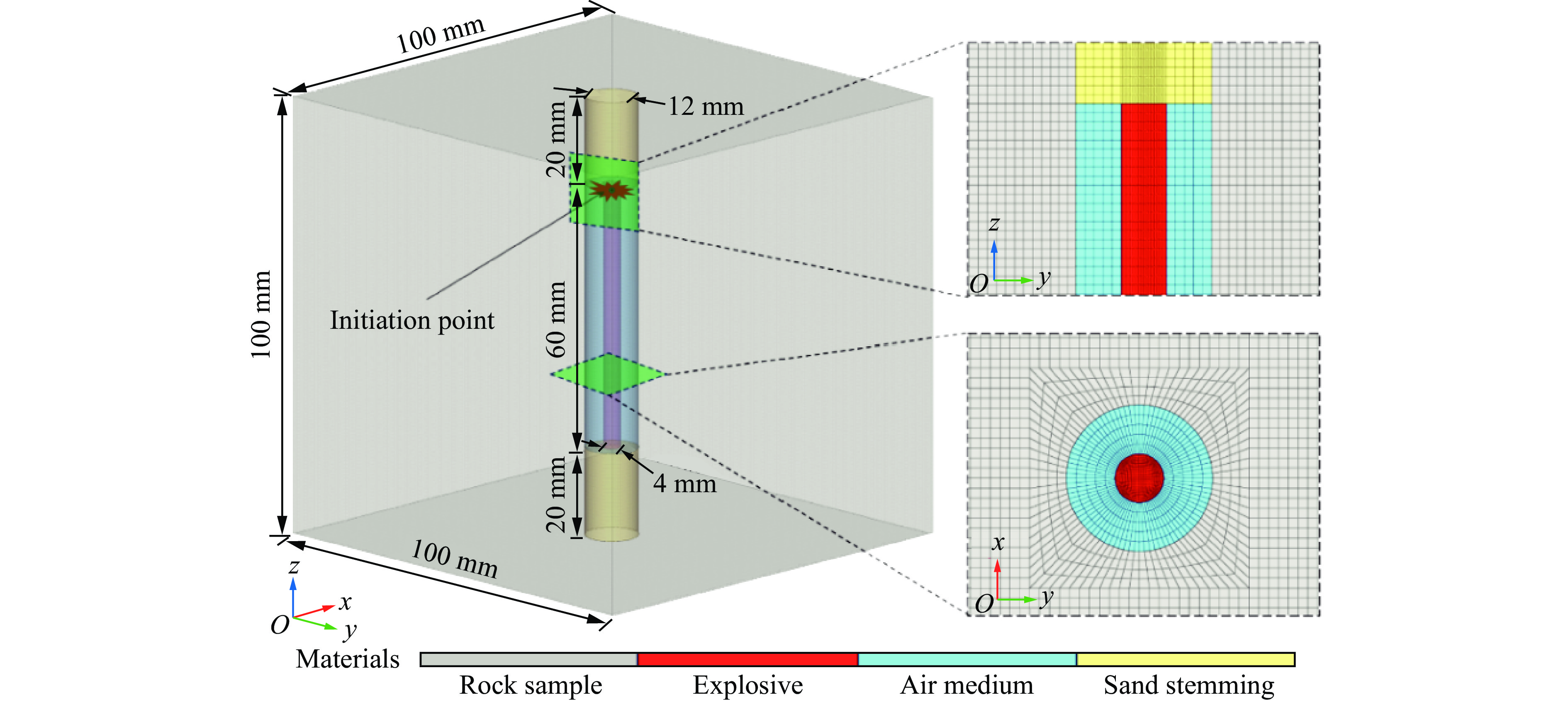
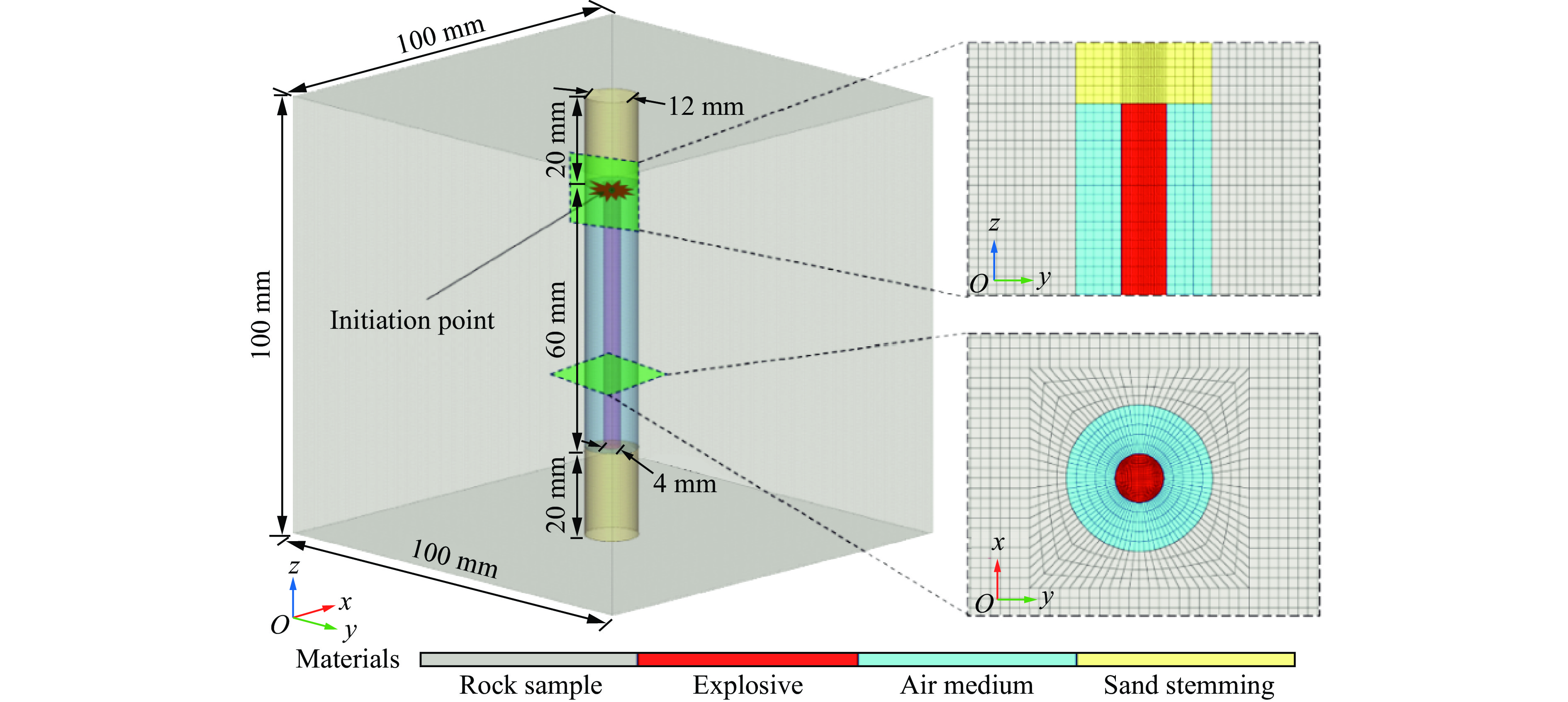
为了验证上述模型参数的合理性和可靠性,采用显式动力学有限元程序LS-DYNA模拟岩石在爆破荷载下的破裂模式和块体尺寸分布特征。在Ansys软件中建立了与试样R1爆破条件一致的数值模型,模型包括岩石、炸药、空气耦合介质和干砂堵塞4种材料,如图5所示。该模型共包含2 065 000个六面体单元和2 112 894个节点,其中炸药的网格尺寸最小,为0.2 mm×0.2 mm×0.8 mm,岩石的网格尺寸最大,为0.8 mm×0.8 mm×0.8 mm。需要说明的是,炸药、耦合介质、堵塞物和岩石组件之间通过共节点方式传递荷载。考虑到计算效率和模拟结果的合理性,炸药和空气介质采用任意拉格朗日欧拉(arbitrary Lagrangian-Eulerian, ALE)单元算法,而对于岩石和干砂材料则选择了拉格朗日单元算法。通过多物质组ALE-Multi-Materials-Group关键字,可以准确地捕捉岩石爆炸过程中不同材料之间复杂的相互作用过程,从而更加逼近实验中岩石的爆炸损伤破裂行为。
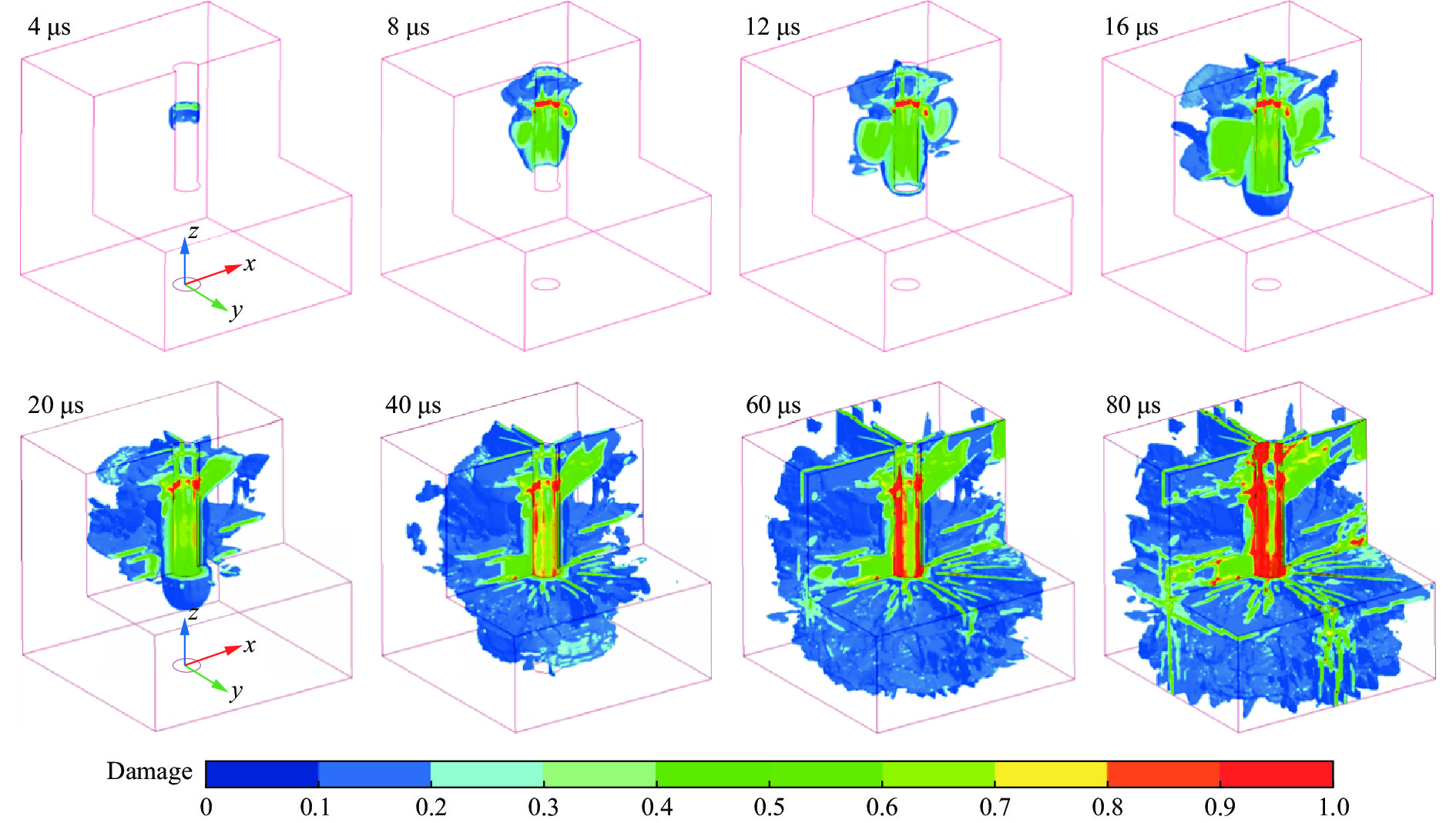
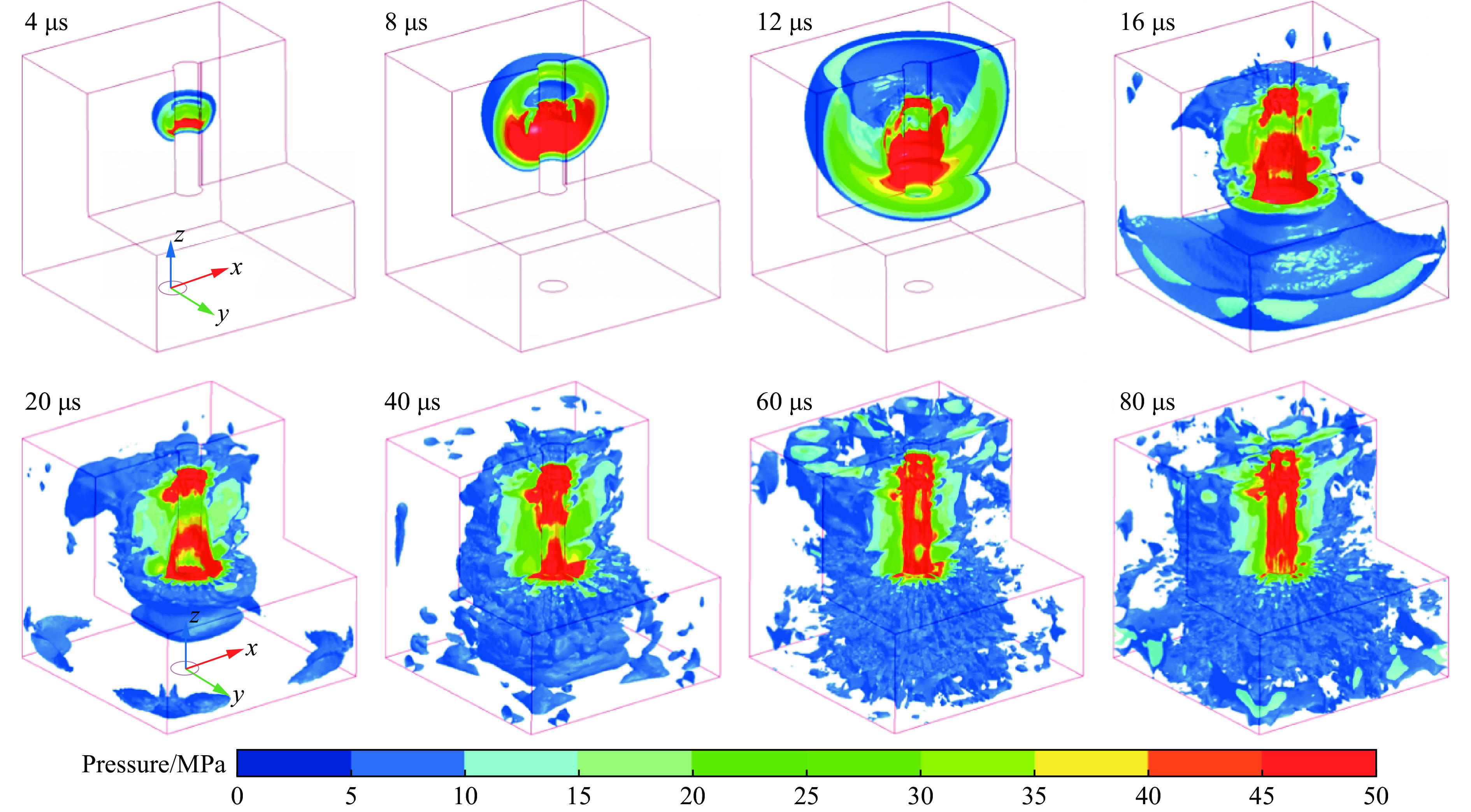
为直观地反映岩石在爆炸荷载下的变形损伤过程,分别讨论岩石内部的压力演化和爆生裂纹扩展行为。炸药起爆后,瞬间产生高压爆轰波,孔壁在高压作用下形成压剪粉碎区。爆轰波穿过空气介质后消耗了一部分能量,随着粉碎区范围的扩展,爆轰波能量急剧衰减,进而转变为应力波。由于应力波峰值强度较低,无法使岩石产生压缩破坏,此时粉碎区的范围几乎不再发生变化。但因岩石动态抗拉强度仍低于应力波峰值,在应力波拉伸作用下便形成了裂隙破碎区,如图6所示。应力波向自由面传播的同时,径向裂纹沿裂隙尖端持续扩展,到达自由面时发生反射,若反射后的拉伸应力波峰值高于岩石抗拉强度,可形成环状裂纹剥落区。冲击波以药卷为中心呈椭球状向外传播,如图7所示。炸药采用自上而下的起爆方式,爆炸能量很快在起爆点上方释放,向下的方向能量不断聚集,当冲击波传播至底部时发生发射,由于聚集的能量较高,在岩石底部便形成了相应的裂纹剥落区。
不耦合装药下岩石爆破数值模拟结果与实验结果的比较如图8所示。爆破裂纹在岩石侧面分布均匀,且呈直线状贯穿整个岩石表面,相较于岩石顶面,底面处的爆破裂纹更密集,且产生了损伤剥落区,如图8(a)所示。这是因为炸药自顶端起爆后,轴向上爆炸应力波不断叠加,致使爆炸应力场增强,进而导致岩石的损伤加剧。值得注意的是,实验结果中爆破裂纹分布更离散,这可能是由于实验中所使用的岩样存在天然裂隙,这些缺陷会影响爆破裂纹扩展的连续性。但从裂纹的区域分布及扩展行为来看,数值模拟结果可以很好地还原岩石的破裂现象。在定性比较的基础上,又定量对比了岩石的破碎块体尺寸分布曲线。其中,数值模型中的岩石爆破块体尺寸需要进行图像处理获取。首先对三维模型进行切片,然后将切片后的二维损伤云图导入到ImageJ软件中,该软件可以自动识别损伤边缘轮廓,将破碎区域分割后可以获得相应的块体尺寸分布数据[29-30]。由图8(b)可知,实验中测得的数据点基本分布在数值模拟计算结果的曲线上。综上所述,数值模拟结果与实验结果吻合较好,该模型参数适用于本文的研究工作。
3. 影响参数分析
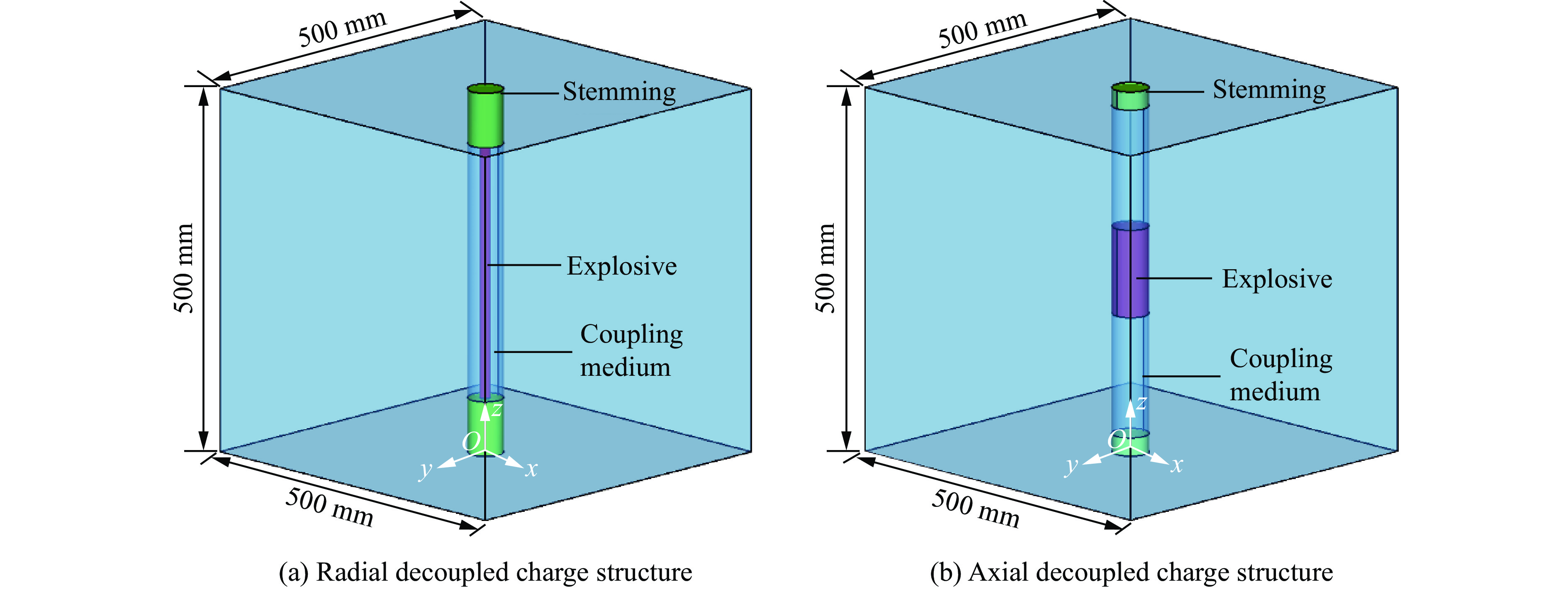
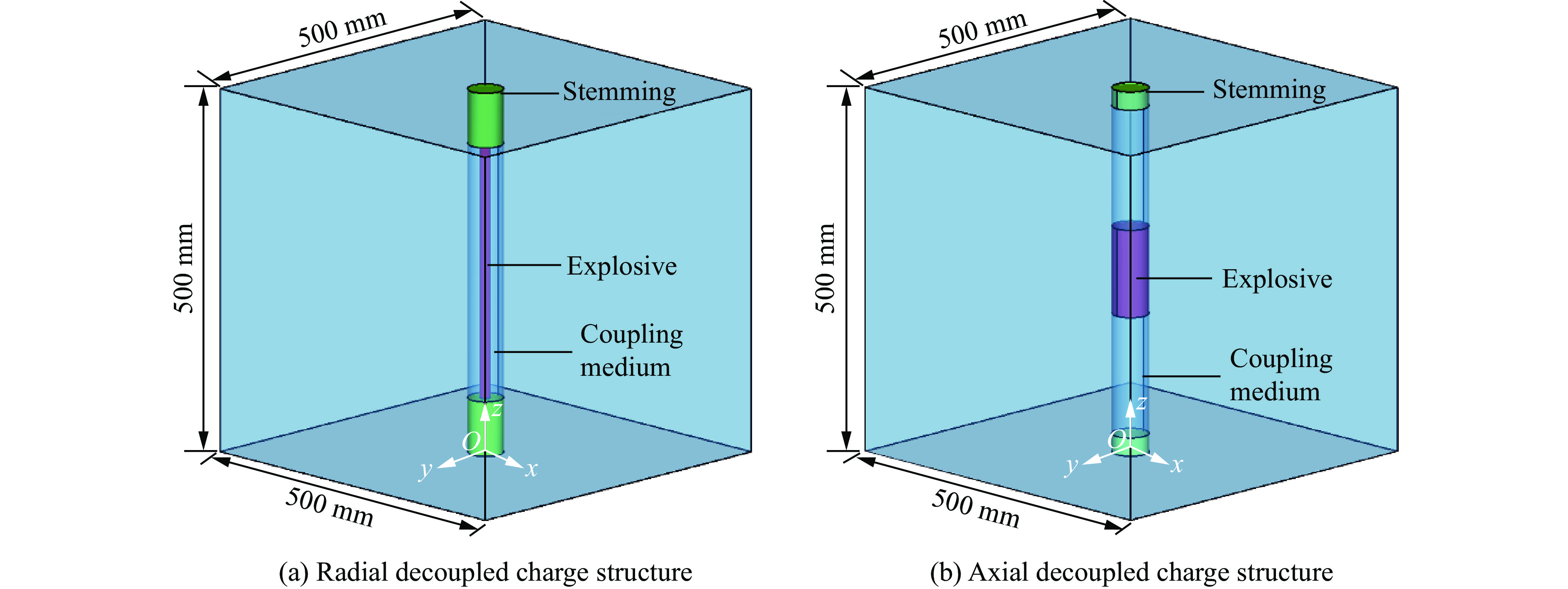
小型爆破实验中发现了装药系数和装药结构对岩石破碎效果的影响规律,并初步讨论了不耦合系数与岩石爆破块体尺寸之间的关系。但考虑到爆破实验中岩石的尺寸有限,与工程岩体爆破可能有所差别,为此,在实验的基础上进一步开展了大尺寸岩石单孔爆破数值计算。岩石单孔不耦合装药结构的几何模型如图9所示,模型主要包括岩石、炸药、耦合介质以及堵塞物,需要说明的是,不同装药条件下药量是保持不变的。
3.1 径向不耦合装药
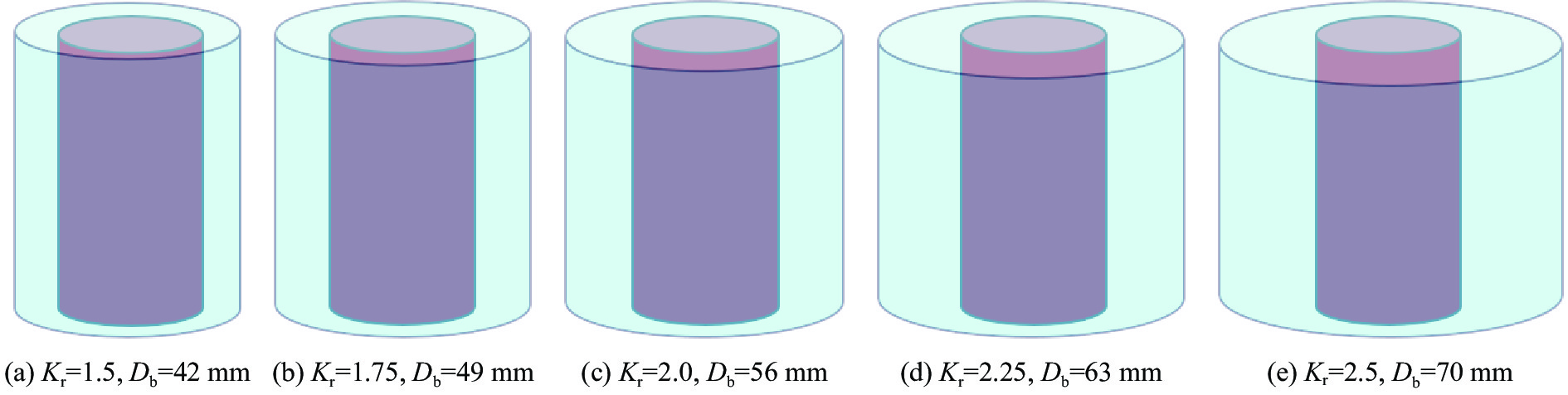
径向不耦合装药固定装药直径,通过改变炮孔直径,实现不同径向不耦合系数下的装药方式,炮孔直径Db分别设置为42、49、56、63和70 mm,如图10所示。
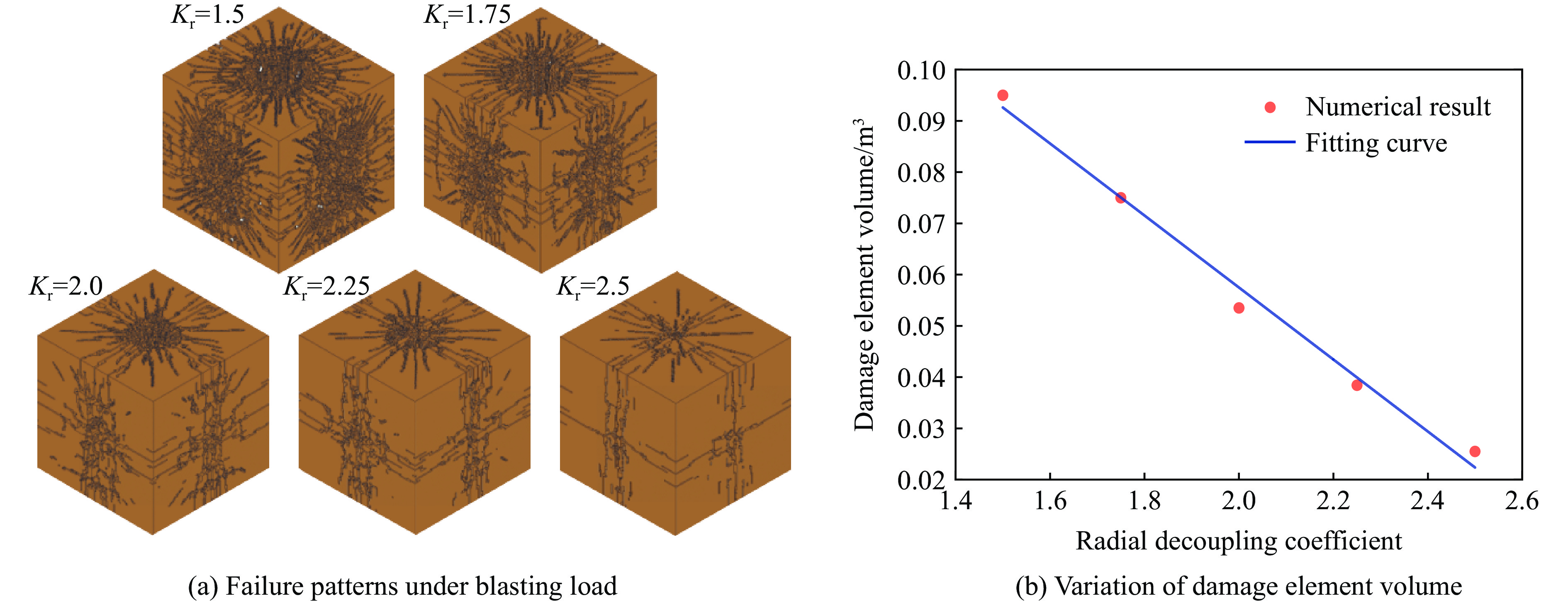
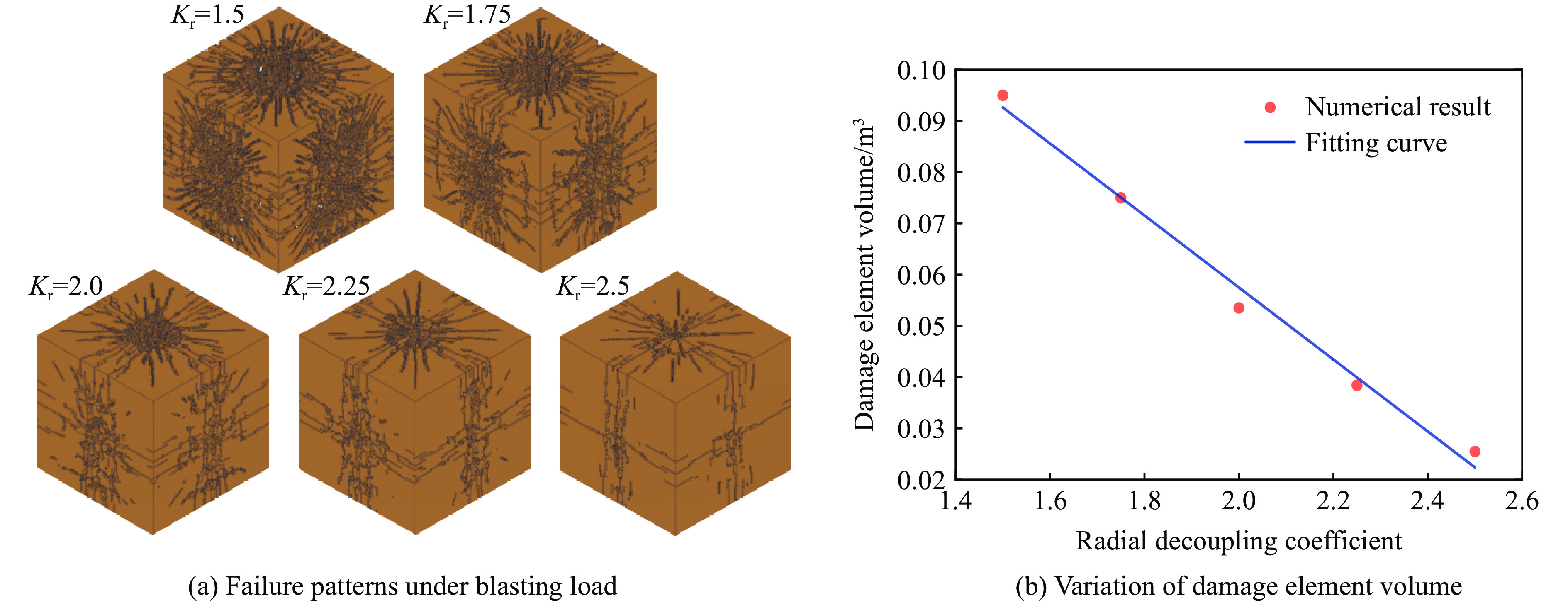
径向不耦合装药条件下岩石的破裂特征如图11所示。随着径向不耦合系数的增大,岩石的损伤程度呈减缓趋势,且其表面的爆破裂纹显著减少。此外,当径向不耦合系数较小时,如Kr = 1.5, 1.75时,岩石表面会出现环向裂隙区,如图11(a)所示。为定量分析岩石的破坏程度,分别统计各径向不耦合系数下岩石损伤单元的数量,进而求得损伤区域的体积,如图11(b)所示。由统计结果可知,岩石的损伤体积随径向不耦合系数的增大而减小,且近似呈线性变化关系:
Vrd=−0.07Kr+0.198R2=0.991 (6) 式中:
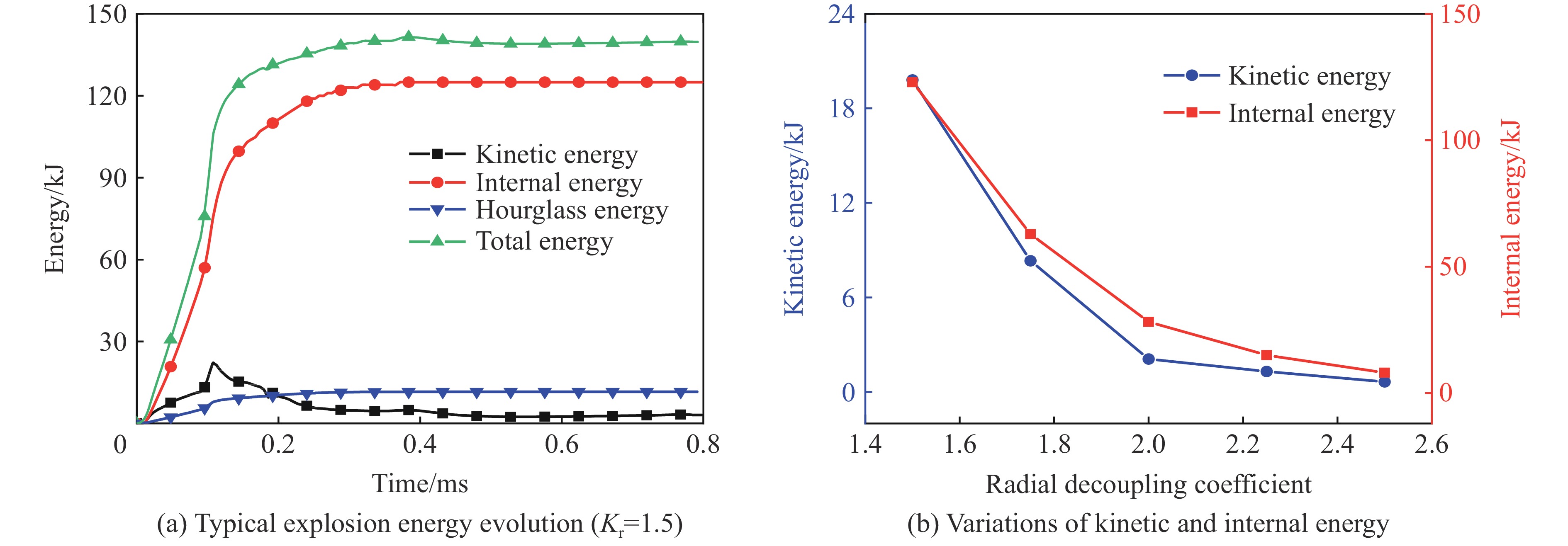
Vrd 为径向装药条件下岩石中损伤单元的总体积,单位为m3。爆炸荷载下岩石内部主要是动能(Ek)和内能(Ei)的变化过程,通过在计算程序中定义能量控制关键字,可输出各能量时程曲线。LS-DYNA程序采用单点积分可大幅度提升计算效率,但缩减积分会造成单元的零能模式,即沙漏问题。通常认为沙漏能控制在总能量的10%以下,数值计算结果才具有一定的可信度,为此,计算程序中还应定义沙漏控制关键字[31-32]。不耦合装药下岩石内部能量的变化过程如图12所示,通过计算可知,沙漏能约占总能量的8.3%,因此,该计算结果符合沙漏控制要求。爆炸荷载下岩石内部能量的变化过程如图12(a)所示,炸药起爆后孔壁受到冲击波高温高压作用,岩石的动能快速增长,增长至峰值点处又急剧衰减然后趋于稳定。此外,内能在爆炸初期也是快速聚集达到峰值,随后逐渐平稳发展。岩石爆炸能量随径向不耦合系数的变化规律如图12(b)所示,随着径向不耦合系数的增大,爆炸动能和内能逐渐减小,且Kr<2.0时,不耦合系数对爆炸能量的影响较显著。
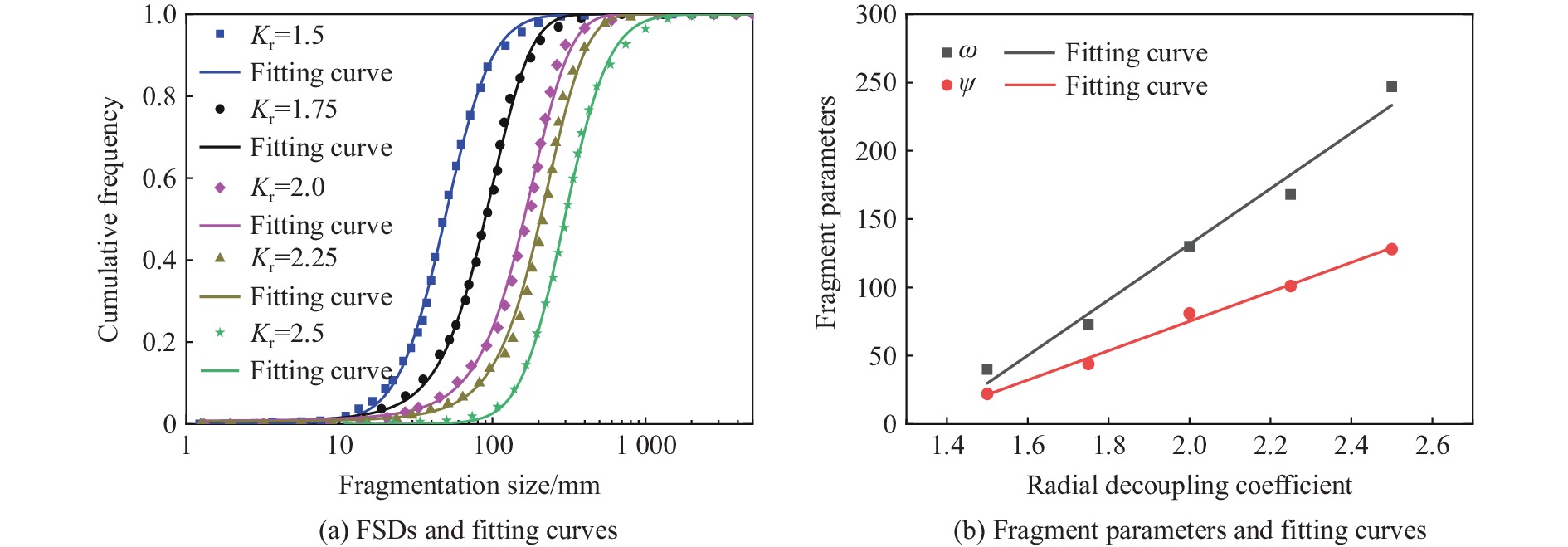
径向不耦合装药下岩石的爆破块体尺寸分布如图13所示。首先导出有限元模型的计算结果,然后结合图像处理技术获得岩石块体尺寸分布的数据,最后通过三参数极值分布函数拟合数据,如图13(a)所示。与实验结果类似,极值分布函数与数值模拟的计算数据匹配性较好。随着径向不耦合系数的减小,拟合曲线“抬头”越早,这表明岩石碎块尺寸越小且更均匀,如图13(b)所示。
3.2 轴向不耦合装药
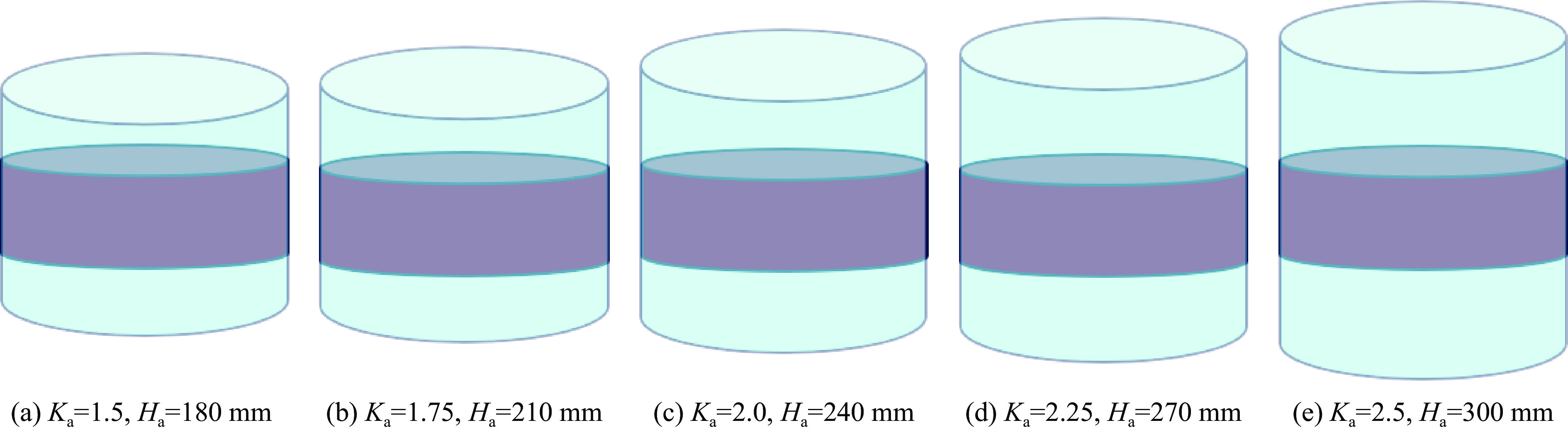
轴向不耦合装药固定装药高度,通过改变空气层的高度,实现不同轴向不耦合系数Ka下的装药方式,空气层的高度Ha分别设置为180、210、240、270和300 mm,如图14所示。
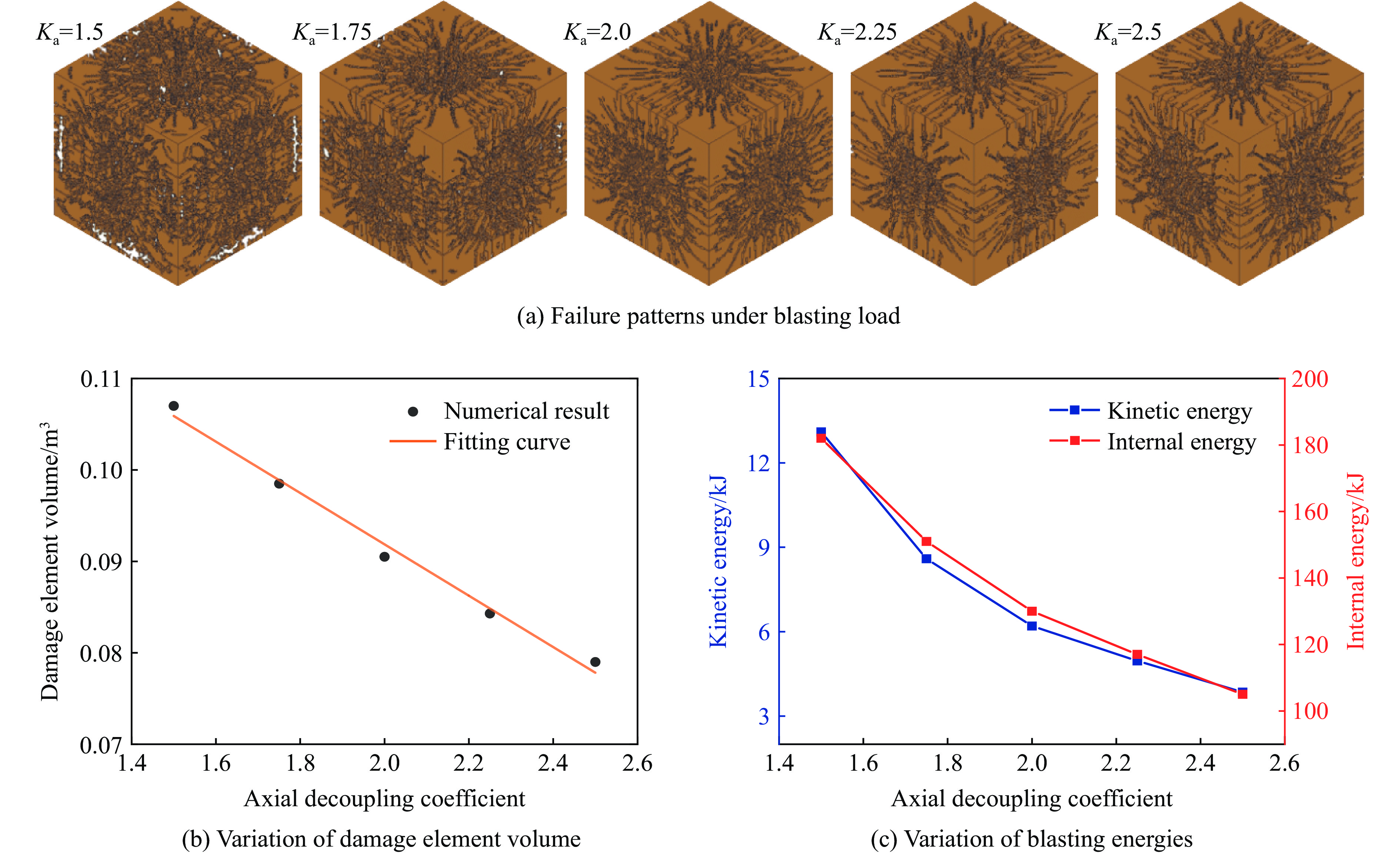
轴向不耦合装药条件下岩石破裂特征如图15所示。随着轴向不耦合系数的增大,岩石的损伤程度逐渐降低,表面的爆破裂纹逐渐减少。且相较于径向不耦合装药而言,轴向不耦合装药条件下,随轴向不耦合系数Ka的改变,岩石损坏程度的变化范围较小,如图15(a)所示,这与1.2节中观察到的实验现象是一致的。岩石损伤体积的统计结果如图15(b)所示,由统计结果可知,岩石的损伤体积随轴向不耦合系数的增大而减小,且近似呈线性的变化关系:
Vad=−0.028Ka+0.148 R2=0.987 (7) 式中:
Vad 为轴向装药条件下岩石中损伤单元的体积,单位为m3。通过对比不同装药结构下岩石损伤体积拟合曲线的斜率,也印证了岩石在轴向装药下破坏程度较高,且破坏变化范围较小的规律。轴向不耦合装药条件下,岩石内部的动能和内能随不耦合系数的变化规律如图15(c)所示。与径向不耦合装药类似,随着轴向不耦合系数的增加,动能和内能会不断减小,且减小的速率逐渐降低,动能减小影响岩石破碎效果,进而导致破岩效率降低。
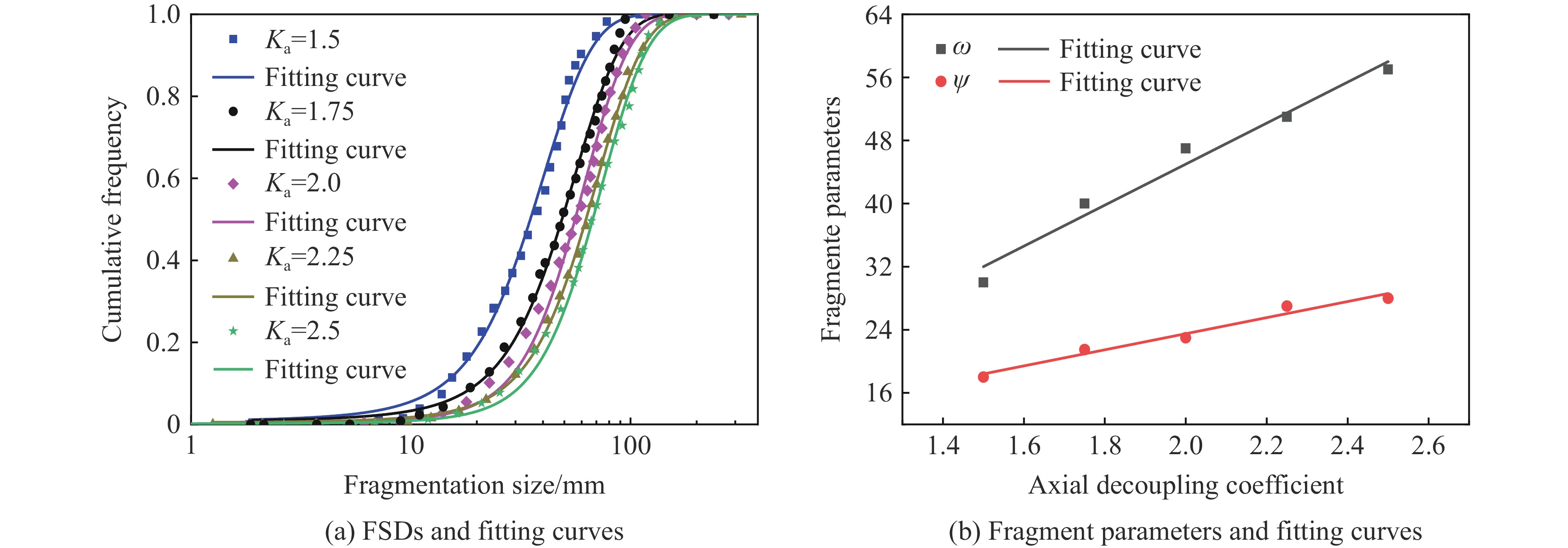
轴向不耦合装药下岩石的爆破块体尺寸分布如图16所示。与径向不耦合装药类似,轴向不耦合装药条件下,岩石爆破块体尺寸分布的数据也可以用三参数极值分布函数很好地进行拟合,当轴向不耦合系数越小时,拟合曲线“抬头”越早,如图16(a)所示。与径向不耦合装药不同的是,随着轴向不耦合系数的增大,岩石块体尺寸的分布范围变化较小,且其爆破块体的平均尺寸整体偏小,如图16(b)所示。通过数值模拟并结合实验结果分析发现,同等装药量的情况下,轴向空气不耦合装药结构可以更好的传递爆炸能量,进而使得岩石的爆破破碎块体尺寸更小、更均匀。
3.3 不同耦合介质的影响
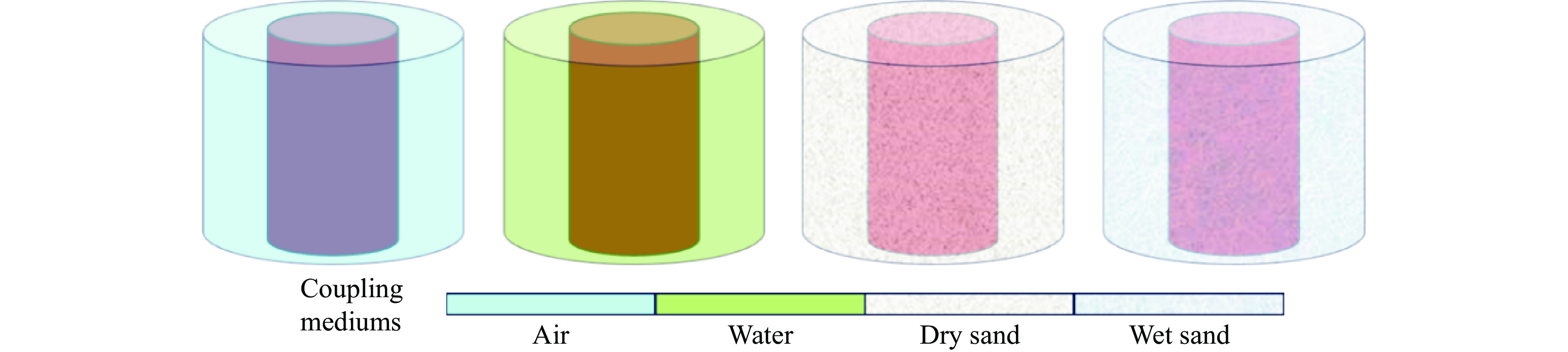
除装药结构外,耦合介质对岩石爆破破碎效果的影响也很大,常用的耦合介质主要包括固、液、气3种形态。本文中考虑了空气、水、干砂和湿砂4种耦合介质材料,如图17所示。通过固定径向不耦合系数Kr = 2.5,改变耦合介质,分析耦合介质对岩石破碎块体尺寸分布的影响。
数值模型验证时分别给出了空气与干砂的材料参数,与空气介质类似,LS-DYNA程序使用09号材料模拟水介质,通过Grüneisen状态方程可以定义水的压力、密度与初始内能之间的关系:
pw=ρwc2wμw[1+(1−γw/γw22)μw−αwμ2w/αwμ2w22][1−(S1−1)μw−S2μ2w/S2μ2w(μw+1)(μw+1)−S3μ3w/S3μ3w(μw+1)2(μw+1)2]2+(γw+αwμw)Ew (8) 式中:pw为水压,cw和Ew分别为水中的声速和水的体积内能,μw为水的动态黏度系数,与空气的求解方法类似。S1、S2、S3、γw和αw为材料常数,水介质的模型参数如表6所示,其中
Vw0 为水的初始相对体积。表 6 水介质模型参数Table 6. Material parameters for waterρw/(kg·m−3) cw/(m·s−1) Ew/(kJ·m−3) S1 S2 S3 γw αw Vw0 1000 1480 1890 2.56 −1.98 1.23 0.35 0.0 1.0 实际工程中含水炮孔十分常见,干砂填塞时的物理力学性质会发生改变,湿砂也选用05号材料模型*MAT_SOIL_AND_FOAM,具体参数参如表7[28]所示,其中
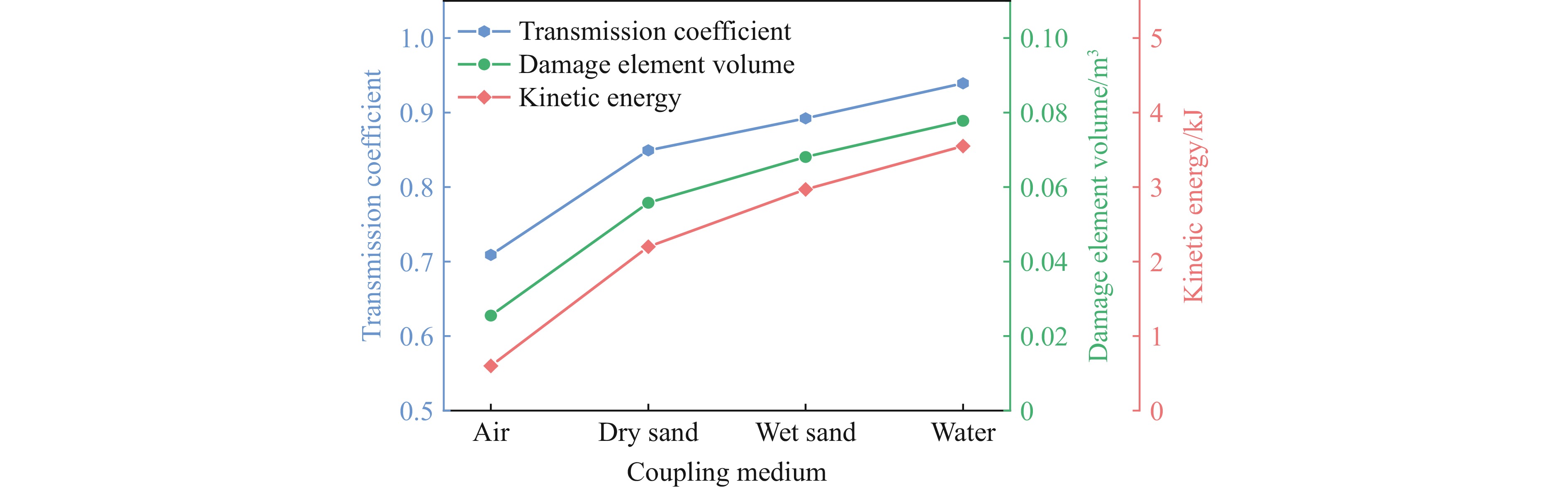
ρws 为湿砂的密度,Gws 和Kws 分别为湿砂材料的剪切模量和卸载体积模量,pws 为湿砂材料的截止压力;aws0 、aws1 和aws2 为湿砂的剪切屈服面系数。同样地,通过10组数据描述湿砂材料体积应变随压力变化的关系,其中体积应变用相对体积的自然对数ln(Vws /Vws0 )表示,Vws 和Vws0 分别为湿砂变形后的体积和初始体积,10组ln(Vws /Vws0 )分别为0.0、0.10、0.16、0.19、0.22、0.25、0.27、0.28、0.29和0.40,相应的压力参数分别为0.0、20.0、40.0、60.0、120.0、200.0、400.0、600.0、800.0和4100.0 MPa,以上数据均来自文献[28]。不同耦合介质装药条件下岩石的破裂特征如图18所示。与气体介质相比,固体与液体作为耦合介质时爆破效果更好,值得注意的是,湿砂相较于干砂而言,湿砂作为耦合介质时岩石的爆破损伤程度更高,如图18(a)所示。这可能是因为干砂相对松散,孔隙度较高,而湿砂密实性较好,爆炸能量传递效率更高。与空气不耦合装药结构类似,其他耦合介质装药时,岩石的破碎块体尺寸分布也可用极值分布函数拟合,如图18(b)所示。通过拟合出的块体尺寸参数也可以看出,水作为耦合介质时,岩石的破碎块体平均尺寸和分布范围最小,湿砂、干砂和空气作为耦合介质时,岩石的破碎块体平均尺寸和分布范围逐渐增大,如图18(c)所示。
岩体爆破采用径向不耦合装药结构时,爆炸应力波通过耦合介质传播至岩体中的过程可简化为线弹性平面应变问题。其中,炮孔和药卷半径分别为rb和re,炸药与耦合介质的交界面定义为界面1,耦合介质与岩石的交界面处定义为界面2,如图19所示。
假设爆轰波穿过耦合介质时,界面1与界面2处的速率和应力保持连续,那么炸药能量传递到岩石中的效率则可以通过等效波阻法求得[33],界面1中的速率ve和应力σe可由下式求得:
ve=v+e+v−e=v+ce+v−ce, σe=σ+e+σ−e=zcv+ce−zcv−ce (9) 式中:zc为应力波在不同耦合介质中传播时的波阻抗,上标“+”和“−”分别表示爆炸应力波的透射和反射,下标“e”和“ce”分别表示炸药区域和耦合介质靠近炸药一侧的区域。
界面2中的速率vcr和应力σcr,通过改变应力波位相因子(正向行进应力波的相位因子乘以e−iζ,负向行进应力波的相位因子乘以eiζ),就可以确定同一时刻该处的速率与应力状态:
v+cr=v+cee−iς,v−cr=v−ceeiς (10) 式中:ζ为位相厚度,
ζ=2π(rb−re)/λc ,其中λc为应力波在不同耦合介质中传播时的波长。λc的表达式为:λc=0.0007ccoupw0.5eK−0.45r (11) 式中:ccoup为耦合介质的声速,we为炸药当量。
根据求解界面1中的速率与位移方法,同理可以求得界面2中的速率vcr和应力σcr。将界面1中与界面2中的速率和应力写成矩阵的表达形式,然后联立2个矩阵进行化简,得到:
[veσe]=[cosςisinς/zcizcsinςcosς][vcrσcr] (12) 根据式(12)便可求得爆炸应力波穿过耦合介质与岩体界面的等效波阻抗zc+r:
zc+r=zcisinς+zrcosςcosς+zr/zrzcisinςzcisinς (13) 不耦合装药下爆炸应力波从炸药传播到岩石中的应力透射系数βT和反射系数βR可表示为:
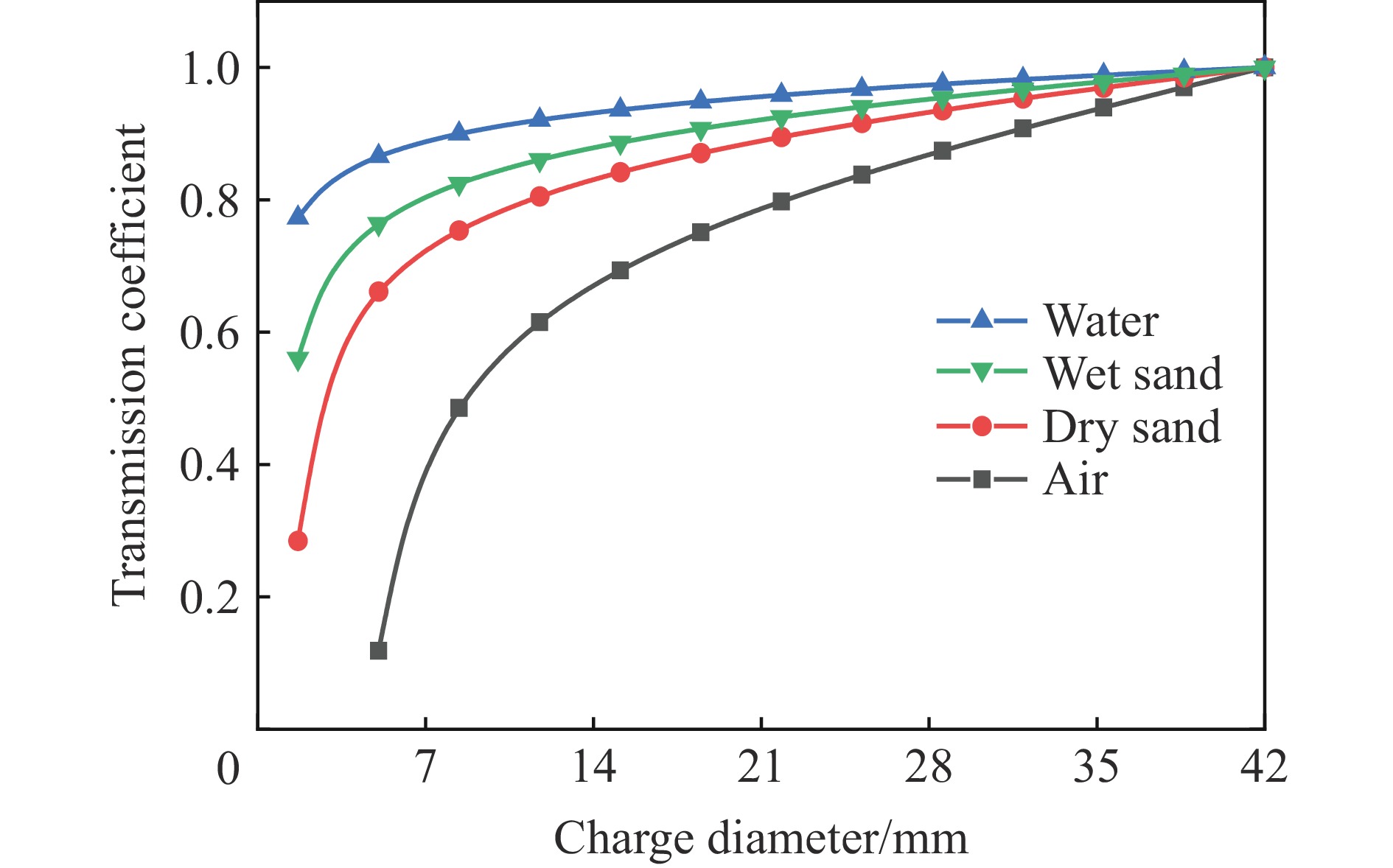
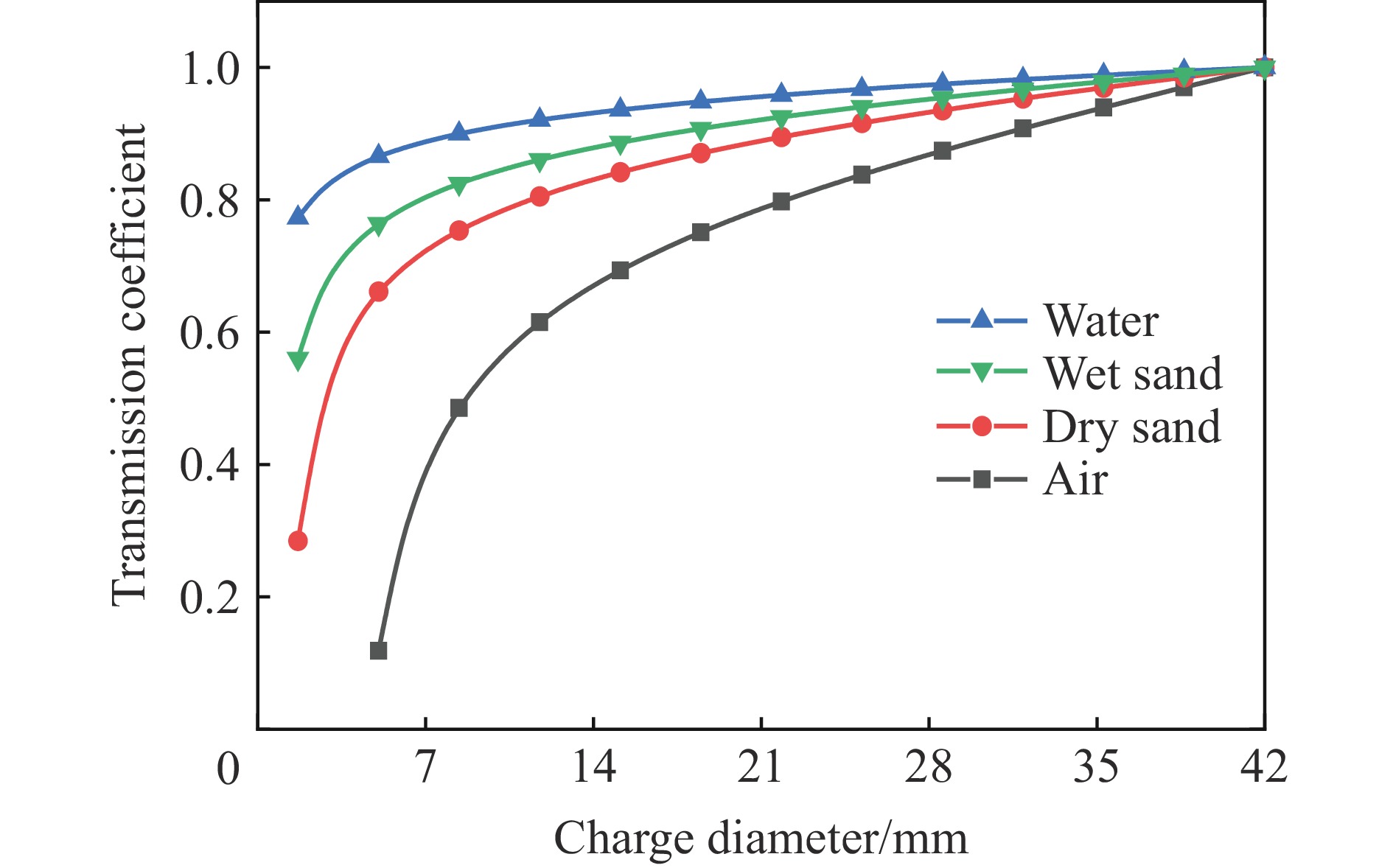
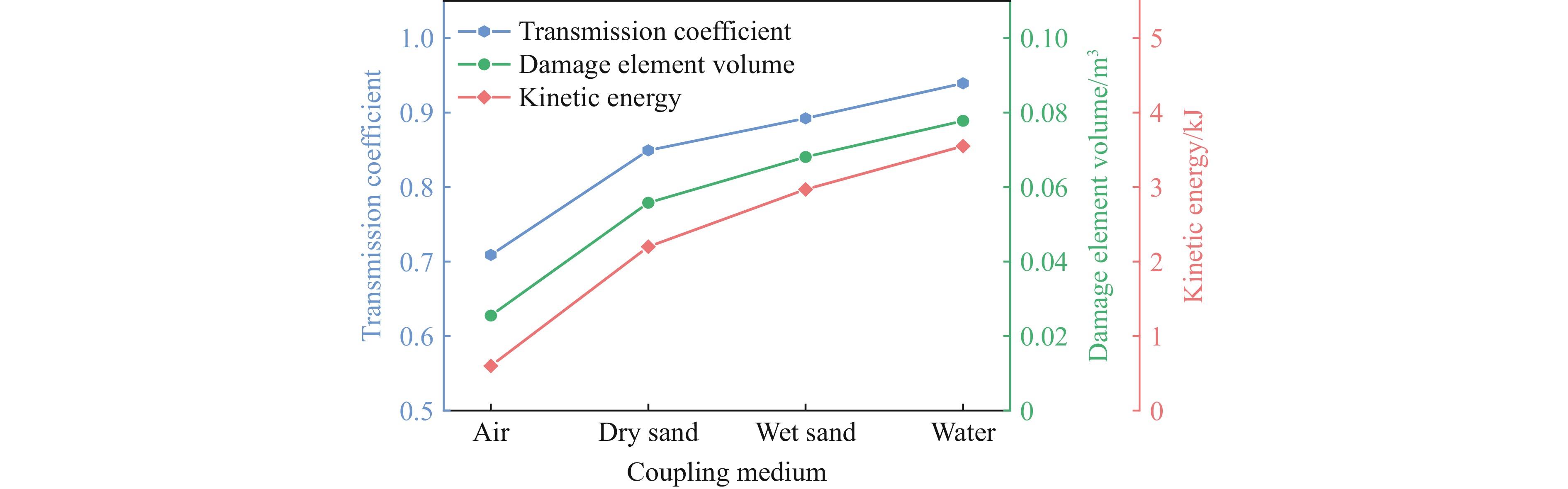
βT=2zc+rze+zc+r,βR=ze−zc+rze+zc+r (14) 根据式(14)并结合文中所给的炸药、岩石和耦合介质的物理力学参数,可分别求出不同耦合介质装药时,爆炸应力波在耦合介质与岩石交界面处的传递效率,如图20所示。应力波透射系数随着装药直径的增大而不断增大,且其增大速度逐渐放缓,对比不同耦合介质时的爆炸应力透射系数,可以发现分别采用水、湿砂、干砂和空气耦合装药时,相应的爆炸应力透射系数以此减小。同一条件下,应力透射系数、损伤体积和动能结果如图21所示,从曲线走向来看,3组数据具有相同的变化趋势,也就是说理论计算的应力透射系数可以较好地预测岩石的损伤和破碎状态。
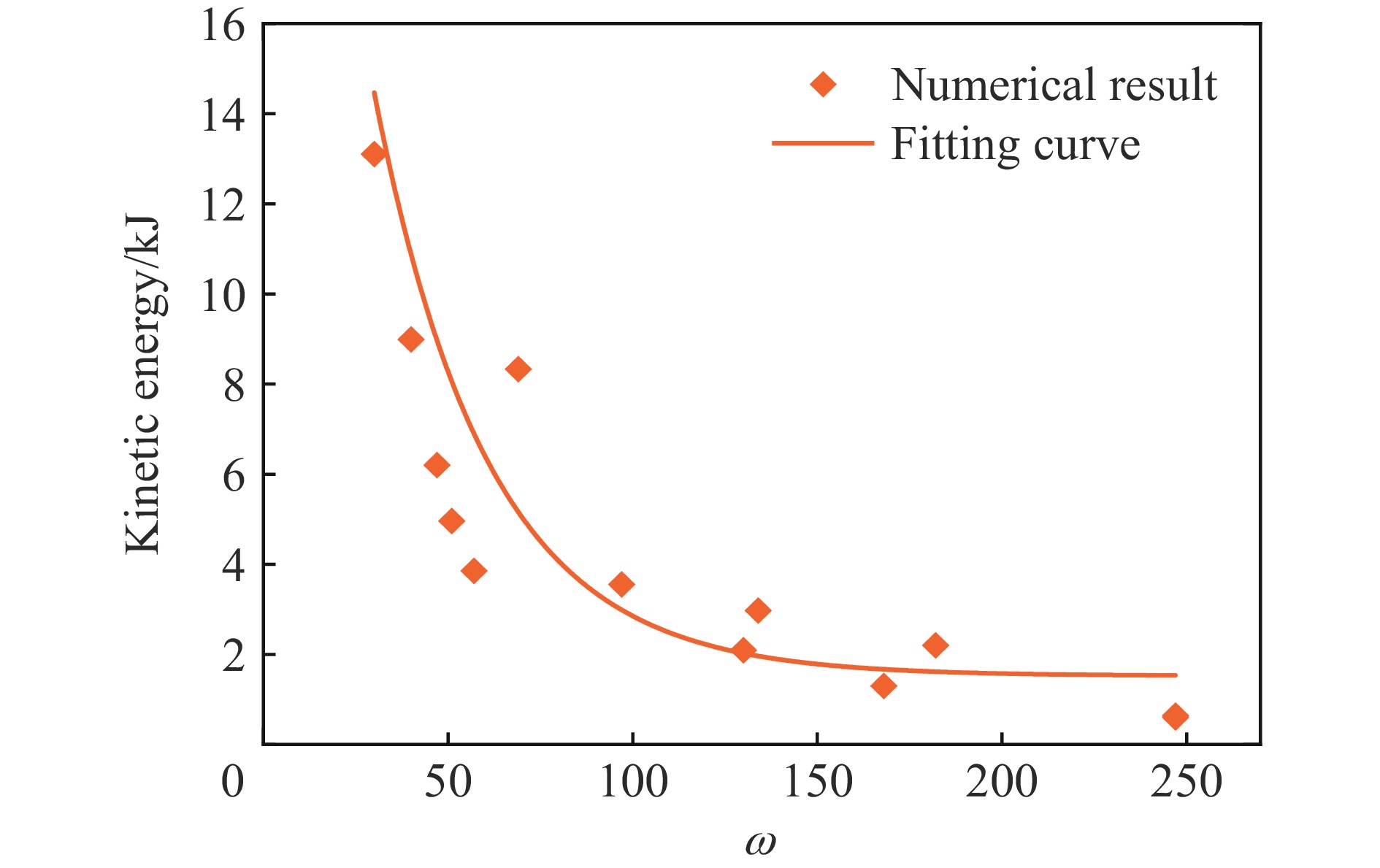
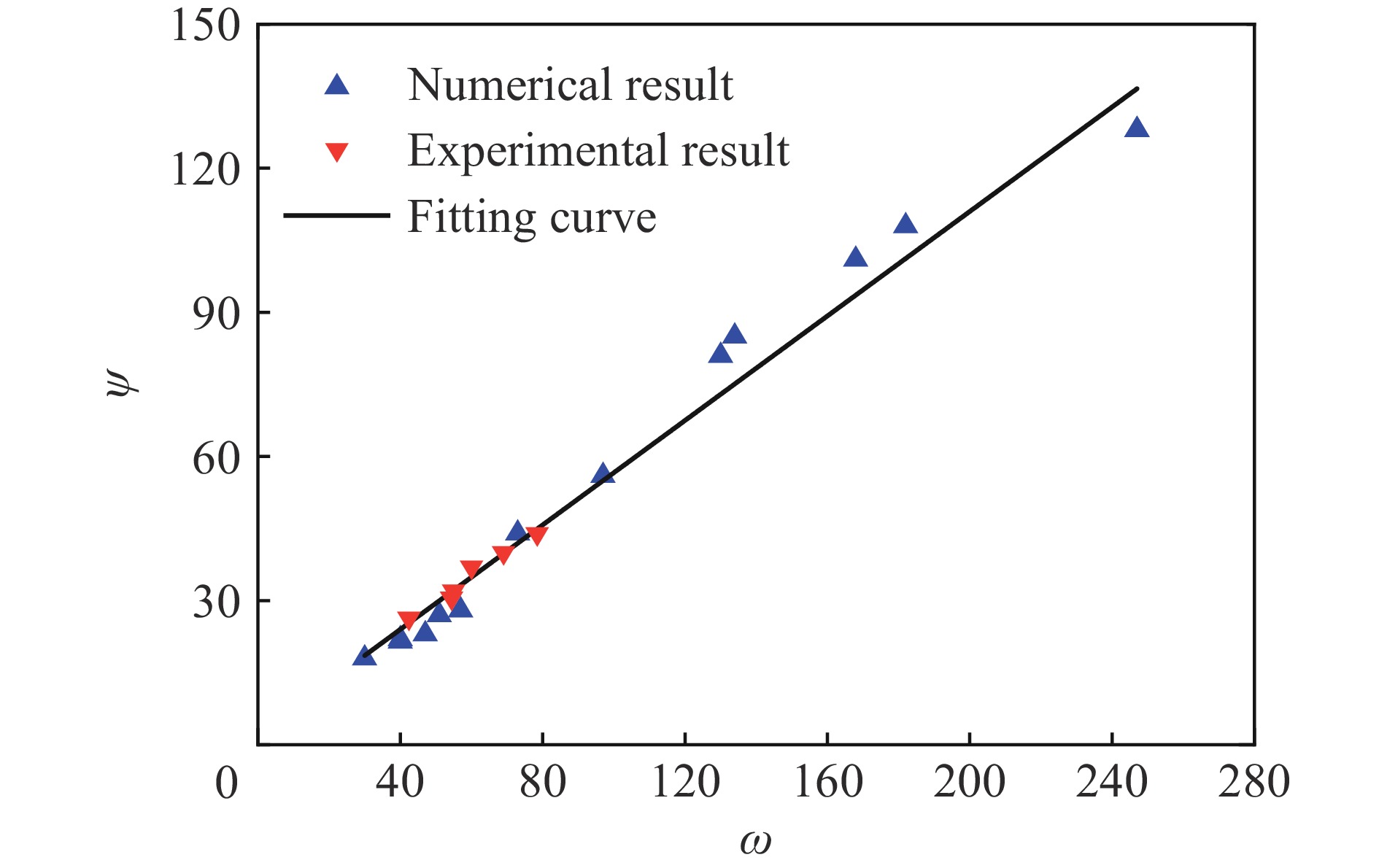
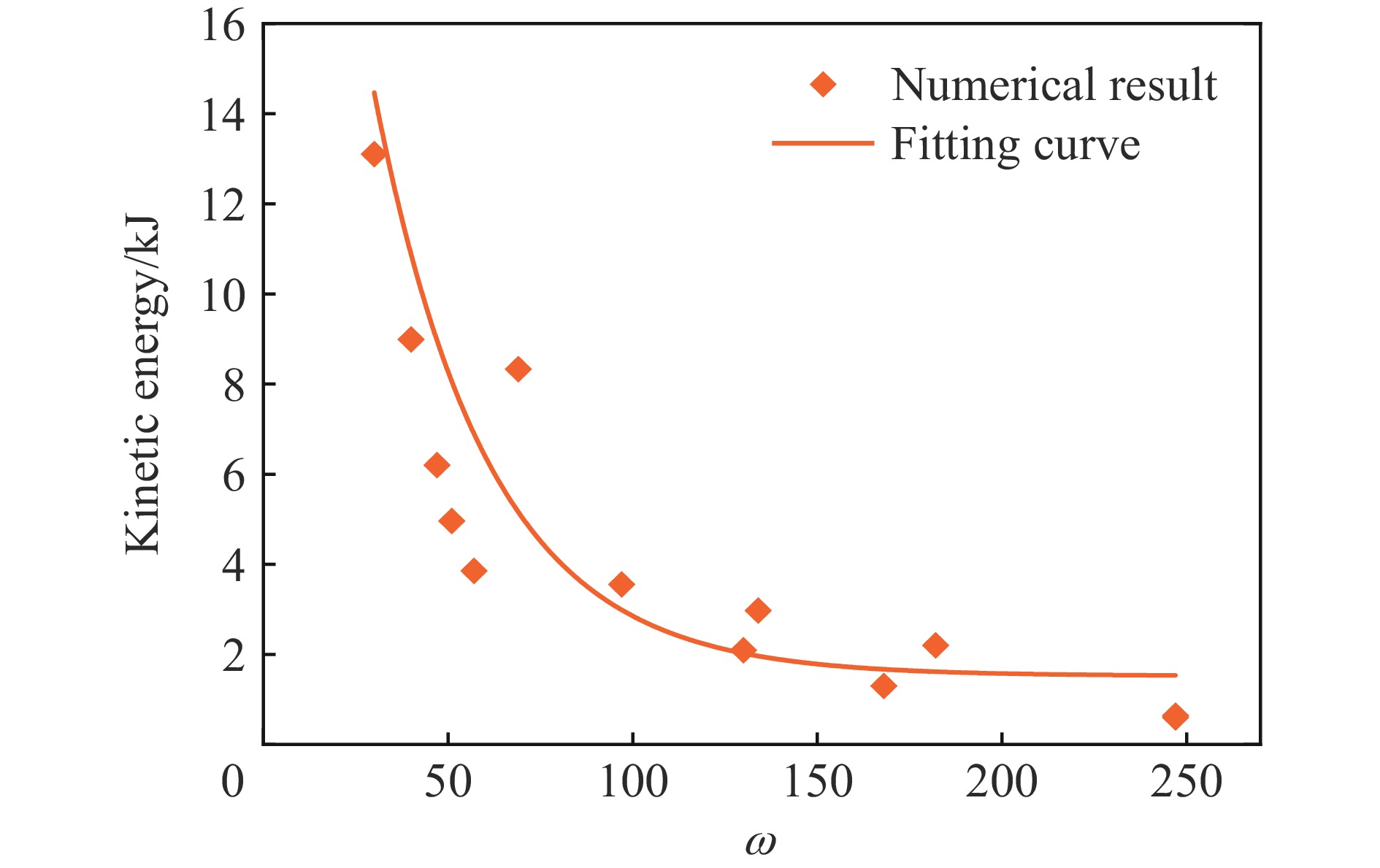
对极值分布函数拟合出的参数ω和ψ进行线性回归,如图22所示。其中蓝色数据点表示模拟结果,红色数据点表示实验结果,其拟合方程为ψ=0.543ω+2.363(R2=0.981),由线性曲线的决定系数可知,岩石的爆破块体平均尺寸与尺寸分布范围有较强的相关性。这是因为爆炸能量传递效率较低时,会产生数量更多的大块岩体,随着块体总体尺寸的增大,大块岩体与小块岩体之间差异性也愈加显著,会导致块体尺寸分布范围扩大。对爆破块体平均尺寸和岩石内的动能进行拟合,可以发现随着岩石动能的减小,块体平均尺寸逐渐增大,且增大的速率逐渐提高。当动能低于3 kJ时,岩石的破碎效果相对较差,如图23所示。
4. 结 论
通过室内小型爆破实验研究了不同装药结构下岩石的破坏模式,引入极值分布函数描述岩石爆破后破碎块体尺寸分布特征,基于验证的数值模型,模拟了不同装药结构下岩石的损伤破裂过程,讨论了不同耦合介质对岩体爆破破碎效果的影响,得到的主要结论如下。
(1)径向和轴向不耦合装药条件下,岩石的破碎程度均会随着不耦合系数的减小而提高。同等药量下,轴向不耦合装药结构比径向不耦合装药结构的破岩效果更好。
(2)三参数极值分布函数可以较好地表征岩石爆破后块体的尺寸分布特征,破碎块体的平均尺寸随不耦合系数的减小而不断减小,且块体总体尺寸趋于均匀化发展,实验结果与模拟结果均证实块体平均尺寸与块体尺寸分布范围近似呈线性正相关。
(3)水、湿砂、干砂和空气作为耦合介质时,爆炸应力波的透射系数依次减小,应力透射系数可以很好地反映不耦合装药下岩石内部能量传递及损伤程度的变化规律。
-
表 1 试样尺寸及装药结构
Table 1. Samples dimensions and charge structures
试样 试样长度/mm 试样宽度/mm 试样高度/mm 炮孔直径/mm 不耦合系数 装药结构 R1 100.29 100.34 100.37 12.00 3.0 径向不耦合 R2 100.38 99.81 99.73 10.00 2.5 R3 100.02 100.53 100.52 8.00 2.0 A1 99.81 100.14 100.12 10.00 3.0 轴向不耦合 A2 100.31 100.27 99.77 10.00 2.5 A3 99.63 99.86 100.11 10.00 2.0 表 2 岩石RHT模型的材料参数
Table 2. Material parameters of the rock RHT model
参数名称 符号 值 来源 参数名称 符号 值 来源 密度/(kg·m−3) ρr 2360 实验测定 残余面参数 Af 1.62
试错法初始孔隙度 αr0 1.12 残余面参数 Nf 0.61 抗压强度/MPa fc 21.6 压缩屈服面参数 G∗c 0.53 压缩应变率指数 βc 0.047 理论计算 拉伸屈服面参数 G∗t 0.70 拉伸应变率指数 βt 0.048 最小损伤残余应变 εmp 0.01 状态方程参数/GPa T1 17.33 孔隙压实压力/GPa pcomp 6.00 Hugoniot多项式系数/GPa A1 17.33 损伤因子 D1 0.04 默认取值 Hugoniot多项式系数/GPa A2 29.11 损伤因子 D2 1.00 Hugoniot多项式系数/GPa A3 17.79 孔隙度指数 NP 3.0 孔隙坍塌压力/MPa pcrush 14.4 参考压缩应变率/s−1 ˙εc0 3.0×10−5 洛德角相关因子 Q0 0.68 参考拉伸应变率/s−1 ˙εt0 3.0×10−6 洛德角相关因子 B 0.05 破坏压缩应变率/s−1 ˙εc 3.0×1022 破坏面参数 A 1.99 破坏拉伸应变率/s−1 ˙εt 3.0×1022 破坏面参数 N 0.59 Grüneisen系数 γ 0.0 相对抗剪强度 F∗s 0.45 理论计算 侵蚀塑性应变 εfs 2.00 默认取值 相对抗拉强度 F∗t 0.10 剪切模量减小因子 ξr 0.50 状态方程参数 B0 1.68 状态方程参数/GPa T2 0.00 状态方程参数 B1 1.68 拉伸体积塑性应变分数 pft 0.001 弹性剪切模量/GPa G 5.10 表 3 炸药材料参数
Table 3. Material parameters of the explosive
ρe/(kg·m−3) vOD/(m·s−1) pCJ/GPa Ae/GPa Be/GPa R1 R2 ωe Ee0/GPa 790 1530 16.0 371 3.23 4.15 0.95 0.33 2.0 ρa/(kg·m−3) C0 C1 C2 C3 C4 C5 C6 Ea0/(kJ·m−3) Va0 1.29 0.0 0.0 0.0 0.0 0.4 0.4 0.0 250 1.0 ρds/(kg·m−3) Gds/MPa Kds/MPa pds/kPa ads0 ads1 ads2 1600 34.8 134 -3.4 0.0 0.0 0.3 表 6 水介质模型参数
Table 6. Material parameters for water
ρw/(kg·m−3) cw/(m·s−1) Ew/(kJ·m−3) S1 S2 S3 γw αw Vw0 1000 1480 1890 2.56 −1.98 1.23 0.35 0.0 1.0 -
[1] LI H B, XIA X, LI J C, et al. Rock damage control in bedrock blasting excavation for a nuclear power plant [J]. International Journal of Rock Mechanics and Mining Sciences, 2011, 48(2): 210–218. DOI: 10.1016/j.ijrmms.2010.11.016. [2] 付玉华, 李夕兵, 董陇军. 损伤条件下深部岩体巷道光面爆破参数研究 [J]. 岩土力学, 2010, 31(5): 1420–1426. DOI: 10.16285/j.rsm.2010.05.005.FU Y H, LI X B, DONG L J. Analysis of smooth blasting parameters for tunnels in deep damaged rock mass [J]. Rock and Soil Mechanics, 2010, 31(5): 1420–1426. DOI: 10.16285/j.rsm.2010.05.005. [3] LU W B, CHEN M, GENG X, et al. A study of excavation sequence and contour blasting method for underground powerhouses of hydropower stations [J]. Tunnelling and Underground Space Technology, 2012, 29: 31–39. DOI: 10.1016/j.tust.2011.12.008. [4] 叶志伟, 陈明, 魏东, 等. 不耦合装药爆破孔壁压力峰值的实验研究 [J]. 爆炸与冲击, 2021, 41(5): 055201. DOI: 10.11883/bzycj-2020-0004.YE Z W, CHEN M, WEI D, et al. Experimental study on the peak pressure of borehole wall in decoupling charge blasting [J]. Explosion and Shock Waves, 2021, 41(5): 055201. DOI: 10.11883/bzycj-2020-0004. [5] HUO X F, QIU X Y, SHI X Z, et al. Experimental and numerical investigation on the peak value and loading rate of borehole wall pressure in decoupled charge blasting [J]. International Journal of Rock Mechanics and Mining Sciences, 2023, 170: 105535. DOI: 10.1016/j.ijrmms.2023.105535. [6] LANGEFORS U, KIHLSTRÖ M B. The modern technique of rock blasting [M]. 3rd ed. New York: John Wiley and Sons Incorporation, 1963. [7] PAINE R S, HOLMES D K, CLARK H E. Controlling overbreak by presplitting [M]//CLARK G B. Mining Research. Amsterdam: Elsevier, 1962. [8] 张正宇, 卢文波, 刘美山, 等. 水利水电工程精细爆破概论 [M]. 北京: 中国水利水电出版社, 2009. [9] CHEN M, LU W B, YI C P. Blasting vibration criterion for a rock-anchored beam in an underground powerhouse [J]. Tunnelling and Underground Space Technology, 2007, 22(1): 69–79. DOI: 10.1016/j.tust.2006.03.005. [10] 王伟, 李小春. 不耦合装药下爆炸应力波传播规律的试验研究 [J]. 岩土力学, 2010, 31(6): 1723–1728. DOI: 10.16285/j.rsm.2010.06.004.WANG W, LI X C. Experimental study of propagation law of explosive stress wave under condition of decouple charge [J]. Rock and Soil Mechanics, 2010, 31(6): 1723–1728. DOI: 10.16285/j.rsm.2010.06.004. [11] 李桐, 陈明, 叶志伟, 等. 不同耦合介质爆破爆炸能量传递效率研究 [J]. 爆炸与冲击, 2021, 41(6): 062201. DOI: 10.11883/bzycj-2020-0381.LI T, CHEN M, YE Z W, et al. Study on the energy transfer efficiency of explosive blasting with different coupling medium [J]. Explosion and Shock Waves, 2021, 41(6): 062201. DOI: 10.11883/bzycj-2020-0381. [12] CHI L Y, WANG C, ZHANG Z X, et al. Experimental investigation of decoupled charge effect on rock fragmentation by blasting [J]. Rock Mechanics and Rock Engineering, 2022, 55(7): 3791–3806. DOI: 10.1007/s00603-022-02823-w. [13] YANG R S, DING C X, YANG L Y, et al. Study of decoupled charge blasting based on high-speed digital image correlation method [J]. Tunnelling and Underground Space Technology, 2019, 83: 51–59. DOI: 10.1016/j.tust.2018.09.031. [14] YANG J C, LIU Z X, LIU K W, et al. Study on the blasting damage of prestressed rock-like specimens with different coupling mediums [J]. International Journal of Impact Engineering, 2023, 181: 104758. DOI: 10.1016/j.ijimpeng.2023.104758. [15] LI X D, LIU K W, SHA Y Y, et al. Numerical investigation on rock fragmentation under decoupled charge blasting [J]. Computers and Geotechnics, 2023, 157: 105312. DOI: 10.1016/j.compgeo.2023.105312. [16] 王志亮, 李永池. 工程爆破中径向水不耦合系数效应数值仿真 [J]. 岩土力学, 2005, 26(12): 1926–1930. DOI: 10.16285/j.rsm.2005.12.013.WANG Z L, LI Y C. Numerical simulation on effects of radial water-decoupling coefficient in engineering blast [J]. Rock and Soil Mechanics, 2005, 26(12): 1926–1930. DOI: 10.16285/j.rsm.2005.12.013. [17] ZHU Z M, XIE H P, MOHANTY B. Numerical investigation of blasting-induced damage in cylindrical rocks [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(2): 111–121. DOI: 10.1016/j.ijrmms.2007.04.012. [18] YUAN W, WANG W, SU X B, et al. Experimental and numerical study on the effect of water-decoupling charge structure on the attenuation of blasting stress [J]. International Journal of Rock Mechanics and Mining Sciences, 2019, 124: 104133. DOI: 10.1016/j.ijrmms.2019.104133. [19] 袁增森, 徐振洋, 潘博, 等. 不同不耦合系数下花岗岩爆破损伤特性的离散元模拟 [J]. 高压物理学报, 2022, 36(1): 015301. DOI: 10.11858/gywlxb.20210804.YUAN Z S, XU Z Y, PAN B, et al. Discrete element simulation of blasting damage characteristics of granite under different decoupling coefficients [J]. Chinese Journal of High Pressure Physics, 2022, 36(1): 015301. DOI: 10.11858/gywlxb.20210804. [20] LI X D, LIU K W, SHA Y Y, et al. Investigation on radial fracturing around borehole under combined static stress and blasting [J]. Theoretical and Applied Fracture Mechanics, 2023, 127: 104038. DOI: 10.1016/j.tafmec.2023.104038. [21] SHEN W G, ZHAO T, CROSTA G B, et al. Analysis of impact-induced rock fragmentation using a discrete element approach [J]. International Journal of Rock Mechanics and Mining Sciences, 2017, 98: 33–38. DOI: 10.1016/j.ijrmms.2017.07.014. [22] 李洪超. 岩石RHT模型理论及主要参数确定方法研究 [D]. 北京: 中国矿业大学(北京), 2017.LI H C. The study of the rock RHT model and to determine the values of main parameters [D]. Beijing: China University of Mining & Technology, Beijing, 2017. [23] XIE L X, LU W B, ZHANG Q B, et al. Analysis of damage mechanisms and optimization of cut blasting design under high in-situ stresses [J]. Tunnelling and Underground Space Technology, 2017, 66: 19–33. DOI: 10.1016/j.tust.2017.03.009. [24] LI S L, LING T L, LIU D S, et al. Determination of rock mass parameters for the RHT model based on the Hoek-Brown criterion [J]. Rock Mechanics and Rock Engineering, 2023, 56(4): 2861–2877. DOI: 10.1007/s00603-022-03189-9. [25] BORRVALL T, SWEDEN L, RIEDEL W. The RHT concrete model in LS-DYNA [C]//Proceedings of the 8th European LS-DYNA Users Conference. Strasbourg, 2011. [26] 马泗洲, 刘科伟, 杨家彩, 等. 初始应力下岩体爆破损伤特性及破裂机理 [J]. 爆炸与冲击, 2023, 43(10): 105201. DOI: 10.11883/bzycj-2023-0151.MA S Z, LIU K W, YANG J C, et al. Blast-induced damage characteristics and fracture mechanism of rock mass under initial stress [J]. Explosion and Shock Waves, 2023, 43(10): 105201. DOI: 10.11883/bzycj-2023-0151. [27] DEHGHAN BANADAKI M M, MOHANTY B. Numerical simulation of stress wave induced fractures in rock [J]. International Journal of Impact Engineering, 2012, 40/41: 16–25. DOI: 10.1016/j.ijimpeng.2011.08.010. [28] LIU K W, QIU T, LI X D, et al. Deep rock blasting using decoupled charge with different coupling mediums [J]. International Journal of Geomechanics, 2023, 23(8): 04023112. DOI: 10.1061/IJGNAI.GMENG-8179. [29] TAO J, YANG X G, LI H T, et al. Numerical investigation of blast-induced rock fragmentation [J]. Computers and Geotechnics, 2020, 128: 103846. DOI: 10.1016/j.compgeo.2020.103846. [30] MA S Z, LIU K W, YANG J C. Investigation of blast-induced rock fragmentation and fracture characteristics with different decoupled charge structures [J]. International Journal of Impact Engineering, 2024, 185: 104855. DOI: 10.1016/j.ijimpeng.2023.104855. [31] WANG H C, WANG Z L, WANG J G, et al. Effect of confining pressure on damage accumulation of rock under repeated blast loading [J]. International Journal of Impact Engineering, 2021, 156: 103961. DOI: 10.1016/j.ijimpeng.2021.103961. [32] 白金泽. LS-DYNA3D理论基础与实例分析 [M]. 北京: 科学出版社, 2005: 167–170. [33] 李夕兵. 岩石动力学基础与应用 [M]. 北京: 科学出版社, 2014: 357–360. 期刊类型引用(6)
1. 夏卫,张金彪. 破碎块度及磨矿效率影响因素分析. 价值工程. 2025(05): 115-117 .  百度学术
百度学术2. Yuanyuan You,Renshu Yang,Jinjing Zuo,Zhen Yang,Jin Li,Yong Zhao,Haibao Yi. Mechanism of iron ore blasting fracture using axial uncoupled charges. International Journal of Minerals, Metallurgy and Materials. 2025(04): 788-801 .  必应学术
必应学术3. 马泗洲,蒋海明,周朝兰,王明洋,刘科伟. 围压与爆破耦合作用下节理岩体裂纹的扩展行为与影响因素. 爆炸与冲击. 2025(06): 6-23 .  本站查看
本站查看4. 刘欢,潘城. 岩石爆破块度预测方法研究现状及展望. 建井技术. 2025(03): 73-81 .  百度学术
百度学术5. 郑选荣,任凯,刘蕊,李帅良,岳雪茹. 连续梁桥局部桥跨爆破拆除技术研究. 爆破. 2025(02): 129-139 .  百度学术
百度学术6. 蒋长春,姚毅,白宇,李旖晴,毛亚纯. 高寒区不耦合介质装药爆破能量分配模拟研究. 黄金科学技术. 2024(06): 1077-1089 .  百度学术
百度学术其他类型引用(0)
-








 下载:
下载:




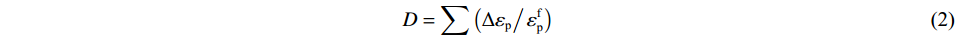
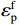
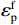
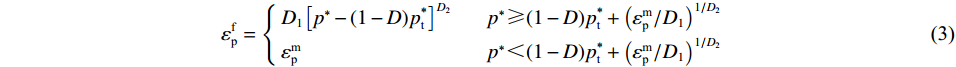
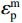



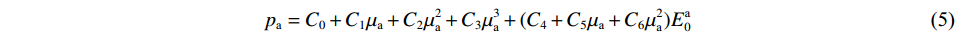
























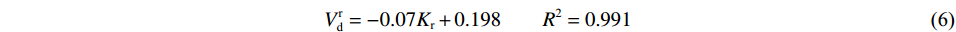





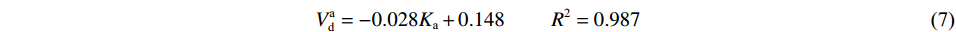




















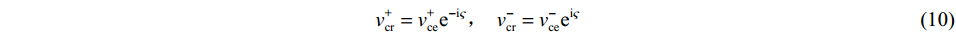


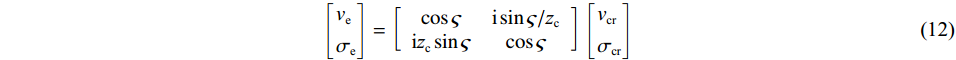
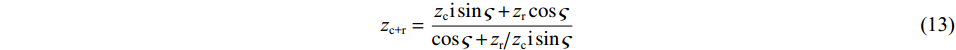






 下载:
下载:



 百度学术
百度学术