Cumulative damage effect and stability analysis of the rock slope with a locked segment under cyclic blasting
-
摘要: 岩石中存在许多微裂纹和微孔洞,这些微裂纹和微孔洞在动荷载作用下会萌生、扩展和聚并,导致岩石失稳和破坏。在进行爆破开挖时,预留岩体会受到循环爆破产生的动荷载影响,产生累积损伤,从而导致岩体强度降低,甚至破坏。为了模拟这一物理过程,将现有的能够较好地描述岩石动态损伤的岩石动态损伤本构模型通过二次开发嵌入到FLAC中,用于分析锁固型岩质边坡在循环爆破作用下的损伤效应及稳定性。结果表明:考虑岩质边坡累积损伤效应后,随着循环爆破次数的增加,边坡稳定性逐渐降低。对于锁固型岩质边坡,锁固段的破坏首先发生在两端,然后向中间扩散,岩体在其中呈现递进破坏模式。由于考虑了岩质边坡的累积损伤,每次爆破后边坡的安全系数都会减小。当不考虑累积损伤时,边坡的安全系数基本不变。另外,锁固段在软弱夹层中的位置影响边坡的破坏模式和稳定性。因此,在进行类似工程活动时,应考虑岩体的累积损伤效应,避免工程事故的发生。Abstract: There are many microcracks and micropores in the rock, which will initiate, propagate, and coalescence under dynamic loading, leading to rock instability and failure. When blasting excavation is carried out, the retained rock mass will be subjected to the dynamic loading generated by cyclic blasting, resulting in cumulative damage, which will lead to the reduction of the rock mass strength, and even failure. In order to simulate this physical process, the existing rock dynamic damage constitutive model, which could perfectly describe the rock dynamic damage induced by blasting, was embedded into FLAC through secondary development to analyze the cumulative damage of rock mass under cyclic blasting. And then it was adopted to simulate the damage effect and stability of the rock slope with the locked segment under cyclic blasting. The stability of the slope under cyclic blasting was determined by the displacement criterion method, and the safety factor of the slope after each blasting was obtained by the strength reduction method. Finally, the relationship between the failure mode and stability of the slope and the location of the locked segment was discussed by analyzing the damage, displacement field, and safety factor of the numerical models for different locations of the locked segment in the soft interlayer. The results show that the slope stability gradually decreases with increasing the number of cyclic blasting after considering the cumulative damage effect of the rock slope. For the rock slope with the locked segment, the damage of the locked segment firstly occurs at both ends, and then propagates to the middle, in which the rock mass shows a progressive failure mode. Because the cumulative damage of the rock slope is considered, the safety factor of the slope will decrease after each blasting. When the cumulative damage is not considered, the safety factor of the slope is basically unchanged. The failure mode of the rock slope with a locked segment under cyclic blasting is the combination of dynamic tensile failure and shear failure caused by rock mass slip. The location of the locked segment in the weak interlayer affects the failure mode and stability of the slope. Therefore, when carrying out similar engineering activities, the cumulative damage effect of rock mass should be considered to avoid engineering accidents.
-
自然界中的边坡在受到动荷载作用时往往容易发生滑坡灾害[1-2]。在工程爆破中,虽然单次爆破产生的动荷载持续时间短,破坏程度弱,难以直接引起滑坡。然而,采矿工程中频繁的生产爆破不可避免地会对边坡的稳定性产生不利的累积影响,特别是当边坡内部存在软弱夹层时,在频繁的动载作用下,滑坡发生的可能性增大。一些受爆破开挖活动影响而发生的滑坡,对人类生命财产安全造成了严重的损失[3-4],因此,对爆破作用下含软弱结构面边坡的稳定性研究极为重要。
锁固段是指岩质边坡潜在滑动面上具有较大承载力的地质结构,当边坡上的危岩体受重力或外界因素影响而产生软弱结构面滑动的趋势时,锁固段可以抑制危岩体的滑动[5]。然而在长时间的扰动下,可能会造成锁固段的断裂破坏,从而引发边坡失稳滑动[6]。Chen等[7]在对新滩滑坡的研究中发现,由降雨等因素造成的锁固段累积损伤引起了边坡的失稳破坏。Li等[8]发现,露天矿的开采活动破坏了滑坡前缘的抗倾倒锁固段,从而造成了大型滑坡的发生。Tang等[9]认为鸡尾山岩崩的原因是锁固段经过长时间的损伤弱化,以致于无法抑制滑体滑动。以上研究都表明当锁固段丧失锁固能力时,边坡极有可能发生失稳破坏。地震或爆破等动态扰动作用下也会造成锁固段的损伤,甚至破裂[10]。然而目前的研究多集中在单次动态荷载作用下边坡的破坏,而对循环动态荷载作用下边坡的累积损伤机理和破坏模式的研究较少。在露天采矿或水利水电工程中,循环爆破开挖引起的边坡失稳也经常发生[11-12]。
岩石在宏观上常常被认为是一种连续介质,然而从微观角度看,岩石内部存在大量的微裂纹和微孔洞[13]。在动态作用下,岩石内部的微裂纹和微孔洞常常会扩展、贯通,从而造成岩石的断裂与破坏,从损伤力学的角度讲,这一过程被视为岩石的累积损伤过程。损伤力学最初用来研究金属材料强度下降的现象[14-15],而后许多学者将损伤力学应用于岩石爆破损伤问题,并建立了一些经典的岩石动态损伤本构模型[16]。Grady等 [17]认为岩石中的初始裂纹服从Weibull分布,其中一些裂纹在外荷载作用下会被激活并扩展,由此提出了岩石在爆破荷载下的GK损伤模型。Taylor等[18]认为岩石的损伤机制归因于岩石内部的微裂纹,并将岩石的动态断裂过程处理为连续的累积损伤,建立了TCK损伤模型。Yang等[19]和Liu等[20]认为可以通过概率形式表示岩石在动态荷载下微裂纹和微孔洞的起裂、扩展而导致的岩石损伤过程,并建立了岩石爆破的损伤本构模型。基于细观力学,Zhou[21]得到了能反映岩石局部变形与损伤的岩石动态损伤本构模型。基于TCK模型,刘红岩等[22]建立了一种考虑岩石宏细观缺陷的动态损伤本构模型。而随着计算机技术的发展,利用数值模拟方法研究动态荷载下岩体损伤效应及稳定性已逐渐成为一种趋势。相较于传统的理论方法与试验方法,数值模拟更加经济方便,计算结果也更加直观。例如,Song等[23]基于牛顿第二定律建立了岩石爆破断裂的离散模型,并在模拟中观察了岩石的损伤过程。Amichai等[24]利用有限元-离散元耦合的方法研究了圆形巷道爆破造成的损伤。Hu等[25]将光滑粒子流体动态学引入LS-DYNA,再现了岩体的完整爆破响应,所得爆破损伤范围与试验结果符合较好。胡英国等[26]利用LS-DYNA软件的二次开发技术,对爆破保留岩体的损伤演化进程进行了数值模拟分析。王磊等[27]利用AUTODYN和ABAQUS软件,研究了动荷载作用下裂纹的扩展路径、动态起裂韧度和裂纹扩展过程中的动态扩展韧度。周磊等[28]利用AUTODYN分析了裂隙岩体在动荷载作用下的动态断裂特性和止裂机理。
爆破对岩体的影响主要分为对爆破近区岩体的影响和对远区岩体的影响。对爆破近区岩体的影响主要表现为爆炸应力波对岩体的冲击损伤,如隧道开挖爆破对围岩的损伤破坏[29-30]。对爆破远区岩体的影响主要表现为爆破地震效应对岩体的振动损伤,如地下爆破引起的振动波对高陡边坡的影响[31]。在岩石爆破工程中,往往会进行数十次或数百次的爆破作业。已有学者研究了循环爆破作用对爆破近区岩体的影响[32-33],然而目前对爆破远区岩体的振动损伤研究多集中在单次爆破的影响,忽略了多次爆破对岩体的累积损伤作用。另外,目前常用的爆破损伤分析软件LS-DYNA很难得到边坡稳定性系数随爆破次数累积的降低过程,而有限差分软件FLAC/FLAC3D在边坡动态稳定性计算中被广泛采用[34-35],但其中并没有可以有效描述岩石损伤性质的本构模型。因此,利用二次开发功能将自定义本构模型嵌入其中是一种有效的方法[36-38]。
综上所述,鉴于已有研究的不足之处,利用有限差分软件FLAC建立顺层直剪式锁固型岩质边坡模型,通过二次开发将动态损伤本构模型嵌入动态计算过程,以探究多次爆破作用下锁固型岩质边坡的累积损伤效应及其对边坡变形和稳定性的影响。
1. 岩石动态损伤本构模型及其在FLAC中的实现
将Yang-Liu动态损伤本构模型[19-20]与Mohr-Coulomb弹塑性本构模型[39]耦合,利用C++语言通过二次开发的形式生成考虑损伤的.dll本构模型文件,并将其嵌入FLAC程序中,以在动态计算过程中考虑岩石的累积损伤效应:
1.1 动态作用下的岩石损伤变量
采用各向同性损伤模型,损伤变量D以裂纹密度的概率形式表示,通过概率形式表示动态荷载下的岩石裂纹数量及损伤过程。
D=1−e−c2d (1) cd 表示单位体积内包含的裂纹数:cd={α(ε−εcr)βtε≥εcr0ε<εcr (2) 式中:
ε 为等效拉应变,εcr 为临界拉应变,α 、β 为材料常数,t 为到达断裂应力所经历的时间。ε=√ε21+ε22+ε23 (3) εcr=1−2vEσst (4) 式中:
εi 为主应变,εi=|εi|+εi2(i=1,2,3) ;v 为泊松比;E 为等效弹性模量;σst 为静态抗拉强度。1.2 动态损伤本构模型在FLAC中的实现
在FLAC内置的Mohr-Coulomb本构模型的基础上加入损伤变量以实现动态损伤本构模型的二次开发。在FLAC中,每一时步的应变以增量形式表示,胡克定律增量表达式为:
dσij=λδijdεij+2Gdεij i,j=1,2,3 (5) 弹塑性本构关系的微分方程可以写为:
dσij=(De−Dp)dεij (6) 加入损伤变量后:
dσdij=(De−Dpd)dεij (7) 式中:
De 为弹性矩阵,Dp 为塑性矩阵,Dpd 为损伤矩阵。Dpd [39]可表示为Dpd=(1−D+D3δij)Dp+(D−D3δij)De (8) 1.3 程序流程
二次开发的程序流程如图1所示。在每一步的迭代计算中,由程序内嵌变量获得单元的应变增量,从而计算出单元的累积应变,而后根据式(3)~(4)计算得到等效拉应变和临界拉应变。判断单元的状态,若单元已经发生了拉裂破坏,则设置单元的损伤变量为1,若单元未发生拉裂破坏,则根据式(2)计算单元的裂纹密度,而后根据式(1)计算单元的损伤值。损伤计算完成后,根据1.2节将损伤值加入到Mohr-Coulomb本构模型中,从而实现在计算过程中考虑单元的动态损伤。
2. 模型验证
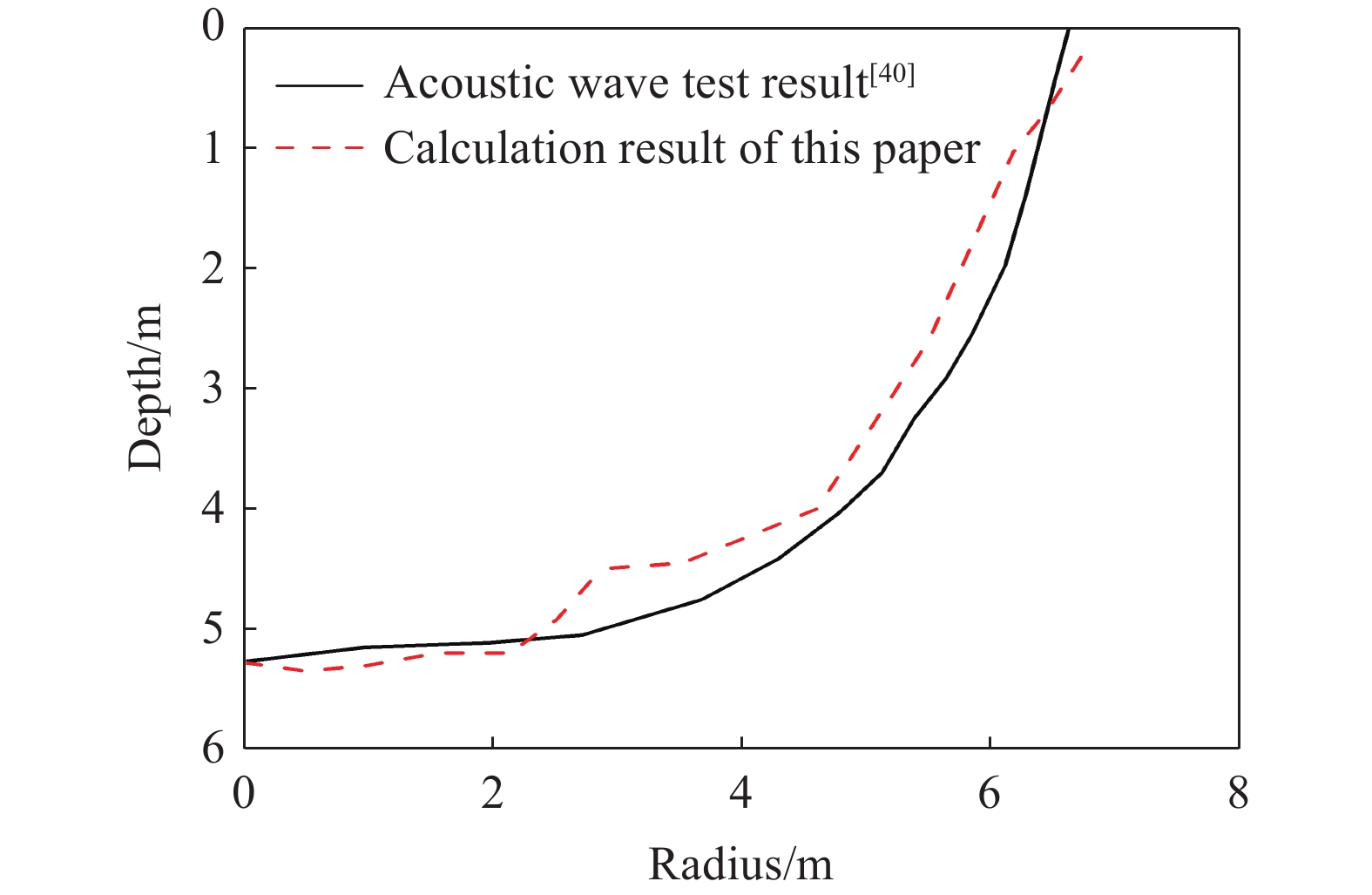
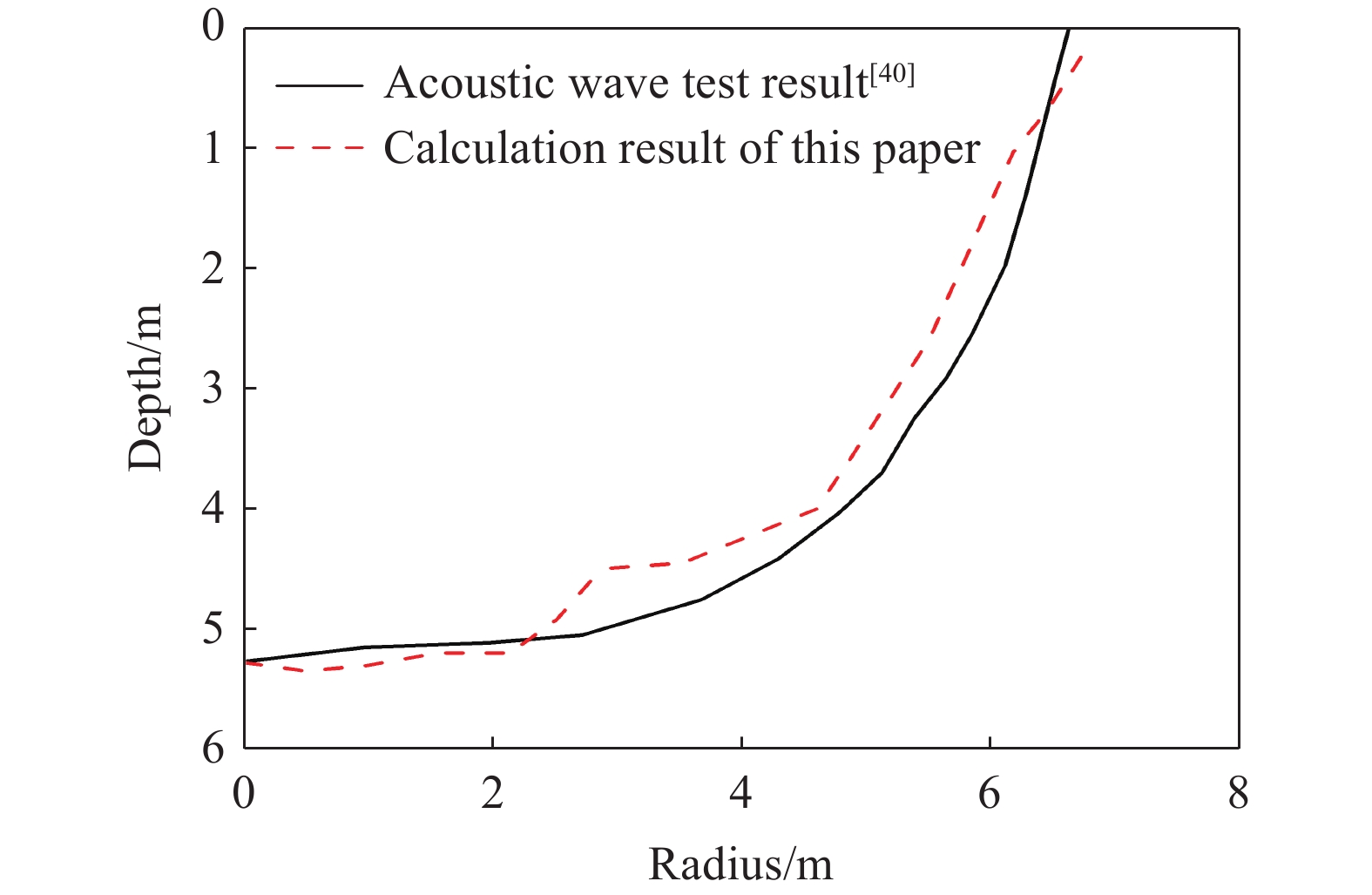
在进行数值计算之前,通过已有文献[40]对本文中的动态损伤本构模型进行验证。夏祥等[40]采用声波试验方法估算了爆破作用下岩体的损伤范围,并首次用试验结果验证了岩体的动态损伤本构模型。该计算模型以某核电站工程基础爆破开挖为例。模型为1/4圆柱体,半径为8 m,高度为8 m。边界条件设为透射边界,装药高度3 m。采用的动态损伤本构模型计算参数如表1所示。图2为计算得到的爆炸荷载下岩体的损伤范围与声波试验测得的损伤范围的对比曲线,可以看到计算结果与试验结果整体一致,由此验证了本文中所使用本构模型的准确性。
3. 数值计算模型
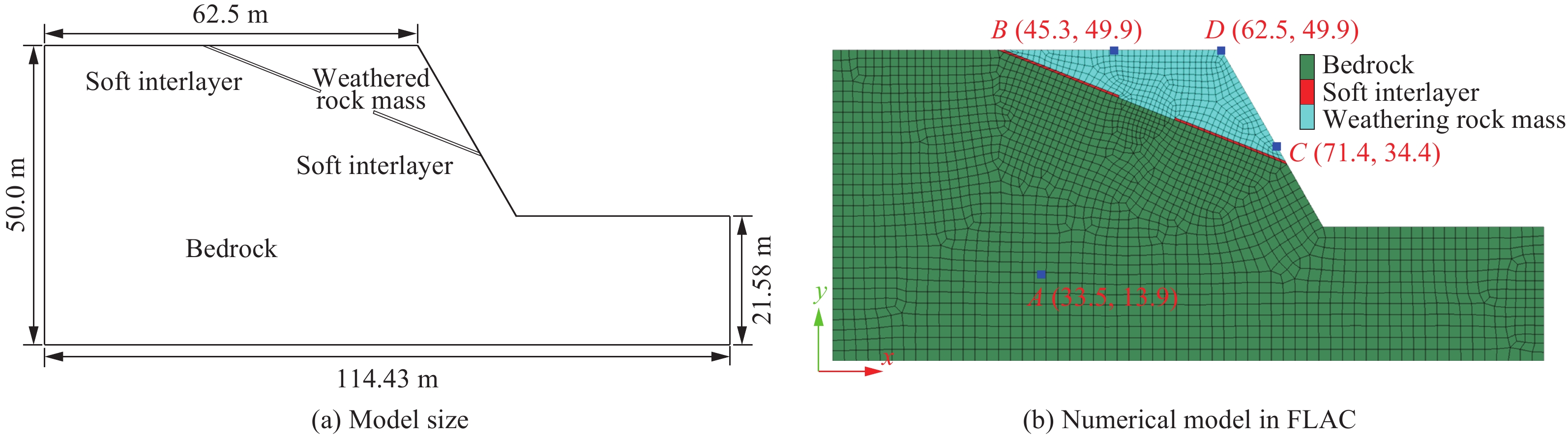
数值计算模型为顺层直剪式锁固型边坡,边坡模型如图3所示。模型由基岩、风化岩体和软弱结构面组成,边坡滑面上下部分沿软弱结构面滑动,中间为锁固段。计算模型采用前述动态损伤本构模型,此外,在循环动态的作用下张拉破坏只是岩体损伤的一部分,由岩体滑动引起的剪切破坏同样会对岩体造成损伤。因此,在计算过程中,同样将发生剪切破坏的单元的损伤值设置为1。参数取值如表2所示。
表 2 模型物理力学参数Table 2. Physical parameters for the models岩体类型 密度/(kg·m−3) 弹性模量/GPa 泊松比 黏聚力/MPa 内摩擦角/(°) 抗拉强度/MPa 基岩 2700 20.0 0.24 5.00 42 3.00 风化岩体及锁固段 2500 0.8 0.25 0.20 34 0.20 软弱夹层 2200 0.6 0.25 0.04 18 0.02 模型周围采用自由场边界,阻尼选用局部阻尼,阻尼系数为0.125。所用动态波为通过爆破试验采集的水平加速度时程曲线[41] ,爆破试验的爆破点位置和相关参数可参考文献[41]。通过转化,将动态波以速度时程曲线的形式输入到边坡底部。为方便计算与对比,每次爆破产生的动态波的形式相同,单次动态波的加速度及速度时程曲线如图4所示。共进行10次爆破,每次动态作用的时间为3 s,在进行计算之前对动态波作滤波和基线校正处理。
4. 计算结果
4.1 锁固型边坡的损伤效应与稳定性分析
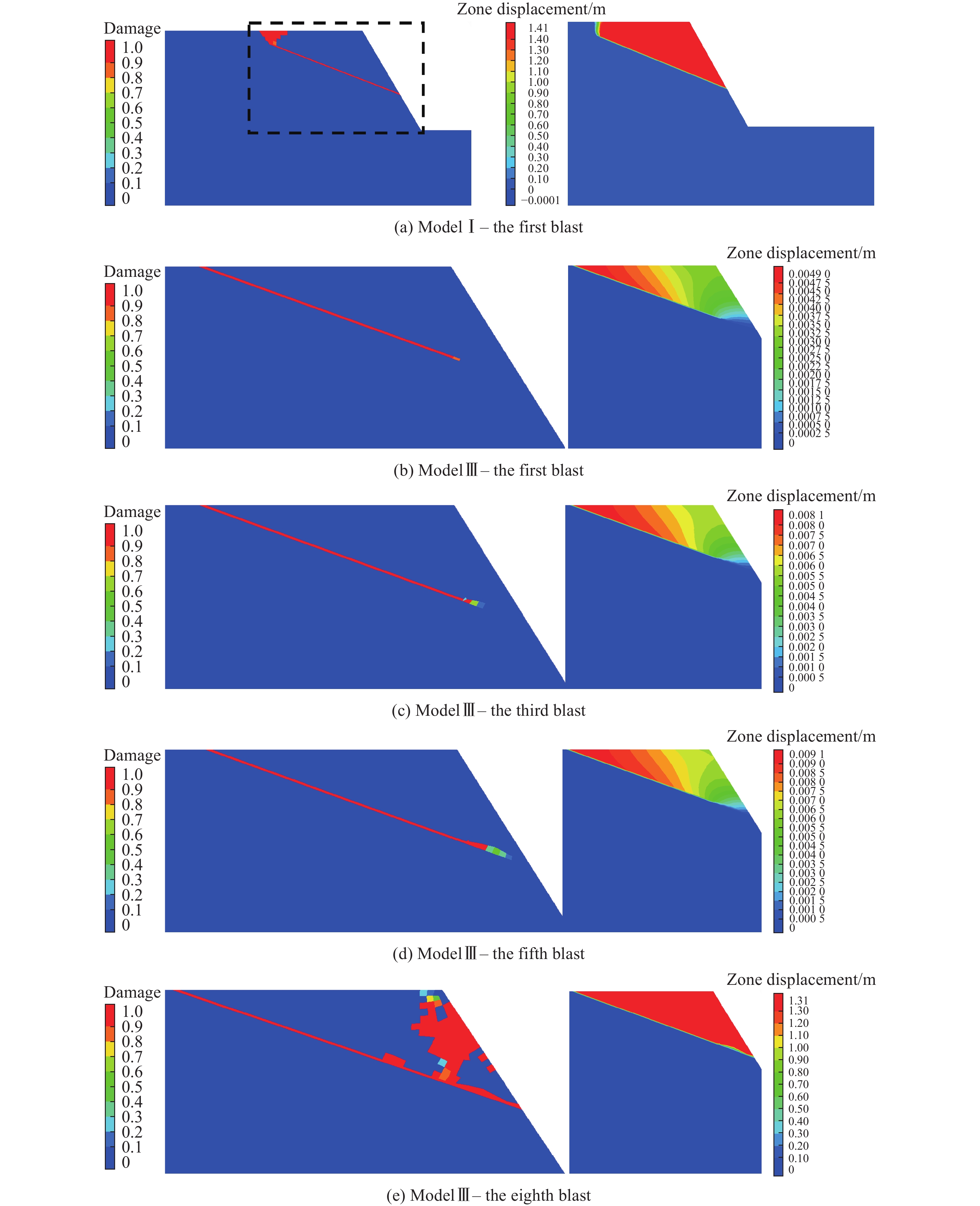
共计算10次爆破作用,当第8次爆破作用结束时,计算不再收敛。图5为采用动态损伤本构模型时,每个爆破周期结束后锁固型岩质边坡的位移场和位移矢量场,其中云图表示位移场,箭头表示位移矢量场。可以看到,在爆破动态波的作用下,风化岩体整体呈向下滑动的趋势,由于锁固段的锁固作用,风化岩体的前缘位移大于后缘位移,随着爆破次数的累积,风化岩体呈渐进破坏的模式,直到第8次爆破作用结束后,锁固段破裂,风化岩体整体失稳滑动。图6为每次爆破作用结束后锁固型边坡的损伤分布的局部放大图,可以看到,第1次爆破作用结束后,损伤主要分布在软弱夹层岩体,随着爆破次数的增加,锁固段部分出现损伤,并由锁固段两端逐渐向内贯通,最终锁固段岩体完全损伤,坡体发生失稳滑动。由图中还可以看出,锁固段下缘的损伤程度要大于锁固段上缘的损伤程度,这主要是由于在坡体未失稳之前,风化岩体的下缘部分产生了较大的位移,拉动整个风化岩体向下滑动,造成锁固段下缘出现了较大的损伤。
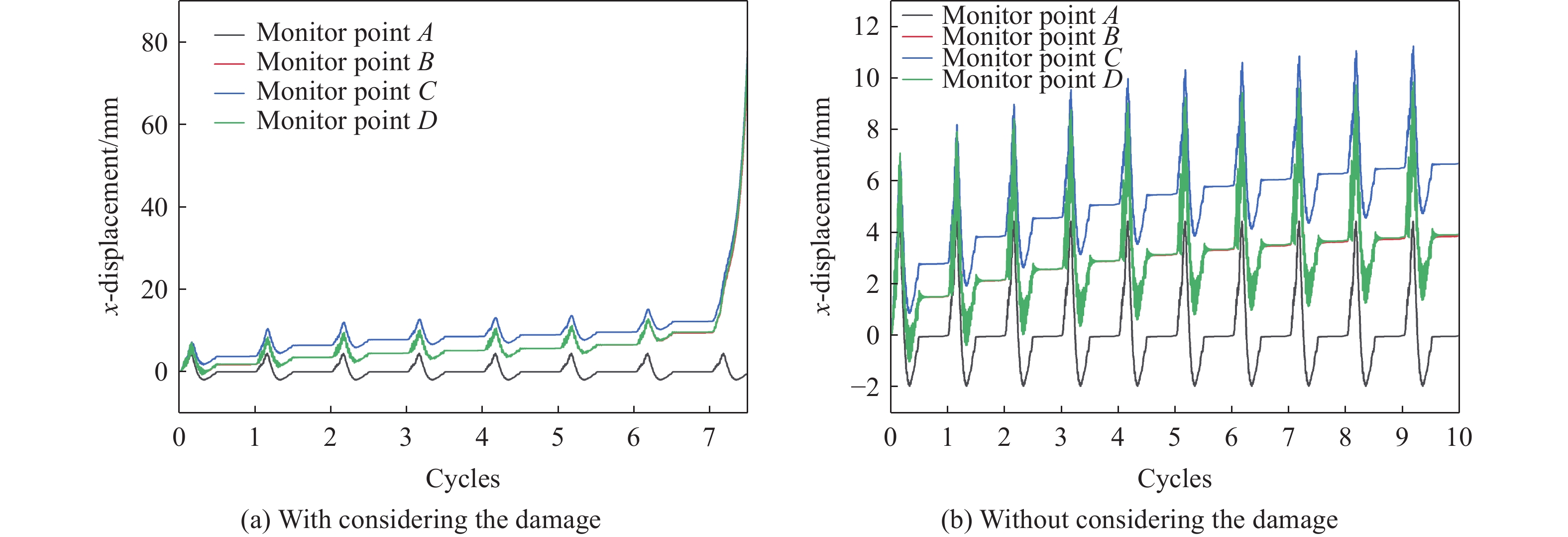
位移判据法常用于判别动态作用下边坡的稳定性,通过在潜在滑体上设置位移监测点以判别锁固型边坡是否达到失稳滑动状态,监测点位置如图3(b)所示。图7(a)为采用动态损伤本构模型时各个监测点的水平位移曲线,可以看到,风化岩体在每次爆破作用结束后都产生了一定的永久位移,但是在7次爆破作用后位移都趋于稳定不再增大,而当第8次爆破作用结束后,监测点的位移不断增大,此时数值计算也不再收敛,因此可以判定锁固型边坡此时已发生失稳滑动。图7(b)为不考虑动态损伤时锁固型边坡监测点的水平位移曲线,可以看到,10次爆破作用结束后,监测点的水平位移趋于稳定,因此此时锁固型边坡未失稳破坏。由此可知,岩体的损伤作用会使边坡的稳定性降低,在进行此类工程模拟时,不应忽略动态作用对岩体造成的累积损伤作用。
4.2 不同爆破次数下锁固型边坡的安全系数
将强度折减法应用于动态分析,可计算出边坡在动态作用下的安全系数[42]。对于岩质边坡而言,抗拉强度也是影响边坡稳定性的重要因素。因此,在每次爆破计算中,通过对软弱夹层与风化岩体的黏聚力、内摩擦角和抗拉强度以一定系数进行折减,来探究不同爆破次数下锁固型边坡的安全系数,需要说明的是,强度折减法仅用于计算边坡的安全系数,折减后的强度不会代入到下一次的爆破计算中。
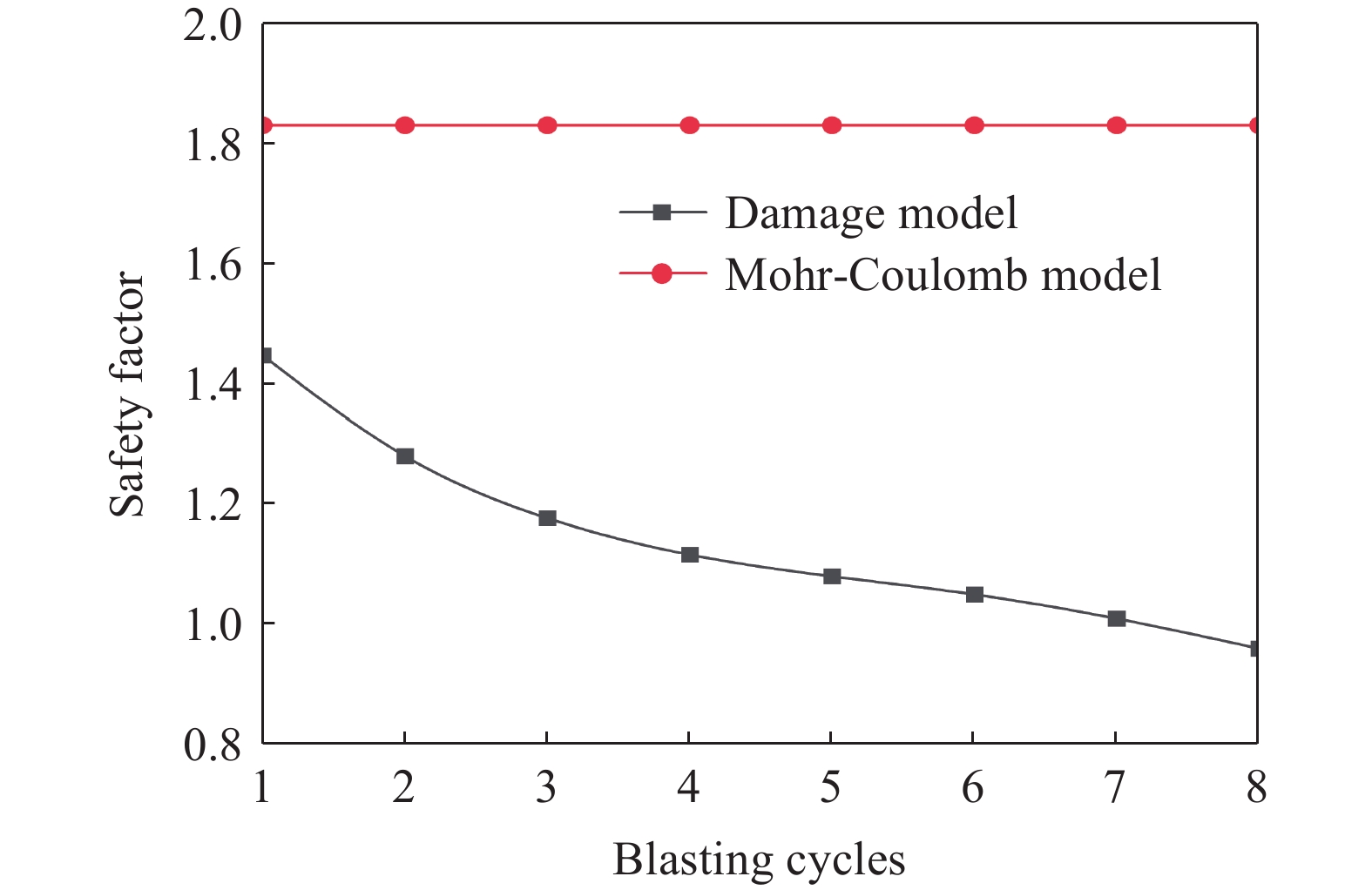
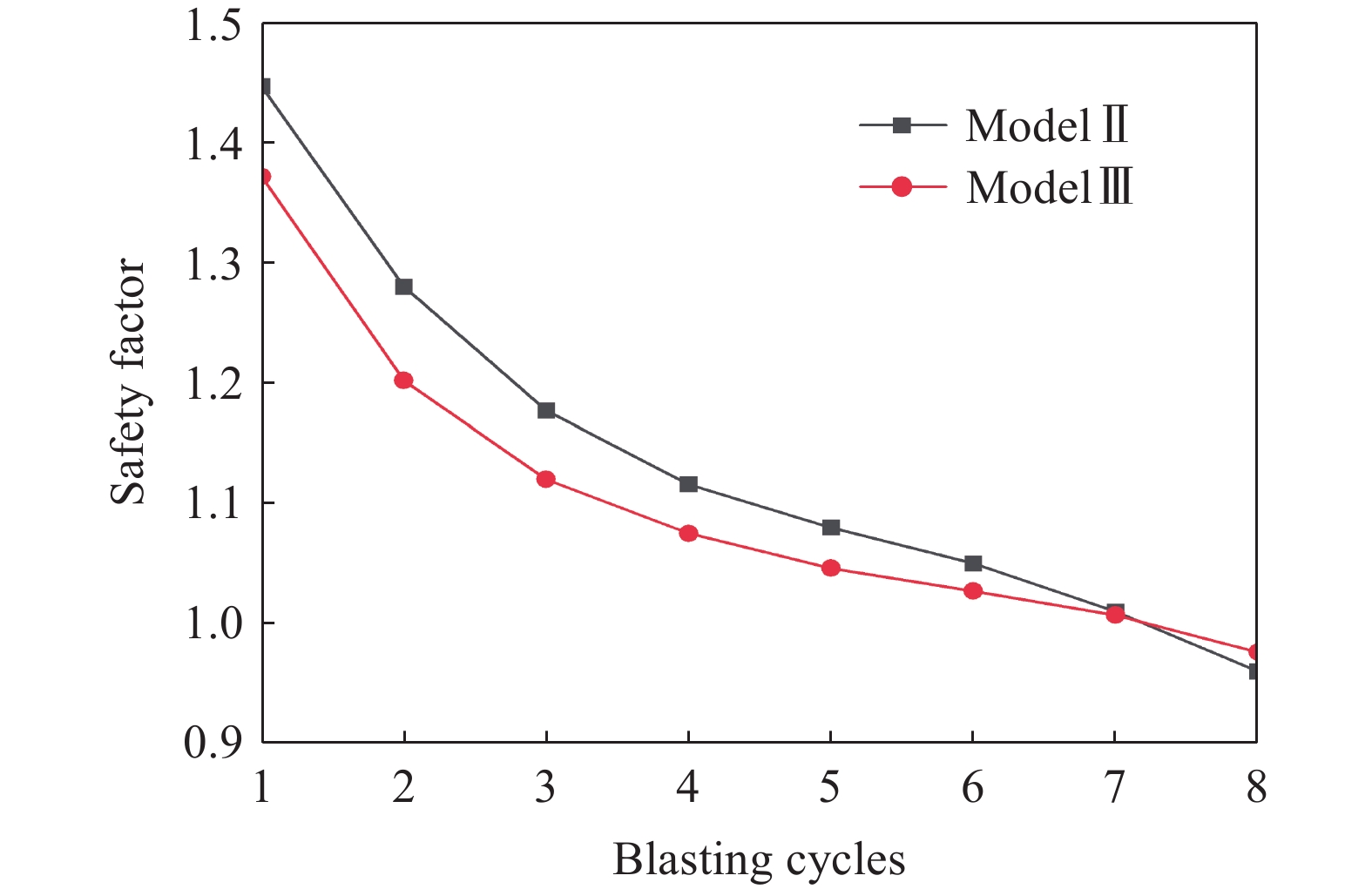
首先以一次爆破作用为例,来说明爆破作用下锁固型边坡安全系数的计算方法。选取监测点C作为判定点,图8(a)为不同折减系数下C点的水平位移曲线。可以看到,当折减系数小于1.45时,监测点水平位移在动态作用结束后保持不变,当折减系数为1.45时,监测点水平位移在动态作用结束后仍不断增大。由图8(b)可以看到,折减系数增加到1.45时,爆破动态作用结束后监测点的水平位移出现突变。因此可以判定在受到一次爆破作用后,锁固型边坡的安全系数为1.44。利用以上方法分别计算了锁固型边坡在不同爆破作用次数时的安全系数。由图9可以看出,随着爆破作用次数的增加,不考虑岩体的累积损伤效应时,锁固型边坡的安全系数基本不变,而当考虑岩体的累积损伤效应时,锁固型边坡的安全系数在不断减小。
5. 讨 论
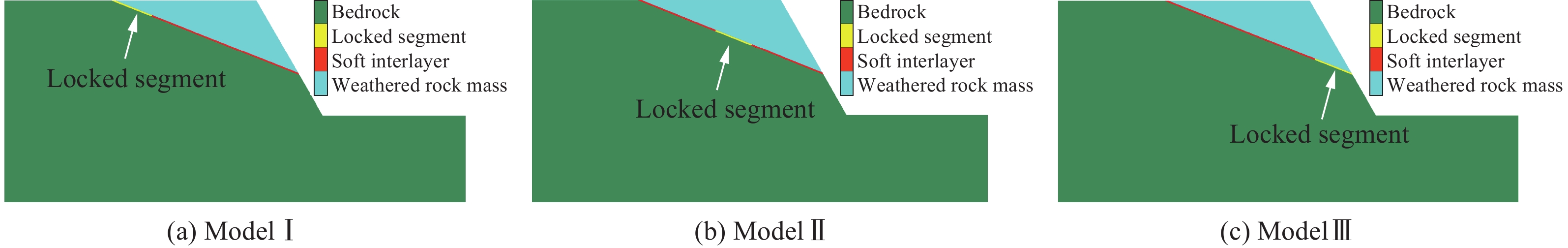
边坡的破坏模式和稳定性与锁固段的结构形状、规模和位置有关。对于顺层边坡,Bao等[43]揭示了软弱夹层锁固段比例对边坡破坏模式的影响。然而,在循环爆破作用下,软弱夹层中锁固段的位置对边坡渐进破坏的影响尚不清楚。为此,建立软弱夹层中锁固段不同位置的数值模型(图10),分别为锁固段位于软弱夹层上部时(Model Ⅰ)、锁固段位于软弱夹层中部时(Model Ⅱ)和锁固段位于软弱夹层下部时(Model Ⅲ)的边坡模型,其中Model Ⅱ已在第4节中进行了分析。
图11为3种边坡在循环爆破作用下的损伤分布云图。Model Ⅰ 在第1次爆破循环后立即发生失稳破坏。在动态作用和滑动岩体的牵引作用下,风化岩体产生拉伸损伤直至完全破坏,导致锁固段无法防止边坡滑动。Model Ⅲ在循环爆破8次后失稳,锁固段损伤在靠近软弱夹层的一端开始累积,并逐渐向自由面发展。与Model Ⅱ不同的是,在Model Ⅲ底部锁固段的影响下,风化岩体后缘位移明显大于前缘位移,后缘岩体不断推进,在爆破产生的拉应力和后缘岩体推进产生的剪应力的作用下,锁固段逐渐损伤,直至完全破坏。此外,从边坡稳定性来看,Model Ⅰ在第1次爆破时不稳定,稳定性最差,而Model Ⅲ在循环爆破时的安全系数低于Model Ⅱ(图12)。
6. 结 论
在FLAC中引入了动态损伤本构模型,通过与已有文献的研究结果对比验证了模型的正确性,利用岩石动态损伤本构模型研究了循环爆破动态作用下顺层直剪式锁固型边坡的损伤效应及稳定性。得到的主要结论如下。
(1)顺层直剪式锁固型边坡在受到多次爆破动态作用后呈渐进破坏的模式,同时失稳时具有脆性和突然性的特点。风化岩体在每次爆破作用结束后都产生了永久位移,由于锁固段的锁固作用,风化岩体的前缘位移较大,锁固段损伤由两端向内贯通,在第8次爆破作用后,风化岩体失稳滑动。
(2)利用强度折减法计算了边坡在每次爆破作用结束时的安全系数,在不考虑岩体的损伤效应时,边坡的安全系数基本不变,而当考虑岩体的损伤效应时,边坡的安全系数不断减小。
(3)软弱夹层中锁固段的位置影响边坡的破坏模式和稳定性。当锁固段位于软弱夹层上部时,边坡的稳定性最差,当锁固段位于软弱夹层中部时,边坡的稳定性最好。循环爆破作用下,锁固型顺层岩质边坡的破坏模式为动态张拉破坏与岩体滑移引起的剪切破坏相结合。
在实际工程活动中,边坡在受到几十次或几百次爆破作用后依然稳定。在本文的模拟中,为了节省计算量,在降低边坡岩体强度参数的前提下研究了岩体的损伤效应对边坡稳定性的影响,但计算结果依然可以对实际工程活动起到警示。研究表明,边坡岩体的损伤效应会显著降低边坡的稳定性。因此在进行爆破工程活动时,应该考虑受影响边坡岩体的累积损伤效应,避免由于爆破动态波影响造成的边坡失稳现象的发生。
-
密度/(kg·m−3) 弹性模量/GPa 泊松比 黏聚力/MPa 内摩擦角/(°) 抗拉强度/MPa 剪胀角/(°) α β 2700 68.69 0.228 27.7 55 5.6 12 3.15×106 2 表 2 模型物理力学参数
Table 2. Physical parameters for the models
岩体类型 密度/(kg·m−3) 弹性模量/GPa 泊松比 黏聚力/MPa 内摩擦角/(°) 抗拉强度/MPa 基岩 2700 20.0 0.24 5.00 42 3.00 风化岩体及锁固段 2500 0.8 0.25 0.20 34 0.20 软弱夹层 2200 0.6 0.25 0.04 18 0.02 -
[1] 黄润秋. 汶川8.0级地震触发崩滑灾害机制及其地质力学模式 [J]. 岩石力学与工程学报, 2009, 28(6): 1239–1249. DOI: 10.3321/j.issn:1000-6915.2009.06.021.HUANG R Q. Mechanism and geomechanical modes of landslide hazards triggered by Wenchuan 8.0 earthquake [J]. Chinese Journal of Rock Mechanics and Engineering, 2009, 28(6): 1239–1249. DOI: 10.3321/j.issn:1000-6915.2009.06.021. [2] ROBACK K, CLARK M K, WEST A J, et al. The size, distribution, and mobility of landslides caused by the 2015 M(w)7.8 Gorkha earthquake, Nepal [J]. Geomorphology, 2018, 301: 121–138. DOI: 10.1016/j.geomorph.2017.01.030. [3] WANG M, MA G T, WANG F. Numerically investigation on blast-induced wave propagation in catastrophic large-scale bedding rockslide [J]. Landslides, 2021, 18: 785–797. DOI: 10.1007/s10346-020-01537-w. [4] CUI F P, LI B, XIONG C, et al. Dynamic triggering mechanism of the Pusa mining-induced landslide in Nayong County, Guizhou Province, China [J]. Geomatics, Natural Hazards & Risk, 2022, 13(1): 123–147. DOI: 10.1080/19475705.2021.2017020. [5] XUE L, QIN S Q, PAN X H, et al. A possible explanation of the stair-step brittle deformation evolutionary pattern of a rockslide [J]. Geomatics, Natural Hazards & Risk, 2017, 8(2): 1456–1476. DOI: 10.1080/19475705.2017.1345793. [6] HUANG R Q. Mechanisms of large-scale landslides in China [J]. Bulletin of Engineering Geology and the Environment, 2012, 71(1): 161–170. DOI: 10.1007/s10064-011-0403-6. [7] CHEN H R, QIN S Q, XUE L, et al. Why the Xintan landslide was not triggered by the heaviest historical rainfall: mechanism and review [J]. Engineering Geology, 2021, 294: 106379. DOI: 10.1016/j.enggeo.2021.106379. [8] LI S Y, LI D D, LIU H D, et al. Formation and failure mechanism of the landslide: a case study for Huaipa, western Henan, China [J]. Environmental Earth Sciences, 2021, 80(15): 478. DOI: 10.1007/s12665-021-09781-6. [9] TANG H M, ZOU Z X, XIONG C R, et al. An evolution model of large consequent bedding rockslides, with particular reference to the Jiweishan rockslide in southwest China [J]. Engineering Geology, 2015, 186: 17–27. DOI: 10.1016/j.enggeo.2014.08.021. [10] DONG J Y, WANG C, HUANG Z Q, et al. Dynamic response characteristics and instability criteria of a slope with a middle locked segment [J]. Soil Dynamics and Earthquake Engineering, 2021, 150: 106899. DOI: 10.1016/j.soildyn.2021.106899. [11] DAI Z Y, ZHANG L, WANG Y L, et al. Deformation and failure response characteristics and stability analysis of bedding rock slope after underground adverse slope mining [J]. Bulletin of Engineering Geology and the Environment, 2021, 80(6): 4405–4422. DOI: 10.1007/s10064-021-02258-7. [12] WANG W C, YAN Y F, QU Y, et al. Shallow failure of weak slopes in Bayan Obo West Mine [J]. International Journal of Environmental Research and Public Health, 2022, 19(5): 9755. DOI: 10.3390/ijerph19159755. [13] WU Z J, JI X K, LIU Q S, et al. Study of microstructure effect on the nonlinear mechanical behavior and failure process of rock using an image-based-FDEM model [J]. Computers and Geotechnics, 2020, 121: 103480. DOI: 10.1016/j.compgeo.2020.103480. [14] KACHANOV L. Time of rupture process under creep conditions [J]. Izvestiia Akademii Nauk SSSR, Otdelenie Teckhnicheskikh Nauk, 1958, 8: 26–31. [15] LEMAITRE J. How to use damage mechanics [J]. Nuclear Engineering and Desig, 1984, 80(2): 233–245. DOI: 10.1016/0029-5493(84)90169-9. [16] ZHANG Q B, ZHAO J. A review of dynamic experimental techniques and mechanical behaviour of rock materials [J]. Rock Mechanics and Rock Engineering, 2014, 47: 1411–1478. DOI: 10.1007/s00603-013-0463-y. [17] GRADY D E, KIPP M E. Continuum modelling of explosive fracture in oil shale [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1980, 17(3): 147–157. DOI: 10.1016/0148-9062(80)91361-3. [18] TAYLOR L M, CHEN E P, KUSZMAUL J S. Microcrack-induced damage accumulation in brittle rock under dynamic loading [J]. Computer Methods in Applied Mechanics and Engineering, 1986, 55(3): 301–320. DOI: 10.1016/0045-7825(86)90057-5. [19] YANG R, BAWDEN W F, KATSABANIS P D. A new constitutive model for blast damage [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1996, 33(3): 245–254. DOI: 10.1016/0148-9062(95)00064-X. [20] LIU L Q, KATSABANIS P D. Development of a continuum damage model for blasting analysis [J]. International Journal of Rock Mechanics and Mining Sciences, 1997, 34(2): 217–231. DOI: 10.1016/S0148-9062(96)00041-1. [21] ZHOU X P. Analysis of the localization of deformation and the complete stress-strain relation for mesoscopic heterogeneous brittle rock under dynamic uniaxial tensile loading [J]. International Journal of Solids and Structures, 2004, 41(5/6): 1725–1738. DOI: 10.1016/j.ijsolstr.2003.07.007. [22] 刘红岩, 李俊峰, 裴小龙. 单轴压缩下断续节理岩体动态损伤本构模型 [J]. 爆炸与冲击, 2018, 38(2): 316–323. DOI: 10.11883/bzycj-2016-0261.LIU H Y, LI J F, PEI X L. A dynamic damage constitutive model for rockmass with intermittent joints under uniaxial compression [J]. Explosion and Shock Waves, 2018, 38(2): 316–323. DOI: 10.11883/bzycj-2016-0261. [23] SONG J, KIM K. Micromechanical modeling of the dynamic fracture process during rock blasting [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1996, 33(4): 387–391, 393–394. DOI: 10.1016/0148-9062(95)00072-0. [24] AMICHAI M, DAVIDE E. Modelling of blast-induced damage in tunnels using a hybrid finite-discrete numerical approach [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2014, 6(6): 565–573. DOI: 10.1016/j.jrmge.2014.09.002. [25] HU Y G, LU W B, CHEN M, et al. Numerical simulation of the complete rock blasting response by SPH-DAM-FEM approach [J]. Simulation Modelling Practice and Theory, 2015, 56: 55–68. DOI: 10.1016/j.simpat.2015.04.001. [26] 胡英国, 卢文波, 陈明, 等. 岩体爆破近区临界损伤质点峰值震动速度的确定 [J]. 爆炸与冲击, 2015, 35(4): 547–554. DOI: 10.11883/1001-1455(2015)04-0547-08.HU Y G, LU W B, CHEN M, et al. Determination of critical damage PPV near the blast hole of rock-mass [J]. Explosion and Shock Waves, 2015, 35(4): 547–554. DOI: 10.11883/1001-1455(2015)04-0547-08. [27] 王磊, 朱哲明, 周磊, 等. 冲击载荷作用下圆孔缺陷对裂纹动态扩展行为的影响规律 [J]. 爆炸与冲击, 2021, 41(8): 083105. DOI: 10.11883/bzycj-2021-0062.WANG L, ZHU Z M, ZHOU L, et al. Influence of circular hole defect on dynamic crack propagation behavior under impact loads [J]. Explosion and Shock Waves, 2021, 41(8): 083105. DOI: 10.11883/bzycj-2021-0062. [28] 周磊, 姜亚成, 朱哲明, 等. 动载荷作用下裂隙岩体的止裂机理分析 [J]. 爆炸与冲击, 2021, 41(5): 053102. DOI: 10.11883/bzycj-2020-0125.ZHOU L, JIANG Y C, ZHU Z M, et al. Mechanism study of preventing crack propagation of fractured rock under dynamic loads [J]. Explosion and Shock Waves, 2021, 41(5): 053102. DOI: 10.11883/bzycj-2020-0125. [29] VERMA H K, SAMADHIYA N K, SINGH M, et al. Blast induced rock mass damage around tunnels [J]. Tunnelling and Underground Space Technology, 2018, 71: 149–158. DOI: 10.1016/j.tust.2017.08.019. [30] ZAREIFARD M R. A new semi-numerical method for elastoplastic analysis of a circular tunnel excavated in a Hoek-Brown strain-softening rock mass considering the blast-induced damaged zone [J]. Computers and Geotechnics, 2020, 122: 103476. DOI: 10.1016/j.compgeo.2020.103476. [31] JIANG N, ZHOU C B, LU S W, et al. Propagation and prediction of blasting vibration on slope in an open pit during underground mining [J]. Tunnelling and Underground Space Technology, 2017, 70: 409–421. DOI: 10.1016/j.tust.2017.09.005. [32] CAO F, ZHANG S, LING T H. Analysis of cumulative damage for shared rock in a neighborhood tunnel under cyclic blasting loading using the ultrasonic test [J]. Shock and Vibration, 2020(1): 8810089. DOI: 10.1155/2020/8810089. [33] TU W F, LI L P, ZHOU Z Q, et al. Thickness calculation of accumulative damaged zone by rock mass blasting based on Hoek-Brown failure criterion [J]. International Journal of Geomechanics, 2022, 22(2): 04021273. DOI: 10.1061/(ASCE)GM.1943-5622.0002257. [34] HE M C, FENG J L, SUN X M. Stability evaluation and optimal excavated design of rock slope at Antaibao open pit coal mine, China [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(3): 289–302. DOI: 10.1016/j.ijrmms.2007.05.007. [35] QI X, ZHANG Y P. Stability analysis of soil-rock mixed slope under earthquake environment [J]. Fresenius Environmental Bulletin, 2021, 30(4A): 4384–4390. [36] FAHIMIFAR A, KARAMI M, FAHIMIFAR A. Modifications to an elasto-visco-plastic constitutive model for prediction of creep deformation of rock samples [J]. Soils and Foundations, 2015, 55(6): 1364–1371. DOI: 10.1016/j.sandf.2015.10.003. [37] KABWE E, KARAKUS M, CHANDA E K. Creep constitutive model considering the overstress theory with an associative viscoplastic flow rule [J]. Computers and Geotechnics, 2020, 124: 103629. DOI: 10.1016/j.compgeo.2020.103629. [38] SUN B J, LIU Q W, LI W T, et al. Numerical implementation of rock bolts with yield and fracture behaviour under tensile-shear load [J]. Engineering Failure Analysis, 2022, 139: 106462. DOI: 10.1016/j.engfailanal.2022.106462. [39] 左双英, 肖明, 续建科, 等. 隧道爆破开挖围岩动态损伤效应数值模拟 [J]. 岩土力学, 2011, 32(10): 3171–3176, 3184. DOI: 10.16285/j.rsm.2011.10.040.ZUO S Y, XIAO M, XU J K, et al. Numerical simulation of dynamic damage effect of surrounding rocks for tunnels by blasting excavation [J]. Rock and Soil Mechanics, 2011, 32(10): 3171–3176, 3184. DOI: 10.16285/j.rsm.2011.10.040. [40] 夏祥, 李海波, 李俊如, 等. 岭澳核电站二期工程基岩爆破安全阈值分析 [J]. 岩土力学, 2008, 29(11): 2945–2951, 2956. DOI: 10.16285/j.rsm.2008.11.022.XIA X, LI H B, LI J R, et al. Research on vibration safety threshold for rock under blasting excavation [J]. Rock and Soil Mechanics, 2008, 29(11): 2945–2951, 2956. DOI: 10.16285/j.rsm.2008.11.022. [41] 费鸿禄, 苑俊华. 基于爆破累积损伤的边坡稳定性变化研究 [J]. 岩石力学与工程学报, 2016, 35(S2): 3868–3877. DOI: 10.13722/j.cnki.jrme.2015.1192.FEI H L, YUAN J H. Study of slope stability based on blasting cumulative damage [J]. Chinese Journal of Rock Mechanics and Engineering, 2016, 35(S2): 3868–3877. DOI: 10.13722/j.cnki.jrme.2015.1192. [42] DENG Z Y, LIU X R, LIU Y Q, et al. Model test and numerical simulation on the dynamic stability of the bedding rock slope under frequent microseisms [J]. Earthquake Engineering and Engineering Vibration, 2020, 19(4): 919–935. DOI: 10.1007/s11803-020-0604-8. [43] BAO M, CHEN Z H, ZHANG L F, et al. Experimental study on the sliding instability mechanism of slopes with weak layers under creeping action [J]. Measurement, 2023, 212: 112690. DOI: 10.1016/j.measurement.2023.112690. -







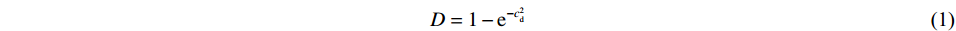
















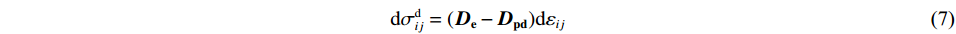






 下载:
下载:












 下载:
下载: