近地小天体对地撞击成坑模型研究进展

刘文近; 张庆明; 马晓荷; 龙仁荣; 任健康; 龚自正; 武强; 任思远

doi:10.11883/bzycj-2021-0255

近地小天体对地撞击成坑模型研究进展

doi: 10.11883/bzycj-2021-0255

1.
北京理工大学爆炸科学与技术国家重点实验室，北京 100081
2.
北京卫星环境工程研究所，北京 100094

基金项目: 民用航天预研项目（D020304）

详细信息

作者简介:
刘文近（1993－　），男，博士研究生，lwj931@163.com

通讯作者:
张庆明（1963－　），男，教授，博士生导师，qmzhang@bit.edu.cn

中图分类号: O383；O303
计量
- 文章访问数: 785
- HTML全文浏览量: 404
- PDF下载量: 185
- 被引次数: 0
出版历程
- 收稿日期: 2021-06-28
- 修回日期: 2021-08-24
- 网络出版日期: 2021-10-28
- 刊出日期: 2021-12-05

A review of the models of near-Earth object impact cratering on Earth

1.
State Key Laboratory of Explosion Science and Technology, Beijing Institute of Technology, Beijing 100081, China
2.
Beijing Institute of Spacecraft Environment Engineering, Beijing 100094, China

摘要

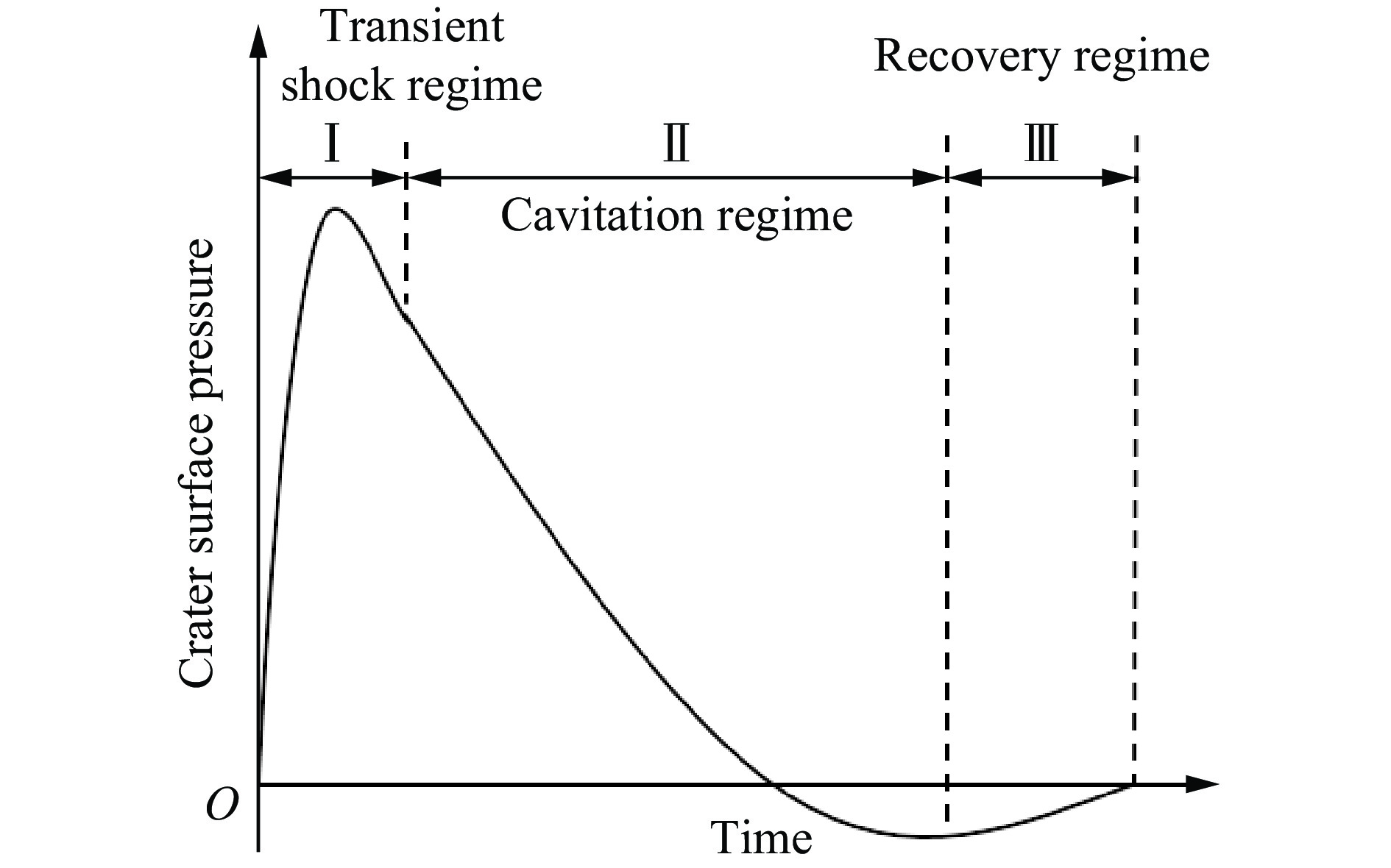
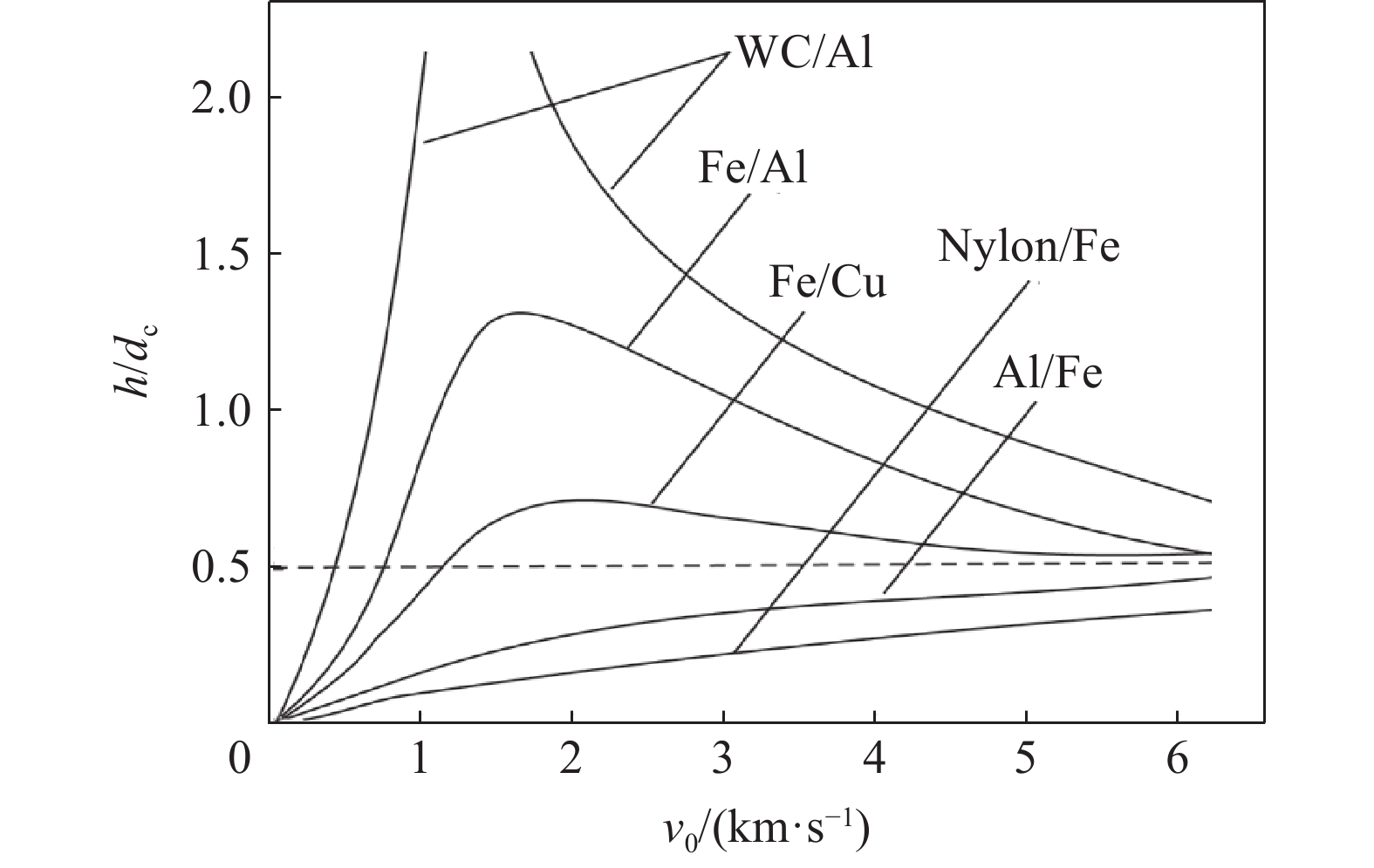
摘要: 近地小天体对地撞击成坑是行星研究的前沿问题之一。本文中介绍了陨石坑成坑过程与类型、实验室模拟成坑现象和陨石坑成坑模型律，分析了近地小天体对地撞击成坑机理和点源模型的不足，指出了近地小天体对地撞击成坑未来研究的发展趋势。
- 陨石坑 /
- 超高速碰撞 /
- 点源模型 /
- 相似律 /
- 耦合参数
Abstract: Near-Earth object (NEO) impact cratering is one of the frontier themes in planetary research. The cratering process and types, laboratory impact cratering phenomena, and cratering scaling are introduced. The hypervelocity impact-cratering process is conventionally divided into three successive stages: contact and compression, excavation, and modification. When large impact craters are formed in geological materials, shearing is the main deformation mode. At small scales, cratering in brittle materials is dominated by surface spalling; much of the crater volume consists of a wide, flat spall zone. According to the morphological characteristics of impact craters, impact craters are generally divided into two groups: simple and complex craters. The cratering mechanism of NEO impact cratering and the deficiency of the point -source model are analyzed. The cratering mechanism can be divided into strength regime and gravity regime. In the strength regime, the cratering results are controlled by strength, and in the gravity regime, the cratering results are dominated by gravity. Crater scaling laws have been established based on dimensional analysis, point-source approximation and the results of experimental and numerical impact. The scaling law is a specific power rate form, which describes well the scaling of crater size, ejecta, and crater growth. But the scaling law of the point-source model is not applicable to the experimental phenomena in several impactor radii. The suggestions for future research of NEO impact cratering are pointed out: (1) scaling where the point-source hypothesis is not applicable; (2) the effect of melting, gasification, atmosphere and temperature on the cratering process; (3) the scaling law and model of oblique impact; (4) momentum enhancement effect of impact; (5) experimental and numerical methods to simulate the formation of impact craters.
- impact crater /
- hypervelocity impact /
- point source model /
- scaling /
- coupling parameter

HTML全文

对任何一个在轨运行的航天器而言，由于其太阳能电池阵长时间暴露于微流星和空间碎片撞击环境中，受到微流星和空间碎片撞击可能非常大。这种碰撞速度介于1~20 km/s的超高速撞击，往往会造成太阳能电池阵穿孔破坏，进而造成输出功率降低，影响航天器正常工作，为此科学家们进行了大量研究^[1-5]。在超高速碰撞过程的早期，喷出物的部分物质会发生电离，产生等离子体^[6-9^]。因此，开展超高速碰撞对太阳电池阵造成的物理损伤及碰撞中产生等离子体对太阳电池输出的研究，有助于更加深入了解超高速撞击对航天器太阳电池阵的影响。本文中利用自行构建的实验电路及诊断方法，对上述问题开展实验研究。

1. 实验设计

1.1 实验系统组成

本实验系统共由3部分组成：碎片加载系统、等离子体诊断系统和太阳阵输出监测系统。实验采用二级轻气炮作为发射装置，自行设计了外电路及等离子体诊断装置，如图 1所示。撞击靶板采用图2所示的太阳电池单元。该单元共由4片硅太阳电池通过两两串联、串间断开的方式组成，以模拟高压太阳电池阵在空间环境下相邻电池串间的高压差状态，串间距取标准太阳电池片间距0.6 mm。

图 1 实验系统示意图

Figure 1. Configuration of experimental system

下载: 全尺寸图片幻灯片

图 2 太阳电池阵单元

Figure 2. Solar array unit

下载: 全尺寸图片幻灯片

电池阵放置于靶室内部可任意调节角度的靶架上，通过对两串电池外加电压来模拟太阳电池阵的输出状态。采用如图 3所示的测试电路，对碰撞瞬间太阳电池输出状态变化进行监测。其中，电流探针CP₁用于对整个回路电流进行测量，电流探针CP₂用于相邻串间电池电流测量，电流探针CP₃用于电池片与铝蜂窝基板间电流测量，电压探针V用于对整个回路中的电压进行测量。在碰撞瞬间，碰撞点附近产生高浓度的等离子体，可能导致相邻电池串间、电池片与基板间形成瞬间短路，在高电压的作用下会对电池产生严重损伤。为模拟高压太阳阵状态，本实验选择外加电压100 V，弹丸入射方向与靶板放置法线方向夹角为60°，弹丸为4.7 mm的铝制球形弹丸。实验共进行4组，测得碰撞速度分别为2.80、4.11、4.36、5.37 km/s。

图 3 放电检测电路

Figure 3. Arc detecting circuit

下载: 全尺寸图片幻灯片

1.2 瞬态等离子体诊断

采用Langmuir三探针对碰撞过程产生的等离子体进行诊断，可获得高速碰撞产生的瞬态等离子体的电子密度和电子温度^[10]，其结构如图 4所示，三探针中各个探头位置成等边三角形分布。其计算公式如下：

图 4 等离子体诊断原理图

Figure 4. Mechanism of plasma diagnosis

下载: 全尺寸图片幻灯片

电子温度：

$\frac{{{I_1} + {I_2}}}{{{I_1} + {I_3}}} = \frac{{1 - \exp \left( { - {\phi _{{\text{d}}2}}} \right)}}{{1 - \exp \left( { - {\phi _{{\text{d}}3}}} \right)}}$

(1)

$I_{1}=I_{2}+I_{3}$

(2)

式中： $\phi_{\mathit{\boldsymbol{d}} 2}=\frac{e V_{\mathit{\boldsymbol{d}} 2}}{k T_{\mathit{\boldsymbol{e}}}}$ , $\phi_{\mathit{\boldsymbol{d}} 3}=\frac{e V_{\mathit{\boldsymbol{d}} 3}}{k T_{\mathit{\boldsymbol{e}}}}$ , 其中e为电荷，k为波尔兹曼常数。

电子密度：

$I_{i}=\frac{I_{3}-I_{2} \exp \left(-\phi_{\Delta V}\right)}{1-\exp \left(-\phi_{\Delta V}\right)}$

(3)

$N_{\mathit{\boldsymbol{e}}}=\left[\frac{M^{\frac{1}{2}}}{S}\right] I_{i} f_{1}\left(V_{\mathit{\boldsymbol{d}} 2}\right)$

(4)

$f_{1}\left(V_{d 2}\right)=1.05 \times 10^{15}\left(T_{e}\right)^{-\frac{1}{2}}\left[\exp \left(\phi_{d 2}\right)-1\right]^{-1}$

(5)

式中：M是离子(由于基体及弹丸材料为铝，因此本实验的离子即为铝离子)质量，g; S为探针的表面积，mm²; I_i为离子电流，μA；V_d2=3 V, V_d3=18 V，R=10 kΩ。图 5为本次实验过程中，等离子体温度和密度随碰撞速度的变化规律。可以看出，随着速度的增大，等离子体的密度和温度均随着碰撞速度有显著地增加。

图 5 等离子体参数随碰撞速度变化示意图

Figure 5. Diagrams of plasma parameters vs. colliding velocity

下载: 全尺寸图片幻灯片

2. 结果与分析

图 6为碰撞后的太阳电池阵单元示意图。从图中可以清楚看出，碰撞点位于四片太阳电池交汇中心，碰撞过程的强大冲击导致了太阳电池片出现不可修复的物理损伤，尤其是弹道入射方向同电池阵单元间夹角为锐角的两个电池片，几乎完全损坏。

图 6 撞击完成后的太阳阵单元实物图

Figure 6. Solar array unit after impact

下载: 全尺寸图片幻灯片

以碰撞速度为4.36 km/s的实验结果对本次实验进行分析。图 7为等离子体密度随时间的演化规律。随着等离子体在碰撞点附近的产生、膨胀、冷却和复合，探针测得的等离子体密度符合实际情况。高浓度的等离子体成为电池阵单元片间以及片与基板间产生电弧放电现象的诱因，测得的等离子体密度高达1.62×10¹⁶ m^-3，远高于近地轨道空间等离子体的密度。虽然持续时间在微秒级，但伴随着高电压，其能量足以引起电弧放电，并导致了电池阵单元基板上的铝蜂窝产生燃烧，造成基板结构的严重损伤。

图 7 等离子体密度随时间的变化

Figure 7. Variation of plasma density with different times

下载: 全尺寸图片幻灯片

图 8所示为放电监测电路电流、电压探针测得的信号。从图中可以看出，撞击导致了电池阵单元电池片间、电池片与基板间瞬间导通，由于基板铝蜂窝的导电性优于电池片与电池片间，其产生的放电要更强，因而造成的损伤亦更大。

图 8 放电监测电路电流、电压探针波形

Figure 8. Current and voltage waveforms of arc detecting circuit

下载: 全尺寸图片幻灯片

3. 结论

空间碎片撞击太阳电池阵会产生高浓度的等离子体，进而诱发产生瞬间短路现象，对太阳电池阵单元产生机械损伤的同时，亦会产生严重的电损伤，导致基板铝蜂窝产生严重烧毁的现象；且随着碰撞速度的增大，产生的损伤效应也更加严重。

感谢沈阳理工大学唐恩凌教授在实验过程中给予的指导和帮助。

图 1 地球撞击坑数据库世界地图

Figure 1. Earth impact cratering database world map

下载: 全尺寸图片幻灯片

图 2 简单坑和复杂坑形成过程的示意图^{[12, 19]}

Figure 2. Series of formation of simple and complex craters^{[12, 19]}

下载: 全尺寸图片幻灯片

图 3 简单的陨石坑^[12]

Figure 3. Simple impact crater^[12]

下载: 全尺寸图片幻灯片

图 4 复杂的陨石坑^[19]

Figure 4. Complex impact craters^[19]

下载: 全尺寸图片幻灯片

图 5 波音公司600g土工离心机^[35]

Figure 5. The Boeing 600g geotechnical centrifuge^[35]

下载: 全尺寸图片幻灯片

图 6 成坑表面压力与时间曲线^[42]

Figure 6. The crater surface pressure versus time ^[42]

下载: 全尺寸图片幻灯片

图 7 钢弹丸以4.8 km/s撞击干砂岩成坑过程中抛射物的演化^[45]

Figure 7. Typical evolution of ejecta at different times after a steel projectile impacting dry sandstone at 4.8 km /s ^[45]

下载: 全尺寸图片幻灯片

图 8 成坑形状与撞击速度的关系^[46]

Figure 8. The relationship between crater shape and impact velocity^[46]

下载: 全尺寸图片幻灯片

图 9 典型的半球形金属坑和剖面图^[47]

Figure 9. Typical hemispherical metal crater and profile^[47]

下载: 全尺寸图片幻灯片

图 10 岩石类陨石坑^{[48, 50]}

Figure 10. Rocky impact crater^{[48, 50]}

下载: 全尺寸图片幻灯片

图 11 不同实验拟合的经验公式曲线

Figure 11. Empirical formula curves fitted by different experiments

下载: 全尺寸图片幻灯片

图 12 成坑效率 ${\varPi }_{V}$ 与 ${\varPi }_{2}$ 大小的关系示意图^[53]

Figure 12. Schematic illustration of cratering efficiency ${\varPi }_{V}$ depends on ${\varPi }_{2}$ ^[53]

下载: 全尺寸图片幻灯片

表 1 公式(11)中参数

Table 1. The parameters value of equation (11)

m	n	v^*	注释	来源
2/3	2/3	$v/{c}_{{\rm{t}}}$		文献 [62-63]
1/2	2/3	$v/{c}_{{\rm{t}}}$		文献 [64]
1/3	0.58	$v/{c}_{{\rm{t}}}$		文献 [65]
2/3	2/3	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{Y}_{\mathrm{t}}}$		文献 [47, 66-67]
1/3	2/3	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{Y}_{\mathrm{t}}}$		文献 [68]
0.725	2/3	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{Y}_{\mathrm{t}}}$		文献 [69]
0.523	0.3545	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{Y}_{\mathrm{t}}}$		文献 [55]
0.448	0.563	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{Y}_{\mathrm{t}}}$		文献 [44]
2/3	2/3	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{H}_{{\rm{B}}}}$	${H}_{{\rm{B}}}$ 是布氏硬度	文献 [70]
0.62	0.48	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{H}_{{\rm{B}}}}$	2.6 km/s $\text{＜}v \text{≤}$ 5 km/s	文献 [71]
0.5	0.68	$\sqrt{{\rho }_{\mathrm{t}}{v}^{2}/{H}_{{\rm{B}}}}$	$v \text{＞}$ 5 km/s	文献 [71]

下载: 导出CSV

表 2 强度和重力机理控制下成坑变量相似律

Table 2. Summary of cratering variables scaling in strength and gravity regimes

成坑结果	一般形式相似	点源，强度区间(假设 $Y\gg \rho ga$ )	点源，重力区间(假设 $\rho ga\gg Y$ )
体积 $V$	$\dfrac{\rho V}{m}=f\left(\dfrac{ga}{{U}^{2}},\dfrac{Y}{\rho {U}^{2}}\right)$	$V{\propto \dfrac{m}{\rho }\left(\dfrac{Y}{\rho {U}^{2} }\right)}^{-\frac{3\mu }{2} }{\left(\dfrac{\rho }{\delta }\right)}^{1-3\nu +\frac{3\mu }{2} }$	$V\propto \dfrac{m}{\rho }{\left(\dfrac{ga}{ {U}^{2} }\right)}^{ \frac{-3\mu }{2+\mu } }{\left(\dfrac{\rho }{\delta }\right)}^{\frac{2+\mu -6\nu }{2+\mu } }$
半径R	$R{\left(\dfrac{\rho }{m}\right)}^{{1}/{3} }=f\left(\dfrac{ga}{ {U}^{2} },\dfrac{Y}{\rho {U}^{2} }\right)$	$R{ {\left(\dfrac{\rho }{m}\right)}^{ \frac{1}{3} }\propto \left(\dfrac{Y}{\rho {U}^{2} }\right)}^{-\frac{\mu }{2} }{\left(\dfrac{\rho }{\delta }\right)}^{ \frac{1}{3}-\nu +\frac{\mu }{2} }$	$R{\left(\dfrac{\rho }{m}\right)}^{\frac {1}{3} }\propto {\left(\dfrac{ga}{ {U}^{2} }\right)}^{\frac {-\mu }{2+\mu } }{\left(\dfrac{\rho }{\delta }\right)}^{\frac{2+\mu -6\nu }{3\left(2+\mu \right)} }$
深度 $h$	$h{\left(\dfrac{\rho }{m}\right)}^{{1}/{3} }=f\left(\dfrac{ga}{ {U}^{2} },\dfrac{Y}{\rho {U}^{2} }\right)$	$h{ {\left(\dfrac{\rho }{m}\right)}^{ \frac{1}{3} }\propto \left(\dfrac{Y}{\rho {U}^{2} }\right)}^{-\frac{\mu }{2} }{\left(\dfrac{\rho }{\delta }\right)}^{ \frac{1}{3}-\nu +\frac{\mu }{2} }$	$h{\left(\dfrac{\rho }{m}\right)}^{ \frac{1}{3} }\propto {\left(\dfrac{ga}{ {U}^{2} }\right)}^{ \frac{-\mu }{2+\mu } }{\left(\dfrac{\rho }{\delta }\right)}^{\frac{2+\mu -6\nu }{3\left(2+\mu \right)} }$

下载: 导出CSV

表 3 各种地质材料地质材料耦合参数指数和成坑体积相似律^[53]

Table 3. Coupling parameter exponent of various geological materials and scaling law of crater volume ^[53]

材料	相似指数 $\alpha$	相似指数 $\ \mu$	${K}_{1}$	$\overline{Y}/{\rm{MPa}}$	强度区间¹⁾	重力区间¹⁾	强度向重力机理转换的冲击器直径/m²⁾
砂	0.51	0.41	0.24	0	−	$V=0.14{m}^{0.83}{g}^{-0.51}{U}^{1.02}$	接近 0
干土	0.51	0.41	0.24	0.18	$V=0.04m{U}^{1.23}$	$V=0.14{m}^{0.83}{g}^{-0.51}{U}^{1.02}$	0.2
湿土	0.65	0.55	0.20	0.14	$V=0.05m{U}^{1.65}$	$V=0.60{m}^{0.783}{g}^{-0.65}{U}^{1.3}$	1.2
水	0.648	0.55	2.30	0	−	$V=13.0{m}^{0.783}{g}^{-0.65}{U}^{1.3}$	接近 0
软岩	0.65	0.55	0.20	7.6	$V=0.009m{U}^{1.65}$	$V=0.48{m}^{0.783}{g}^{-0.65}{U}^{1.3}$	11
硬岩	0.60	0.55	0.20	18	$V=0.005m{U}^{1.65}$	$V=0.48{m}^{0.783}{g}^{-0.65}{U}^{1.3}$	32
1) 弹丸的质量 $m$ 的单位是kg，速度 $U$ 的单位是km/s，成坑体积 $V$ 的单位是m³; 2) 地球加速度下10 km/s冲击。

下载: 导出CSV

参考文献(78)

[1]	MARUYAMA S, EBISUZAKI T. Origin of the Earth: A proposal of new model called ABEL [J]. Geoscience Frontiers, 2017, 8(2): 253–274. DOI: 10.1016/j.gsf.2016.10.005.
[2]	PELTON J N, ALLAHDADI F. Handbook of cosmic hazards and planetary defense [M]. London: Springer, 2015: 5−13.
[3]	ALVAREZ W. Comparing the evidence relevant to impact and flood basalt at times of major mass extinctions [J]. Astrobiology, 2003, 3(1): 153–161. DOI: 10.1089/153110703321632480.
[4]	COLLINS G S, MELOSH H J, MARCUS R A. Earth impact effects program: a web-based computer program for calculating the regional environmental consequences of a meteoroid impact on Earth [J]. Meteoritics & Planetary Science, 2005, 40(6): 817–840. DOI: 10.1111/j.1945-5100.2005.tb00157.x.
[5]	SCHULTE P, ALEGRET L, ARENILLAS I, et al. The Chicxulub asteroid impact and mass extinction at the Cretaceous-Paleogene boundary [J]. Science, 2010, 327(5970): 1214–1218. DOI: 10.1126/science.1177265.
[6]	HESTROFFER D, SÁNCHEZ P, STARON L, et al. Small solar system bodies as granular media [J]. The Astronomy and Astrophysics Review, 2019, 27(1): 6. DOI: 10.1007/s00159-019-0117-5.
[7]	柳森, 党雷宁, 赵君尧, 等. 小行星撞击地球的超高速问题 [J]. 力学学报, 2018, 50(6): 1311–1327. DOI: 10.6052/0459-1879-18-313. LIU S, DANG L N, ZHAO J Y, et al. Hypervelocity issues of earth impact by asteroids [J]. Chinese Journal of Theoretical and Applied Mechanics, 2018, 50(6): 1311–1327. DOI: 10.6052/0459-1879-18-313.
[8]	SHOEMAKER E M, WEISSMAN P R, SHOEMAKER C S. The flux of periodic comets near Earth [M]. Arizona: University of Arizona Press, 1994: 313−335.
[9]	BLAND P A, ARTEMIEVA N A. Efficient disruption of small asteroids by Earth’s atmosphere [J]. Nature, 2003, 424(6946): 288–291. DOI: 10.1038/nature01757.
[10]	WANG X Y, LUO L, GUO H D, et al. Cratering process and morphological features of the Xiuyan impact crater in Northeast China [J]. Science China Earth Sciences, 2013, 56(10): 1629–1638. DOI: 10.1007/s11430-013-4695-1.
[11]	陈鸣, 谢先德, 肖万生, 等. 依兰陨石坑: 我国东北部一个新发现的撞击构造 [J]. 科学通报, 2020, 65(10): 948–954. DOI: 10.1360/TB-2019-0704. CHEN M, XIE X D, XIAO W S, et al. Yilan crater, a newly identified impact structure in northeast China [J]. China Science Bulletin, 2020, 65(10): 948–954. DOI: 10.1360/TB-2019-0704.
[12]	OSINSKI G R, PIERAZZO E. Impact cratering: processes and products [M]. Chichester: John Wiley & Sons, 2013.
[13]	GRIEVE R A F, THERRIAULT A M. Observations at terrestrial impact structures: Their utility in constraining crater formation [J]. Meteoritics & Planetary Science, 2004, 39(2): 199–216. DOI: 10.1111/j.1945-5100.2004.tb00336.x.
[14]	DYPVIK H, PLADO J, HEINBERG C, et al. Impact structures and events: a Nordic perspective [J]. Episodes, 2008, 31(1): 107–114. DOI: 10.18814/epiiugs/2008/v31i1/015.
[15]	PLADO J. Meteorite impact craters and possibly impact-related structures in Estonia [J]. Meteoritics & Planetary Science, 2012, 47(10): 1590–1605. DOI: 10.1111/j.1945-5100.2012.01422.x.
[16]	GAULT D E, QUAIDE W L, OBERBECK V R. Impact cratering mechanics and structures [M]//FRENCH B M, SHORT N M. Shock Metamorphism of Natural Materials. Mono Book Corporation, 1968: 23−24.
[17]	MELOSH H J. Impact cratering: a geologic process [M]. New York: Oxford University Press, 1989.
[18]	COLLINS G S, MELOSH H J, OSINSKI G R. The impact-cratering process [J]. Elements, 2012, 8(1): 25–30. DOI: 10.2113/gselements.8.1.25.
[19]	OSINSKI G R, TORNABENE L L, GRIEVE R A F. Impact ejecta emplacement on terrestrial planets [J]. Earth and Planetary Science Letters, 2011, 310(3−4): 167–181. DOI: 10.1016/j.jpgl.2011.08.012.
[20]	KIEFFER S W, SIMONDS C H. The role of volatiles and lithology in the impact cratering process [J]. Reviews of Geophysics, 1980, 18(1): 143–181. DOI: 10.1029/RG018i001p00143.
[21]	O’KEEFE J D, AHRENS T J. Cometary and meteorite swarm impact on planetary surfaces [J]. Journal of Geophysical Research: Solid Earth, 1982, 87(B8): 6668–6680. DOI: 10.1029/JB087iB08p06668.
[22]	PIERAZZO E, MELOSH H J. Melt production in oblique impacts [J]. Icarus, 2000, 145(1): 252–261. DOI: 10.1006/icar.1999.6332.
[23]	AHRENS T J, O’KEEFE J D. Shock melting and vaporization of Lunar rocks and minerals [J]. The Moon, 1972, 4(41): 214–249. DOI: 10.1007/bf00562927.
[24]	DENCE M R. The extraterrestrial origin of Canadian craters [J]. Annals of the New York Academy of Sciences, 1965, 123(2): 941–969. DOI: 10.1111/j.1749-6632.1965.tb20411.x.
[25]	TURTLE E P, PIERAZZO E, COLLINS G S, et al. Impact structures: what does crater diameter mean? [M]. Portland: The Geological Society of America, 2005: 1−24. DOI: 10.1130/0-8137-2384-1.1.
[26]	MELOSH H J, IVANOV B A. Impact crater collapse [J]. Annual Review of Earth and Planetary Sciences, 1999, 27(1): 385–415. DOI: 10.1146/annurev.earth.27.1.385.
[27]	PIKE R J. Control of crater morphology by gravity and target type: Mars, Earth, Moon. [C]// 11th Lunar and Planetary Science Conference. New York, USA: Pergamon Press, 1980.
[28]	PIKE R J. Size-dependence in the shape of fresh impact craters on the moon [M]. New York, USA: Pergamon Press, 1977: 489−509.
[29]	PILKINGTON M, GRIEVE R A F. The geophysical signature of terrestrial impact craters [J]. Reviews of Geophysics, 1992, 30(2): 161–181. DOI: 10.1029/92RG00192.
[30]	OSINSKI G R, BUNCH T E, FLEMMING R L, et al. Impact melt- and projectile-bearing ejecta at Barringer Crater, Arizona [J]. Earth and Planetary Science Letters, 2015, 432: 283–292. DOI: 10.1016/j.jpgl.2015.10.021.
[31]	GRIEVE R A F, GARVIN J B. A geometric model for excavation and modification at terrestrial simple impact craters [J]. Journal of Geophysical Research: Solid Earth, 1984, 89(B13): 11561–11572. DOI: 10.1029/JB089iB13p11561.
[32]	TREDOUX M, HART R J, CARLSON R W, et al. Ultramafic rocks at the center of the Vredefort structure: further evidence for the crust on edge model [J]. Geology, 1999, 27(10): 923–926.
[33]	OSINSKI G R, LEE P, SPRAY J G, et al. Geological overview and cratering model for the Haughton impact structure, Devon Island, Canadian High Arctic [J]. Meteoritics & Planetary Science, 2005, 40(12): 1759–1776. DOI: 10.1111/j.1945-5100.2005.tb00145.x.
[34]	CLARKE J, KNIGHTLY P, RUPERT S. Melt-water formed dark streaks on slopes of Haughton crater as possible Mars analogues [J]. International Journal of Astrobiology, 2019, 18: 518–526. DOI: 10.1017/S1473550418000526.
[35]	HOUSEN K R, SWEET W J, HOLSAPPLE K A. Impacts into porous asteroids [J]. Icarus, 2018, 300: 72–96. DOI: 10.1016/j.icarus.2017.08.019.
[36]	STÖFFLER D, GAULT D E, WEDEKIND J, et al. Experimental hypervelocity impact into quartz sand: distribution and shock metamorphism of ejecta [J]. Journal of Geophysical Research, 1975, 80(29): 4062–4077. DOI: 10.1029/jb080i029p04062.
[37]	GAULT D E, WEDEKIND J A. Experimental impact “craters” formed in water: gravity scaling realized [J]. Eos, Transactions-American Geophysical Union, 1978, 59(12): 1121.
[38]	KENKMANN T, DEUTSCH A, THOMA K, et al. The MEMIN research unit: Experimental impact cratering [J]. Meteoritics & Planetary Science, 2013, 48(1): 1–2. DOI: 10.1111/maps.12035.
[39]	EBERT M, HECHT L, DEUTSCH A, et al. Geochemical processes between steel projectiles and silica-rich targets in hypervelocity impact experiments [J]. Geochimica et Cosmochimica Acta, 2014, 133: 257–279. DOI: 10.1016/j.gca.2014.02.034.
[40]	HARRISS K H, BURCHELL M J. Hypervelocity impacts into ice-topped layered targets: Investigating the effects of ice crust thickness and subsurface density on crater morphology [J]. Meteoritics & Planetary Science, 2017, 52(7): 1505–1522. DOI: 10.1111/maps.12913.
[41]	HOLSAPPLE K A, SCHMIDT R M. On the scaling of crater dimensions: 1. explosive processes [J]. Journal of Geophysical Research: Solid Earth, 1980, 85(B12): 7247–7256. DOI: 10.1029/JB085iB12p07247.
[42]	SUN Y H, SHI C C, LIU Z, et al. Theoretical research progress in high-velocity/hypervelocity impact on semi-infinite targets [J]. Shock and Vibration, 2015, 2015: 265321. DOI: 10.1155/2015/265321.
[43]	李卧东, 王明洋, 施存程, 等. 地质类材料超高速撞击相似关系与实验研究综述 [J]. 防护工程, 2015, 37(2): 55–62. LI W D, WANG M Y, SHI C C, et al. Review of similarity laws and scaling experiments research of hypervelocity impact on geological material targets [J]. Protective Engineering, 2015, 37(2): 55–62.
[44]	张庆明, 黄风雷. 超高速碰撞动力学引论 [M]. 北京: 科学出版社, 2000. ZHANG Q M, HUANG F L. An introduction to the dynamics of hypervelocity collisions [M]. Beijing: Science Press, 2000.
[45]	HOERTH T, SCHAEFER F, THOMA K, et al. Hypervelocity impacts on dry and wet sandstone: observations of ejecta dynamics and crater growth [J]. Meteoritics & Planetary Science, 2013, 48(1): 23–32. DOI: 10.1111/maps.12044.
[46]	经福谦. 超高速碰撞现象 [J]. 爆炸与冲击, 1990, 10(3): 279–288. JING F Q. Hypervelocity impact phenomena [J]. Explosion and Shock Waves, 1990, 10(3): 279–288.
[47]	王马法, 周智炫, 黄洁, 等. 镁合金弹丸10 km/s 撞击铝靶成坑特性实验 [J]. 爆炸与冲击, 2021, 41(5): 053302. DOI: 10.11883/bzycj-2020-0129. WANG M F, ZHOU Z X, HUANG J, et al. Experiment on crater characteristics of aluminium targets impacted by magnesium projectiles at velocities of about 10 km/s [J]. Explosion and Shock Waves, 2021, 41(5): 053302. DOI: 10.11883/bzycj-2020-0129.
[48]	POELCHAU M H, KENKMANN T, HOERTH T, et al. Impact cratering experiments into quartzite, sandstone and tuff: The effects of projectile size and target properties on spallation [J]. Icarus, 2014, 242: 211–224. DOI: 10.1016/j.icarus.2014.08.018.
[49]	POELCHAU M H, KENKMANN T, THOMA K, et al. The MEMIN research unit: Scaling impact cratering experiments in porous sandstones [J]. Meteoritics & Planetary Science, 2013, 48(1): 8–22. DOI: 10.1111/maps.12016.
[50]	DUFRESNE A, POELCHAU M H, KENKMANN T, et al. Crater morphology in sandstone targets: The MEMIN impact parameter study [J]. Meteoritics & Planetary Science, 2013, 48(1): 50–70. DOI: 10.1111/maps.12024.
[51]	BUHL E, POELCHAU M H, DRESEN G, et al. Deformation of dry and wet sandstone targets during hypervelocity impact experiments, as revealed from the MEMIN Program [J]. Meteoritics & Planetary Science, 2013, 48(1): 71–86. DOI: 10.1111/j.1945-5100.2012.01431.x.
[52]	HOLSAPPLE K A, HOUSEN K R. Momentum transfer in asteroid impacts. Ⅰ. theory and scaling [J]. Icarus, 2012, 221(2): 875–887. DOI: 10.1016/j.icarus.2012.09.022.
[53]	HOLSAPPLE K A. The scaling of impact processes in planetary sciences [J]. Annual Review of Earth and Planetary Sciences, 1993, 21(1): 333–373. DOI: 10.1146/annurev.ea.21.050193.002001.
[54]	HOLSAPPLE K A, SCHMIDT R M. Point-Source solutions and coupling parameters in cratering mechanics [J]. Journal of Geophysical Research:Solid Earth, 1987, 92(B7): 6350–6376. DOI: 10.1029/JB092iB07p06350.
[55]	HOLSAPPLE K A, SCHMIDT R M. On the scaling of crater dimensions: 2. impact processes. [J]. Journal of Geophysical Research:Solid Earth, 1982, 87(B3): 1849–1870. DOI: 10.1029/jb087ib03p01849.
[56]	HOUSEN K R, SCHMIDT R M, HOLSAPPLE K A. Crater ejecta scaling laws: Fundamental forms based on dimensional analysis [J]. Journal of Geophysical Research:Solid Earth, 1983, 88(B3): 2485–2499. DOI: 10.1029/JB088iB03p02485.
[57]	KENKMANN T, DEUTSCH A, THOMA K, et al. Experimental impact cratering: A summary of the major results of the MEMIN research unit [J]. Meteoritics & Planetary Science, 2018, 53(8): 1543–1568. DOI: 10.1111/maps.13048.
[58]	HERRMANN W, WILBECK J S. Review of hypervelocity penetration theories [J]. International Journal of Impact Engineering, 1987, 5(1−4): 307–322. DOI: 10.1016/0734-743x(87)90048-0.
[59]	SEDGWICK R T. Numerical techniques for modeling high velocity penetration and perforation processes [J]. 1980, 5: 253−272. DOI: 10.1016/B978-0-444-41928-6.50016-8.
[60]	向家琳. 金属材料可压缩性效应对超高速碰撞中厚靶成坑的影响 [D]. 北京: 中国科学院力学研究所, 1990. XIANG J L. Effect of compressibility of metal materials on craters of thick targets in hypervelocity collision [D]. Beijing: Institute of mechanics, Chinese Academy of Science, 1990.
[61]	罗忠文. 金属材料超高速碰撞的数值模拟 [D]. 北京: 中国科学院力学研究所, 1990. LUO Z W. Numerical simulation of hypervelocity impact of metallic materials [D]. Beijing: Institute of Mechanics, Chinese Academy of Science, 1990.
[62]	CHRISTIANSEN E L. Design and performance equations for advanced meteoroid and debris shields [J]. International Journal of Impact Engineering, 1993, 14(1−4): 145–156. DOI: 10.1016/0734-743x(93)90016-z.
[63]	SUMMERS J L, CHARTERS A C. High speed impact of metal projectiles in targets of various materials [C]// Proceedings of the 3rd Symposium on Hypervelocity Impact. Chicago, USA, 1958.
[64]	BRUCE E P. Review and analysis of high velocity impact data [C]// Proceedings of the 5th Symposium on Hypervelocity Impact. Denver, Colorado, USA: Defense Technical Information Center, 1961.
[65]	WALSH J. On the theory of hypervelocity impact [C]// 7th Symposium on Hypervelocity Impact. Florida, USA, 1964.
[66]	CHRISTMAN D R, GEHRING J W. Analysis of high-velocity projectile penetration mechanics [J]. Journal of Applied Physics, 1966, 37(4): 1579–1587. DOI: 10.1063/1.1708570.
[67]	CHARTERS A C, SUMMERS J L. Some comments on the phenomena of high speed impact [C]// Proceedings of the Dicennial Symposium. White Oak, Maryland, USA: US Naval Ordnance Laboratory, 1959.
[68]	EICHELBERGER R J, Gehring J. W. Effects of Meteoroid Impacts on space vehicles [J]. ARS Journal, 1962, 32(10): 1583–1591. DOI: 10.2514/8.6339.
[69]	YU S B, SUN G C, TAN Q M. Experimental laws of cratering for hypervelocity impacts of spherical projectiles into thick target [J]. International Journal of Impact Engineering, 1994, 15(1): 67–77. DOI: 10.1016/s0734-743x(05)80007-7.
[70]	HERRMANN W, JONES A. Correlation of hypervelocity impact data [C]// The Fifth Symposium on Hypervelocity Impact. Denver, Colorado, USA, 1961.
[71]	周劲松, 甄良, 杨德庄. 几种金属材料在2.6~7 km/s 弹丸撞击下的损伤行为 [J]. 宇航学报, 2000(2): 75–81. DOI: 10.3321/j.issn:1000-1328.2000.02.012. ZHOU J S, ZHEN L, YANG D Z. Damage behaviors of several metal materials under impacts of projectiles with hypervelocities of 2.6−7 km /s [J]. Journal of Astronautics, 2000(2): 75–81. DOI: 10.3321/j.issn:1000-1328.2000.02.012.
[72]	HOLSAPPLE K A, SCHMIDT R M. A material-strength model for apparent crater volume [C]// Proceedings of the 10th Lunar and Planetary Science Conference. 1979.
[73]	HOLSAPPLE K A. Material strength and explosive property effects in cratering and ground shock [C]// The Sixth International Symposium of Blast Simulation. Cahors, France: Centre D’ Etudesde Gramat, 1979.
[74]	HOLSAPPLE K A. The scaling of impact phenomena [J]. International Journal of Impact Engineering, 1987, 5(1−4): 343–355. DOI: 10.1016/0734-743X(87)90051-0.
[75]	SCHMIDT R M, HOLSAPPLE K A. Theory and experiments on centrifuge cratering [J]. Journal of Geophysical Research: Solid Earth, 1980, 85(B1): 235–252. DOI: 10.1029/JB085iB01p00235.
[76]	ÖPIK E J. Meteor impact on solid surface [J]. Irish Astronomical Journal, 1958, 5(1): 14–33.
[77]	SCHMIDT R M, HOUSEN K R. Some recent advances in the scaling of impact and explosion cratering [J]. International Journal of Impact Engineering, 1987, 5(1−4): 543–560. DOI: 10.1016/0734-743X(87)90069-8.
[78]	ASPHAUG E, MOORE J M, MORRISON D, et al. Mechanical and geological effects of impact cratering on Ida [J]. Icarus, 1996, 120(1): 158–184. DOI: 10.1006/icar.1996.0043.