Dynamic characteristics and damage mechanism of freeze-thaw treated red sandstone under cyclic impact
-
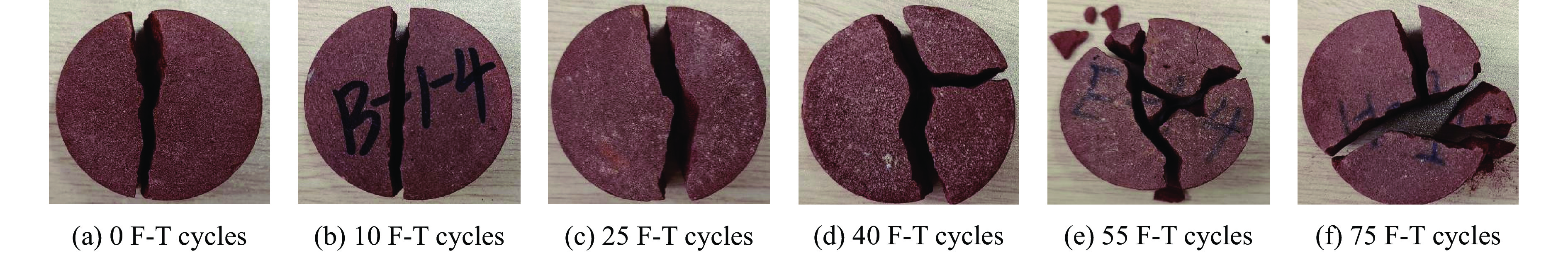
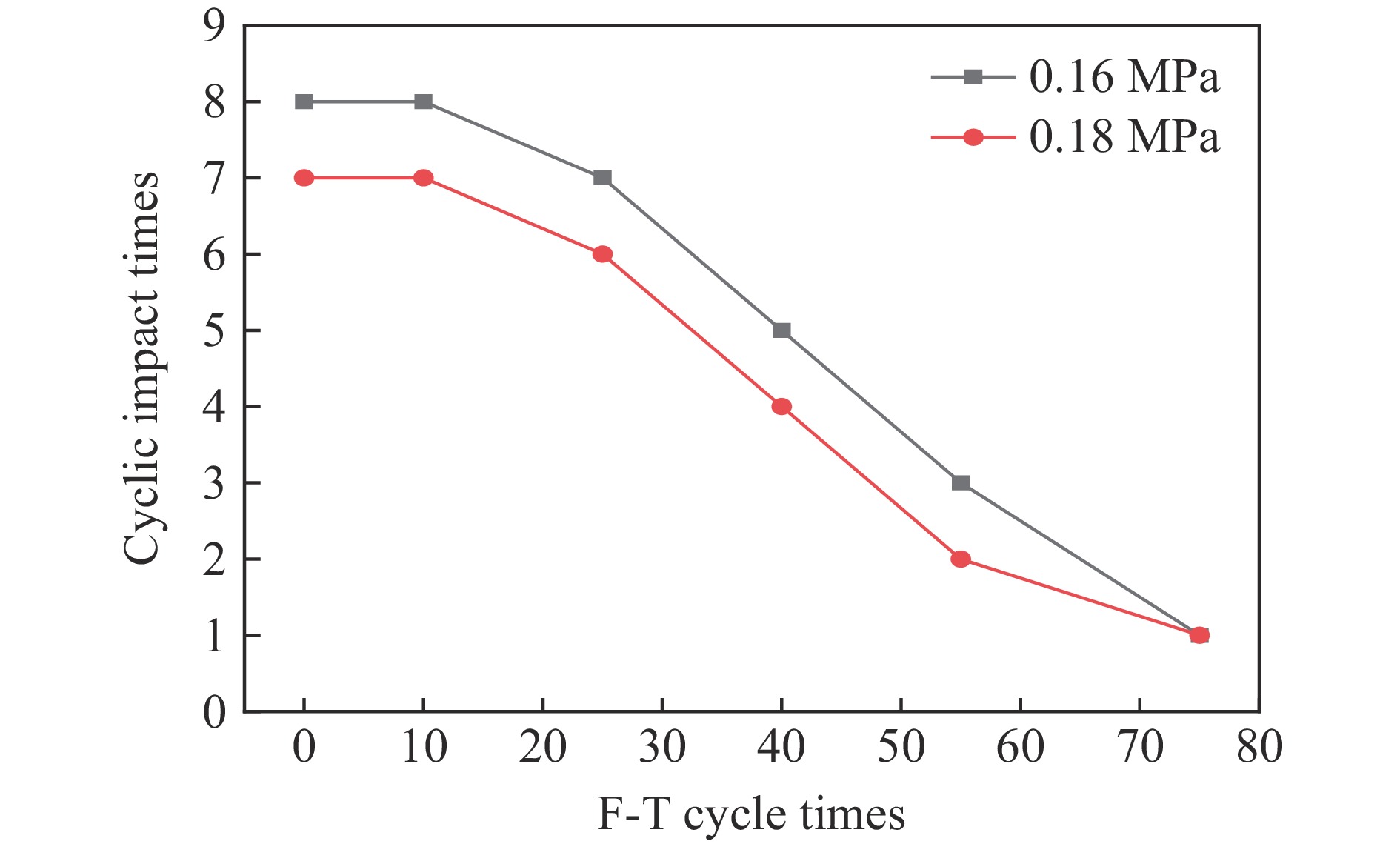
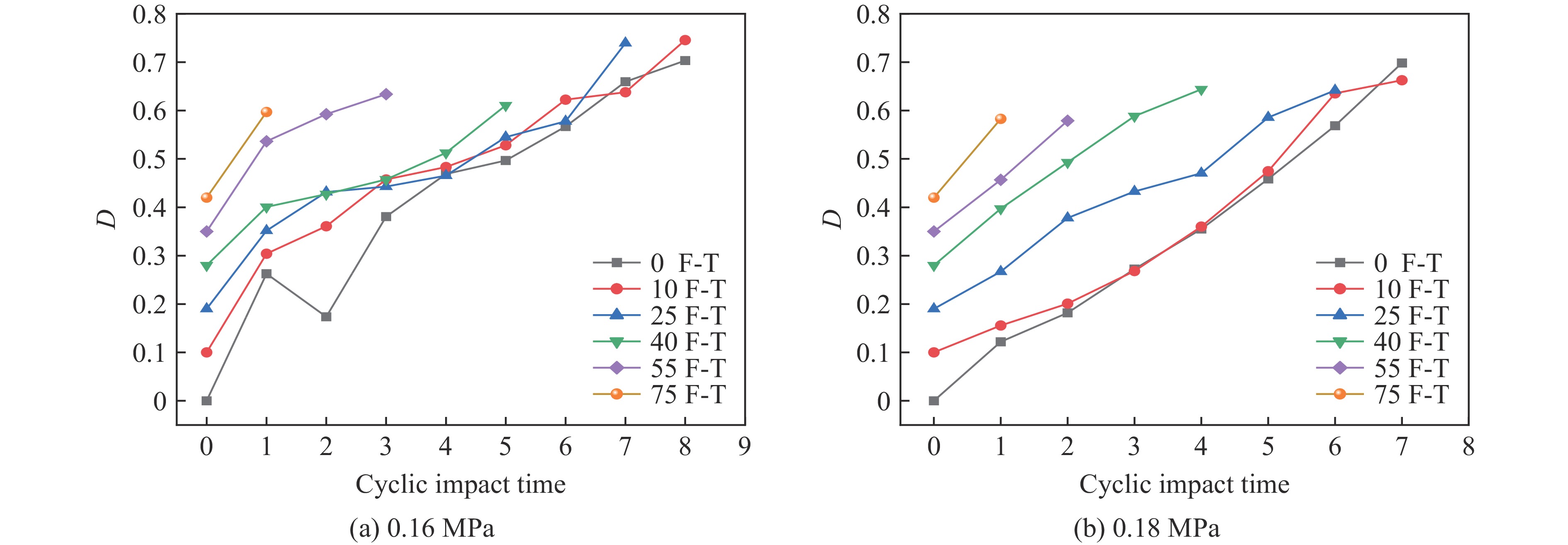
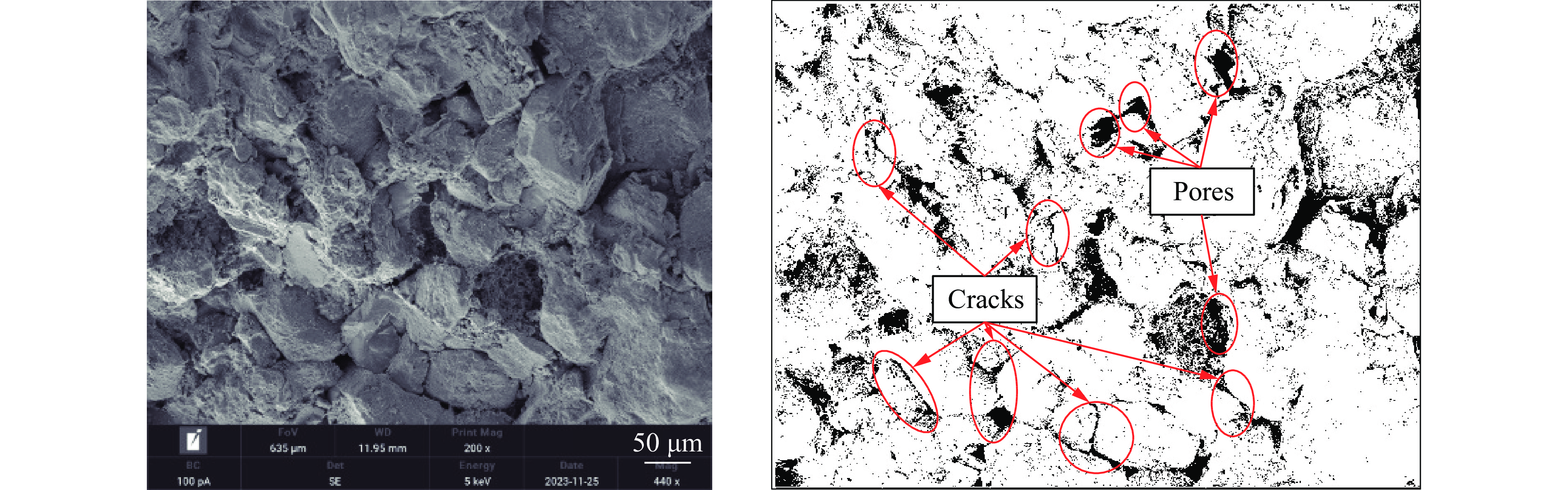
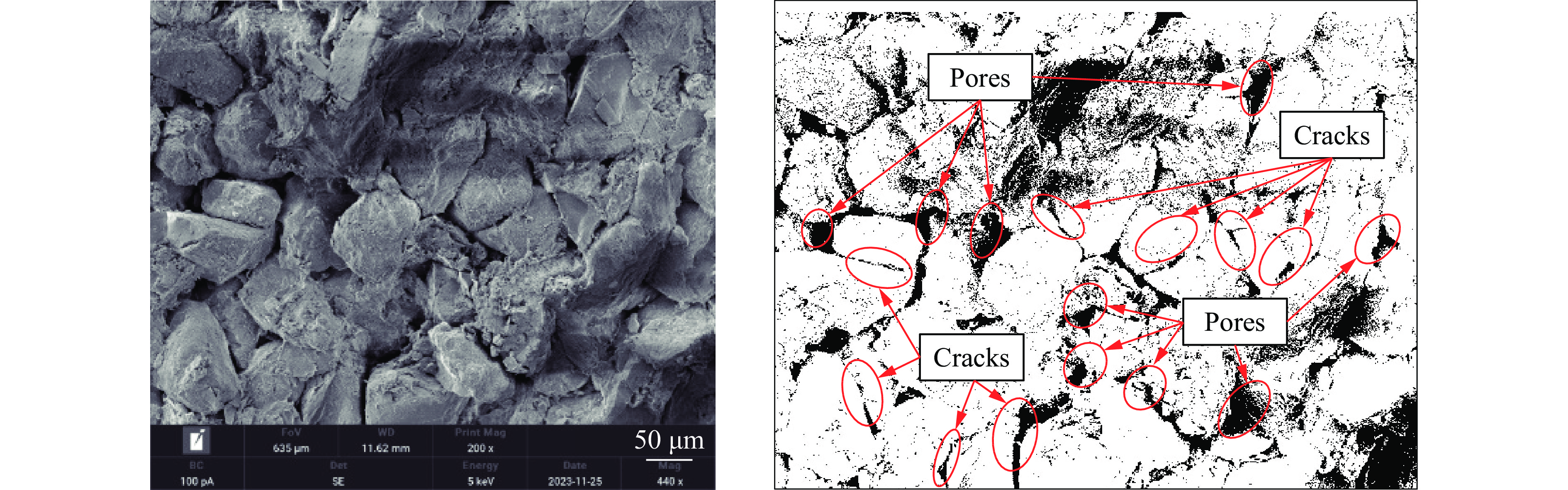
摘要: 为探索循环动力扰动作用下冻融岩体的强度和变形特性及损伤机理,开展了两种冲击气压下冻融红砂岩的循环冲击试验,研究了循环冲击次数和冻融次数对应力波传播、动态应力-应变曲线、峰值应力和峰值应变的影响规律;基于Lemaitre应变等效原理,提出了能够综合考虑循环冲击和冻融影响的累积损伤因子的计算方法,分析了冻融和循环冲击作用后红砂岩的微观结构特征。结果表明:循环冲击荷载下不同冻融次数处理后的红砂岩试样均呈拉伸破坏模式;红砂岩试样可承受的循环冲击次数与冻融次数呈负相关,冻融75次后试样在首次冲击后即达到破坏状态;循环冲击次数主要影响透射波的起跳点、峰值点对应的横坐标和振幅以及反射波的振幅,而冻融循环次数对第一次冲击时透射波的起跳点、峰值点对应的横坐标和振幅影响较大;红砂岩试样累积损伤因子与动态峰值应力呈现较好的负相关变化规律;冻融和循环冲击复合作用后红砂岩内部裂纹沿颗粒边界扩展且与孔洞连接形成较为复杂的网络。
-
关键词:
- 循环冲击 /
- 红砂岩 /
- 冻融循环 /
- 分离式Hopkinson压杆 /
- 累积损伤因子
Abstract: To study the strength, deformation characteristic and damage mechanism of freeze-thaw treated rock mass under the action of cyclic dynamic disturbance, the cyclic impact tests of freeze-thaw treated red sandstone under two kinds of impact pressure were carried out to investigate the effects of cyclic impact number and freeze-thaw number on stress wave propagation, dynamic stress-strain curve, peak stress, and peak strain. In addition, the calculation method of cumulative damage factor, which can comprehensive consider the effects of cyclic impact and freeze-thaw, is proposed based on the Lemaitre strain equivalence principle. Finally, the microstructure characteristics of red sandstone after freeze-thaw and cyclic impact are analyzed in detail. Results show that red sandstone specimens treated with different freeze-thaw number show tensile failure mode under cyclic impact load. The cyclic impact number that red sandstone specimen can withstand is negatively correlated with freeze-thaw cycle number, and red sandstone specimen after 75 freeze-thaw cycles treatments reaches the failure state after the first impact loading. Moreover, the cyclic impact number mainly affects the jump point, abscissa corresponding to peak point and amplitude of transmitted waves, and the amplitude of reflected waves. While the freeze-thaw number shows a great effect on the jump point, abscissa corresponding to peak point, and amplitude of transmitted waves during the first impact process. The cumulative damage factor of red sandstone specimen exhibits a good negative correlation with the dynamic peak stress. After the combination effects of freeze-thaw and cyclic impact, the cracks inside red sandstone spread along the grain boundary and connect with the pores to form a complex network. -
在近几十年中, 对于爆炸焊接的理论研究以及工业应用得到了迅速发展[1-2]。在炸药爆轰载荷作用下, 2金属板之间高速碰撞形成金属射流和周期性波纹状的复合界面, 通常被称为界面波。金属复合板强度以及复合率与界面波状态密切相关, 金属爆炸焊接只有在复合界面上形成大小适当的波纹才能保证复合板的结合强度。关于爆炸焊接复合界面波的形成机理[3-4]有4种经典理论:(1)复板侵彻刻入机理, 复板的周期性刻入基板并在驻点前沿形成驼峰而形成波状界面的特征; (2)亥尔姆霍尔兹失稳模型, 复板再入射流和基板之间存在速度差而形成界面波; (3)应力波模型, 在复板与基板的碰撞点处的可压缩冲击波与在自由边界反射的稀疏波相互作用而形成界面波; (4)卡门涡街模型, 在金属板碰撞点附近材料的卡尔曼涡街流动形成界面波。然而, 这些界面波理论模型还不完善, 迄今为止, 爆炸焊接界面波的形成机理仍没有统一的认识。
由于界面波的形成涉及多种物理现象, 单纯使用固体力学计算模型对此很难进行分析, 而采用爆炸焊接实验又难以观察到界面波的动态形成过程, 因此, 本文中基于冲击动力学和物质点法[5]对爆炸焊接界面波的形成过程进行三维数值模拟, 并开展相关的爆炸焊接实验。
1. 物质点法基本理论
采用物质点法求解冲击动力学问题时, 首先定义背景网格, 然后在背景网格内将连续体剖分为具有集中质量的离散质点, 再利用形函数实现质点和背景网格节点之间的2次映射, 通过求解连续方程和运动方程以及材料本构方程计算所有质点的应变和应力值[5], 在计算过程中背景网格固定不变。与其他数值计算方法相同, 物质点法满足的连续方程、运动方程和能量方程分别为:
dρdt+ρ∇⋅v=0 (1) ρa=∇⋅σ+ρb (2) ρ˙e=σ:˙ε (3) 式中:ρ为质量密度, a为加速度, b为单位体积力, v为速度, σ为柯西应力张量, e为质量热力学能, 应变率

将连续体离散为Np个质点后, 为了利用这些质点来描述连续体的变形过程, 假定质点p(p=1, 2, …, NP)在t时刻位置为xp(t), 质量为Mp, 密度为ρp(t), 速度为vp(t), 柯西应力张量为σp(t), 将每个质点看作集中质量点, 并且每个离散质点的质量保持不变, 那么连续方程自然满足。为了求解运动方程则需要采用弱形式[6]:
∫Ωρw⋅a dΩ=−∫Ωρσs:∇w dΩ+∫∂Ωcρcs⋅w dS+∫Ωρw⋅bdΩ (4) 式中:σs为比应力张量, σs=σ/ρ; Ω为当前连续体构形, ∂Ωc为指定应力边界; w为试函数; 假设边界上应力为c, 且cs=c/ρ, w在指定位移边界上为零。这样每个质点密度写成δ函数形式[6]:
ρ(x,t)=Np∑p=1Mpδ(x−x(t)p) (5) 背景网格节点包含了多个参量, 坐标xi(t)、位移ui(t)、速度vi(t)和加速度ai(t)以及试函数wi(t)等, 统一使用变量ri(t)(i=1, 2, …, Nn)表示, 则有[7]:
r(t)p=Nn∑i=1r(t)iNi(x(t)p) (6) 利用形函数实现从背景网格节点到质点的映射, 更新质点的位移、速度、应变等参量。由于物质点法采用显式积分算法, 将网格节点插值得到的参数代入式(4)可得:
Nn∑i=1w(t)i⋅Nn∑j=1m(t)ija(t)j=−Nn∑i=1w(t)i⋅Np∑p=1Mpσ(s,t)p⋅∇Ni(x)|xϕ)+Nn∑i=1w(t)i⋅(c(t)i+b(t)i)m(t)ij=Np∑p=1MpNi(x(t)p)Nj(x(t)p)c(t)i=Np∑p=1Mpc(s,t)pNi(x(t)p)/hb(t)i=Np∑p=1Mpb(x(t)p,t)Ni(x(t)p) Nn∑i=1w(t)i⋅Nn∑j=1m(t)ija(t)j=−Nn∑i=1w(t)i⋅Np∑p=1Mpσ(s,t)p⋅∇Ni(x)|xϕ)+Nn∑i=1w(t)i⋅(c(t)i+b(t)i) (7) m(t)ij=Np∑p=1MpNi(x(t)p)Nj(x(t)p) (8) c(t)i=Np∑p=1Mpc(s,t)pNi(x(t)p)/h (9) b(t)i=Np∑p=1Mpb(x(t)p,t)Ni(x(t)β) (10) 将运动方程(7)写成以下形式:
Nn∑j=1m(t)ija(t)j=(f(t)i)int+(f(t)i)exti=1,2,⋯,Nn (11) (f(t)i)int=−Np∑p=1Mpσ(s,t)p⋅∇Ni|xφt) (12) (f(t)i)ext=b(t)p+c(t)p (13) 采用行求和方式获得对角质量阵, 这样式(8)和式(10)分别变为:
m(t)i=Np∑p=1MpNi(x(t)p,t) (14) b(t)i=m(t)ib(x(t)p,t) (15) 从而式(11)变为:
m(t)ia(t)i=(f(t)i)int+(f(t)i)exti=1,2,⋯,Nn (16) 基于材料本构模型对质点应力进行更新, 计算应力增量为:
Δσ(t+Δt)p=E:Δε(t+Δt)p (17) 式中:E是切线刚度张量。计算质点当前时刻的应力张量为:
σ(t+Δt)p=σ(t)p+Δσ(t+Δt)p (18) 计算当前时刻质点内能为:
e(t+Δt)p=e(t)p+σ(t+Δt)p:Δ˙ε(t+Δt)p/[ρ(t+Δt)pΔt] (19) 物质点法的理论模型以及数值计算方法都较成熟, 对于爆炸冲击问题通常采用显式积分算法完成。关于物质点法的具体计算步骤和求解过程可以参考文献[8-12]。
2. 爆炸焊接数值计算模型
2.1 爆炸焊接布置形式
实际爆炸焊接工程中, 一般采用飞板与基板平行的方式进行爆炸焊接, 2金属板之间保持一定间距, 为了避免飞板上表面被炸药烧伤, 还要加一薄缓冲层, 如图 1所示, 爆炸焊接装置主要由雷管、炸药层、缓冲层、飞板、支撑以及基板等组成。
在爆炸焊接过程中, 雷管起爆后, 炸药会在起爆端不远处达到稳定的爆轰状态, 爆轰波以vd的速度向炸药未起爆区域传播, 如图 2所示。
炸药爆炸产物驱动飞板向下运动, 在预置间隙中经历加速过程, 与基板发生碰撞之前获得较高的飞行速度vp, 称为碰撞速度。随着炸药稳定爆轰的推进, 碰撞点会以稳定速度vc向未焊合区域行进, 该速度称为碰撞点移动速度, 平行布置时vc=vd。在碰撞点附近会产生高压, 同时金属复合界面会受到剧烈的剪切作用, 进而发生强烈的塑性变形, 导致界面材料升温, 甚至发生熔化流动, 并形成向前的微喷射射流使界面完成自清理, 最终实现爆炸焊接。由于应用物质点法对爆炸焊接界面波的模拟研究计算量很大, 不可能对炸药爆轰、基板与飞板碰撞、焊接界面形成的全部过程进行模拟。同时, 基于爆炸焊接的实际情况, 炸药爆轰驱动飞板, 飞板在预置间隙加速后, 爆轰产物对飞板背面施加的压力已经下降为0.1~1GPa量级, 飞板以一定速度与基板碰撞后的压力却高达10~100GPa量级, 远大于炸药爆轰对飞板的压力, 爆轰压力在2金属板接触后对爆炸焊接界面的影响已经很小。因此, 在对爆炸焊接界面的数值模拟过程中可忽略炸药爆炸驱动过程, 单独对高速碰撞过程进行建模, 爆炸焊接的计算模型如图 3所示。
飞板向下以一定速度和倾斜角度β碰撞基板, 选择飞板与基板的倾斜角度应该在爆炸焊接窗口上下限的最大与最小弯折角之间, 焊接窗口上下限是指保证爆炸焊接可以复合的飞板碰撞基板的最大与最小速度, 很多金属的爆炸焊接窗口上下限已经通过实验测定。在已知炸药爆速的前提下, 由飞板碰撞点速度计算公式[13]:
vp=2vdsin(β/2) (20) 可以获得本文计算模型飞板的初始倾斜角度, 这样设计的计算模型与轻气炮加速、电磁加速碰撞焊接情况是完全相符的。当确定了飞板与基板材料的爆炸焊接窗口碰撞点速度的上下限以及炸药的爆轰速度后, 由式(20)就能够计算出飞板与基板的碰撞角, 飞板的初始速度、倾斜角度和垂直向下的初始速度也可以由此确定。
2.2 爆炸焊接材料模型
确定爆炸焊接的计算模型后, 假定飞板和基板材料都为普通碳钢, 普通碳钢的爆炸焊接窗口下限为272m/s, 飞板的初始速度设计为300m/s, 碰撞点速度处于焊接窗口中间。由于爆炸焊接整个过程历时较短, 在微秒量级范围内, 所以忽略热传导并假定为绝热过程。2块金属板碰撞过程中, 复合界面材料发生塑性流动。采用物质点法计算与有限元法类似, 为了描述爆炸焊接复合界面处的金属塑性流动变形, 材料模型采用Johnson-Cook塑性模型[14]:
σy=[A+B(εpe)n](1+Cln˙ε∗) (21) 式中:σy为Von Mises流动应力, εep为等效塑性应变,
 为相对等效塑性应变率, 取1s-1, B、n为材料应变硬化特性参数, C为材料应变率敏感特性参数; 式中第1个乘积因子表示当˙ε∗=1.0时流动应力同等效塑性应变之间的关系, 第2个乘积因子表示应变率强化效应。
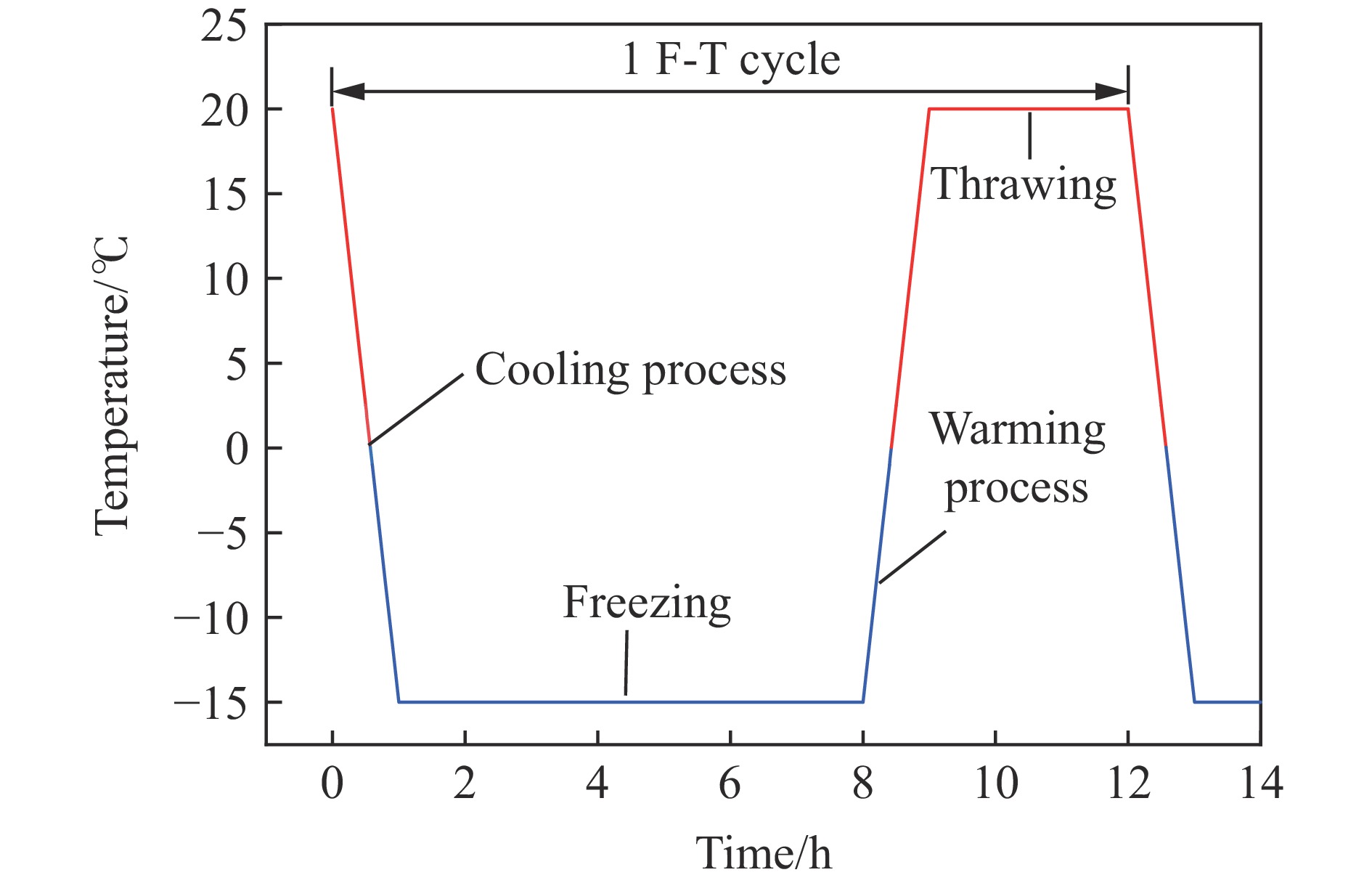
为相对等效塑性应变率, 取1s-1, B、n为材料应变硬化特性参数, C为材料应变率敏感特性参数; 式中第1个乘积因子表示当˙ε∗=1.0时流动应力同等效塑性应变之间的关系, 第2个乘积因子表示应变率强化效应。
爆炸焊接过程还涉及到材料在高压条件下的力学行为, 所以对其还要运用相应的状态方程进行描述, 这里材料状态方程选用Mie-Grüneisen状态方程。当材料处于拉伸膨胀状态时, 状态方程形式为:
p=ρ0c2gμμ+(γ0+aμ)E (22) 当材料处于压缩状态时, 状态方程形式为:
p=ρ0c2gμ(1+(1−γ02)μ−a2μ2)(1−(s1−1)μ−s2μ2μ+1−s3μ3μ+1)2+(γ0+aμ)E (23) 式中:cg、a、s1、s2和s3是与材料冲击压缩特性有关的常数, cg是冲击波速度(us)-质点速度(up)曲线的截距, s1、s2和s3是us-up曲线斜率的系数, a是一阶体积修正系数,γ0是Grüneisen系数, E为材料比内能, 体积变化率μ=ρ/ρ0-1。
本文中普通钢状态方程模型参数[15-16]为:ρ=7 850kg/m3, a=0.43, G=124MPa, A=400MPa, B=220MPa, n=0.08, C=0.025, cg=4 573m/s, s1=1.33, s2=0, s3=0, γ0=1.07。
3. 爆炸焊接界面波形成数值模拟
假设爆炸焊接计算模型的尺寸板X方向板长100mm, Y方向板厚20mm, Z方向板宽16mm, 在Z方向的板两侧面施加固定约束条件。利用物质点法对本文中设计的爆炸焊接计算模型进行三维数值模拟之前, 需要划分背景网格和质点单元等, 三维背景网格单元为六面体立方体单元, 网格单元大小为2mm, 在X、Y、Z3个方向划分的背景网格单元数量分别为100、100和50, 每个背景网格单元内的质点数量划分为8个, 飞板和基板的材料模型选择Johnson-Cook模型, 材料在高压下的力学行为选用Mie-Grüneisen状态方程进行描述。爆炸焊接计算模型的三维前处理结果见图 4。
爆炸焊接数值模拟是利用自主研发的物质点法程序SPS2.0完成的, 该程序是应用C++编制实现的, 并提供了基本的后处理功能, 图 5给出了爆炸焊接数值模拟的三维变形图, 图 6给出了爆炸焊接数值模拟的中间剖面变形图。爆炸焊接中间剖面的等效应力σe后处理云图, 见图 7。爆炸焊接中间剖面的压力p场后处理云图, 见图 8。
为了验证物质点法计算的准确性, 本文中设计了普通钢板爆炸焊接实验:飞板和基板采用平行布置, 基板和飞板的长度均为1 000mm, 宽度均为400mm, 厚度均为20mm; 炸药采用铵油, 爆速为2 400m/s; 2金属板之间的支撑间距为10~12mm, 保证飞板碰撞速度vp在爆炸焊接窗口内, 约300m/s。爆炸焊接实验后, 取复合板中间部分做为试样进行研磨, 利用电子金相显微镜获得复合界面金相显微组织照片, 如图 9所示。
将爆炸焊接复合界面的金相组织以及界面波纹形状(图 9)与数值模拟结果(图 6)进行比较可知, 两者的界面波形状基本一致, 由此说明采用物质点法对爆炸焊接界面波形成问题的模拟结果具有一定的准确性和可靠性。
分析复合界面的金相照片, 可以发现界面处的金属材料有熔化现象, 这与数值模拟结果(图 7)是相符的。在飞板与基板碰撞点位置附近计算的等效应力等于零, 说明飞板与基板在冲击碰撞位置的材料几乎处于流体状态。为什么在碰撞点附近区域出现熔化流体现象, 其中重要原因是该处的压力最高。数值计算获得的压力大小在12GPa以上, 远高于材料强度。通过数值模拟得到的爆炸焊接压力分布图(图 8)可以直观地说明这一点。
4. 结论
(1) 爆炸焊接界面波的形成机理是由于飞板与基板的高速碰撞, 在碰撞点处压力最高, 材料发生熔化形成流体涡旋变形并且迅速冷却而复合为一体, 这个过程随着碰撞点的移动不断地向前推进, 使得2金属板复合形成周期性波状界面。
(2) 物质点法的三维数值模拟结果与爆炸焊接实验结果比较符合, 由此说明物质点法用于模拟爆炸焊接界面波的形成过程具有实际工程应用价值。
(3) 此外, 基于本文数值模拟结果也证明了物质点法用于爆炸焊接和冲击动力学问题计算具有突出的优点:一是避免了有限元法在复合界面处难以克服的网格畸变问题; 二是与其他无网格法相比, 物质点法计算效率更高, 本文数值模拟质点数量10万多, 台式机计算时间约1h, 而利用光滑粒子流体动力学法则需要几天时间。因此, 物质点法在爆炸焊接、高速冲击、塑性大变形等力学问题计算中具有广泛的应用前景。
-
表 1 红砂岩试样物理力学参数
Table 1. Physical and mechanical parameters of red sandstone specimen
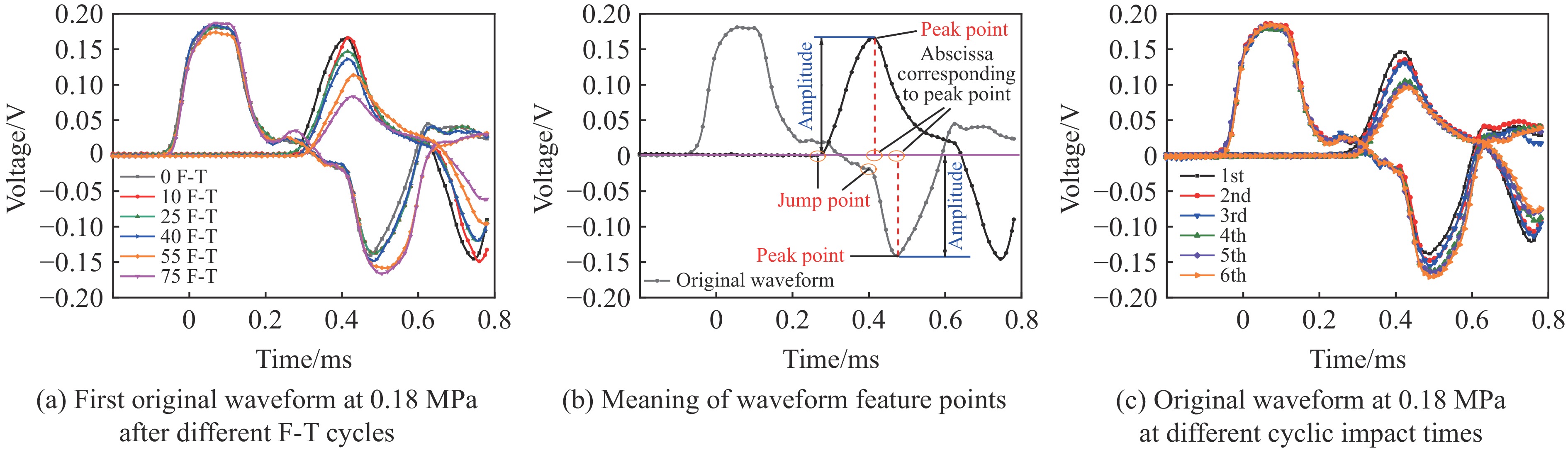
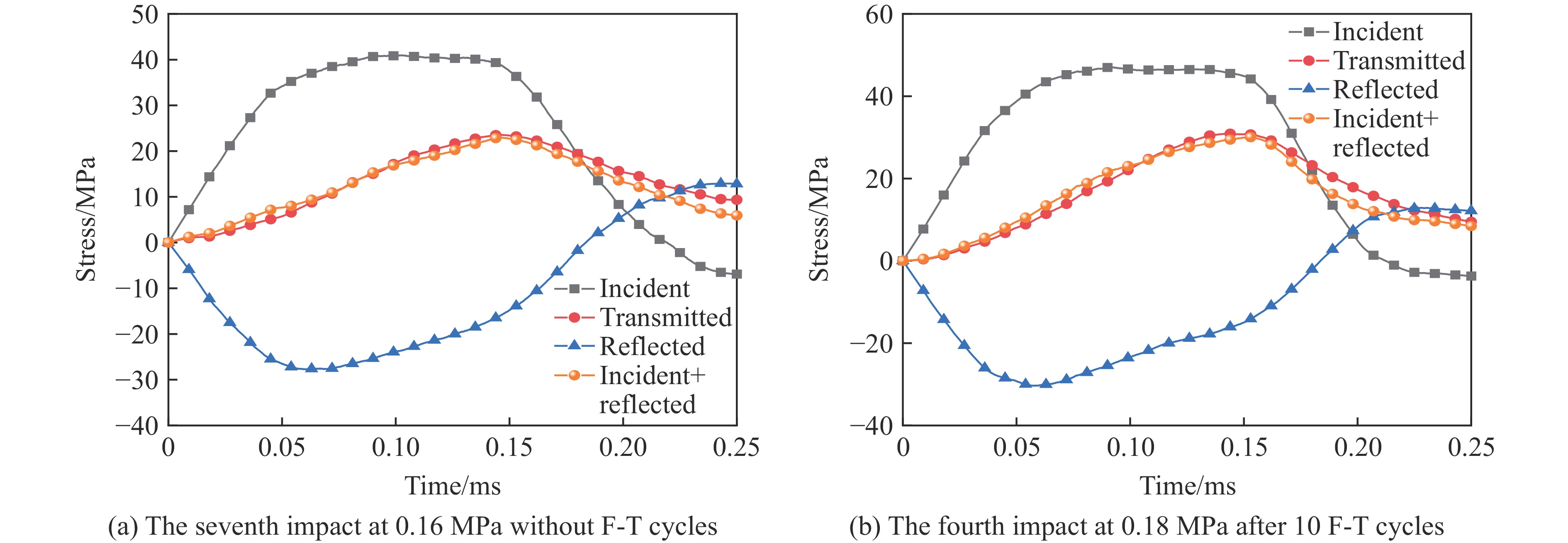
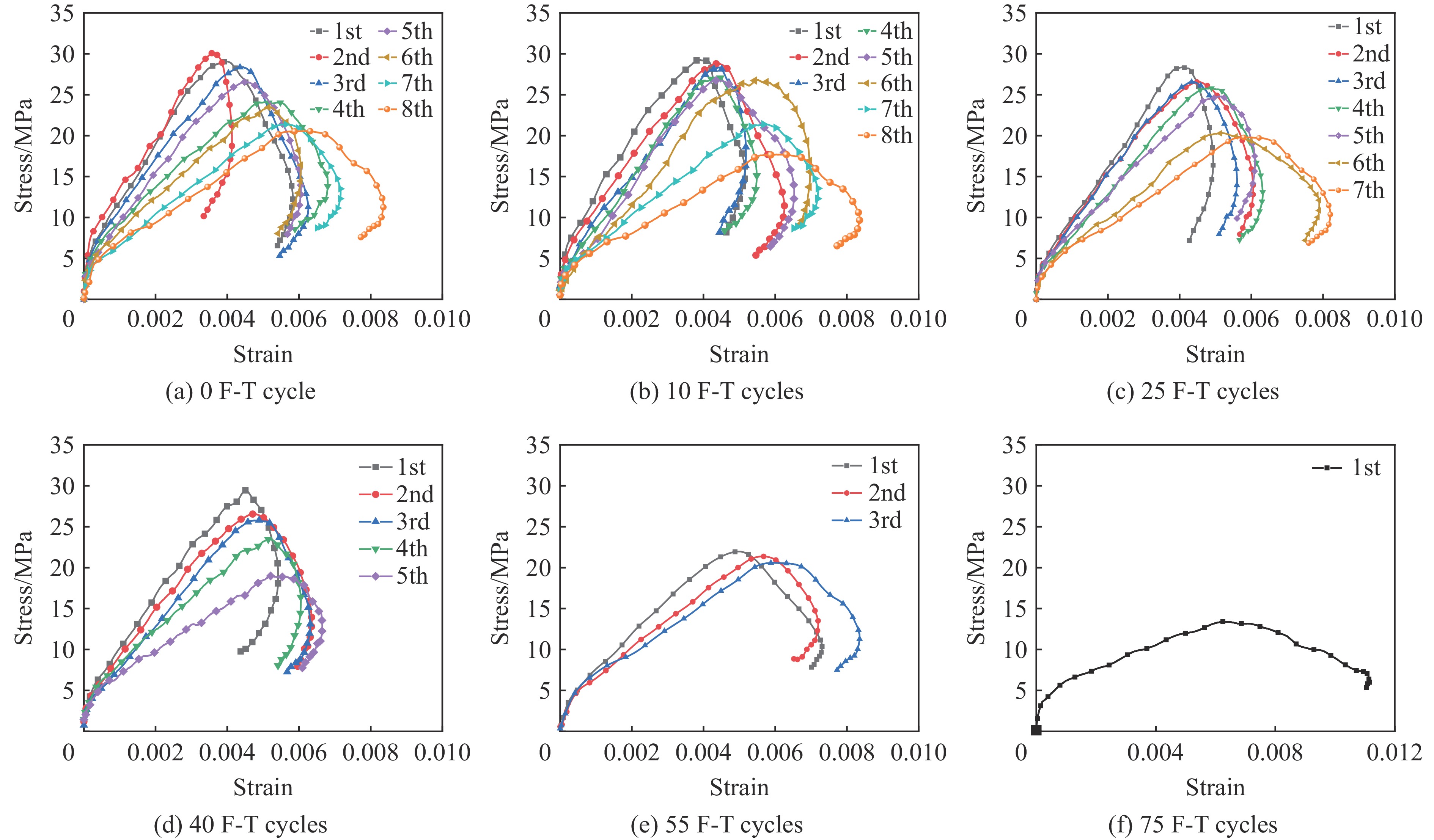
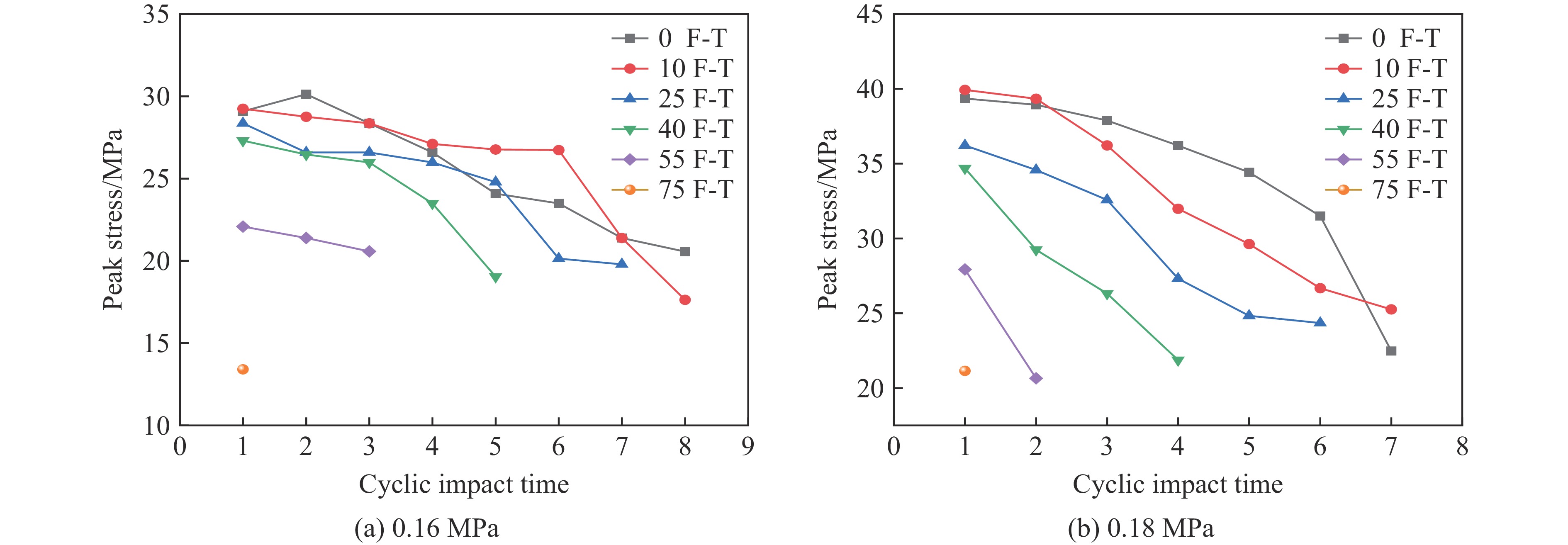
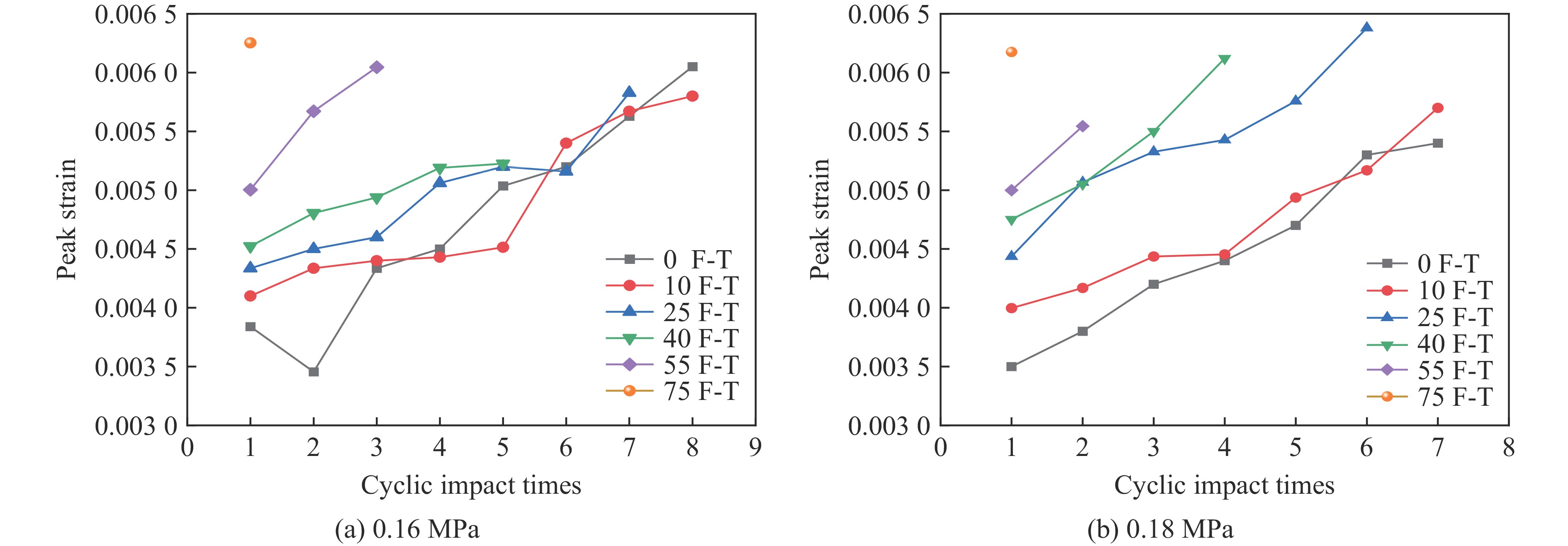
密度/(kg·m−3) 孔隙率/% 静态抗压强度/MPa 静态变形模量/GPa 动态抗压强度/MPa 动态变形模量/GPa 2391 7.61 76.65 4.65 97.53 19.32 注:准静态应变率为1.67×10−4 s−1,动态应变率为212 s−1。 表 2 冲击气压为0.16 MPa时红砂岩试样的动态峰值应力、峰值应变和变形模量
Table 2. Dynamic peak stresses, peak strains, and deformation moduli of red sandstone specimens at the impact gas pressure of 0.16 MPa
冻融循环
次数循环冲击
次数动态峰值
应力/MPa动态峰值
应变动态变形
模量/GPa冻融循环
次数循环冲击
次数动态峰值
应力/MPa动态峰值
应变动态变形
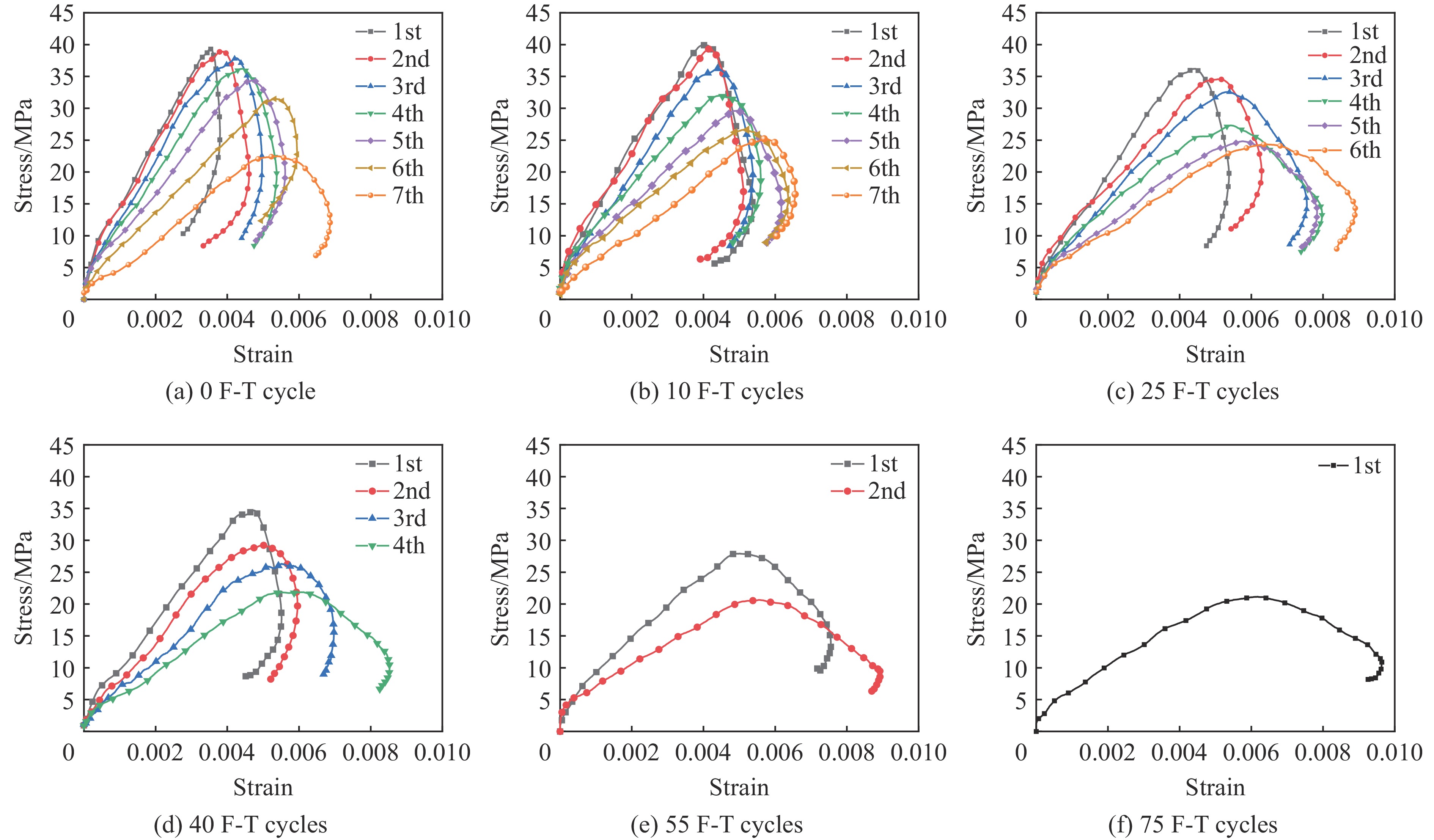
模量/GPa0 1 29.08 0.0038 11.13 25 1 28.36 0.0043 9.35 2 30.12 0.0035 12.48 2 26.63 0.0044 8.02 3 28.40 0.0042 9.35 3 26.58 0.0046 7.84 4 26.59 0.0045 8.01 4 25.97 0.0051 7.48 5 24.08 0.0050 7.60 5 24.78 0.0052 6.23 6 23.49 0.0052 6.53 6 20.13 0.0052 5.73 7 21.39 0.0056 5.14 7 19.79 0.0058 3.39 8 20.56 0.0061 4.48 40 1 27.30 0.0045 8.49 10 1 29.24 0.0041 10.25 2 26.46 0.0048 8.01 2 28.76 0.0043 9.36 3 25.90 0.0049 7.48 3 28.36 0.0044 7.84 4 23.52 0.0052 6.54 4 27.11 0.0044 7.45 5 19.04 0.0054 4.98 5 26.76 0.0045 6.76 55 1 22.08 0.0051 6.06 6 26.73 0.0054 5.36 2 21.38 0.0057 5.12 7 21.39 0.0056 5.13 3 20.52 0.0060 4.48 8 17.64 0.0058 3.56 75 1 13.41 0.0063 4.98 表 3 冲击气压为0.18 MPa时红砂岩试样的动态峰值应力、峰值应变和变形模量
Table 3. Dynamic peak stresses, peak strains, and deformation moduli of red sandstone specimens at the impact gas pressure of 0.18 MPa
冻融循环
次数循环冲击
次数动态峰值
应力/MPa动态峰值
应变动态变形
模量/GPa冻融循环
次数循环冲击
次数动态峰值
应力/MPa动态峰值
应变动态变形
模量/GPa0 1 39.35 0.0035 13.25 25 1 36.23 0.0043 10.83 2 38.93 0.0038 12.35 2 34.57 0.0051 8.91 3 37.88 0.0042 10.98 3 32.57 0.0053 8.00 4 36.21 0.0044 9.74 4 27.31 0.0054 7.39 5 34.42 0.0047 8.18 5 24.83 0.0058 5.61 6 31.50 0.0053 6.51 6 24.34 0.0064 4.78 7 22.48 0.0054 4.56 40 1 34.69 0.0048 8.56 10 1 39.93 0.0040 12.66 2 29.24 0.0051 6.86 2 39.33 0.0042 11.92 3 26.31 0.0055 5.33 3 36.21 0.0044 10.83 4 21.87 0.0061 4.49 4 31.90 0.0045 9.35 55 1 27.92 0.0051 7.53 5 29.62 0.0049 7.58 2 20.65 0.0055 5.34 6 26.68 0.0052 5.16 75 1 21.14 0.0062 5.23 7 25.25 0.0057 4.76 -
[1] 马泗洲, 刘科伟, 杨家彩, 等. 初始应力下岩体爆破损伤特性及破裂机理 [J]. 爆炸与冲击, 2023, 43(10): 105201. DOI: 10.11883/bzycj-2023-0151.MA S Z, LIU K W, YANG J C, et al. Blast-induced damage characteristics and fracture mechanism of rock mass under initial stress [J]. Explosion and Shock Waves, 2023, 43(10): 105201. DOI: 10.11883/bzycj-2023-0151. [2] 倪苏黔, 徐颖, 葛进进, 等. 干-酸侵蚀下深地白砂岩动静态损伤特性研究 [J]. 岩石力学与工程学报, 2023, 42(10): 2528–2539. DOI: 10.13722/j.cnki.jrme.2022.1218.NI S Q, XU Y, GE J J, et al. Dynamic and static damage characteristics of deep-earth white sandstone under dry-acid erosion [J]. Chinese Journal of Rock Mechanics and Engineering, 2023, 42(10): 2528–2539. DOI: 10.13722/j.cnki.jrme.2022.1218. [3] 王俊奇, 汪志刚. 确定裂隙岩体渗透系数张量的一维环单元模型研究 [J]. 水利学报, 2023, 54(5): 575–586. DOI: 10.13243/j.cnki.slxb.20220458.WANG J Q, WANG Z G. Study on one-dimensional ring unit model for determining the permeability coefficient tensor of fractured rock masses [J]. Journal of Hydraulic Engineering, 2023, 54(5): 575–586. DOI: 10.13243/j.cnki.slxb.20220458. [4] SONG Z Y, WANG Y, KONIETZKY H, et al. Mechanical behavior of marble exposed to freeze-thaw-fatigue loading [J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 138: 104648. DOI: 10.1016/j.ijrmms.2021.104648. [5] 高要辉, 张春生, 苏方声, 等. 深部硬岩剪切边界下应力诱发片帮的机制研究 [J]. 岩土力学, 2022, 43(4): 1103–1111, 1122. DOI: 10.16285/j.rsm.2021.1220.GAO Y H, ZHANG C S, SU F S, et al. Mechanism of stress-induced spalling of deep hard rocks under shear boundary condition [J]. Rock and Soil Mechanics, 2022, 43(4): 1103–1111, 1122. DOI: 10.16285/j.rsm.2021.1220. [6] 金解放, 张睿, 王熙博, 等. 岩石梯度应力加载试验装置研制及初步试验研究 [J]. 岩石力学与工程学报, 2020, 39(8): 1547–1559. DOI: 10.13722/j.cnki.jrme.2019.1206.JIN J F, ZHANG R, WANG X B, et al. Development of a rock gradient stress loading test device and its primary application [J]. Chinese Journal of Rock Mechanics and Engineering, 2020, 39(8): 1547–1559. DOI: 10.13722/j.cnki.jrme.2019.1206. [7] 姜亚成, 周磊, 朱哲明, 等. 冻融循环对含纯Ⅰ型裂隙围岩的动态起裂特性影响规律 [J]. 爆炸与冲击, 2021, 41(4): 043104. DOI: 10.11883/bzycj-2020-0330.JIANG Y C, ZHOU L, ZHU Z M, et al. Effects of freeze-thaw cycles on dynamic fracture initiation characteristics of surrounding rock with pure Ⅰ type fracture under impact loads [J]. Explosion and Shock Waves, 2021, 41(4): 043104. DOI: 10.11883/bzycj-2020-0330. [8] 宋凯文, 黄俊红, 罗忆, 等. 循环冲击荷载下的礁灰岩力学特性研究 [J]. 岩石力学与工程学报, 2023, 42(S2): 3956–3965. DOI: 10.13722/j.cnki.jrme.2022.0935.SONG K W, DAI J H, LUO Y, et al. Mechanical properties of reef limestone under cyclic impact loading [J]. Chinese Journal of Rock Mechanics and Engineering, 2023, 42(S2): 3956–3965. DOI: 10.13722/j.cnki.jrme.2022.0935. [9] 罗宁, 索云琛, 张浩浩, 等. 循环冲击层理煤岩动力学行为及破坏规律研究 [J]. 爆炸与冲击, 2023, 43(4): 043102. DOI: 10.11883/bzycj-2022-0253.LUO N, SUO Y C, ZHANG H H, et al. On dynamic behaviors and failure of bedding coal rock subjected to cyclic impact [J]. Explosion and Shock Waves, 2023, 43(4): 043102. DOI: 10.11883/bzycj-2022-0253. [10] LI R, ZHU J B, QU H L, et al. An experimental investigation on fatigue characteristics of granite under repeated dynamic tensions [J]. International Journal of Rock Mechanics and Mining Sciences, 2022, 158: 105185. DOI: 10.1016/j.ijrmms.2022.105185. [11] ZHOU T, HAN Z Y, LI D Y, et al. Experimental study of the mechanical and fracture behavior of flawed sandstone subjected to coupled static-repetitive impact loading [J]. Theoretical and Applied Fracture Mechanics, 2022, 117: 103161. DOI: 10.1016/j.tafmec.2021.103161. [12] LI X B, LOK T S, ZHAO J. Dynamic characteristics of granite subjected to intermediate loading rate [J]. Rock Mechanics and Rock Engineering, 2005, 38(1): 21–39. DOI: 10.1007/s00603-004-0030-7. [13] 王志亮, 杨辉, 田诺成. 单轴循环冲击下花岗岩力学特性与损伤演化机理 [J]. 哈尔滨工业大学学报, 2020, 52(2): 59–66. DOI: 10.11918/201811085.WANG Z L, YANG H, TIAN N C. Mechanical property and damage evolution mechanism of granite under uniaxial cyclic impact [J]. Journal of Harbin Institute of Technology, 2020, 52(2): 59–66. DOI: 10.11918/201811085. [14] WANG X Y, LIU Z Y, GAO X C, et al. Dynamic characteristics and fracture process of marble under repeated impact loading [J]. Engineering Fracture Mechanics, 2022, 276: 108926. DOI: 10.1016/j.engfracmech.2022.108926. [15] 李地元, 孙小磊, 周子龙, 等. 多次冲击荷载作用下花岗岩动态累计损伤特性 [J]. 实验力学, 2016, 31(6): 827–835. DOI: 10.7520/1001-4888-16-009.LI D Y, SUN X L, ZHOU Z L, et al. On the dynamic accumulated damage characteristics of granite subjected to repeated impact load action [J]. Journal of Experimental Mechanics, 2016, 31(6): 827–835. DOI: 10.7520/1001-4888-16-009. [16] MENG X Z, ZHANG H M, YUAN C, et al. Damage constitutive prediction model for rock under freeze-thaw cycles based on mesoscopic damage definition [J]. Engineering Fracture Mechanics, 2023, 293: 109685. DOI: 10.1016/j.engfracmech.2023.109685. [17] NIU C Y, ZHU Z M, ZHOU L, et al. Study on the microscopic damage evolution and dynamic fracture properties of sandstone under freeze-thaw cycles [J]. Cold Regions Science and Technology, 2021, 191: 103328. DOI: 10.1016/j.coldregions.2021.103328. [18] 肖鹏, 陈有亮, 杜曦, 等. 冻融循环作用下砂岩的力学特性及细观损伤本构模型研究 [J]. 岩土工程学报, 2023, 45(4): 805–815. DOI: 10.11779/CJGE20220219.XIAO P, CHEN Y L, DU X, et al. Mechanical properties of sandstone under freeze-thaw cycles and studies on meso-damage constitutive model [J]. Chinese Journal of Geotechnical Engineering, 2023, 45(4): 805–815. DOI: 10.11779/CJGE20220219. [19] 于洋, 徐倩, 刁心宏, 等. 循环冲击对围压作用下砂岩特征的影响 [J]. 华中科技大学学报(自然科学版), 2019, 47(6): 127–132. DOI: 10.13245/j.hust.190623.YU Y, XU Q, DIAO X H, et al. Effect of cyclic impact on sandstone characteristics under confining pressures [J]. Journal of Huazhong University of Science and Technology (Natural Science Edition), 2019, 47(6): 127–132. DOI: 10.13245/j.hust.190623. [20] 唐礼忠, 程露萍, 王春, 等. 高静载条件下受频繁动力扰动时蛇纹岩动力学特性研究 [J]. 岩土力学, 2016, 37(10): 2737–2745. DOI: 10.16285/j.rsm.2016.10.001.TANG L Z, CHENG L P, WANG C, et al. Dynamic characteristics of serpentinite under condition of high static load and frequent dynamic disturbance [J]. Rock and Soil Mechanics, 2016, 37(10): 2737–2745. DOI: 10.16285/j.rsm.2016.10.001. [21] 闻磊, 梁旭黎, 冯文杰, 等. 冲击损伤砂岩动静组合加载力学特性研究 [J]. 岩土力学, 2020, 41(11): 3540–3552. DOI: 10.16285/j.rsm.2020.0214.WEN L, LIANG X L, FENG W J, et al. An investigation of the mechanical properties of sandstone under coupled static and dynamic loading [J]. Rock and Soil Mechanics, 2020, 41(11): 3540–3552. DOI: 10.16285/j.rsm.2020.0214. [22] 吕晓聪, 许金余, 赵德辉, 等. 冲击荷载循环作用下砂岩动态力学性能的围压效应研究 [J]. 工程力学, 2011, 28(1): 138–144.LV X C, XU J Y, ZHAO D H, et al. Research on confining pressure effect of sandstone dynamic mechanical performance under the cyclical impact loadings [J]. Engineering Mechanics, 2011, 28(1): 138–144. [23] 田诺成, 王志亮, 熊峰, 等. 循环冲击荷载下轴压对花岗岩动力学特性的影响 [J]. 哈尔滨工业大学学报, 2021, 53(5): 156–164. DOI: 10.11918/201908134.TIAN N C, WANG Z L, XIONG F, et al. Influence of axial pressure on dynamic mechanical properties of granite under cyclic impact loading [J]. Journal of Harbin Institute of Technology, 2021, 53(5): 156–164. DOI: 10.11918/201908134. [24] 金解放, 李夕兵, 殷志强, 等. 循环冲击下波阻抗定义岩石损伤变量的研究 [J]. 岩土力学, 2011, 32(5): 1385–1393, 1410. DOI: 10.3969/j.issn.1000-7598.2011.05.017.JIN J F, LI X B, YIN Z Q, et al. A method for defining rock damage variable by wave impedance under cyclic impact loadings [J]. Rock and Soil Mechanics, 2011, 32(5): 1385–1393, 1410. DOI: 10.3969/j.issn.1000-7598.2011.05.017. [25] SHU R H, YIN T B, LI X B, et al. Effect of thermal treatment on energy dissipation of granite under cyclic impact loading [J]. Transactions of Nonferrous Metals Society of China, 2019, 29(2): 385–396. DOI: 10.1016/S1003-6326(19)64948-4. [26] WANG Z L, TIAN N C, WANG J G, et al. Experimental study on damage mechanical characteristics of heat-treated granite under repeated impact [J]. Journal of Materials in Civil Engineering, 2018, 30(11): 04018274. DOI: 10.1061/(ASCE)MT.1943-5533.0002465. [27] WANG Z L, TIAN N C, WANG J G, et al. Mechanical response and energy dissipation analysis of heat-treated granite under repeated impact loading [J]. Computers, Materials & Continua, 2019, 59(1): 275–296. DOI: 10.32604/cmc.2019.04247. [28] WANG P, YIN T B, LI X B, et al. Dynamic properties of thermally treated granite subjected to cyclic impact loading [J]. Rock Mechanics and Rock Engineering, 2019, 52(4): 991–1010. DOI: 10.1007/s00603-018-1606-y. [29] 贾蓬, 毛松泽, 卢佳亮, 等. 冻融循环对绿砂岩动态抗压性能影响的试验研究 [J]. 北京理工大学学报, 2023, 43(8): 841–851. DOI: 10.15918/j.tbit1001-0645.2022.194.JIA P, MAO S Z, LU J L, et al. Experimental study on the effect of freeze-thaw cycles on the dynamic characteristics of green sandstone [J]. Transactions of Beijing Institute of Technology, 2023, 43(8): 841–851. DOI: 10.15918/j.tbit1001-0645.2022.194. [30] 孟凡东, 翟越, 李宇白, 等. 冻融循环作用后砂岩的动态抗拉性能及能量演化试验研究 [J]. 岩石力学与工程学报, 2021, 40(12): 2445–2453. DOI: 10.13722/j.cnki.jrme.2021.0289.MENG F D, ZHAI Y, LI Y B, et al. Experimental study on dynamic tensile properties and energy evolution of sandstone after freeze-thaw cycles [J]. Chinese Journal of Rock Mechanics and Engineering, 2021, 40(12): 2445–2453. DOI: 10.13722/j.cnki.jrme.2021.0289. [31] 张蓉蓉, 经来旺, 马冬冬. 冻融和热冲击循环作用后红砂岩SHPB试验和本构模型研究 [J]. 振动与冲击, 2022, 41(9): 267–275. DOI: 10.13465/j.cnki.jvs.2022.09.034.ZHANG R R, JING L W, MA D D. SHPB tests and constitutive model of red-sandstone after freeze-thaw and thermal shock cycles [J]. Journal of Vibration and Shock, 2022, 41(9): 267–275. DOI: 10.13465/j.cnki.jvs.2022.09.034. [32] MA Q Y, MA D D, YAO Z M. Influence of freeze-thaw cycles on dynamic compressive strength and energy distribution of soft rock specimen [J]. Cold Regions Science and Technology, 2018, 153: 10–17. DOI: 10.1016/j.coldregions.2018.04.014. [33] WANG P, XU J Y, LIU S, et al. A prediction model for the dynamic mechanical degradation of sedimentary rock after a long-term freeze-thaw weathering: Considering the strain-rate effect [J]. Cold Regions Science and Technology, 2016, 131: 16–23. DOI: 10.1016/j.coldregions.2016.08.003. [34] WANG P, XU J Y, FANG X Y, et al. Energy dissipation and damage evolution analyses for the dynamic compression failure process of red-sandstone after freeze-thaw cycles [J]. Engineering Geology, 2017, 221: 104–113. DOI: 10.1016/j.enggeo.2017.02.025. [35] ZHAI Y, MENG F D, LI Y B, et al. Research on dynamic compression failure characteristics and damage constitutive model of sandstone after freeze-thaw cycles [J]. Engineering Failure Analysis, 2022, 140: 106577. DOI: 10.1016/j.engfailanal.2022.106577. [36] HATHEWAY A W. The complete ISRM suggested methods for rock characterization, testing and monitoring; 1974—2006 [J]. Environmental and Engineering Geoscience, 2009, 15(1): 47–48. DOI: 10.2113/gseegeosci.15.1.47. [37] 中华人民共和国住房和城乡建设部. 工程岩体试验方法标准: GB/T 50266—2013 [S]. 北京: 中国计划出版社, 2013.Ministry of Housing and Urban-Rural Development of the People’s Republic of China. Standard for test methods of engineering rock mass: GB/T 50266—2013 [S]. Beijing: China Planning Press, 2013. [38] 申艳军, 杨更社, 荣腾龙, 等. 岩石冻融循环试验建议性方案探讨 [J]. 岩土工程学报, 2016, 38(10): 1775–1782. DOI: 10.11779/CJGE201610005.SHEN Y J, YANG G S, RONG T L, et al. Proposed scheme for freeze-thaw cycle tests on rock [J]. Chinese Journal of Geotechnical Engineering, 2016, 38(10): 1775–1782. DOI: 10.11779/CJGE201610005. [39] ZHANG R R, YANG Y, MA D D, et al. Experimental study on effect of freeze-thaw cycles on dynamic mode-Ⅰ fracture properties and microscopic damage evolution of sandstone [J]. Engineering Fracture Mechanics, 2023, 279: 109043. DOI: 10.1016/j.engfracmech.2023.109043. [40] 王宇, 翟成, 唐伟, 等. 循环冲击载荷作用下页岩动力学响应及能量耗散特征 [J]. 爆炸与冲击, 2023, 43(6): 063102. DOI: 10.11883/bzycj-2022-0248.WANG Y, ZHAI C, TANG W, et al. Dynamic response and energy dissipating characteristics of shale under cyclic impact loadings [J]. Explosion and Shock Waves, 2023, 43(6): 063102. DOI: 10.11883/bzycj-2022-0248. [41] 刘伟, 曾鹏, 闫雷, 等. 循环冲击下弱风化岩石力学特性与渗透率演化 [J]. 煤炭学报, 2021, 46(6): 1855–1863. DOI: 10.13225/j.cnki.jccs.2020.0066.LIU W, ZENG P, YAN L, et al. Mechanical properties and permeability evolution of weakly weathered rocks under cyclic impact [J]. Journal of China Coal Society, 2021, 46(6): 1855–1863. DOI: 10.13225/j.cnki.jccs.2020.0066. [42] 金解放, 李夕兵, 常军然, 等. 循环冲击作用下岩石应力应变曲线及应力波特性 [J]. 爆炸与冲击, 2013, 33(6): 613–619. DOI: 10.11883/1001-1455(2013)06-0613-07.JIN J F, LI X B, CHANG J R, et al. Stress-strain curve and stress wave characteristics of rock subjected to cyclic impact loadings [J]. Explosion and Shock Waves, 2013, 33(6): 613–619. DOI: 10.11883/1001-1455(2013)06-0613-07. [43] MA D D, XIANG H S, MA Q Y, et al. Dynamic damage constitutive model of frozen silty soil with prefabricated crack under uniaxial load [J]. Journal of Engineering Mechanics, 2021, 147(6): 104021033. DOI: 10.1061/(ASCE)EM.1943-7889.0001933. [44] 中华人民共和国国家质量监督检验检疫总局, 中国国家标准化管理委员会. 煤和岩石物理力学性质测定方法 第8部分: 煤和岩石变形参数测定方法: GB/T 23561.8—2009 [S]. 北京: 中国标准出版社, 2009.General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China, Standardization Administration of the People’s Republic of China. Methods for determining the physical and mechanical properties of coal and rock - Part 8: Methods for determining the deformation parameters of coal and rock: GB/T 23561.8—2009 [S]. Beijing: Standards Press of China, 2009. [45] ASTM. Standard test methods for compressive strength and elastic moduli of intact rock core specimens under varying states of stress and temperatures: ASTM D7012-14e1 [S]. Pennsylvania, USA: ASTM International, 2014. 期刊类型引用(5)
1. 王宇新,李晓杰,杨国俊,范述宁,王小红,闫鸿浩. 304L/Q235B大面积金属板爆炸焊接物质点法模拟分析. 爆炸与冲击. 2022(03): 150-159 .  本站查看
本站查看2. 曾翔宇,李晓杰,王小红,闫鸿浩,李科斌. 爆炸焊接波状界面的形成和发展. 稀有金属材料与工程. 2020(06): 1977-1983 .  百度学术
百度学术3. 陈沛,段卫东,曾国伟,金沐. 钛/钢爆炸焊接界面波形及缺陷组织的形成机理. 爆破. 2019(01): 126-132 .  百度学术
百度学术4. 曾翔宇,李晓杰,曹景祥,王小红,闫鸿浩. 材料强度对爆炸焊接结合界面的影响. 爆炸与冲击. 2019(05): 139-145 .  本站查看
本站查看5. 闫建文,雷方超,俞祺洋,羊科印. 炸药量对爆炸焊接界面波影响的数值模拟. 工程爆破. 2018(06): 10-17 .  百度学术
百度学术其他类型引用(15)
-








 下载:
下载:









 下载:
下载:






 百度学术
百度学术