Research progress of shock induced polarization effect in solid mediums
-
摘要: 冲击波在固体介质内传播时,内部电荷随冲击波作用向两极迁移形成电势差并对外输出电压/电流的极化效应称作冲击极化效应。针对晶体、金属、陶瓷以及聚合物等典型固体介质的冲击极化效应进行了系统梳理;总结了现阶段发展的冲击极化测试方法,分析了落锤/摆锤、SHPB、轻气炮以及炸药爆轰等加载方式诱发固体介质极化响应的差异;概述了有限元方法、分子动力学、近场动力学方法以及相场分析方法在固体介质冲击极化数值模拟领域的应用;围绕Allison理论、张裕恒理论、冲击挠曲电理论以及冲击波相关理论,总结了固体介质冲击极化的宏观唯象理论,并从固体介质微观结构、载流子输运模式、输运模型、迁移率以及态密度等方面说明了冲击极化的微观机理;分析了冲击极化效应在传感器、俘能器以及致动器等领域的应用前景,对固体介质冲击极化效应的发展趋势和需求进行了展望。Abstract: When shock waves propagate in solid mediums, the internal charge carriers migrate to the electrodes under the action of shock waves to form electric potential and output voltage/current -shock induced polarization (SIP) effect. The development of SIP effect has challenged the traditional understanding of physical response of solid medium. In this paper, the SIP effects of typical solid mediums such as crystals, metals, ceramics and polymers are systematically reviewed. The SIP test methods developed at present are also summarized, and the characteristics of SIP induced by different loading methods such as drop hammer/pendulum, SHPB, light gas gun and explosive detonation are analyzed. The applications of finite element method, molecular dynamics, peridynamic and phase field analysis in the numerical simulation of SIP of solid mediums are summarized. The macroscopic phenomenology theories of SIP of solid mediums are summarized based on Allison theory, Zhang Yuheng theory, shock flexoelectric theory and shock wave theory, and the microscopic mechanism of SIP is explained considering the microstructure of solid medium, carrier transport mode, transport model, mobility and state density. Furthermore, the application prospect of SIP effect in sensors, energy harvesters and actuators are analyzed, also, the development trend and demand of SIP in solid media are also prospected.
-
Key words:
- shock induced polarization /
- solid medium /
- charge carriers /
- shock wave
-
高超声速飞行器热防护系统(thermal protection system, TPS)的结构材料必须具有轻质、耐高温、环境稳定等特点, 以满足长航程安全服役的要求。近年来, 陶瓷基复合材料在飞行器大面积热防护领域应用广泛, 这类材料可以比传统的金属TPS减重50%, 同时可以延长使用寿命, 降低制造成本[1]。
基于化学气相沉积技术(chemical vapor infiltration, CVI)[2]制造的碳纤维增韧碳化硅陶瓷基复合材料(C/SiCS)以其低密度、抗氧化[3]、耐高温[4]等优异特性逐渐得到重视, 目前已经成功应用于飞行器的鼻锥和机翼前缘的防热结构中。然而, 与金属材料不同, 陶瓷基材料不具备产生塑性变形的能力, 材料的内部分层、基体裂纹、纤维断裂等破坏是其吸收冲击能量的主要方式, 这些损伤会降低材料的力学性能, 从而严重威胁材料的服役安全。因此, 为了飞行器内部结构及设备在服役过程中的安全性考虑, 这类结构材料在冲击载荷作用下的破坏行为及其数值预测一直是众多学者关注的热点。
目前, 针对平纹编织C/SiC(2D-C/SiC)复合材料的研究并不多见, 已有的相关文献多数仅限于对材料简单力学性能的实验研究[5-6]。杨扬等[7]基于电炮加载装置驱动Mylar飞片完成了冲击速度在3 400~9 300 m/s范围内2D-C/SiC材料的超高速撞击实验, 并对材料损伤演变和破坏特征进行了分析研究。然而, 关于材料在低速冲击载荷作用下的实验及其数值模拟目前尚未发现。
材料的各向异性特征使得在对其进行模拟计算时面临着巨大的挑战, R.A.Clegg等[8]提出的正交各向异性模型可以较好地描述复合材料在冲击载荷作用下的力学响应, 已经在Kevlar-Epoxy复合材料的冲击模拟中得到了成功的应用[9]。然而, 该模型重点关注了材料的非线性应变硬化行为, 在模拟C/SiC这种典型的脆性材料时无法得到理想的数值结果。本文中在已完成的球形弹丸低速冲击2D-C/SiC薄板的实验基础上, 引入材料的各向异性本构模型, 基于Autodyn商用软件对2D-C/SiC复合材料在钢球弹丸冲击作用下的数值模拟问题进行研究。
1. 2D-C/SiC材料低速冲击实验
基于空气炮加载装置对2D-C/SiC复合材料薄板在钢球撞击作用下的力学响应问题进行了实验研究。在实验过程中, 使用高速摄影技术记录了2D-C/SiC薄板受撞击后碎片云团的发展过程。
1.1 实验材料与计划
实验中选用的2D-C/SiC复合材料由西北工业大学超高温复合材料重点实验室研制。首先, T300碳纤维正交编织成碳布预制体, 在低压炉内基于CVI技术沉积6次SiC基体, 得到孔隙率约15%、体积分数约45%的2D-C/SiC材料, 而后基于CVI沉积2次SiC涂层。最终加工成型后, 切割为大小115 mm×115 mm、厚度3 mm、密度约2.11 g/cm3的正方形薄板作为目标靶板。实验中选用5、6 mm两种直径的普通钢球作为冲击弹丸。具体实验计划及主要结果列于表 1中, 表中D表示弹丸直径, vp表示弹丸的冲击速度, E表示弹丸的动能。
表 1 低速冲击实验计划Table 1. Low-speed impact experimental scheme实验编号 D/mm vp/(m·s-1) E/J 实验结果 1 5 79.0 1.61 未穿透 2 5 92.7 2.22 未穿透 3 5 98.5 2.51 未穿透 4 5 130.0 4.37 穿透 5 6 144.0 9.26 穿透 6 6 147.0 9.65 穿透 7 6 211.0 19.89 穿透 8 6 218.0 21.23 穿透 9 6 219.0 21.43 穿透 1.2 碎片云团结构
限于实验条件, 高速摄像机置于靶板斜后方位置, 图 1给出了试样8的高速摄影视频截图。由图 1可见, 2D-C/SiC碎片云团的发展过程大致分为2个阶段:(1)簇状飞散(图 1(a))。弹丸接触靶板时, 在作用点局部区域迅速发生纤维断裂、基体粉碎等多种形式破坏, 大量固体颗粒成簇状形式堆积飞出; (2)两区域形成(图 1(b)~(d))。簇状颗粒群运动发展, 逐渐在碎片云团中形成了界限清晰的两区域结构, 即柱状区和分散区。在柱状区内, 颗粒分布密度较高、颗粒尺寸较小、运动速度较快, 并沿轴线向前运动; 在分散区内, 颗粒分布较为稀疏、颗粒尺寸较大、运动速度较慢, 且沿一定的飞散角辐射飞出。2D-C/SiC碎片云结构与以往的金属材料及树脂基复合材料截然不同, 是其脆性特征的典型表现。在数值模拟中, 建立合理的本构模型对材料特有的力学响应特征进行模拟计算是本文研究的重点。
2. 耦合的各向异性材料模型
2.1 正交各向异性弹性模型
复合材料在冲击载荷作用下的力学响应过程十分复杂, 通常是诸如体积响应、材料各向异性等多种因素的耦合。在数值计算中, 不仅球应变会影响偏应力的计算, 偏应变同样会在球应力的计算中起作用, 通常需要面临求解状态方程和本构关系这两个高度耦合的子模型[10]。传统的计算方法是将这两个子模型独立计算, 然而这样的过程较为繁琐, C.E.Anderson等[11]首先提出了一种适用于求解各向异性材料中多重响应耦合问题的计算方法。本文中也以此方法为基础, 建立如下的计算模型。
2D-C/SiC是典型的正交各向异性复合材料。基于塑性力学, 全应变可分解为与体积变化相关的球应变ε0和与形状变化相关的偏应变
 之和。此时, 2D-C/SiC的本构关系可用如下全应力应变关系表述为:
之和。此时, 2D-C/SiC的本构关系可用如下全应力应变关系表述为:
[σ11σ22σ33σ23σ31σ12]=[C11C12C13000C12C22C23000C13C23C33000000C44000000C55000000C66][ε0+εd11−1ε0+εd22ε0+εd33ε23ε31ε12] 由于各向同性压力P是3个正应力的平均值的相反数, 因此由上式可以推导得出:
P=−13(C11+C22+C33+2C12+2C13+2C23)ε0−3∑i=113[C1i+C2i+C3i]εdii (1) 从(1)式中可以清晰地看出, 与各向同性材料中球应力仅依赖于体积应变不同, 各向异性材料中偏应变也会在一定程度上影响球应力的计算。其中ε0一项的系数可称为材料的等效体积模量, 记为A。
2.2 正交各向异性失效模型
失效模型主要包括失效初始化和后失效响应两个阶段。在失效初始化阶段, 采用最大应力判断准则, 即预先设置材料在3个拉伸方向和3个剪切方向的失效应力, 当任一方向的应力值达到失效应力时, 材料即在该方向失效, 进入后失效响应阶段。
对于材料的后失效响应, 需要在计算开始前按照如下准则进行相关设置:当材料在任一方向进入后失效阶段时, 材料在该方向的承载能力瞬间丧失, 但在其他方向的承载能力保持不变; 同时, 材料在3个剪切方向的承载能力下降[8]。例如, 假设1方向为薄板厚度方向, 2、3方向为面内的2个正交方向。那么, 当1方向拉伸应力大于拉伸失效应力或12(或13)方向剪切应力大于剪切失效应力时, 材料会发生分层破坏。此时, 材料在1方向应力立即置为0, 不再具有承载能力, 但在面内的2个方向承载能力不变; 同时由于分层破坏的发生, 材料在剪切方向的承载能力也将有所下降, 需要对刚度矩阵中的剪切刚度系数进行退化, 可对材料的刚度矩阵作如下修正(α为刚度退化因子):
[0σ22σ33σ23σ31σ12]=[0000000C22C230000C23C33000000αC44000000αC55000000αC66][ε11ε22ε33ε23ε31ε12] 表 2 正交弹性模型材料参数Table 2. Parameters of orthotropic elastic model刚度系数 体积模量 C11/GPa C22/GPa C33/GPa C12/GPa C23/GPa C31/GPa G12/GPa G23/GPa G31/GPa A/GPa 19 120.5 120.5 7.8 20 7.8 8.8 23 8.8 36.8 表 3 正交失效模型材料参数Table 3. Parameters of orthotropic failure model失效初始化 σfail-11/MPa σfail-22/MPa σfail-33/MPa σfail-12/MPa σfail-23/MPa σfail-31/MPa 50 455 455 40 165 40 后失效响应 刚度退化因子 失效模式 0.2 11失效
11方向拉伸
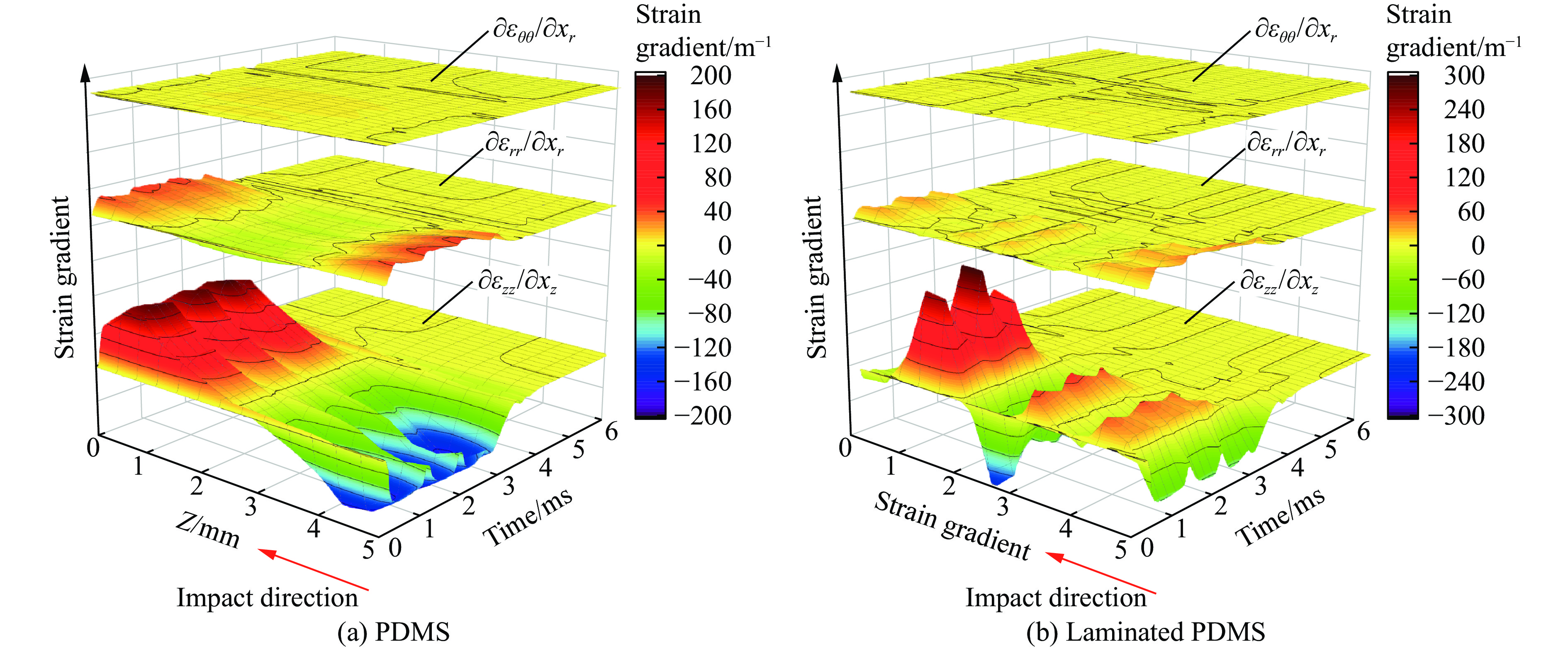
应力置022失效
22方向拉伸
应力置033失效
33方向拉伸
应力置012失效
11方向拉伸
应力置023失效
11方向拉伸
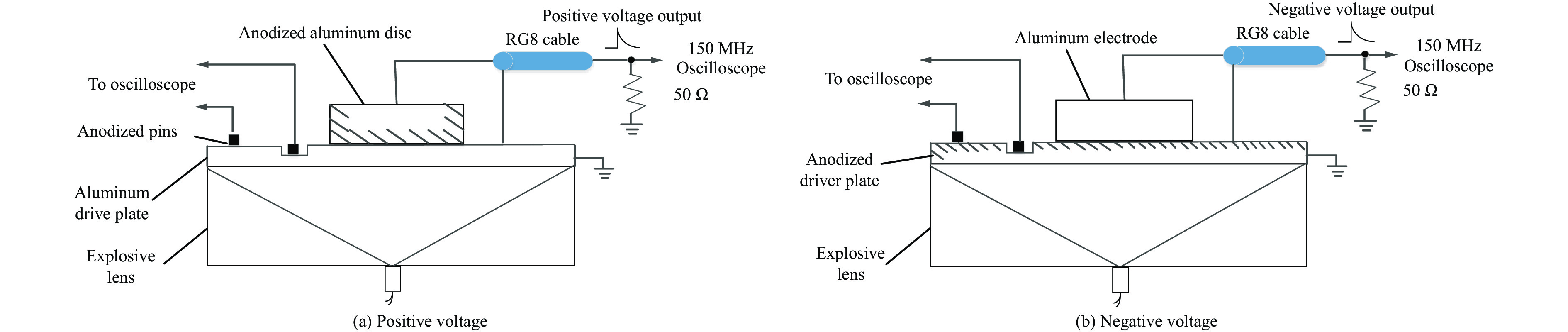
应力置031失效
11方向拉伸
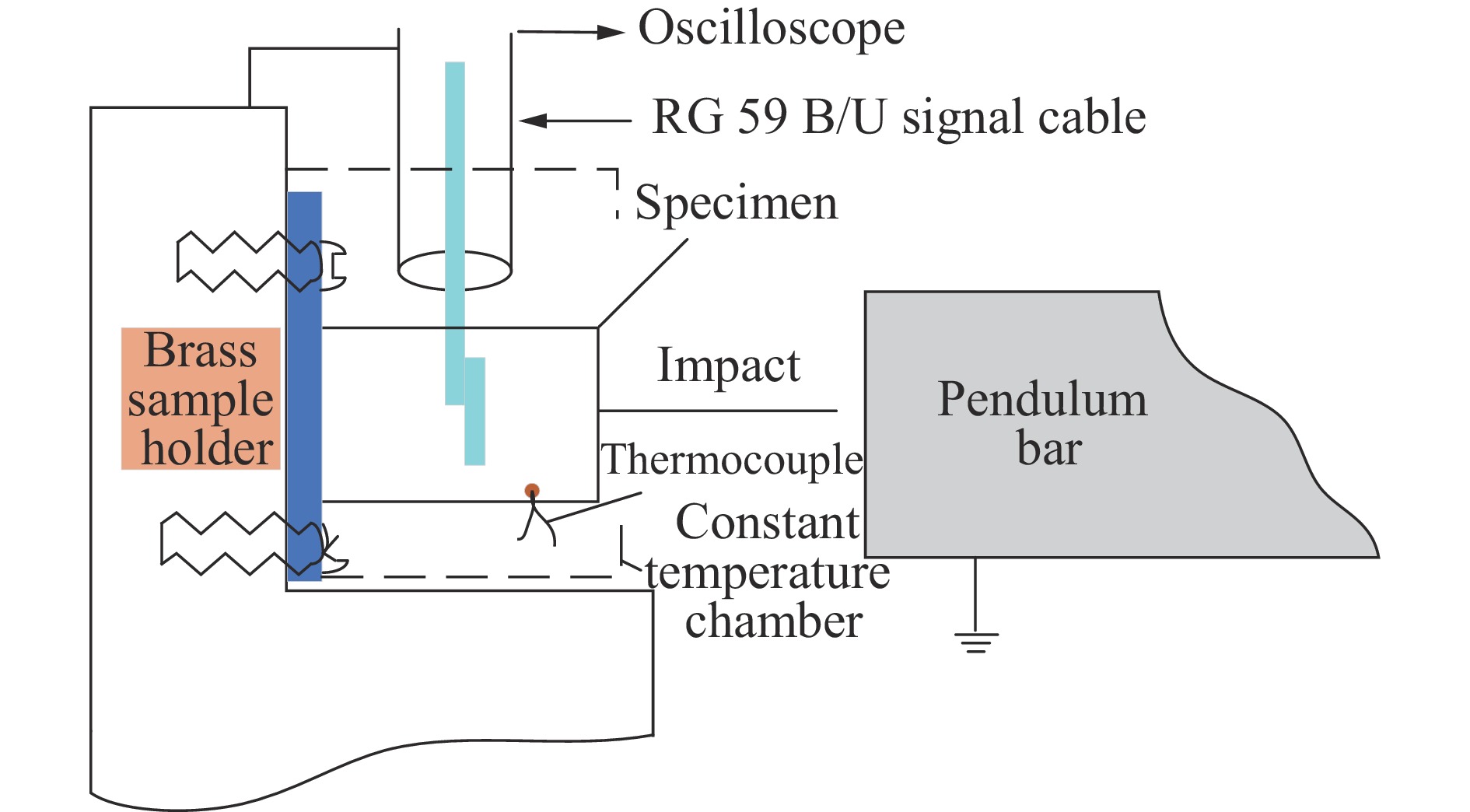
应力置03. 数值模拟及分析
3.1 计算模型
由于正撞击的对称性, 基于Autodyn软件建立如图 2所示的二维半模型计算。2D-C/SiC靶板选用SPH求解器; 钢弹丸steel 4340基于FEM求解器计算; 弹丸与靶板之间设置接触, 接触间距0.01 mm; 在靶板边缘3 mm×4 mm范围内施加全局固定边界, 即限定此区域内的粒子在x和y方向速度恒为0。
下面通过对碎片云结构和典型破坏形式等实验结果的数值验证, 证明该模型对描述2D-C/SiC材料在冲击载荷作用下力学响应的合理性和可行性。
3.2 数值合理性的实验对比
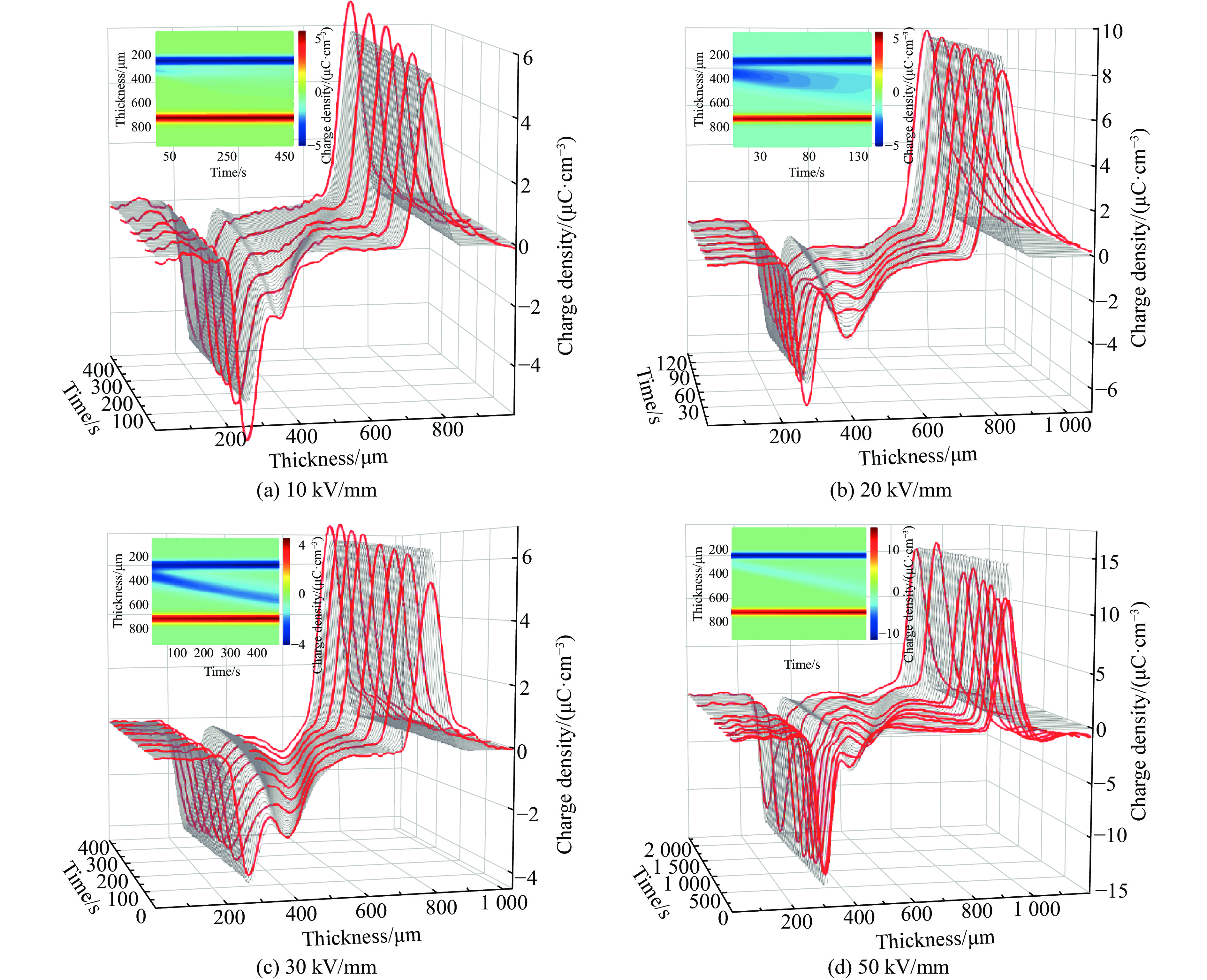
3.2.1碎片云团结构的数值验证
图 3 (b)、(c)分别给出了试样8的数值模拟结果与对应的速度矢量图。通过对比可以看出, 实验结果和数值模拟结果吻合较好, 具有以下相似特征:(1)碎片云团均呈现明显的双区域结构, 即均存在沿冲击轴线方向的柱状区和轴线两侧的飞散区, 这是2D-C/SiC在冲击载荷作用下的特殊响应特征; (2)从速度矢量图可以看出, 柱状区粒子运动方向均为沿轴线方向; 而飞散区内的粒子速度方向与轴线有大小不同的夹角。这与实验结果是完全一致的, 表明该模型可以较好地表征2D-C/SiC在弹丸撞击下碎片云团的结构特征。
3.2.2 B扫描结果的数值验证
B扫描结果显示的实际上是试样内部损伤的二维截面图。图 4(a)给出试样8的B扫结果, 其中虚线区域的黄色部分表示试样; 图 4(b)~(c)给出工况8靶板扩展稳定后内部损伤的模拟计算结果。
从图 4看出:(1)数值模拟与B扫描显示的穿孔形状十分相似, 穿孔直径近似为弹丸直径(6 mm); (2)从B扫结果看, 靶材背面显示黄色较正面偏淡, 这是由于材料在靠近背面的区域损伤严重, 导致超声波信号无法完全被探头接收。相似的现象可以在数值模拟中显示得更加清晰, 图 4(c)给出了靶材穿孔区域的局部放大图。由图可见, 与正面相比, 靶材背面存在明显的材料分层与剥落损伤, 这与实验结果比较吻合。上述相似性也表明该模型可以较好地表征2D-C/SiC在弹丸撞击下的破坏特征。
3.2.3碎片云轴向速度的数值验证
碎片云的轴向速度是表征碎片云团发展状态的重要指标。实验中, 在靶板表面标记了刻度, 据此可以根据高速摄影记录计算出碎片云团柱状区前端的运动速度, 而将此速度视为碎片云轴向运动速度vaxis。同样, 在数值模拟中, 取靶板自由面与冲击轴线的交点为特征点近似柱状区端点。选取靶板穿透工况中冲击速度相差较大的4种情况进行验证, 图 5给出了不同冲击速度vaxis下随时间的变化历程。
从图 5可以看出, 在弹丸接触靶板后的短时间内, 特征点的轴向速度迅速增加并逐渐趋于稳定。将特征点最终的稳定速度视为对vaxis的近似, 图 6给出了实验数据与数值计算的对比结果。可以看出, 二者的吻合度较好, 并且随着冲击速度的增高, 相对误差逐渐减小, 其中冲击速度219 m/s时二者的相对误差仅为2.25%, 表明该模型可以较好地表征2D-C/SiC在冲击载荷作用下碎片云团的速度特性。
4. 极限侵彻深度预测模型
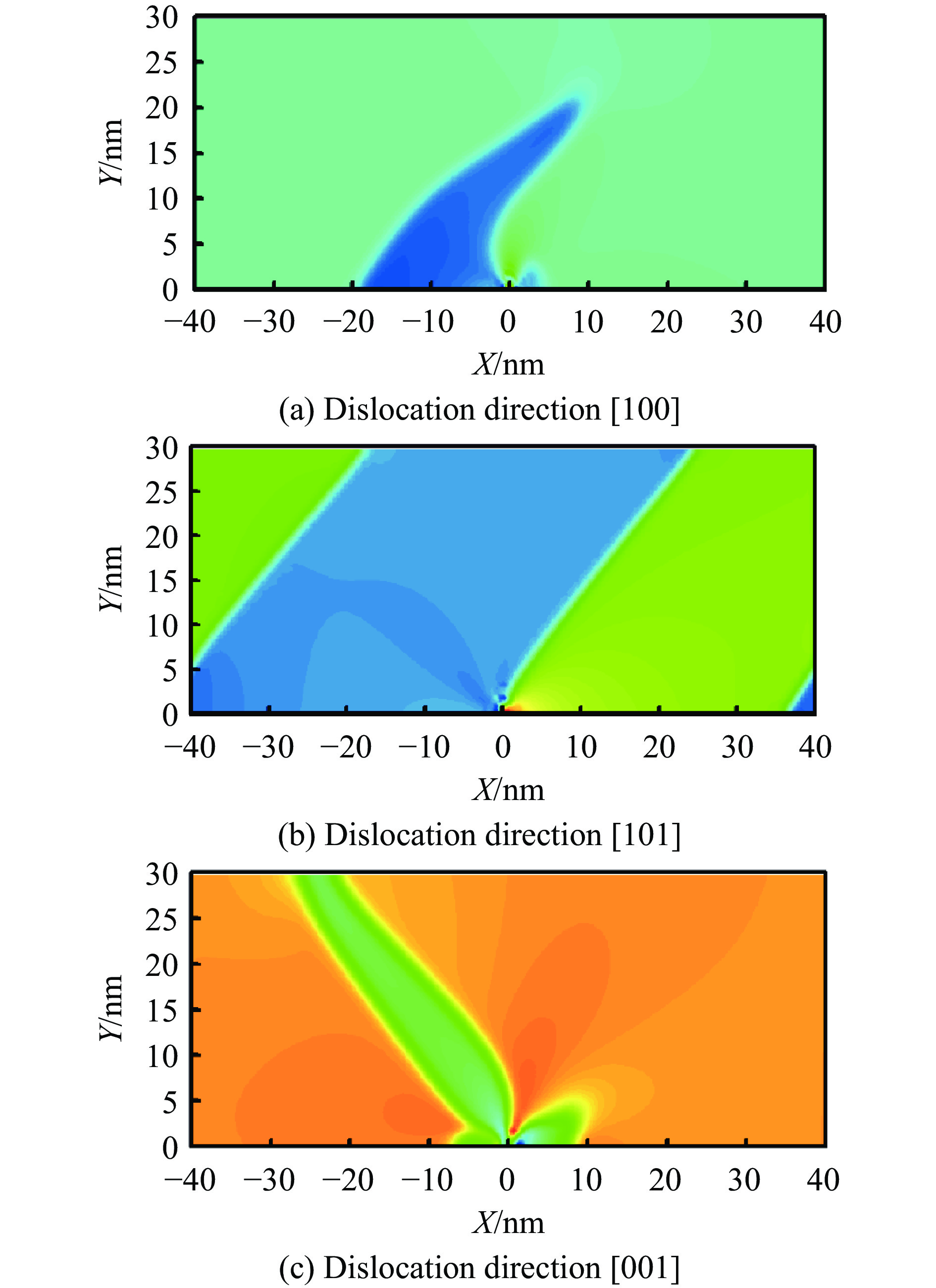
尽管碎片云团中柱状区内的粒子运动速度较高, 但由于颗粒质量较小, 对机体的损伤程度有限。碎片云团中对机体威胁较大的仍是穿透靶板的弹丸, 因此可以通过对弹丸剩余速度的分析研究, 建立合理有效的预测公式评估C/SiC靶板的抗冲击性能。以上通过3方面对比验证了文中使用的材料模型及相关参数的正确性和合理性, 下面将基于此模型对弹丸侵彻下C/SiC靶板的抗冲击特性进行预估。
考虑同一弹丸(直径6 mm)以不同冲击速度对不同厚度靶板进行撞击。设置靶板厚度δ为1、2、3、4、5 mm, 弹丸冲击速度vi为50、100、150、200、250、300、350、400 m/s, 计算得到弹丸剩余速度变化规律如图 7所示。可以看出:(1)对同一厚度的靶板, 剩余速度随着冲击速度的减小不断减小; 而对相同冲击速度, 剩余速度会随着靶板厚度的减小而不断增加; (2)剩余速度在速度较低靶板较厚时出现了负值, 这是由于弹丸在这些工况下未能穿透靶板, 出现回弹现象造成的。
评估材料抗冲击性能比较关心的重要参数是靶板的极限穿透速度, 即上述曲面与剩余速度为0的平面的交线。然而, 如果固定靶板厚度分别观察剩余速度随冲击速度的变化趋势(图 8), 可以看出在临界穿透状态附近(图中虚线), 剩余速度变化曲线斜率陡然增高, 说明在临界穿透状态附近剩余速度变化较剧烈; 而当冲击速度高于极限穿透速度之后, 弹丸剩余速度变化基本稳定, 呈准线性增长趋势。因此, 如果直接对剩余速度曲面进行拟合来求解极限穿透速度, 会导致在临界穿透状态附近的计算误差较大。
为了解决上述问题, 采用分步拟合方法:(1)假设靶板无限厚, 首先固定冲击速度, 分别拟合得到弹丸剩余速度随靶板厚度的关系, 进而求解在此冲击速度下弹丸的极限侵彻深度; (2)其次基于Poncelet型侵彻深度预测模型[12], 利用得到的极限侵彻深度T与弹丸冲击速度vi进行拟合, 得到如下关系:
T=0.0005362ln(1+0.756v2i) 此时, 便可以在已知靶板厚度的情况下, 通过反解上式预测弹丸的极限穿透速度。同时, 在工程设计中, 如果工程人员已经根据飞行器的服役环境预估出外来冲击物的撞击速度, 那么同样可以利用上式预估弹丸的极限侵彻深度, 也就可以为防护板厚度参数的确定提供一定的参考。
5. 结论
基于Autodyn软件选取正交各向异性材料模型, 推导了相关的材料参数, 并从碎片云团结构、B扫描内部损伤形貌和碎片云轴向速度三个方面对比了实验与模拟结果, 表明参数的合理性和正确性, 较好地描述了2D-C/SiC在钢球弹丸撞击下的主要损伤特征和力学特性。在此基础上, 基于数值模拟计算结果推导得到了钢球弹丸撞击2D-C/SiC材料的极限侵彻深度预测公式, 这可为TPS结构设计提供一定的工程参考价值。
-
表 1 典型动态加载技术的特点
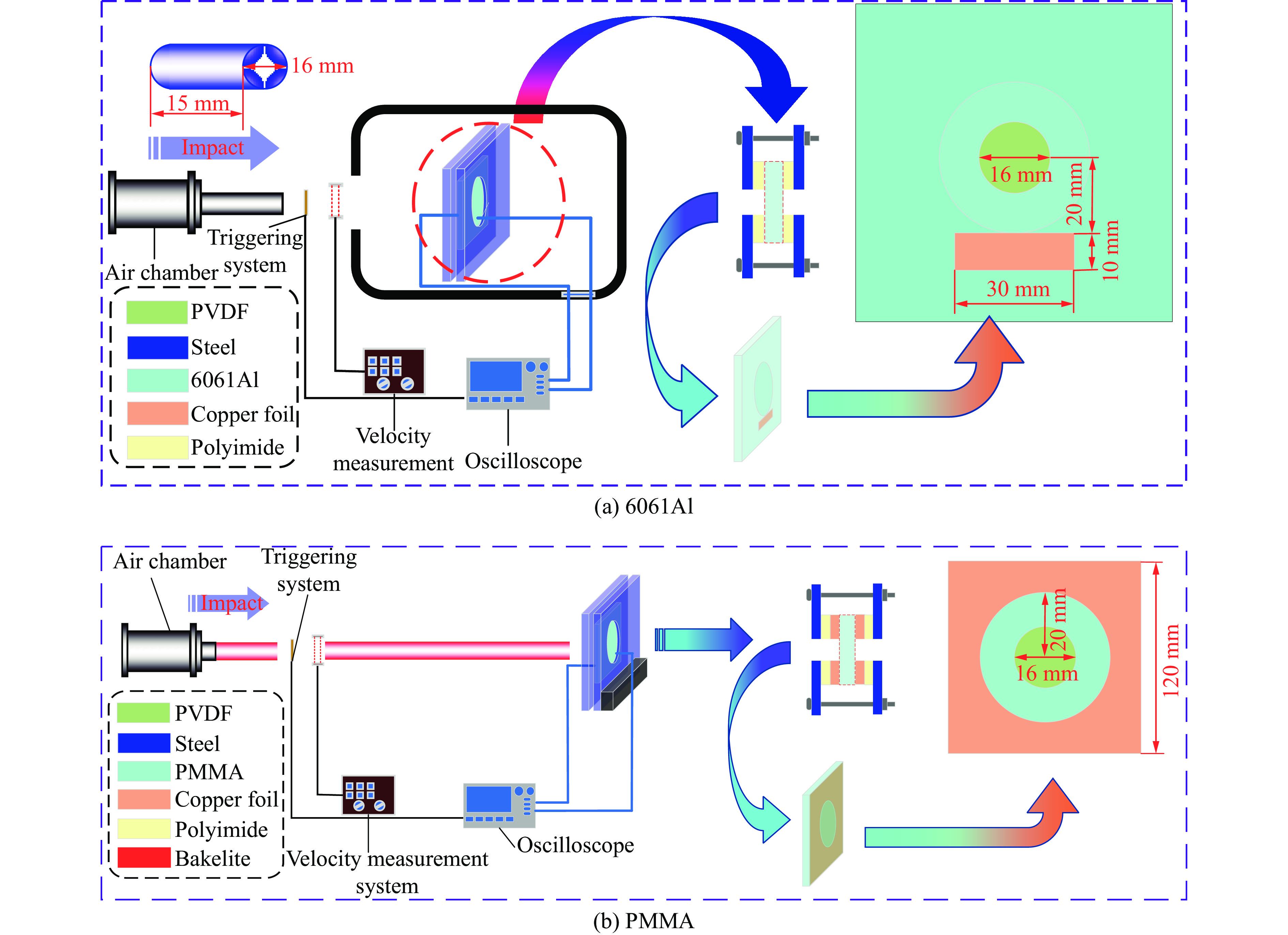
Table 1. Characteristics of typical dynamic loading technology
实验方法 应变率/s−1 响应特性 主要应用 落锤/摆锤 1~102 弹塑性变形,伴随损伤破坏 测定抗冲击强度、变形与吸能 SHPB 102~104 弹塑相变,黏性与应变率效应明显 确定动态本构模型 轻气炮 104~106 出现流体性态,考虑密度与可压缩性 确定状态方程 爆轰 >106 呈流体力学状态,伴有熔融与汽化 可计算爆轰波,确定状态方程 -
[1] LINDE R K, MURRI W J, DORAN D G. Shock-induced electrical polarization of alkali halides [J]. Journal of Applied Physics, 1966, 37(7): 2527–2532. DOI: 10.1063/1.1782079. [2] NABATOV S S, YAKUSHEV V V, DREMIN A N. Shock-induced electrical polarization of nitroglycerine [J]. Combustion, Explosion and Shock Waves, 1976, 12(2): 222–226. DOI: 10.1007/bf00744892. [3] KURTO A P, ANTIPENKO A G, DREMIN A N, et al. Shock-wave-induced electrical polarization of polyvinyl chloride plastic [J]. Combustion, Explosion and Shock Waves, 1983, 19(5): 682–685. DOI: 10.1007/bf00750459. [4] ANTIPENKO A G, DREMIN A N, NABATOV S S, et al. Electrical effects in shock compression and detonation of liquid high explosives [J]. Combustion, Explosion and Shock Waves, 1975, 11(3): 371–375. DOI: 10.1007/bf00740546. [5] IVANOV A G, MINEEV V N, NOVITSKII E Z, et al. Electrical effects associated with shock loading [J]. Combustion, Explosion and Shock Waves, 1969, 5(4): 356–360. DOI: 10.1007/bf00742077. [6] ALLISON F E. Shock-induced polarization in plastics. Ⅰ. Theory [J]. Journal of Applied Physics, 1965, 36(7): 2111–2113. DOI: 10.1063/1.1714428. [7] TAGANTSEV A K. Pyroelectric, piezoelectric, flexoelectric, and thermal polarization effects in ionic crystals [J]. Soviet Physics Uspekhi, 1987, 30(7): 588–603. DOI: 10.1070/pu1987v030n07abeh002926. [8] CHEN Z, HUANG K M. Using the oscillating dipoles model to study the electromagnetic radiation induced by fracture of rocks [J]. Progress in Electromagnetics Research M, 2010, 14: 221–231. DOI: 10.2528/PIERM10041802. [9] MINEEV V N, IVANOV A G. Electromotive force produced by shock compression of a substance [J]. Soviet Physics Uspekhi, 1976, 19(5): 400. DOI: 10.1070/PU1976v019n05ABEH005260. [10] TYUNYAEV Y N, MINEEV V N, IVANOV A G, et al. Connection between shock polarization of ionic crystals and the lattice characteristics [J]. Soviet Physics JETP, 1969, 29(1): 98–100. [11] COLEBURN N L, FORBES J W, JONES H D. Electrical measurements in silicon under shock-wave compression [J]. Journal of Applied Physics, 1972, 43(12): 5007–5012. DOI: 10.1063/1.1661061. [12] MISRA A. A physical model for the stress-induced electromagnetic effect in metals [J]. Applied Physics, 1978, 16(2): 195–199. DOI: 10.1007/bf00930387. [13] TANG E L, LIU P, WANG R Z, et al. Polarization response characteristics of 6061Al under impact loading [J]. Journal of Materials Science: Materials in Electronics, 2023, 34(24): 1732. DOI: 10.1007/s10854-023-11135-w. [14] TANG E L, WANG D B, LI L, et al. Polarization response characteristics of 6061Al and PMMA sheets under impact load [J]. International Journal of Impact Engineering, 2023, 178: 104632. DOI: 10.1016/j.ijimpeng.2023.104632. [15] SETCHELL R E. Recent progress in understanding the shock response of ferroelectric ceramics [J]. AIP Conference Proceedings, 2002, 620(1): 191–196. DOI: 10.1063/1.1483513. [16] HALPIN W J. Current from a shock-loaded short-circuited ferroelectric ceramic disk [J]. Journal of Applied Physics, 1966, 37(1): 153–163. DOI: 10.1063/1.1707798. [17] LYSNE P C, PERCIVAL C M. Electric energy generation by shock compression of ferroelectric ceramics: normal-mode response of PZT 95/5 [J]. Journal of Applied Physics, 1975, 46(4): 1519–1525. DOI: 10.1063/1.321803. [18] LYSNE P C, PERCIVAL C M. Analysis of shock-wave-actuated ferroelectric power supplies [J]. Ferroelectrics, 1976, 10(1): 129–133. DOI: 10.1080/00150197608241963. [19] LYSNE P C. Shock-induced polarization of a ferroelectric ceramic [J]. Journal of Applied Physics, 1977, 48(3): 1024–1031. DOI: 10.1063/1.323802. [20] FURNISH M D, CHHABILDAS L C, SETCHELL R E, et al. Dynamic electromechanical characterization of axially poled PZT 95/5 [J]. AIP Conference Proceedings, 2000, 505(1): 975–978. DOI: 10.1063/1.1303631. [21] MOCK JR W, HOLT W H. Analysis of the ideal response of shock-depoled ferroelectric ceramics [J]. Ferroelectrics, 1980, 23(1): 39–45. DOI: 10.1080/00150198008224809. [22] 贺元吉, 张亚洲, 李传胪, 等. 冲击波加载下PZT 95/5铁电陶瓷电响应的数值模拟 [J]. 高压物理学报, 2000, 14(3): 189–194. DOI: 10.11858/gywlxb.2000.03.006.HE Y J, ZHANG Y Z, LI C L, et al. The numerical simulation of electric response of PZT 95/5 ferroelectric ceramics subjected to shock loading [J]. Chinese Journal of High Pressure Physics, 2000, 14(3): 189–194. DOI: 10.11858/gywlxb.2000.03.006. [23] MASHIMO T, TODA K, NAGAYAMA K, et al. Electrical response of BaTiO3 ceramics to the shock-induced ferroelectric-paraelectric transition [J]. Journal of Applied Physics, 1986, 59(3): 748–756. DOI: 10.1063/1.336595. [24] 刘高旻, 杜金梅, 刘雨生, 等. PZT 95/5铁电陶瓷的冲击压缩Hugoniot特性研究 [J]. 高压物理学报, 2008, 22(1): 30–34. DOI: 10.11858/gywlxb.2008.01.007.LIU G M, DU J M, LIU Y S, et al. Shock wave compression of PZT 95/5 ferroelectric ceramic [J]. Chinese Journal of High Pressure Physics, 2008, 22(1): 30–34. DOI: 10.11858/gywlxb.2008.01.007. [25] PENG P, NIE H C, WANG G S, et al. Shock-driven depolarization behavior in BNT-based lead-free ceramics [J]. Applied Physics Letters, 2018, 113(8): 082901. DOI: 10.1063/1.5045392. [26] GAO Z P, PENG W, CHEN B, et al. Giant power output in lead-free ferroelectrics by shock-induced phase transition [J]. Physical Review Materials, 2019, 3(3): 035401. DOI: 10.1103/physrevmaterials.3.035401. [27] TANG E L, LENG B Y, HAN Y F, et al. Influence of temperature on electromechanical responses of PZT-5H and output energy under shock loading [J]. Materials Chemistry and Physics, 2022, 276: 125309. DOI: 10.1016/j.matchemphys.2021.125309. [28] HAUVER G E. Erratum: Shock-induced polarization in plastics. Ⅱ. Experimental study of plexiglas and polystyrene [J]. Journal of Applied Physics, 1965, 36(7): 2113–2118. DOI: 10.1063/1.1714429. [29] ANTINENKO A G, NABATOV S S, YAKUSHEV V V. Electrical polarization of an insulator with fast relaxation on shock compression [J]. Combustion, Explosion and Shock Waves, 1975, 11(3): 391–394. DOI: 10.1007/bf00740549. [30] GONCHAROV A I, SOLOVIEV S P. Shock-induced polarization of materials [J]. Combustion, Explosion and Shock Waves, 2004, 40(6): 658–662. DOI: 10.1023/b:cesw.0000048267.04438.cc. [31] LYSNE P C. Dielectric properties of shock-wave-compressed PMMA and an alumina-loaded epoxy [J]. Journal of Applied Physics, 1978, 49(7): 4186–4190. DOI: 10.1063/1.325330. [32] TOWNSEND D, BOURNE N K. Measurements of the conductivity of shocked polymethylmethacrylate [J]. AIP Conference Proceedings, 2002, 620(1): 1267–1272. DOI: 10.1063/1.1483770. [33] CHANG M Z, LI K, LIU C, et al. Coupling effect of impact and in-layer voltage on flexoelectricity of PDMS laminated structures [J]. Polymer Testing, 2022, 115: 107741. DOI: 10.1016/j.polymertesting.2022.107741. [34] IVANOV A G, TYUNYAEV Y N, MINEEV V N, et al. Conductivity transition zone and polarization of TNT behind the shock front [J]. Combustion, Explosion, and Shock Waves, 1969, 5(3): 256–262. DOI: 10.1007/bf00748604. [35] 张阳. 乳化炸药微观结构变化对电导率影响的探究 [D]. 淮南: 安徽理工大学, 2015. DOI: 10.7666/d.Y2767930.ZHANG Y. Study on the influence of microstructure change of emulsion explosive on conductivity [D]. Huainan: Anhui University of Science and Technology, 2015. DOI: 10.7666/d.Y2767930. [36] ANTIPENKO A G, DREMIN A N, YAKUSHEV V V. Electrical polarization with initiation of the detonation of homogeneous explosives by a shock wave [J]. Combustion, Explosion, and Shock Waves, 1978, 14(6): 786–790. DOI: 10.1007/bf00786113. [37] 蒋治海, 龙新平, 何碧, 等. TNT和RHT-906炸药起爆过程的电导率研究 [J]. 含能材料, 2007, 15(2): 169–171. DOI: 10.3969/j.issn.1006-9941.2007.02.022.JIANG Z H, LONG X P, HE B, et al. Electrical conductivity of TNT and RHT-906 explosives in initiation process [J]. Chinese Journal of Energetic Materials, 2007, 15(2): 169–171. DOI: 10.3969/j.issn.1006-9941.2007.02.022. [38] HWANG M Y, KANG L H. Characteristics and fabrication of piezoelectric GFRP using smart resin prepreg for detecting impact signals [J]. Composites Science and Technology, 2018, 167: 224–233. DOI: 10.1016/j.compscitech.2018.08.002. [39] CUTHRELL R E. Epoxy polymers. IV. Impact-induced voltage generation [J]. Journal of Applied Polymer Science, 1968, 12(7): 1515–1530. DOI: 10.1002/app.1968.070120703. [40] HU T T, WANG X Z, YAN Y B, et al. Influence of impact velocity on flexoelectric effect [J]. Results in Physics, 2019, 15: 102812. DOI: 10.1016/j.rinp.2019.102812. [41] CHAMPION A R. Electrical response of anodized aluminum layers to shock-wave compression [J]. Journal of Applied Physics, 1969, 40(9): 3766–3771. DOI: 10.1063/1.1658269. [42] 甄广平, 陈福梅. 层状有机玻璃PMMA的冲击极化效应与应用 [J]. 北京理工大学学报, 1992, 12(2): 16–24.ZHEN G P, CHEN F M. Shock induced polarization of laminate PMMA and its application [J]. Journal of Beijing Institute of Technology, 1992, 12(2): 16–24. [43] 张春生. 一种改进的冲击极化实验装置 [J]. 爆炸与冲击, 1986, 6(1): 67–71.ZHANG C S. An improved experimental setup for shock-induced polarization [J]. Explosion and Shock Waves, 1986, 6(1): 67–71. [44] SAXENA K, HIRONAKA Y, HIRAI H, et al. Shock-induced polarization in normal-hexane [J]. Applied Physics Letters, 1996, 68(7): 920–922. DOI: 10.1063/1.116231. [45] 潘锴. 针对PFM技术的铁电材料纳米尺度力电耦合分析 [D]. 湘潭: 湘潭大学, 2014. DOI: 10.7666/d.D583513.PAN K. Analyzing nanoscale electromechanical coupling of ferroelectrics as probed by PFM [D]. Xiangtan: Xiangtan University, 2014. DOI: 10.7666/d.D583513. [46] KAJIMOTO K, ARAKI K, USAMI Y, et al. Visualization of charge migration in conductive polymers via time-resolved electrostatic force microscopy [J]. The Journal of Physical Chemistry A, 2020, 124(25): 5063–5070. DOI: 10.1021/acs.jpca.9b12017. [47] CODONY D, GUPTA P, MARCO O, et al. Modeling flexoelectricity in soft dielectrics at finite deformation [J]. Journal of the Mechanics and Physics of Solids, 2021, 146: 104182. DOI: 10.1016/j.jmps.2020.104182. [48] MENG J, ZHANG Y W, HOLÉ S, et al. Charge mobility retrieval approach from apparent charge packet movements based on the negative differential resistance theory [J]. Scientific Reports, 2018, 8(1): 5928. DOI: 10.1038/s41598-018-24327-w. [49] HONG J W, CATALAN G, SCOTT J F, et al. The flexoelectricity of barium and strontium titanates from first principles [J]. Journal of Physics: Condensed Matter, 2010, 22(11): 112201. DOI: 10.1088/0953-8984/22/11/112201. [50] HONG J W, VANDERBILT D. First-principles theory of frozen-ion flexoelectricity [J]. Physical Review B, 2011, 84(18): 180101. DOI: 10.1103/PhysRevB.84.180101. [51] HONG J W, VANDERBILT D. First-principles theory and calculation of flexoelectricity [J]. Physical Review B, 2013, 88(17): 174107. DOI: 10.1103/PhysRevB.88.174107. [52] 李贝贝. 金属Pt和Pd的AMOEBA极化力场的构建 [D]. 大连: 辽宁师范大学, 2013. DOI: 10.7666/d.Y2376309.LI B B. A building for AMOEBA polarization force fields of metals Pt and Pd [D]. Dalian: Liaoning Normal University, 2013. DOI: 10.7666/d.Y2376309. [53] PONOMAREVA I, TAGANTSEV A K, BELLAICHE L. Finite-temperature flexoelectricity in ferroelectric thin films from first principles [J]. Physical Review B, 2012, 85(10): 104101. DOI: 10.1103/PhysRevB.85.104101. [54] ROYO M, STENGEL M. First-principles theory of spatial dispersion: dynamical quadrupoles and flexoelectricity [J]. Physical Review X, 2019, 9(2): 021050. DOI: 10.1103/physrevx.9.021050. [55] DREYER C E, STENGEL M, VANDERBILT D. Current-density implementation for calculating flexoelectric coefficients [J]. Physical Review B, 2018, 98(7): 075153. DOI: 10.1103/PhysRevB.98.075153. [56] STENGEL M. Surface control of flexoelectricity [J]. Physical Review B, 2014, 90(20): 201112. DOI: 10.1103/physrevb.90.201112. [57] HE B, JAVVAJI B, ZHUANG X Y. Size dependent flexoelectric and mechanical properties of barium titanate nanobelt: a molecular dynamics study [J]. Physica B: Condensed Matter, 2018, 545: 527–535. DOI: 10.1016/j.physb.2018.01.031. [58] TIAN X B, YANG X H, CAO W Z. Atomistic simulation of strain-induced domain evolution in a uniaxially compressed BaTiO3 single-crystal nanofilm [J]. Journal of Electronic Materials, 2013, 42(8): 2504–2509. DOI: 10.1007/s11664-013-2597-9. [59] 孙素涛. 共轭聚合物中荷电载流子动力学性质的频谱研究 [D]. 石家庄: 河北师范大学, 2020. DOI: 10.27110/d.cnki.ghsfu.2020.000586.SUN S T. Spectral analysis on charged carrier dynamics in conjugated polymers [D]. Shijiazhuang: Hebei Normal University, 2020. DOI: 10.27110/d.cnki.ghsfu.2020.000586. [60] SKRYL Y, KUKLJA M M. Numerical simulation of diffusion of electrons and holes in shocked silicon [J]. AIP Conference Proceedings, 2004, 706(1): 267–270. DOI: 10.1063/1.1780232. [61] SKRYL Y, BELAK A A, KUKLJA M M. Numerical simulation of shock induced polarization in binary electrolytes [J]. AIP Conference Proceedings, 2006, 845(1): 355–358. DOI: 10.1063/1.2263336. [62] 郭莉莉. 微观结构对铁电电畴影响机理的相场理论研究 [D]. 湘潭: 湘潭大学, 2016.GUO L L. Phase field simulation of the impact mechianism of microstructure on domain structure of ferroelectric [D]. Xiangtan: Xiangtan University, 2016. [63] CAO Y, MOROZOVSKA A, KALININ S V. Pressure-induced switching in ferroelectrics: phase-field modeling, electrochemistry, flexoelectric effect, and bulk vacancy dynamics [J]. Physical Review B, 2017, 96(18): 184109. DOI: 10.1103/physrevb.96.184109. [64] ZEL’DOVICH Y B. EMF produced by a shockwave moving in a dielectric [J]. Soviet Physics JETP, 1968, 26(1): 159–162. [65] DREMIN A N, ROZANOV O K, YAKUSHEV V V. Some problems of the polarization of dielectrics in shock waves [J]. Journal of Applied Mechanics and Technical Physics, 1968, 9(5): 562–565. DOI: 10.1007/bf02614757. [66] 冷冰玉. 6061Al与PMMA薄板的冲击极化响应规律研究 [D]. 沈阳: 沈阳理工大学, 2021. DOI: 10.27323/d.cnki.gsgyc.2022.000123.LENG B Y. Study on shock induced polarization response of 6061Al and PMMA thin plate [D]. Shenyang: Shenyang Ligong University, 2021. DOI: 10.27323/d.cnki.gsgyc.2022.000123. [67] DE ICAZA-HERRERA M. Phenomenological theory of shock-induced polarization. Ⅰ [J]. Journal of Applied Physics, 1983, 54(5): 2352–2359. DOI: 10.1063/1.332347. [68] DE ICAZA-HERRERA M. Phenomenological theory of shock-induced polarization. Ⅱ. Mathematical treatment of the oscillogram [J]. Journal of Applied Physics, 1983, 54(5): 2360–2365. DOI: 10.1063/1.332348. [69] HUANG Y J. Yuheng Zhang effect: Strain-induced electric effect in metals [J]. Journal of Materials Sciences and Applications, 2019, 5(3): 58–62. [70] HUANG Y. Strain-induced electric effects in condensed matters [J]. Journal of Materials Sciences and Application, 2019, 5(3): 44–57. [71] HADJESFANDIARI A R. Size-dependent piezoelectricity [J]. International Journal of Solids and Structures, 2013, 50(18): 2781–2791. DOI: 10.1016/j.ijsolstr.2013.04.020. [72] GARCÍA N, LEVANYUK A P, OSIPOV V V. Nature of sonoluminescence: noble gas radiation excited by hot electrons in cold water [J]. Physical Review E, 2000, 62(2): 2168–2176. DOI: 10.1103/physreve.62.2168. [73] SEDOV S Y, BORISENOK V A. Flexoelectric effect and shock-induced polarization in polar liquids [J]. Physics of Atomic Nuclei, 2019, 82(11): 1547–1551. DOI: 10.1134/s1063778819120275. [74] HU T T, DENG Q, LIANG X, et al. Measuring the flexoelectric coefficient of bulk barium titanate from a shock wave experiment [J]. Journal of Applied Physics, 2017, 122(5): 055106. DOI: 10.1063/1.4997475. [75] HARRIS P, PRESLES H N. The shock induced electrical polarization of water [J]. The Journal of Chemical Physics, 1982, 77(10): 5157–5164. DOI: 10.1063/1.443692. [76] ENIKEEV F U, KUBAREV S I, PONOMAREV O A. Orientation model of shock electrical polarization in a condensed phase [J]. Combustion, Explosion and Shock Waves, 1987, 23(4): 440–446. DOI: 10.1007/bf00749305. [77] KUBAREV S I, PONOMAREV O A, FOKIN A I. A kinetic model of shock electrical polarization [J]. Combustion, Explosion and Shock Waves, 1989, 25(3): 338–343. DOI: 10.1007/bf00788811. [78] WILHELM H E. Shock polarisation of solids between plane electrodes with external load [J]. Journal of Physics D: Applied Physics, 1982, 15(10): 2035–2043. DOI: 10.1088/0022-3727/15/10/022. [79] YAKUSHEV V V. Taking account of the no nuniformity of the dynamical loading of a sample in experiments on polarization of dielectrics under shock compression [J]. Journal of Applied Mechanics and Technical Physics, 1974, 13(4): 564–570. DOI: 10.1007/bf00850404. [80] COLLET B. Shock waves in deformable dielectrics with polarization gradients [J]. International Journal of Engineering Science, 1982, 20(10): 1145–1160. DOI: 10.1016/0020-7225(82)90095-7. [81] HARRIS P. Mechanism for the shock polarization of dielectrics [J]. Journal of Applied Physics, 1965, 36(3): 739–741. DOI: 10.1063/1.1714210. [82] HU T T, YANG W J, LIANG X, et al. Wave propagation in flexoelectric microstructured solids [J]. Journal of Elasticity, 2018, 130(2): 197–210. DOI: 10.1007/s10659-017-9636-3. [83] SALANECK W R. Conformational defects in a conducting polymer [J]. Contemporary Physics, 1989, 30(6): 403–416. DOI: 10.1080/00107518908221989. [84] SPALDIN N A. A beginner’s guide to the modern theory of polarization [J]. Journal of Solid State Chemistry, 2012, 195: 2–10. DOI: 10.1016/j.jssc.2012.05.010. [85] 李冬梅, 李涛, 张大成, 等. 聚吡咯带电态几何结构特征 [J]. 原子与分子物理学报, 2004, 21(1): 105–110. DOI: 10.3969/j.issn.1000-0364.2004.01.023.LI D M, LI T, ZHANG D C, et al. Geometric properties of oxidized states of oligopyrrole [J]. Journal of Atomic and Molecular Physics, 2004, 21(1): 105–110. DOI: 10.3969/j.issn.1000-0364.2004.01.023. [86] ZHANG Y. Soliton excitations in pernigraniline-base polymer: effects of next-nearest-neighbor hopping [J]. Solid State Communications, 2007, 143(6/7): 304–307. DOI: 10.1016/j.ssc.2007.05.040. [87] BABAJANOV D, MATYOKUBOV H, MATRASULOV D. Charged solitons in branched conducting polymers [J]. The Journal of Chemical Physics, 2018, 149(16): 164908. DOI: 10.1063/1.5052044. [88] GODLEWSKI J, OBAROWSKA M. Application of organic materials in electronics [J]. The European Physical Journal Special Topics, 2007, 144(1): 51–66. DOI: 10.1140/epjst/e2007-00108-9. [89] MILLER A, ABRAHAMS E. Impurity conduction at low concentrations [J]. Physical Review, 1960, 120(3): 745–755. DOI: 10.1103/physrev.120.745. [90] MARCUS R A. Nonadiabatic processes involving quantum-like and classical-like coordinates with applications to nonadiabatic electron transfers [J]. The Journal of Chemical Physics, 1984, 81(10): 4494–4500. DOI: 10.1063/1.447418. [91] BÄSSLER H. Charge transport in disordered organic photoconductors a Monte Carlo simulation study [J]. Physica Status Solidi B, 1993, 175(1): 15–56. DOI: 10.1002/pssb.2221750102. [92] GILL W D. Drift mobilities in amorphous charge-transfer complexes of trinitrofluorenone and poly-n-vinylcarbazole [J]. Journal of Applied Physics, 1972, 43(12): 5033–5040. DOI: 10.1063/1.1661065. [93] TANASE C, MEIJER E J, BLOM P W M, et al. Unification of the hole transport in polymeric field-effect transistors and light-emitting diodes [J]. Physical Review Letters, 2003, 91(21): 216601. DOI: 10.1103/physrevlett.91.216601. [94] TANASE C, BLOM P W M, DE LEEUW D M. Origin of the enhanced space-charge-limited current in poly (p-phenylene vinylene) [J]. Physical Review B, 2004, 70(19): 193202. DOI: 10.1103/physrevb.70.193202. [95] PASVEER W F, COTTAAR J, TANASE C, et al. Unified description of charge-carrier mobilities in disordered semiconducting polymers [J]. Physical Review Letters, 2005, 94(20): 206601. DOI: 10.1103/physrevlett.94.206601. [96] NOVIKOV S V, DUNLAP D H, KENKRE V M, et al. Essential role of correlations in governing charge transport in disordered organic materials [J]. Physical Review Letters, 1998, 81(20): 4472–4475. DOI: 10.1103/physrevlett.81.4472. [97] BOUHASSOUNE M, VAN MENSFOORT S L M, BOBBERT P A, et al. Carrier-density and field-dependent charge-carrier mobility in organic semiconductors with correlated Gaussian disorder [J]. Organic Electronics, 2009, 10(3): 437–445. DOI: 10.1016/j.orgel.2009.01.005. [98] POWER D V, LOMBARD D B. Multidirectional peak pressure gauge for strong shock waves [J]. Review of Scientific Instruments, 1966, 37(4): 480–485. DOI: 10.1063/1.1720220. [99] EBRAHIMI F, BARATI M R. Dynamic modeling of embedded nanoplate systems incorporating flexoelectricity and surface effects [J]. Microsystem Technologies, 2019, 25(1): 175–187. DOI: 10.1007/s00542-018-3946-7. [100] BAROUDI S, NAJAR F. Dynamic analysis of a nonlinear nanobeam with flexoelectric actuation [J]. Journal of Applied Physics, 2019, 125(4): 044503. DOI: 10.1063/1.5057727. [101] SLADEK J, SLADEK V, REPKA M, et al. Flexoelectric effect in dielectrics under a dynamic load [J]. Composite Structures, 2021, 260: 113528. DOI: 10.1016/j.compstruct.2020.113528. [102] HU S D, LI H, TZOU H. Comparison of flexoelectric and piezoelectric dynamic signal responses on flexible rings [J]. Journal of Intelligent Material Systems and Structures, 2014, 25(7): 832–844. DOI: 10.1177/1045389x14521701. [103] DENG Q, LV S H, LI Z Q, et al. The impact of flexoelectricity on materials, devices, and physics [J]. Journal of Applied Physics, 2020, 128(8): 080902. DOI: 10.1063/5.0015987. [104] LU Q Q, LIU L W, LAN X, et al. Dynamic responses of SMA-epoxy composites and application for piezoelectric energy harvesting [J]. Composite Structures, 2016, 153: 843–850. DOI: 10.1016/j.compstruct.2016.07.008. [105] FAN M, DENG B L, TZOU H. Dynamic flexoelectric actuation and vibration control of beams [J]. Journal of Vibration and Acoustics, 2018, 140(4): 041005. DOI: 10.1115/1.4039238. [106] MOURA A G, ERTURK A. Combined piezoelectric and flexoelectric effects in resonant dynamics of nanocantilevers [J]. Journal of Intelligent Material Systems and Structures, 2018, 29(20): 3949–3959. DOI: 10.1177/1045389x18803441. 期刊类型引用(5)
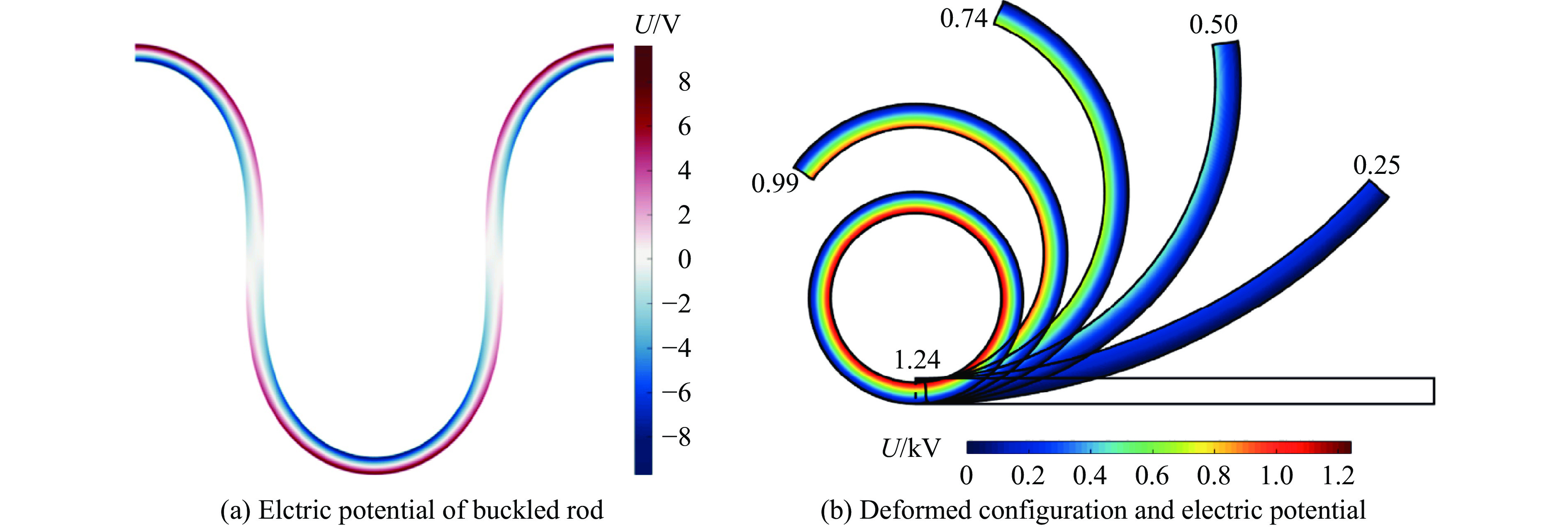
1. 张萌,杨扬,张岳青,柳柳,王慧. 一种修正的GFRP本构模型及其双层板结构抗冲击特性预测. 振动与冲击. 2021(03): 73-80 .  百度学术
百度学术2. 董新刚,郑凯斌,喻琳峰,吴玉燕,李岩芳. 爆炸冲击作用下绝热挡环破碎性能研究. 应用力学学报. 2021(04): 1309-1317 .  百度学术
百度学术3. 张晨曦,娄源峰,铁瑛,丛世凡,李要磊. 基于渐进均匀化多尺度方法的CFR平纹机织材料冲击后压缩损伤研究. 复合材料科学与工程. 2021(10): 5-12 .  百度学术
百度学术4. 张洁皓,段晨,侯玉亮,铁瑛,李成. 基于渐进均匀化的平纹编织复合材料低速冲击多尺度方法. 力学学报. 2019(05): 1411-1423 .  百度学术
百度学术5. 张明,原梅妮,向丰华,王振兴. Kevlar-129纤维复合材料抗侵彻性能数值模拟. 材料导报. 2015(24): 117-121 .  百度学术
百度学术其他类型引用(5)
-







 下载:
下载:








 下载:
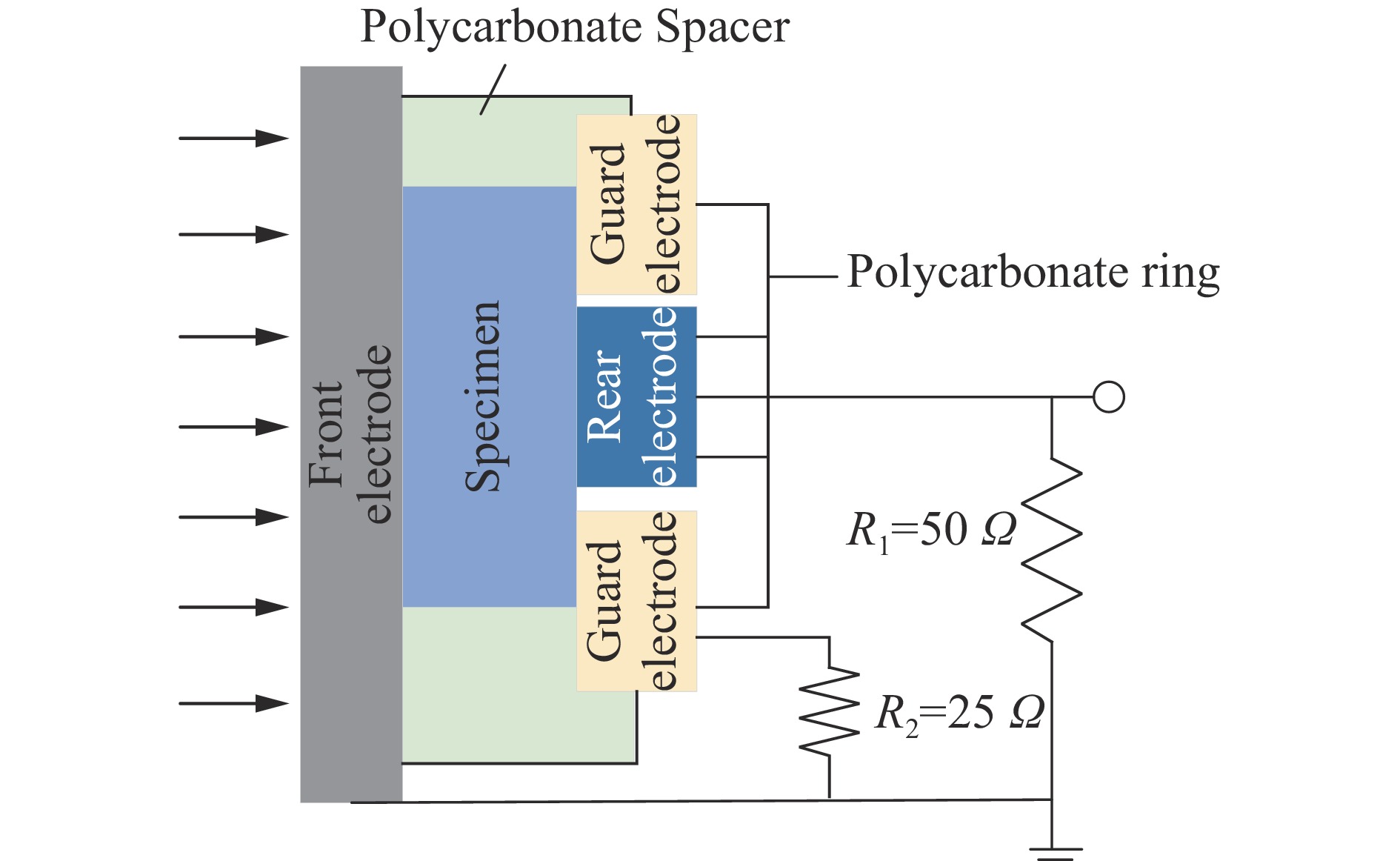
下载:



































 百度学术
百度学术






