混凝土中带壳柱形装药爆炸应力波衰减规律的数值模拟

杨耀宗; 孔祥振; 方秦; 洪智捷; 高矗

doi:10.11883/bzycj-2023-0342

混凝土中带壳柱形装药爆炸应力波衰减规律的数值模拟

doi: 10.11883/bzycj-2023-0342

陆军工程大学爆炸冲击防灾减灾国家重点实验室，江苏南京 210007

基金项目: 国家自然科学基金（52178515）

详细信息

作者简介:
杨耀宗（1998－　），男，博士研究生，yyz542968@163.com

通讯作者:
孔祥振（1988－　），男，博士，副教授，ouckxz@163.com

中图分类号: O385
计量
- 文章访问数: 122
- HTML全文浏览量: 9
- PDF下载量: 83
- 被引次数: 0
出版历程
- 收稿日期: 2023-09-15
- 修回日期: 2024-05-15
- 网络出版日期: 2024-05-16

Numerical investigation on attenuation of stress waves in concrete induced by cylindrical cased charge explosion

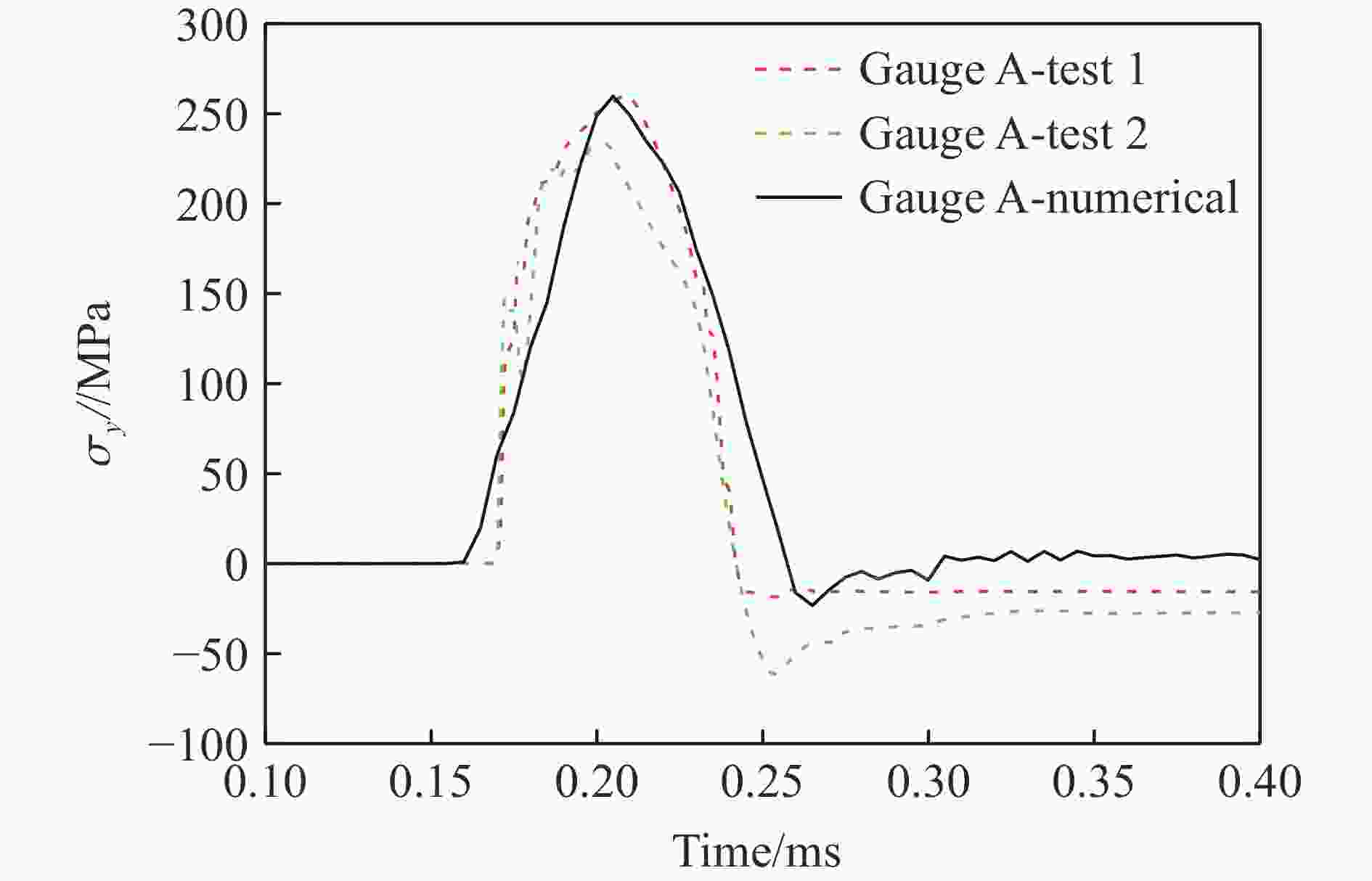
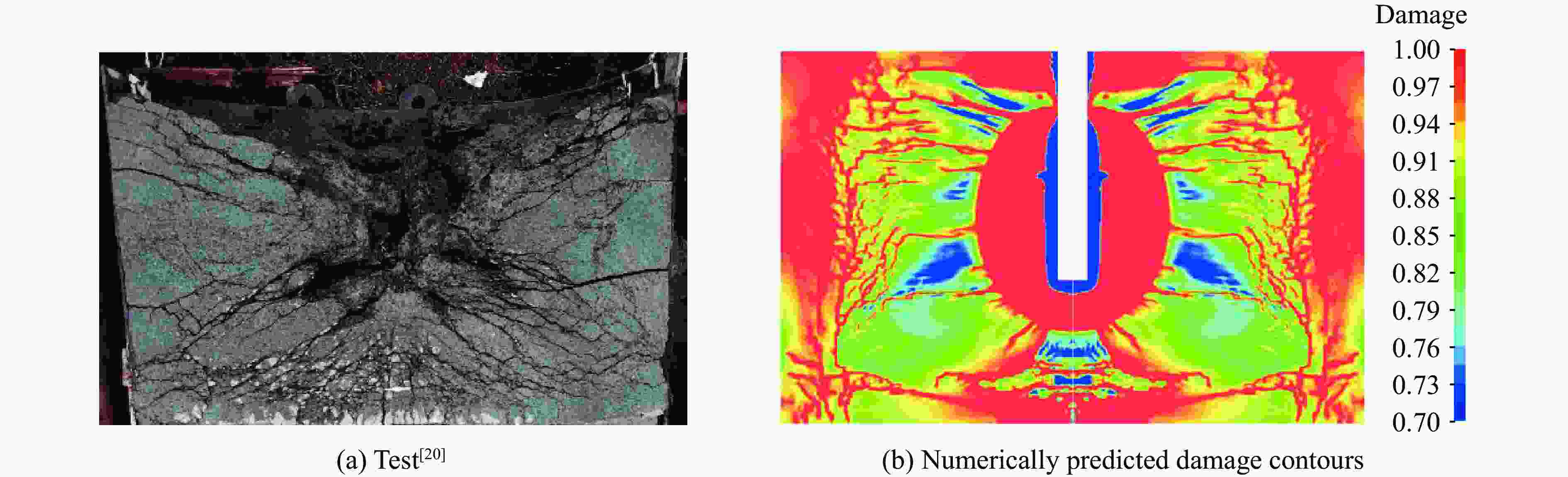
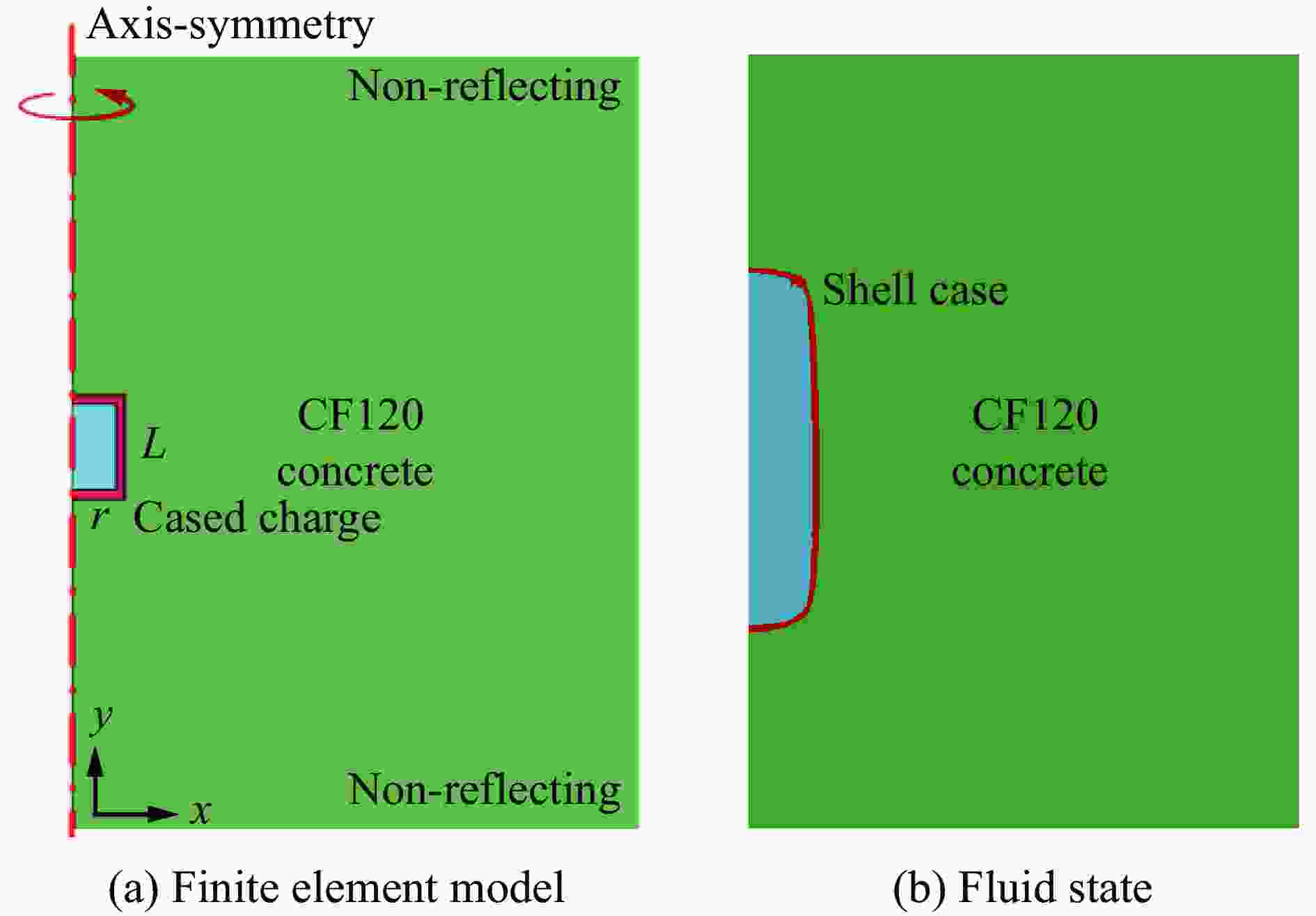
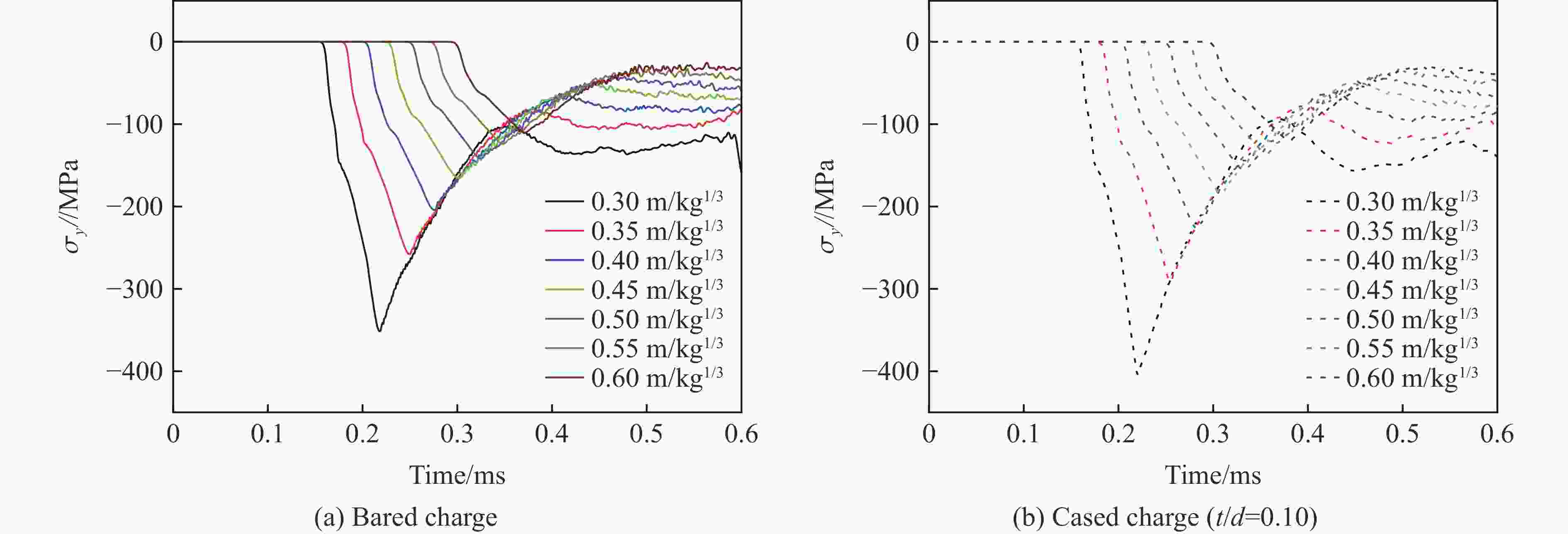
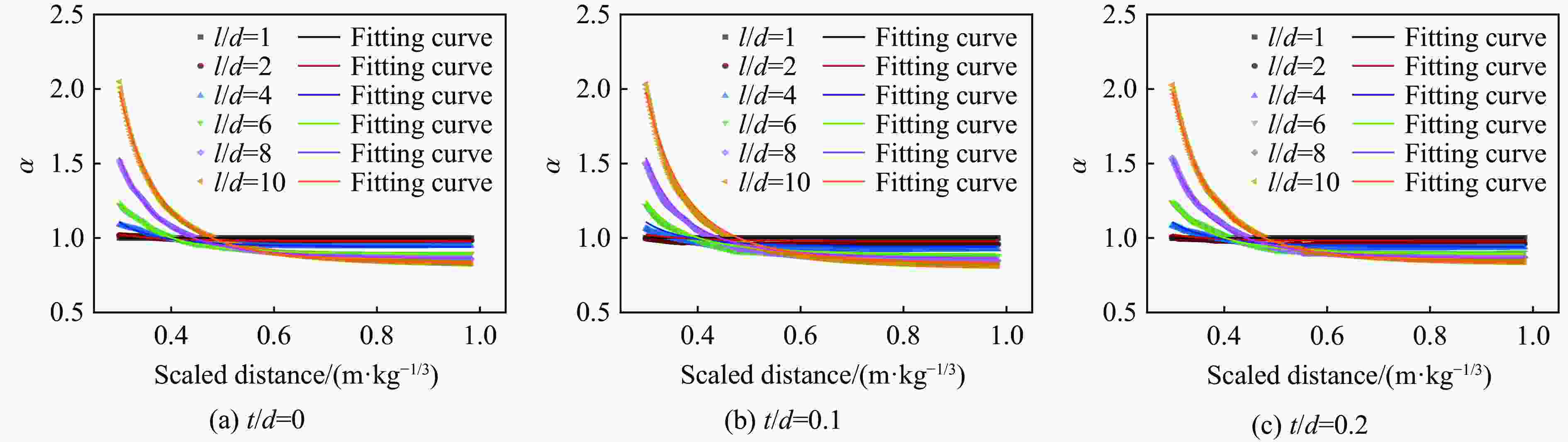
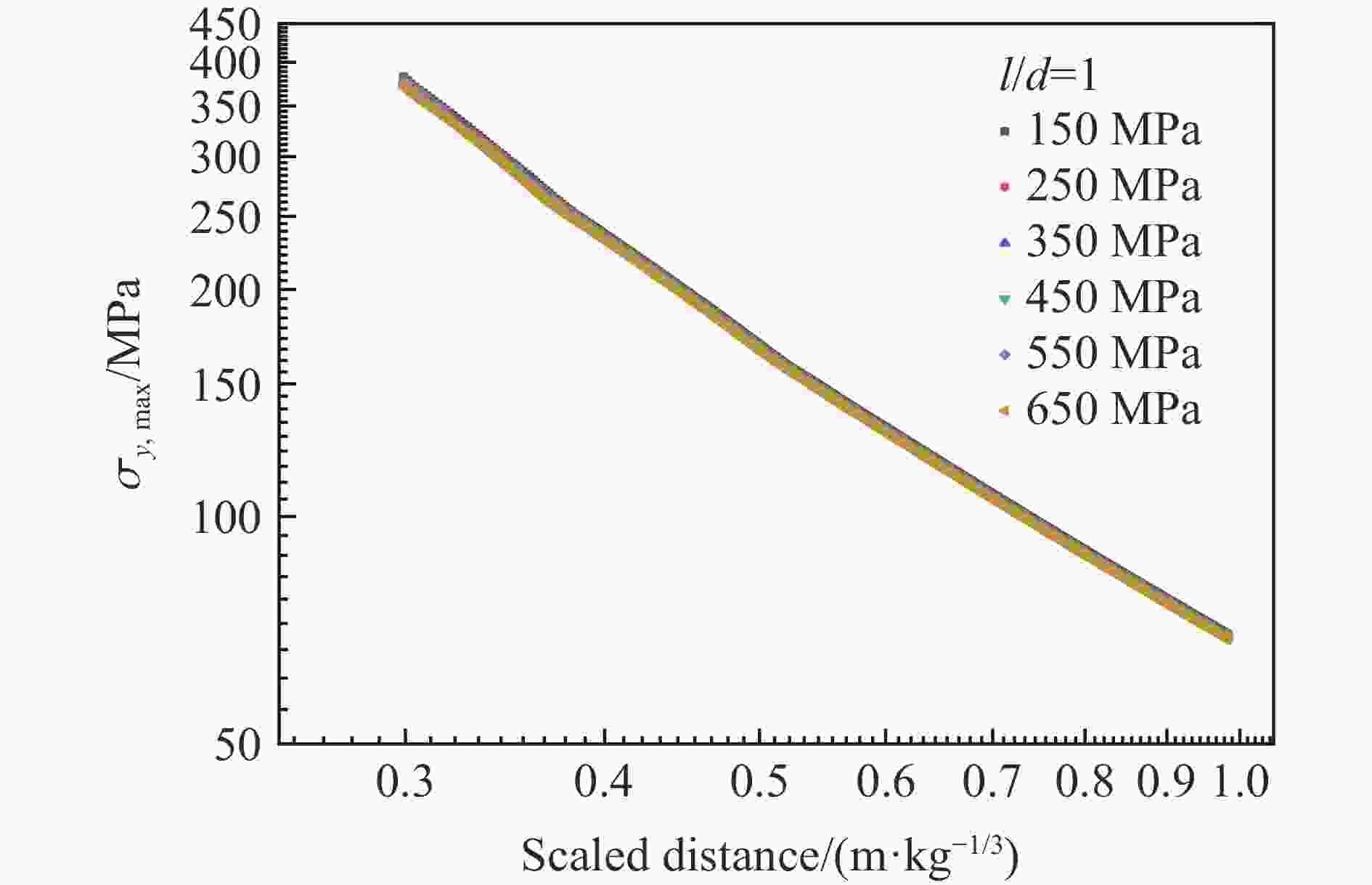
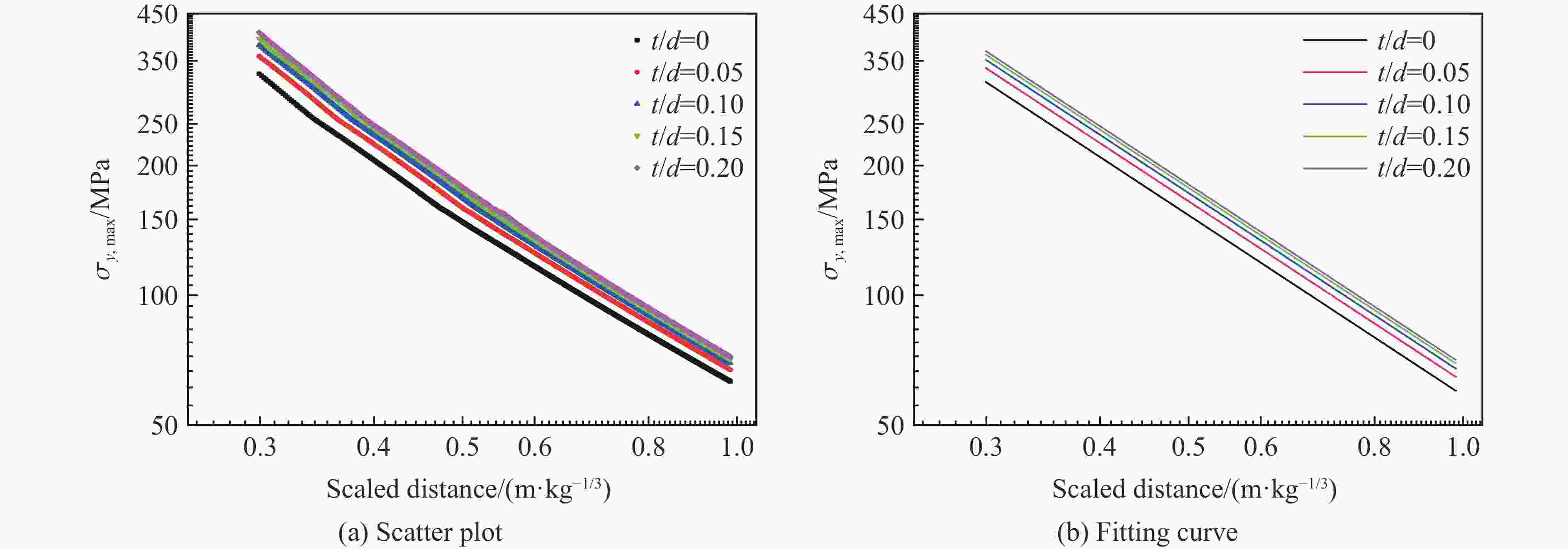
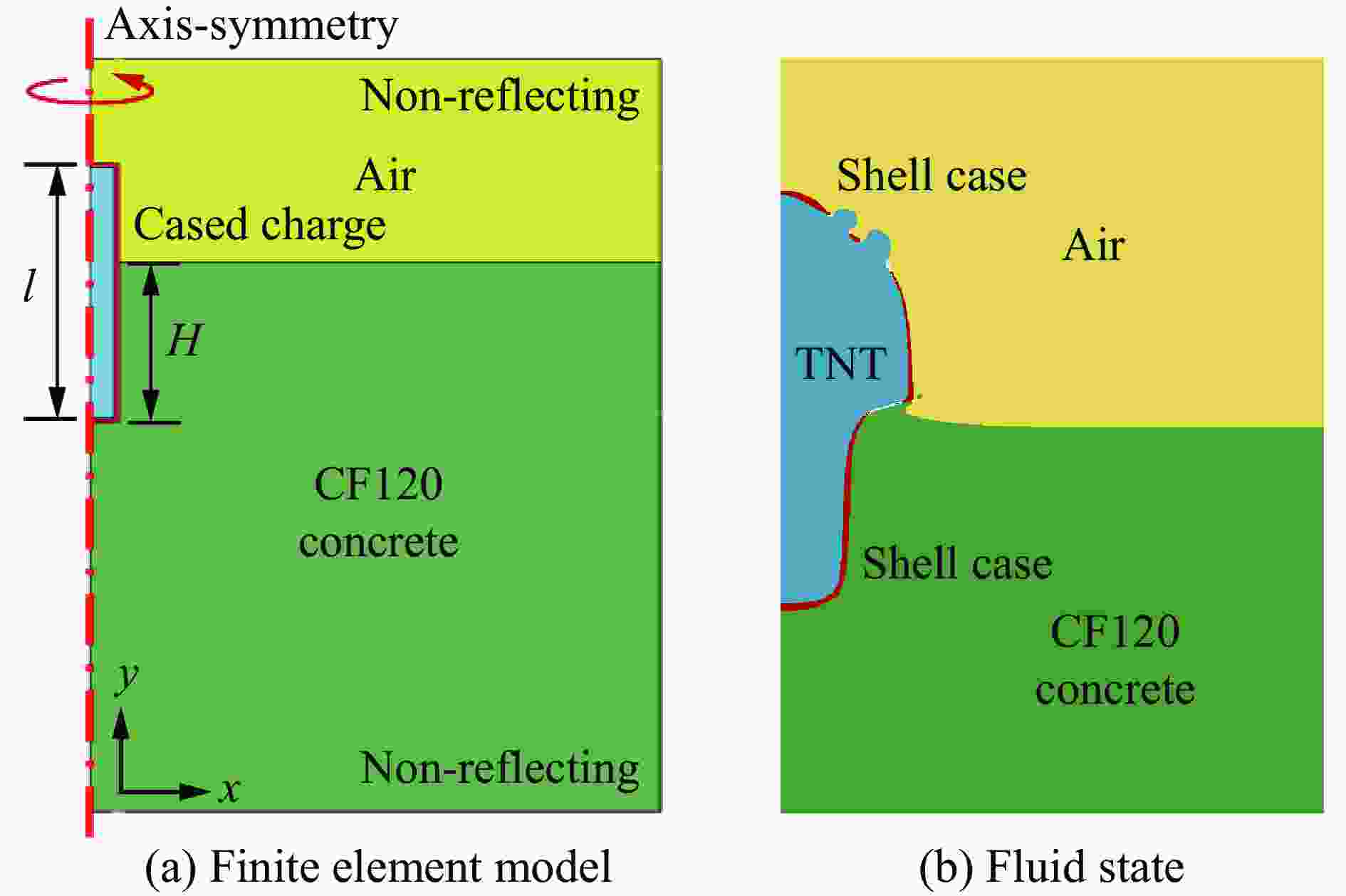
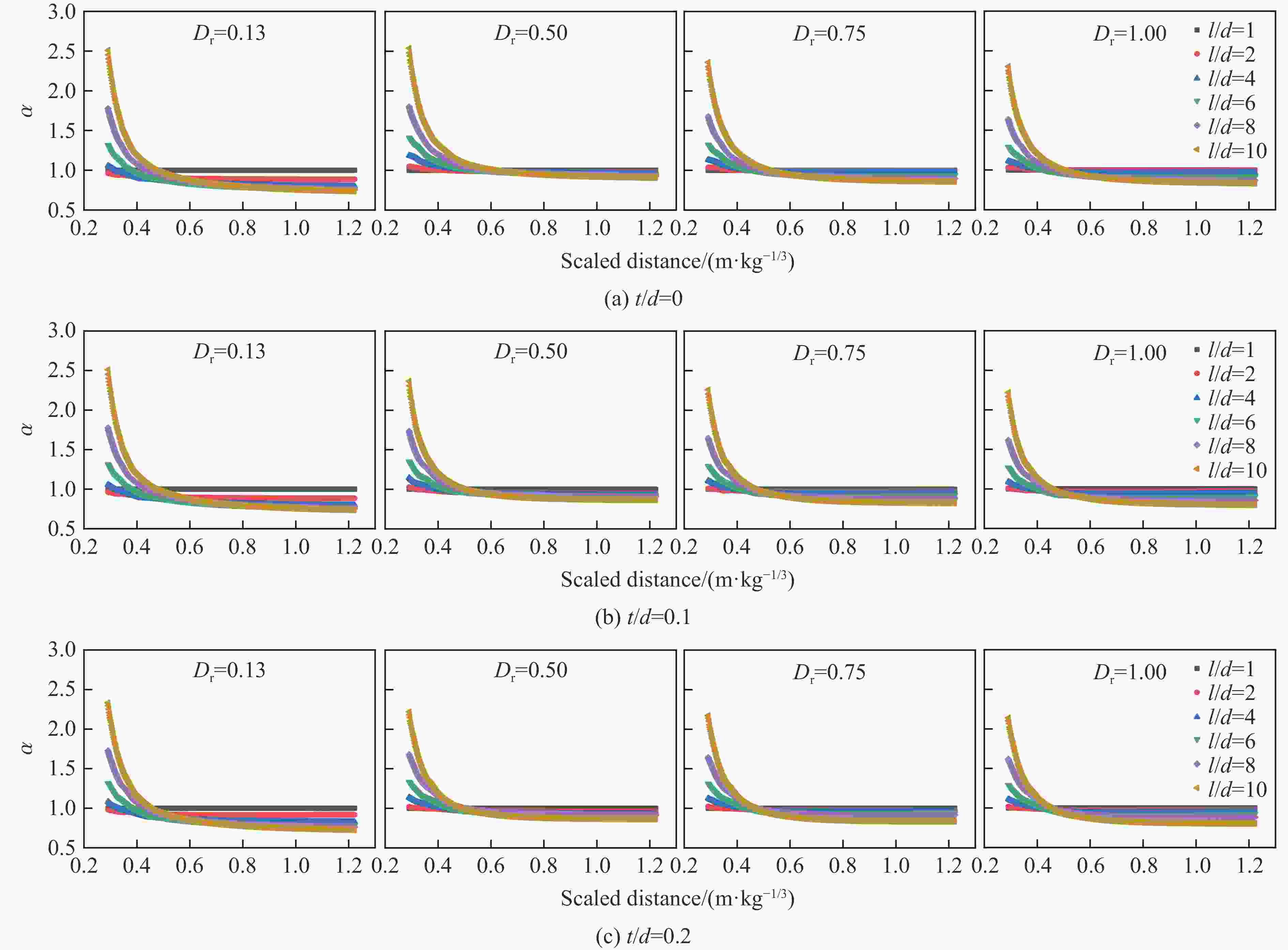
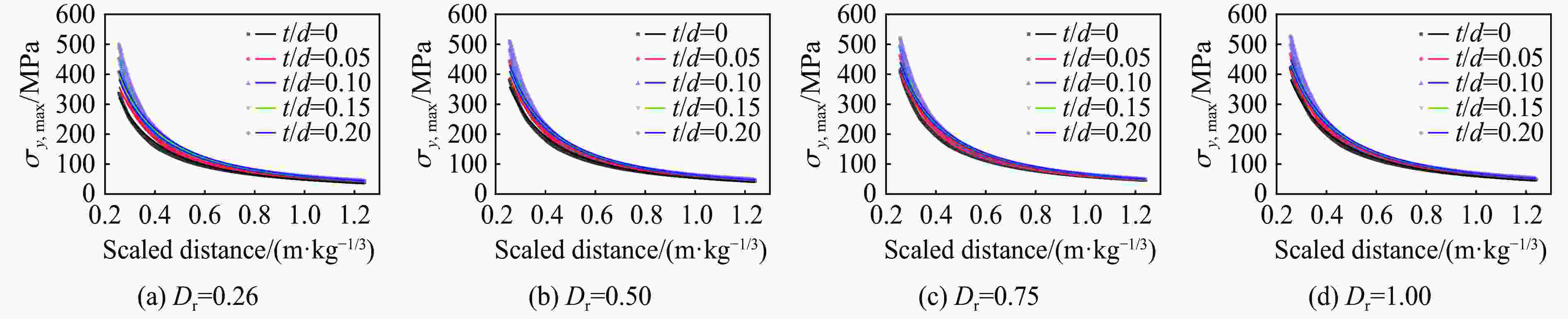
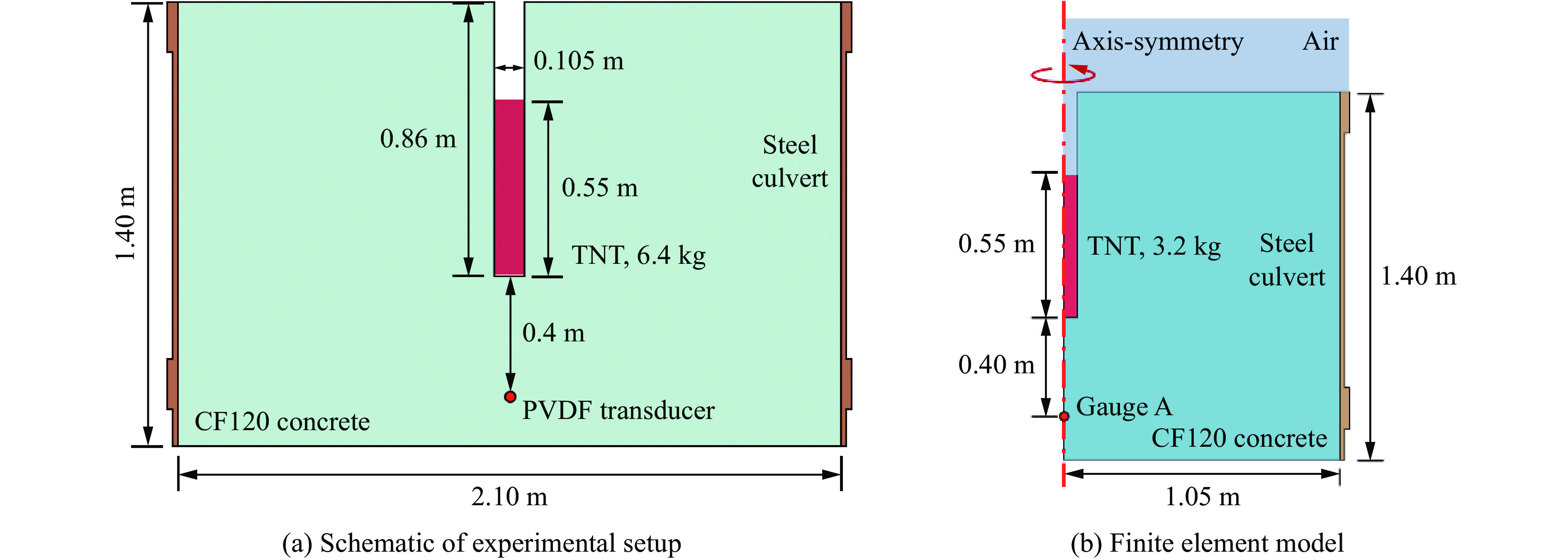
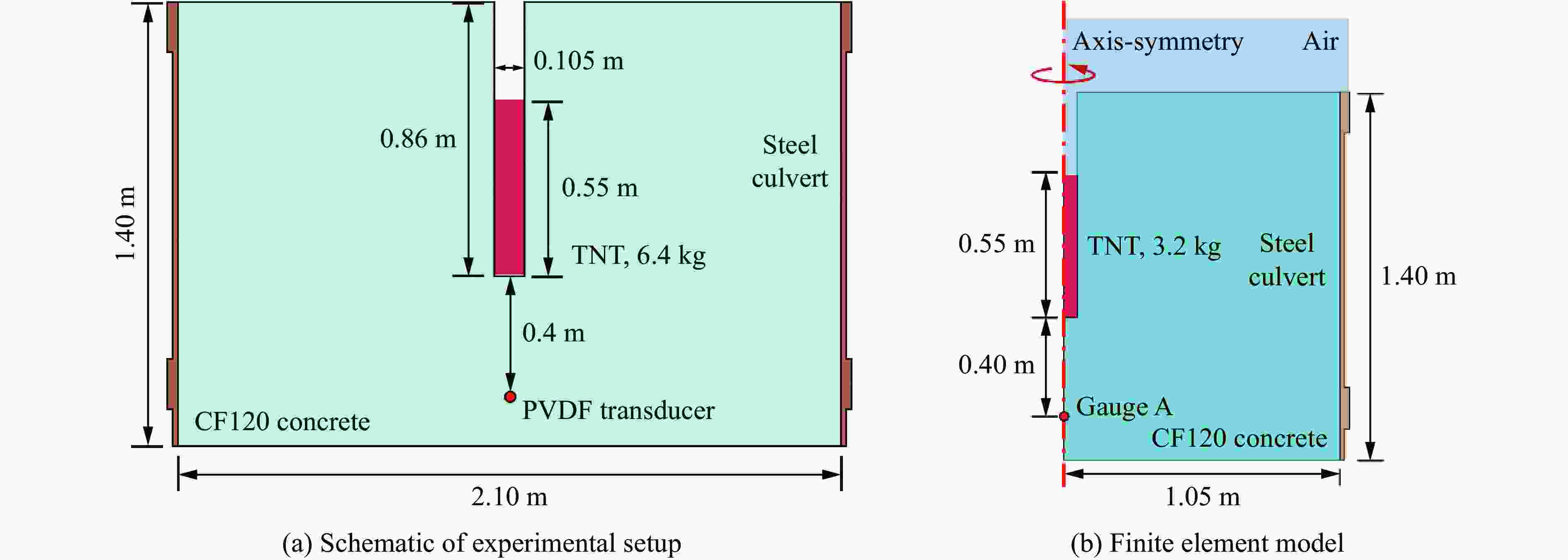
State Key Laboratory of Disaster Prevention & Mitigation of Explosion & Impact, PLA Army Engineering University, Nanjing 210007, Jiangsu, China

摘要

摘要: 基于Kong-Fang混凝土材料模型和LS-DYNA中的多物质ALE算法，开展了CF120混凝土中带壳柱形装药爆炸波衰减规律的数值模拟研究：首先基于已有的柱形装药埋置爆炸试验，对数值算法和材料模型参数进行验证；在此基础上，通过定义长径比系数、壳厚比系数以及峰值应力耦合系数定量分析了装药形状、壳体厚度和埋深对峰值应力的影响规律；最后利用数值模拟数据拟合出混凝土中带壳柱形装药爆炸波峰值应力的计算公式。研究结果表明，带壳装药爆炸近区，长径比越大，峰值应力越大，远区则相反，且壳体越厚，峰值应力越大，但存在一个阈值；建立的爆炸波峰值应力计算公式可实现对不同长径比、不同壳体厚度和不同装药埋深的带壳柱形装药爆炸波峰值应力的快速预测，可为结构抗爆设计提供依据。
- 混凝土 /
- 带壳柱形装药 /
- 爆炸波 /
- 峰值应力
Abstract: The warhead of conventional weapons is usually composed of a cylindrical charge and a metal case, in which the metal case can affect the attenuation law of peak stress induced by explosion. Therefore, it is important for the blast-resistant design to clarify the attenuation law of stress waves in CF120 concrete induced by cylindrical cased charge explosion. Based on the Kong-Fang concrete material model and the multi-material arbitrary Lagrangian-Eulerian (MM-ALE) algorithm available in the LS-DYNA, the attenuation law of stress waves in concrete subjected to cylindrical cased charge explosion was numerically investigated in this paper. Firstly, the numerical algorithm and material model parameters were validated against two sets of cylindrical charge explosion tests. Then a series of fully enclosed and partially buried cylindrical charge explosion numerical models were established, in which different aspect ratios, shell thicknesses, and charge buried depths were considered to analyze the influence of charge shape and shell thickness on stress waves in concrete. Finally, an empirical formula for peak stress of compression wave in concrete induced by cylindrical cased charge explosion was presented based on curve-fitting the numerical data. Numerical results demonstrate that the larger the aspect ratio, the higher the peak stress in the near region, while the opposite law takes on in the far region. Besides, increasing the shell thickness will make the peak stress higher, but there is a threshold. The influence of charge shape, shell thickness, and charge buried depth on the peak stress can be quantified by defining the length-diameter ratio, thickness-diameter ratio, and coupling factor of peak stress. The empirical formula for peak stress of compression wave in concrete is valid for varied aspect ratio, shell thickness, and charge buried depth, which can provide a reliable reference for blast-resistant design to estimate the peak stress induced by cylindrical cased charge explosion.
- concrete /
- cylindrical cased charge /
- explosion wave /
- peak stress

HTML全文

图 1 实验设置和有限元模型示意

Figure 1. Schematic of experiment setup and finite element model

下载: 全尺寸图片幻灯片

图 2 测点A应力时程曲线实验数据和数值模拟的对比

Figure 2. Comparison of stress-time histories between experimental data and numerical predictions of Gauge A

下载: 全尺寸图片幻灯片

图 3 靶体内部损伤破坏情况

Figure 3. Failure mode inside concrete target

下载: 全尺寸图片幻灯片

图 4 带壳装药爆炸的有限元模型示意图和流体状态示意图

Figure 4. Finite element model of cased charge explosion and the fluid state of each part

下载: 全尺寸图片幻灯片

图 5 装药正下方0.30～0.60 m/kg^1/3范围内测点应力时程曲线

Figure 5. Stress-time histories of gauges at scaled distances varied from 0.30 to 0.60 m/kg^1/3

下载: 全尺寸图片幻灯片

图 6 装药正下方峰值应力

Figure 6. Peak stress below the cylindrical charge

下载: 全尺寸图片幻灯片

图 7 长径比系数α的散点图和拟合曲线

Figure 7. Scatter plot and fitting curves of α

下载: 全尺寸图片幻灯片

图 8 不同壳体强度的峰值应力散点图

Figure 8. Peak stress for different shell strengths

下载: 全尺寸图片幻灯片

图 9 长径比为1的柱形装药正下方峰值应力散点图和拟合曲线

Figure 9. Scatter plot and fitting curve of peak stress below the cylindrical charge with the length-diameter ratio of 1

下载: 全尺寸图片幻灯片

图 10 带壳装药埋置爆炸的有限元模型示意图和流体状态示意图

Figure 10. Fnite element model of explosion of buried cased charge and the fluid state of each part

下载: 全尺寸图片幻灯片

图 11 不同相对埋深D_r下的峰值应力耦合系数f₀

Figure 11. Coupling coefficient of peak stress (f₀) at different relative buried depth (D_r)

下载: 全尺寸图片幻灯片

图 12 完全封闭爆炸工况和相对埋置深度为1的峰值应力对比

Figure 12. Comparison of peak stress between closed explosion and buried explosion with the relative buried depth of 1

下载: 全尺寸图片幻灯片

图 13 不同埋深下的长径比系数

Figure 13. Coefficient of length-to-diameter ratio α at different relative buried depth (D_r)

下载: 全尺寸图片幻灯片

图 14 埋深D_r对不同壳厚比t/d下峰值应力σ_m的影响

Figure 14. Affection of buried depth (D_r) on peak stress (σ_m) at different ratio of case-thickness to charge-diameter (t/d)

下载: 全尺寸图片幻灯片

图 15 壳厚比系数γ随埋深D_r变化

Figure 15. Relation between the coeffient of case-thickness to charge-diameter ratio (γ) and burid depth (D_r)

下载: 全尺寸图片幻灯片

表 1 柱形装药尺寸

Table 1. Size of cylindrical charge

l/d	l/m	d/m	l/d	l/m	d/m
1	0.215	0.216	6	0.716	0.118
2	0.346	0.170	8	0.862	0.108
4	0.543	0.136	10	1.000	0.100

下载: 导出CSV

表 2 公式(5)参数取值

Table 2. Parameter values in Eq. (5)

l/d	k	b	c	l/d	k	b	c
1	0	4	1	6	28.9×10⁻⁴	4	0.89
2	3.63×10⁻⁴	4	0.98	8	54.5×10⁻⁴	4	0.86
4	13.4×10⁻⁴	4	0.94	10	93.0×10⁻⁴	4	0.82

下载: 导出CSV

表 3 公式(6)参数取值

Table 3. Parameter values of Eq. (6)

t/d	S	n	t/d	S	n
0	58.5	1.39	0.15	67.8	1.39
0.05	63.1	1.39	0.20	69.0	1.39
0.10	65.9	1.39

下载: 导出CSV

表 4 壳厚比系数取值

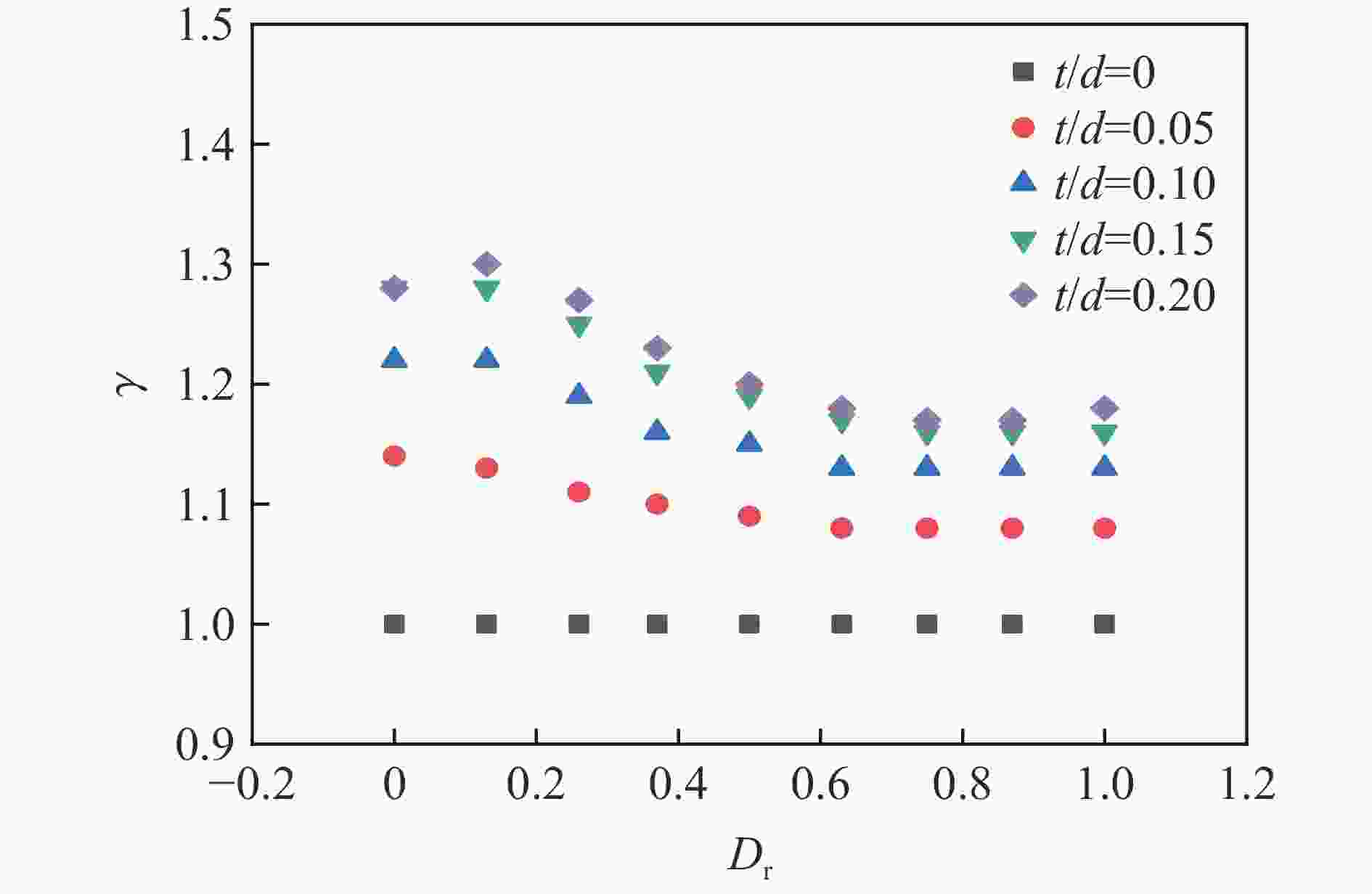
Table 4. Values of γ

t/d	0	0.05	0.10	0.15	0.20
γ	1.00	1.08	1.13	1.16	1.18

下载: 导出CSV

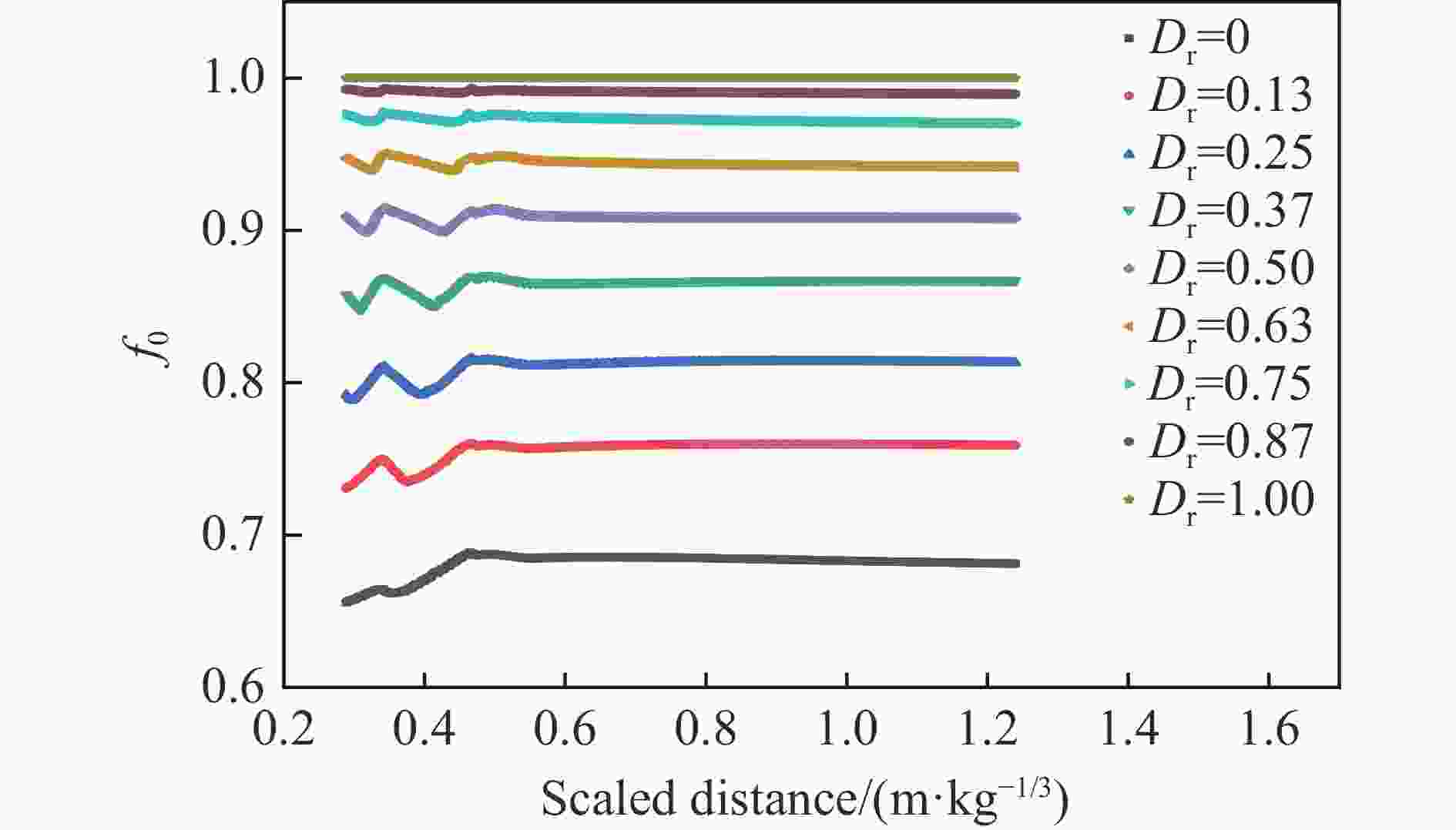
表 5 不同相对埋深下的峰值应力耦合系数$f_0 $（比例爆距范围0.47～1.0 m/kg^1/3）

Table 5. Coupling coefficient of peak stress ( f₀) at different relative buried depth (D_r) within the range of 0.47～1.0 m/kg^1/3

D_r	0.00	0.13	0.25	0.37	0.50	0.63	0.75	0.87	1.00
f₀	0.68	0.76	0.81	0.87	0.91	0.94	0.97	0.99	1.00

下载: 导出CSV

表 6 不同埋深$D_{\mathrm{r}} $下的壳厚比系数γ

Table 6. Coeffient of case-thickness to charge-diameter ratio (γ) at different burid depths (D_r)

D_r	γ
D_r	t/d=0	t/d=0.05	t/d=0.10	t/d=0.15	t/d=0.20
0	1	1.14	1.22	1.28	1.28
0.13	1	1.13	1.22	1.28	1.3
0.26	1	1.11	1.19	1.25	1.27
0.37	1	1.10	1.16	1.21	1.23
0.50	1	1.09	1.15	1.19	1.20
0.63	1	1.08	1.13	1.17	1.18
0.75	1	1.08	1.13	1.16	1.17
0.87	1	1.08	1.13	1.16	1.17
1.00	1	1.08	1.13	1.16	1.18

下载: 导出CSV

参考文献(34)

[1]	张世豪, 韩晶, 张欣欣, 等. 带壳装药壳体厚度对混凝土爆破毁伤效果的影响 [J]. 爆破, 2013, 30(1): 25–29,34. DOI: 10.3963/j.issn.1001-487X.2013.01.006. ZHANG S H, HAN J, ZHANG X X, et al. Effect of shell thickness of shell charge on explosion and damage effect in concrete [J]. Blasting, 2013, 30(1): 25–29,34. DOI: 10.3963/j.issn.1001-487X.2013.01.006.
[2]	LOCKING P M, FLYNN D, DUNNETT J. Warhead filling and casing interactions affect the blast field performance [C]//Proceedings of the 24th International Symposium on Ballistics. New Orleans, LA, USA, 2008.
[3]	梁斌, 陈忠富, 卢永刚, 等. 不同材料壳体装药对爆破威力影响分析 [J]. 解放军理工大学学报(自然科学版), 2007, 8(5): 429–433. DOI: 10.3969/j.issn.1009-3443.2007.05.005. LIANG B, CHEN Z F, LU Y G, et al. Investigation of blast effect of explosive charge with different shell material [J]. Journal of PLA University of Science and Technology, 2007, 8(5): 429–433. DOI: 10.3969/j.issn.1009-3443.2007.05.005.
[4]	GRISARO H Y, BENAMOU D, DANCYGIER A N. Investigation of blast and fragmentation loading characteristics–Field tests [J]. Engineering Structures, 2018, 167: 363–375. DOI: 10.1016/j.engstruct.2018.04.013.
[5]	LI Y, CHEN Z Y, REN X B, et al. Experimental and numerical study on damage mode of RC slabs under combined blast and fragment loading [J]. International Journal of Impact Engineering, 2020, 142: 103579. DOI: 10.1016/j.ijimpeng.2020.103579.
[6]	刘彦, 段卓平, 王新生, 等. 不同厚度壳体装药在混凝土中爆炸的实验研究 [J]. 北京理工大学学报, 2010, 30(7): 771–773,848. DOI: 10.15918/j.tbit1001-0645.2010.07.008. LIU Y, DUAN Z P, WANG X S, et al. Experiments on explosion of explosives with different thickness shells in concretes [J]. Transactions of Beijing Institute of Technology, 2010, 30(7): 771–773,848. DOI: 10.15918/j.tbit1001-0645.2010.07.008.
[7]	王新生, 黄风雷, 刘彦, 等. 大长径比带壳装药爆炸毁伤混凝土试验 [J]. 兵工学报, 2009, 30(S2): 251–254. WANG X S, HUANG F L, LIU Y, et al. Experiment on large length-diameter ratio shell charge explosion and damage in concretes [J]. Acta Armamentarii, 2009, 30(S2): 251–254.
[8]	张奇, 覃彬, 孙庆云, 等. 战斗部壳体厚度对爆炸空气冲击波的影响 [J]. 弹道学报, 2008, 20(2): 17–19,23. ZHANG Q, QIN B, SUN Q Y, et al. Influence of thickness of warhead shell upon explosive shock wave [J]. Journal of Ballistics, 2008, 20(2): 17–19,23.
[9]	李茂, 朱锡, 侯海量, 等. 冲击波和高速破片联合作用下固支方板毁伤效应数值模拟 [J]. 国防科技大学学报, 2017, 39(6): 64–70. DOI: 10.11887/j.cn.201706011. LI M, ZHU X, HOU H L, et al. Numerical simulation of the damage effects of clamped square plate subjected to the impact of blast wave and fragments [J]. Journal of National University of Defense Technology, 2017, 39(6): 64–70. DOI: 10.11887/j.cn.201706011.
[10]	NYSTRÖM U, GYLLTOFT K. Numerical studies of the combined effects of blast and fragment loading [J]. International Journal of Impact Engineering, 2009, 36(8): 995–1005. DOI: 10.1016/j.ijimpeng.2009.02.008.
[11]	苏波, 唐勇, 顾文彬, 等. 带壳装药在多层介质中爆炸的数值模拟研究 [J]. 爆破, 2009, 26(1): 15–18,36. DOI: 10.3963/j.issn.1001-487X.2009.01.004. SU B, TANG Y, GU W B, et al. Numerical simulation of blast effects for charge with case in multi-layer medium [J]. Blasting, 2009, 26(1): 15–18,36. DOI: 10.3963/j.issn.1001-487X.2009.01.004.
[12]	孙善政, 卢浩, 李杰, 等. 侵爆作用下混凝土靶破坏效应试验与数值模拟 [J]. 振动与冲击, 2022, 41(1): 206–212. DOI: 10.13465/j.cnki.jvs.2022.01.026. SUN S Z, LU H, LI J, et al. Tests and numerical simulation for damage effect of concrete target under penetration and explosion [J]. Journal of Vibration and Shock, 2022, 41(1): 206–212. DOI: 10.13465/j.cnki.jvs.2022.01.026.
[13]	梁斌, 陈忠富, 陈小伟. 爆炸载荷对混凝土毁伤效应分析 [J]. 弹箭与制导学报, 2006, 26(3): 104–107. DOI: 10.3969/j.issn.1673-9728.2006.03.034. LAING B, CHEN Z F, CHEN X W. Damage analysis of concrete subject to explosive loading [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2006, 26(3): 104–107. DOI: 10.3969/j.issn.1673-9728.2006.03.034.
[14]	傅学金, 强洪夫, 杨月诚. 固体介质中SPH方法的拉伸不稳定性问题研究进展 [J]. 力学进展, 2007, 37(3): 375–388. DOI: 10.3321/j.issn:1000-0992.2007.03.005. FU X J, QIANG H F, YANG Y C, et al. Advances in the tensile instability of smoothed particle hydrodynamics applied to solid dynamics [J]. Advances in Mechanics, 2007, 37(3): 375–388. DOI: 10.3321/j.issn:1000-0992.2007.03.005.
[15]	WU C T, WU Y C, CRAWFORD J E, et al. Three-dimensional concrete impact and penetration simulations using the smoothed particle Galerkin method [J]. International Journal of Impact Engineering, 2017, 106: 1–17. DOI: 10.1016/j.ijimpeng.2017.03.005.
[16]	王新征, 张松林, 邹广平. 内部短药柱爆炸作用下钢筒破裂特征的数值分析 [J]. 高压物理学报, 2010, 24(1): 61–66. DOI: 10.11858/gywlxb.2010.01.011. WANG X Z, ZHANG S L, ZOU G P. Numerical analysis on fragmentation properties of the steel cylinder subjected to detonation of internal short cylinderical explosive charge [J]. Chinese Journal of High Pressure Physics, 2010, 24(1): 61–66. DOI: 10.11858/gywlxb.2010.01.011.
[17]	KONG X S, WU W G, LI J, et al. A numerical investigation on explosive fragmentation of metal casing using Smoothed Particle Hydrodynamic method [J]. Materials & Design, 2013, 51: 729–741. DOI: 10.1016/j.matdes.2013.04.041.
[18]	李营, 吴卫国, 朱海清, 等. 爆炸冲击波与破片对RC桥的耦合毁伤研究 [J]. 爆破, 2016, 33(2): 142–148. DOI: 10.3963/j.issn.1001-487X.2016.02.028. LI Y, WU W G, ZHU H Q, et al. Damage characteristics of RC bridge under combined effects of blast shock wave and fragments loading [J]. Blasting, 2016, 33(2): 142–148. DOI: 10.3963/j.issn.1001-487X.2016.02.028.
[19]	廖南, 洪建, 方秦, 等. 带壳装药爆炸冲击波与破片荷载规律的数值模拟研究 [J]. 防护工程, 2022, 44(6): 7–14. DOI: 10.3969/j.issn.1674-1854.2022.06.002. LIAO N, HONG J, FANG Q, et al. Numerical simulation of the loading law of shock wave and fragment under cased charge blast [J]. Protective Engineering, 2022, 44(6): 7–14. DOI: 10.3969/j.issn.1674-1854.2022.06.002.
[20]	GAO C, KONG X Z, FANG Q. Experimental and numerical investigation on the attenuation of blast waves in concrete induced by cylindrical charge explosion [J]. International Journal of Impact Engineering, 2023, 174: 104491. DOI: 10.1016/j.ijimpeng.2023.104491.
[21]	KONG X Z, FANG Q, CHEN L, et al. A new material model for concrete subjected to intense dynamic loadings [J]. International Journal of Impact Engineering, 2018, 120: 60–78. DOI: 10.1016/j.ijimpeng.2018.05.006.
[22]	WANG Y, KONG X Z, FANG Q, et al. Modelling damage mechanisms of concrete under high confinement pressure [J]. International Journal of Impact Engineering, 2021, 150: 103815. DOI: 10.1016/j.ijimpeng.2021.103815.
[23]	ZHANG S B, KONG X Z, FANG Q, et al. Numerical prediction of dynamic failure in concrete targets subjected to projectile impact by a modified Kong-Fang material model [J]. International Journal of Impact Engineering, 2020, 144: 103633. DOI: 10.1016/j.ijimpeng.2020.103633.
[24]	MANDAL J, GOEL M D, AGARWAL A K. Surface and buried explosions: an explorative review with recent advances [J]. Archives of Computational Methods in Engineering, 2021, 28(7): 4815–4835. DOI: 10.1007/s11831-021-09553-2.
[25]	高矗, 孔祥振, 方秦, 等. 混凝土中爆炸应力波衰减规律的数值模拟研究 [J]. 爆炸与冲击, 2022, 42(12): 123202. DOI: 10.11883/bzycj-2022-0041. GAO C, KONG X Z, FANG Q, et al. Numerical study on attenuation of stress wave in concrete subjected to explosion [J]. Explosion and Shock Waves, 2022, 42(12): 123202. DOI: 10.11883/bzycj-2022-0041.
[26]	YANG S B, KONG X Z, WU H, et al. Constitutive modelling of UHPCC material under impact and blast loadings [J]. International Journal of Impact Engineering, 2021, 153: 103860. DOI: 10.1016/j.ijimpeng.2021.103860.
[27]	WILLIAMS E M, GRAHAM S S, AKERS S A, et al. Mechanical properties of a baseline UHPC with and without steel fibers [J]. WIT Transactions on Engineering Sciences, 2009, 64(12): 93–104. DOI: 10.2495/MC090091.
[28]	REN G M, WU H, FANG Q, et al. Triaxial compressive behavior of UHPCC and applications in the projectile impact analyses [J]. Construction and Building Materials, 2016, 113: 1–14. DOI: 10.1016/j.conbuildmat.2016.02.227.
[29]	TARVER C M, MCGUIRE E M. Reactive Flow modeling of the interaction of TATB detonation waves with inert materials: UCRL-JC-145013 [R]. Livermore: Lawrence Livermore National Lab. , 2002.
[30]	王银, 孔祥振, 方秦, 等. 弹体对混凝土材料先侵彻后爆炸损伤破坏效应的数值模拟研究 [J]. 爆炸与冲击, 2022, 42(1): 013301. DOI: 10.11883/bzycj-2021-0132. WANG Y, KONG X Z, FANG Q, et al. Numerical investigation on damage and failure of concrete targets subjected to projectile penetration followed by explosion [J]. Explosion and Shock Waves, 2022, 42(1): 013301. DOI: 10.11883/bzycj-2021-0132.
[31]	XIAO W F, ANDRAE M, GEBBEKEN N. Effect of charge shape and initiation configuration of explosive cylinders detonating in free air on blast-resistant design [J]. Journal of Structural Engineering, 2020, 146(8): 04020146. DOI: 10.1061/(ASCE)ST.1943-541X.0002694.
[32]	RIGBY S E, OSBORNE C, LANGDON G S, et al. Spherical equivalence of cylindrical explosives: effect of charge shape on deflection of blast-loaded plates [J]. International Journal of Impact Engineering, 2021, 155: 103892. DOI: 10.1016/j.ijimpeng.2021.103892.
[33]	方秦, 柳锦春. 地下防护结构 [M]. 北京: 中国水利水电出版社, 2010: 45–47.
[34]	李玉节, 张效慈, 汪俊, 等. 带壳有隙TNT炸药包的水下爆炸 [J]. 船舶力学, 2005, 9(3): 118–125. DOI: 10.3969/j.issn.1007-7294.2005.03.012. LI Y J, ZHANG X C, WANG J, et al. Underwater explosion of TNT dynamite with a metal shell and annular gap [J]. Journal of Ship Mechanics, 2005, 9(3): 118–125. DOI: 10.3969/j.issn.1007-7294.2005.03.012.