Analysis on true triaxial mechanical properties of deep marbleby using a discrete element-finite difference coupling method
-
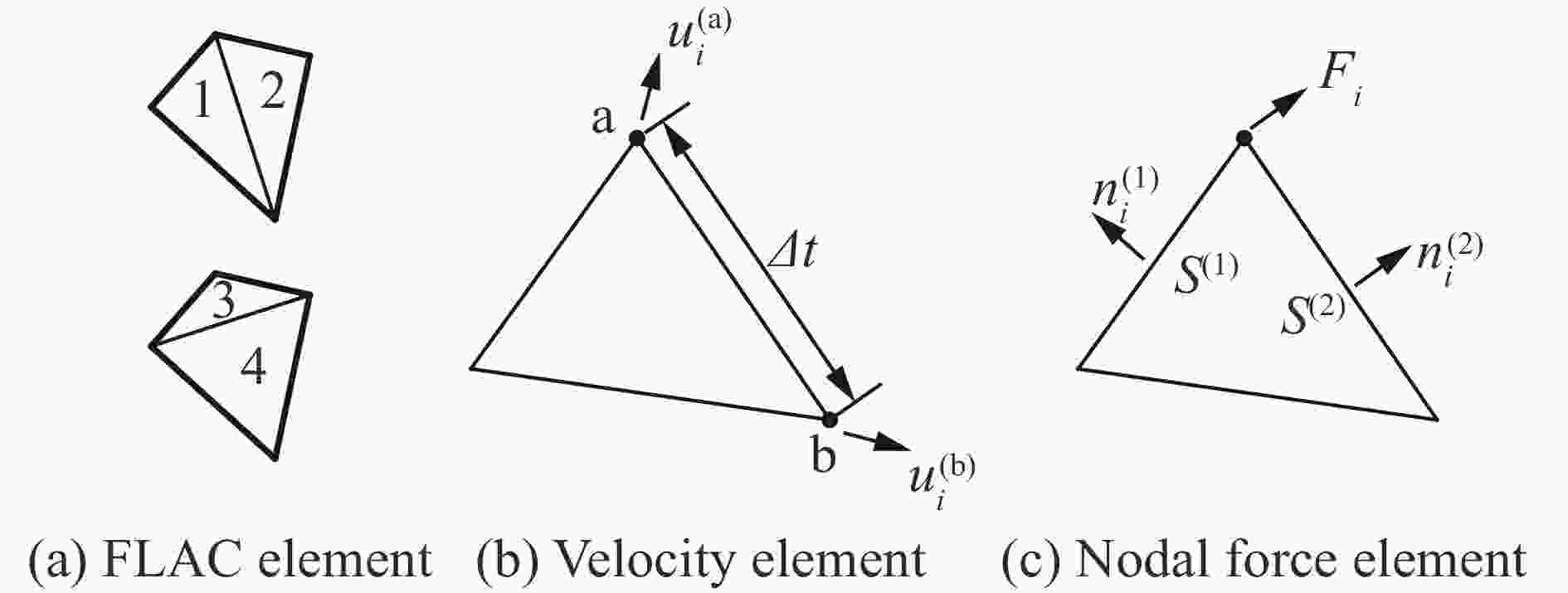
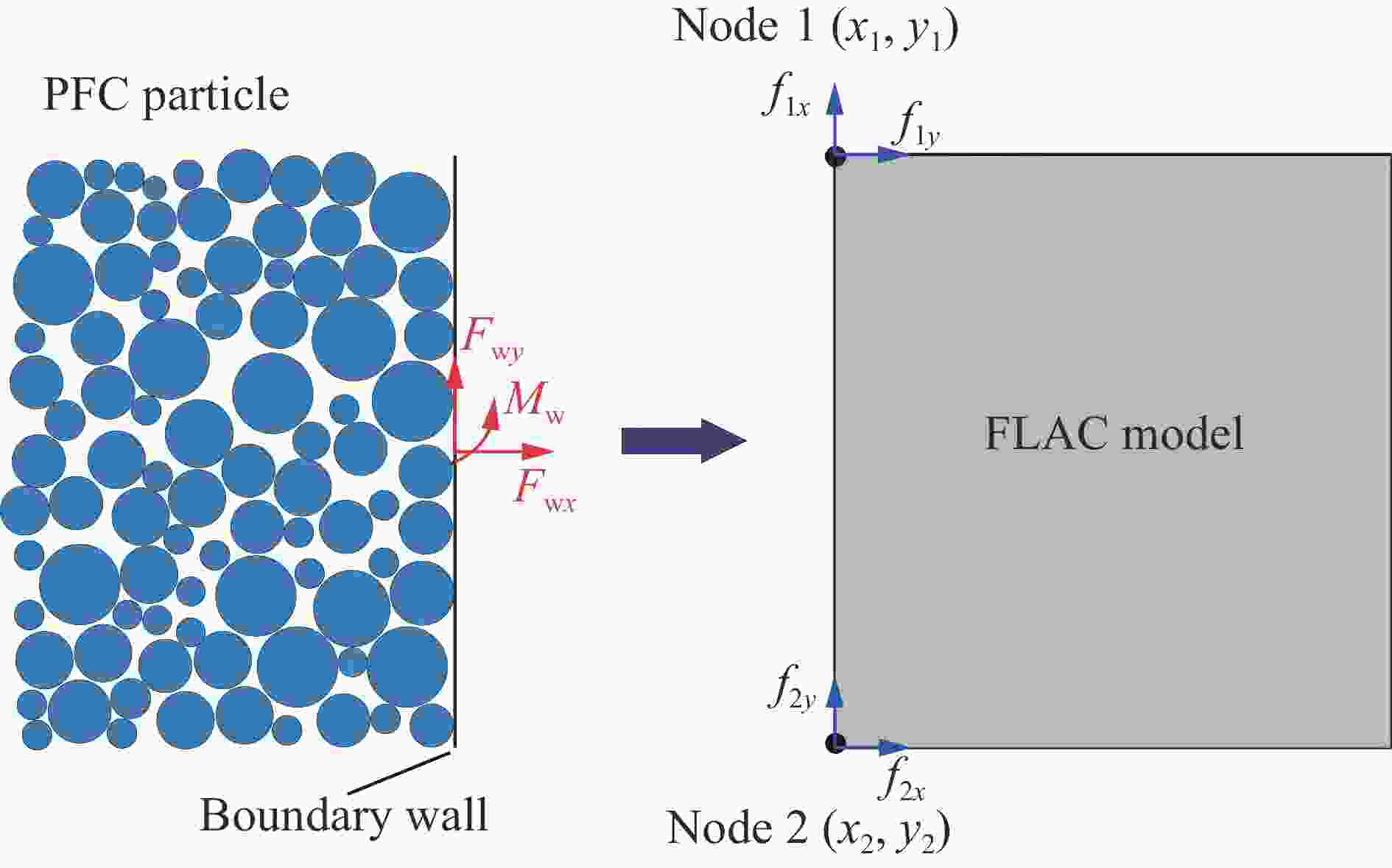
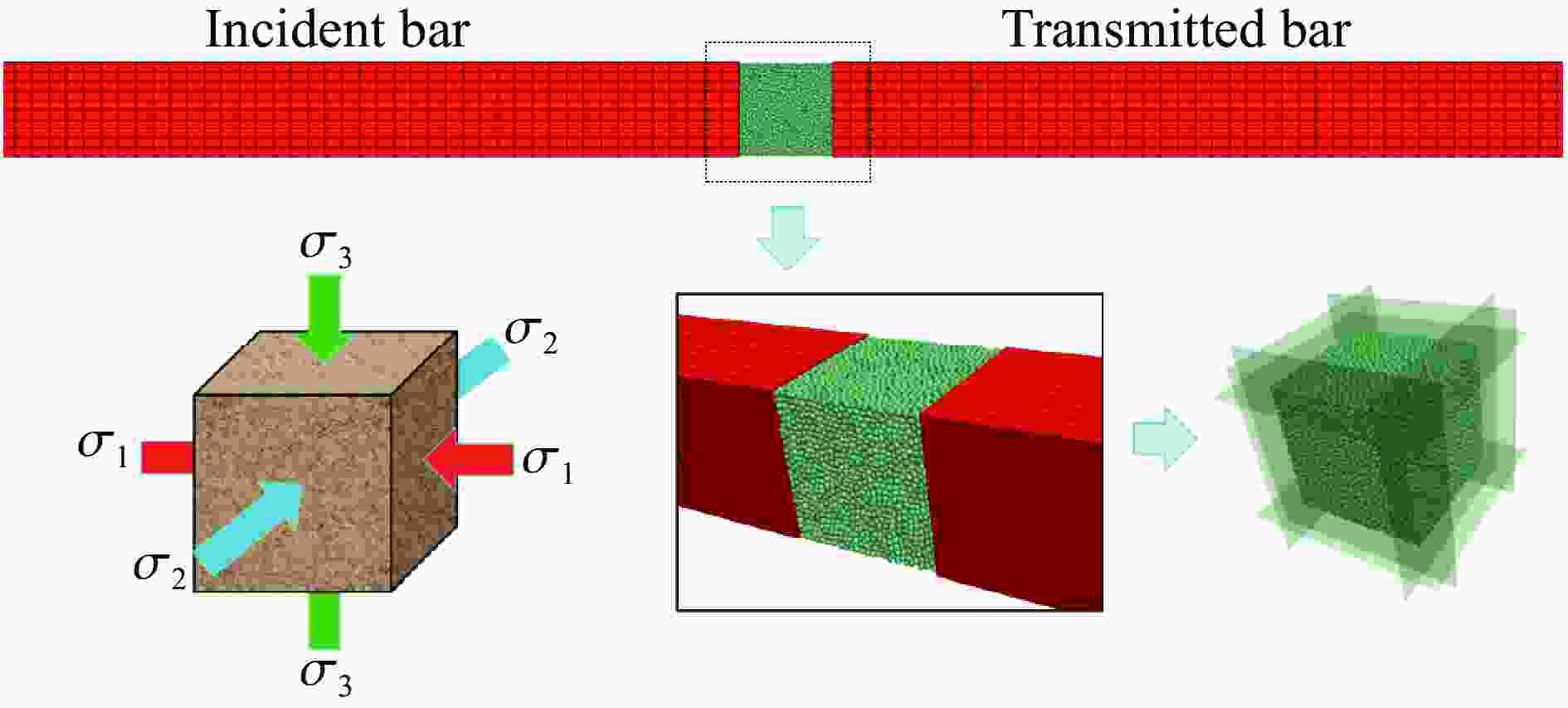
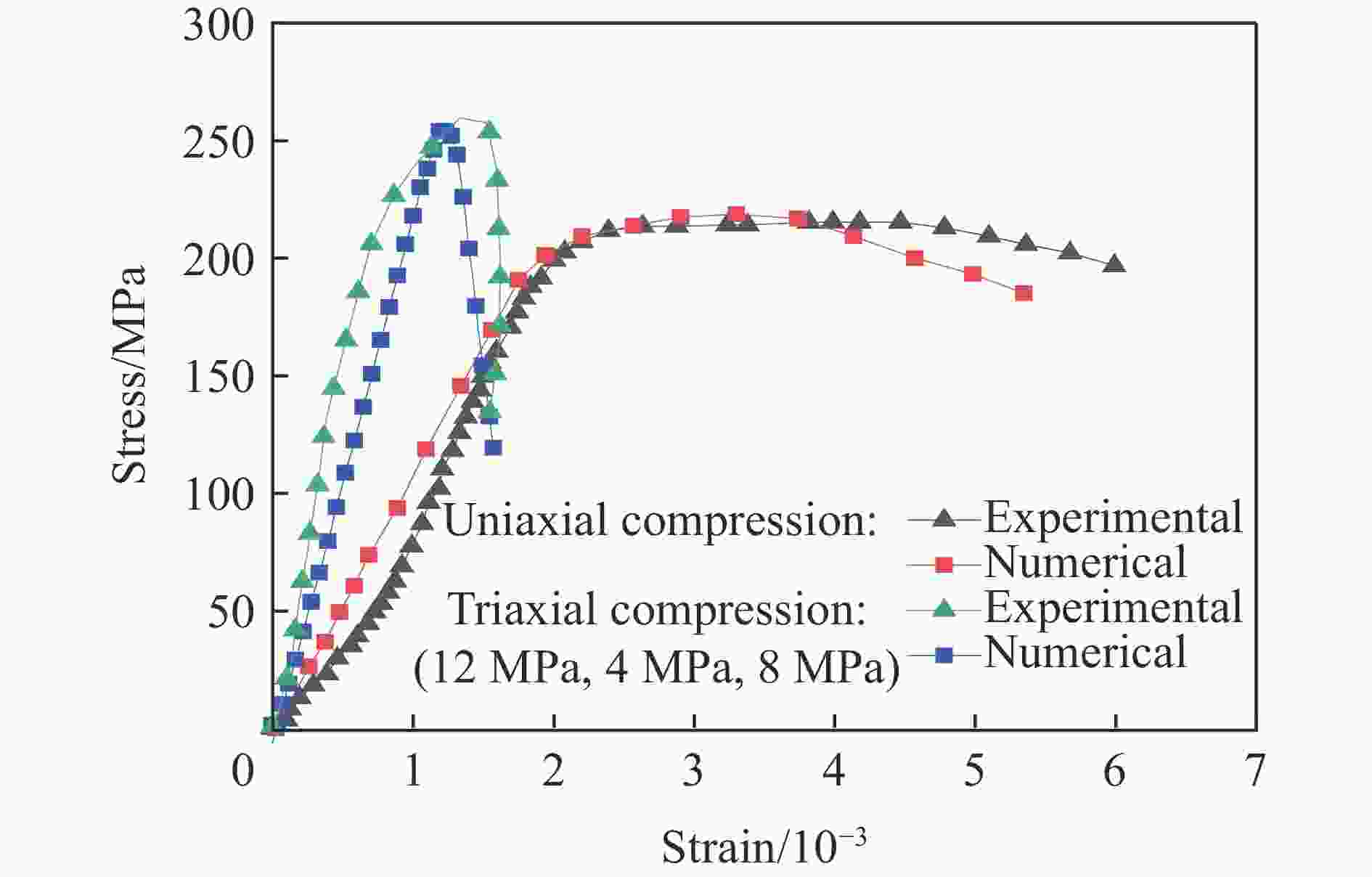
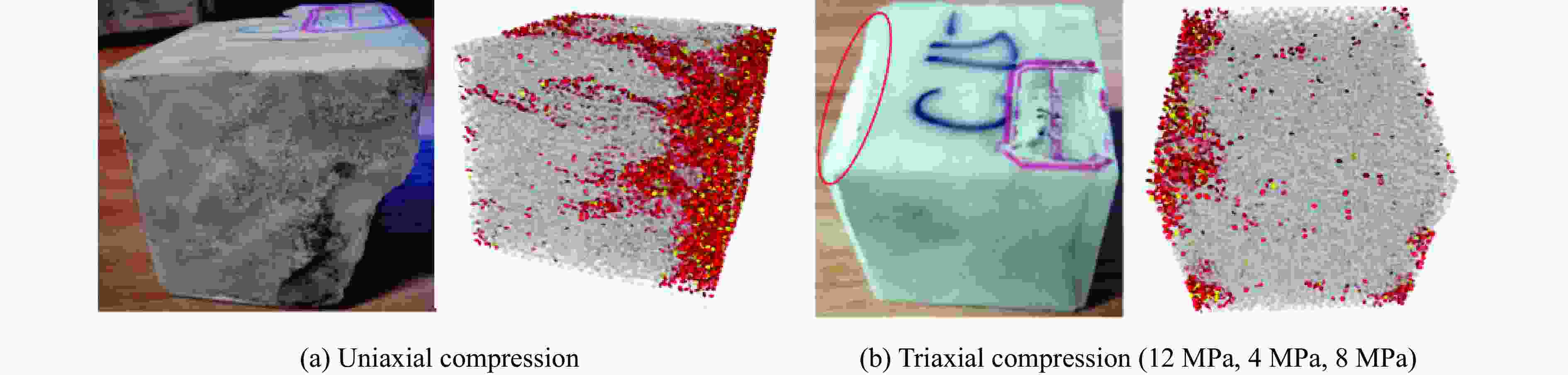
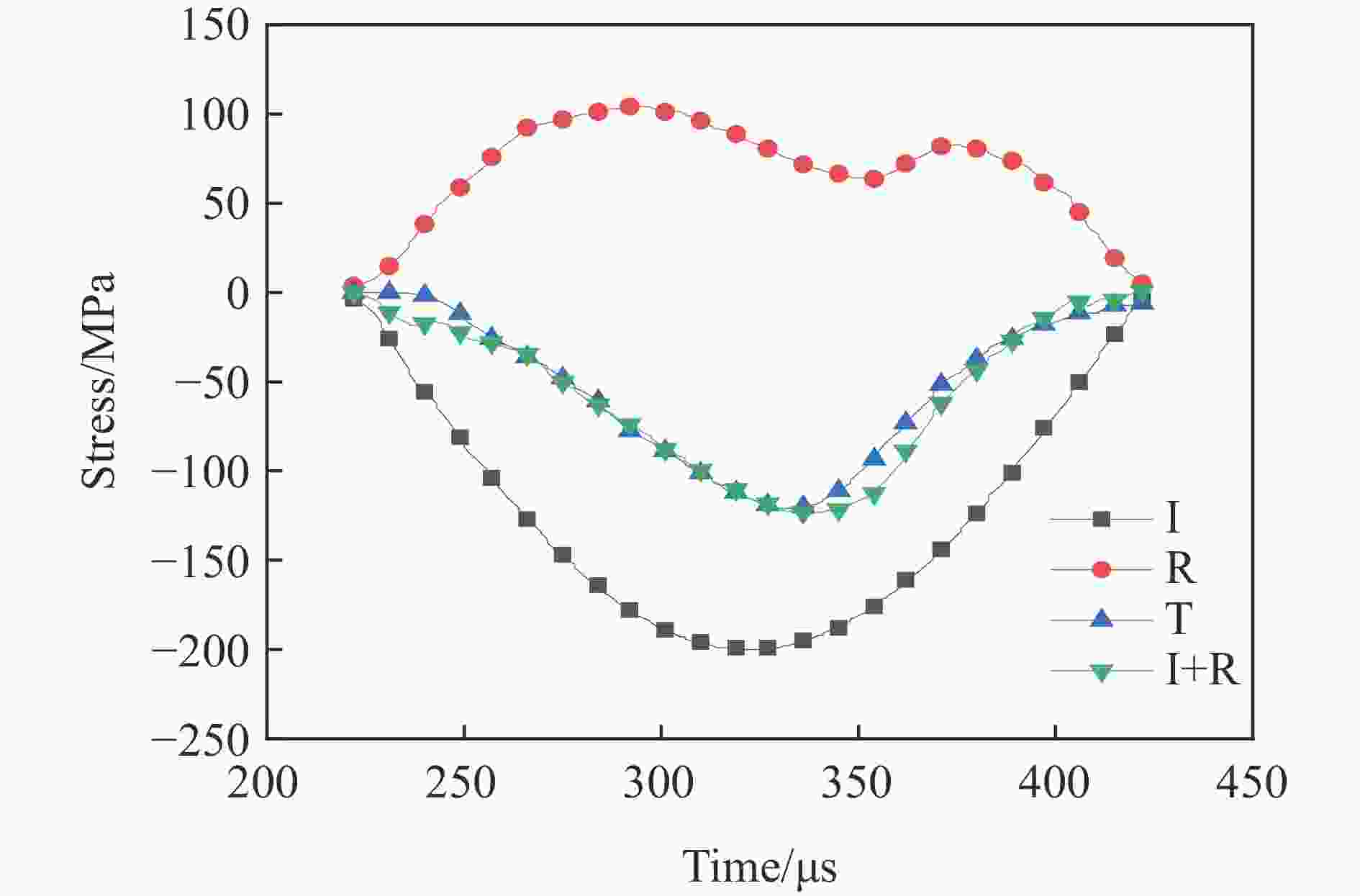
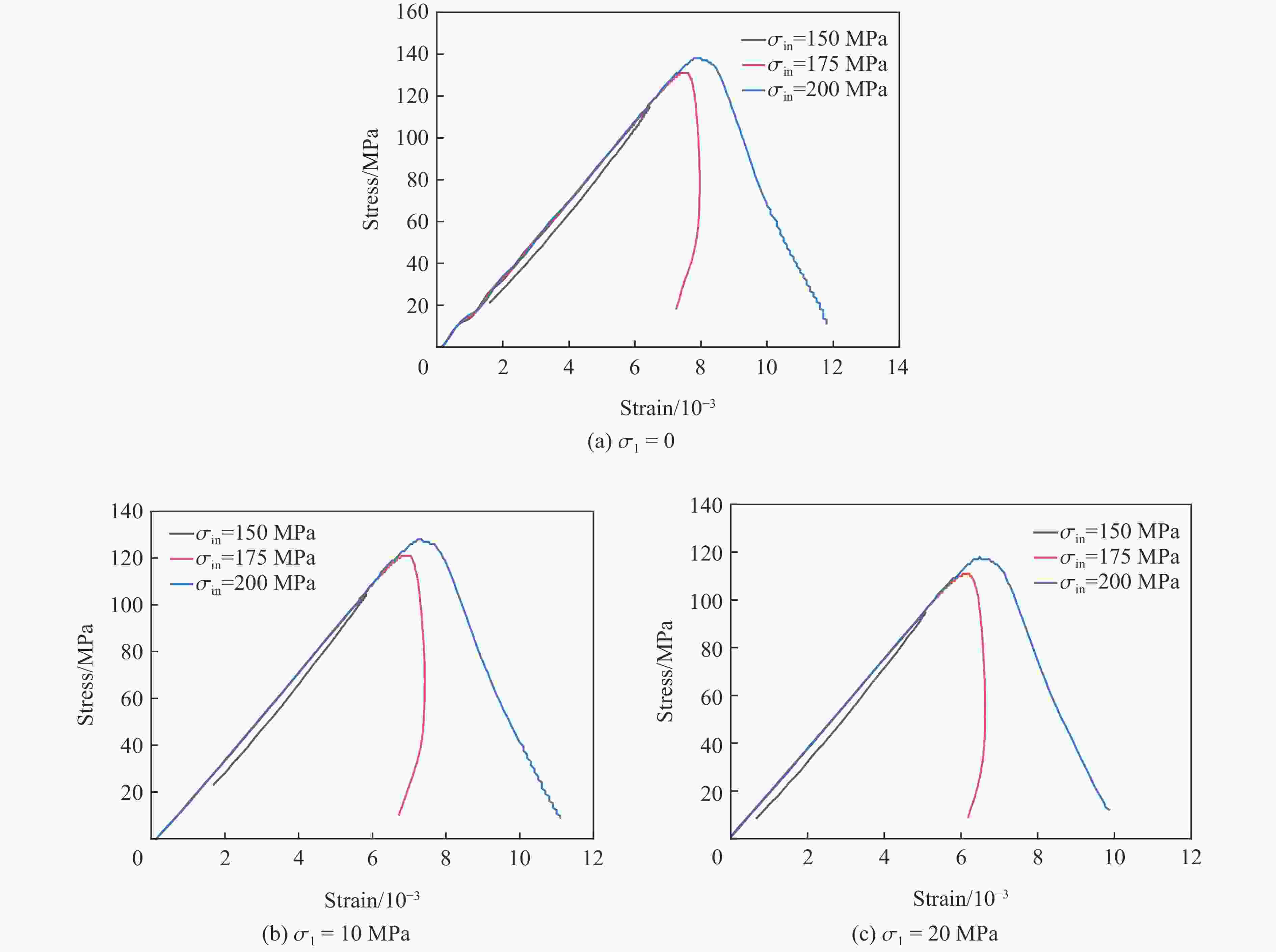
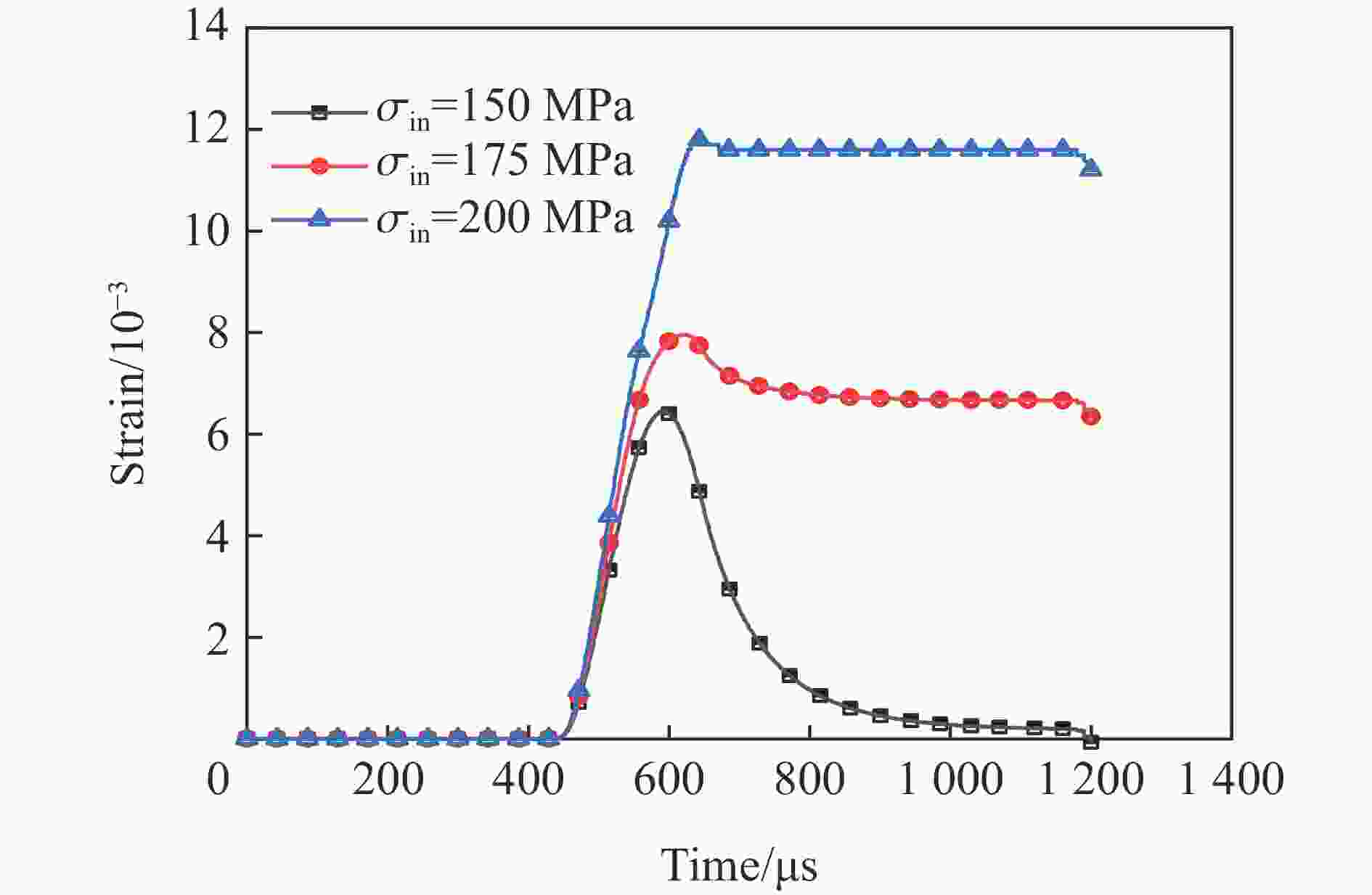
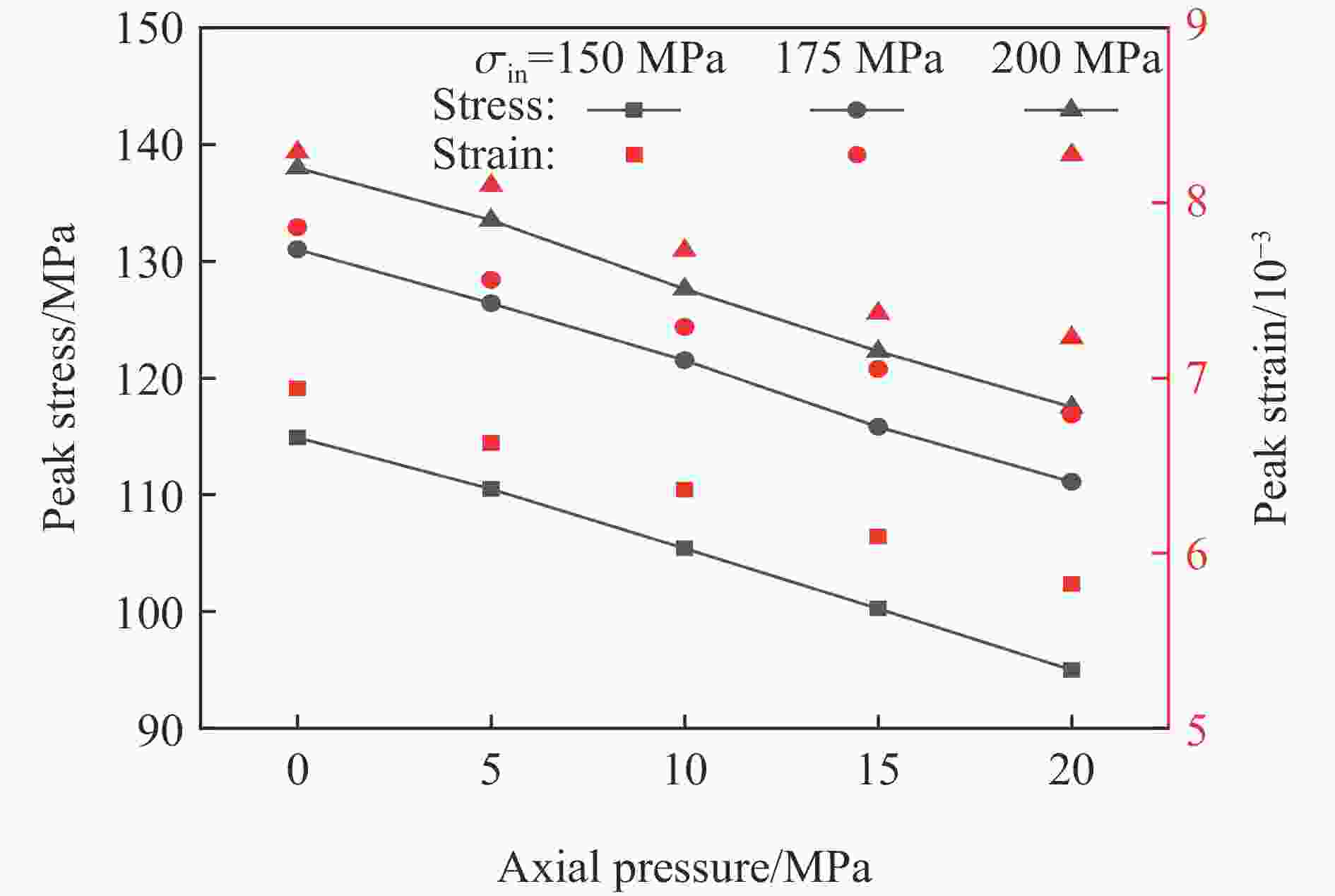
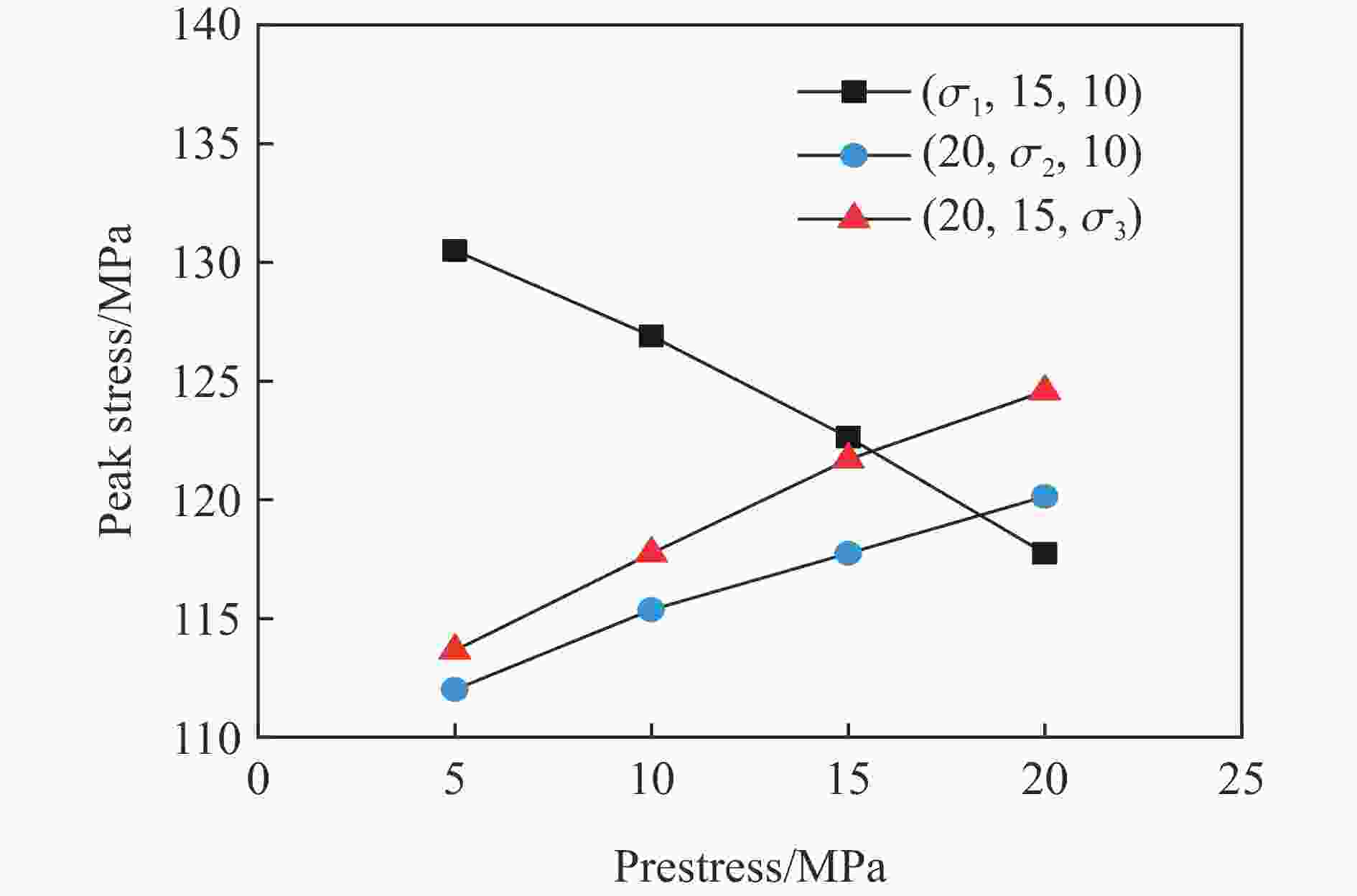
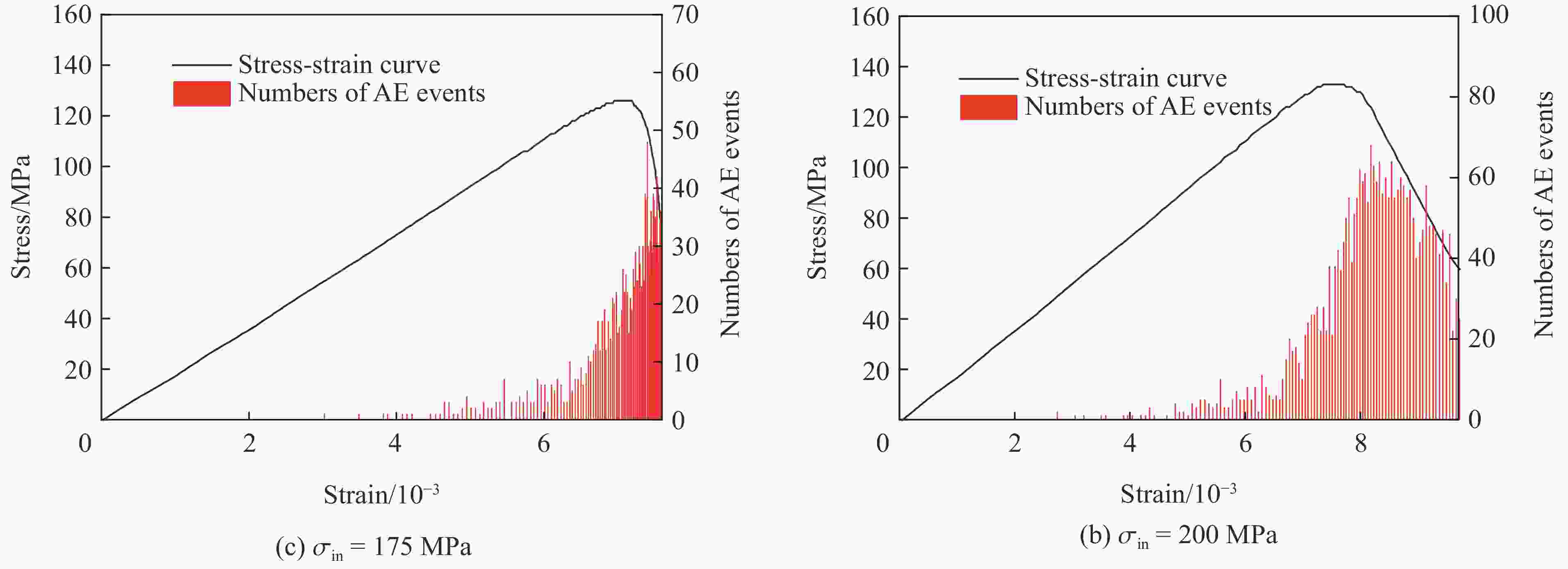
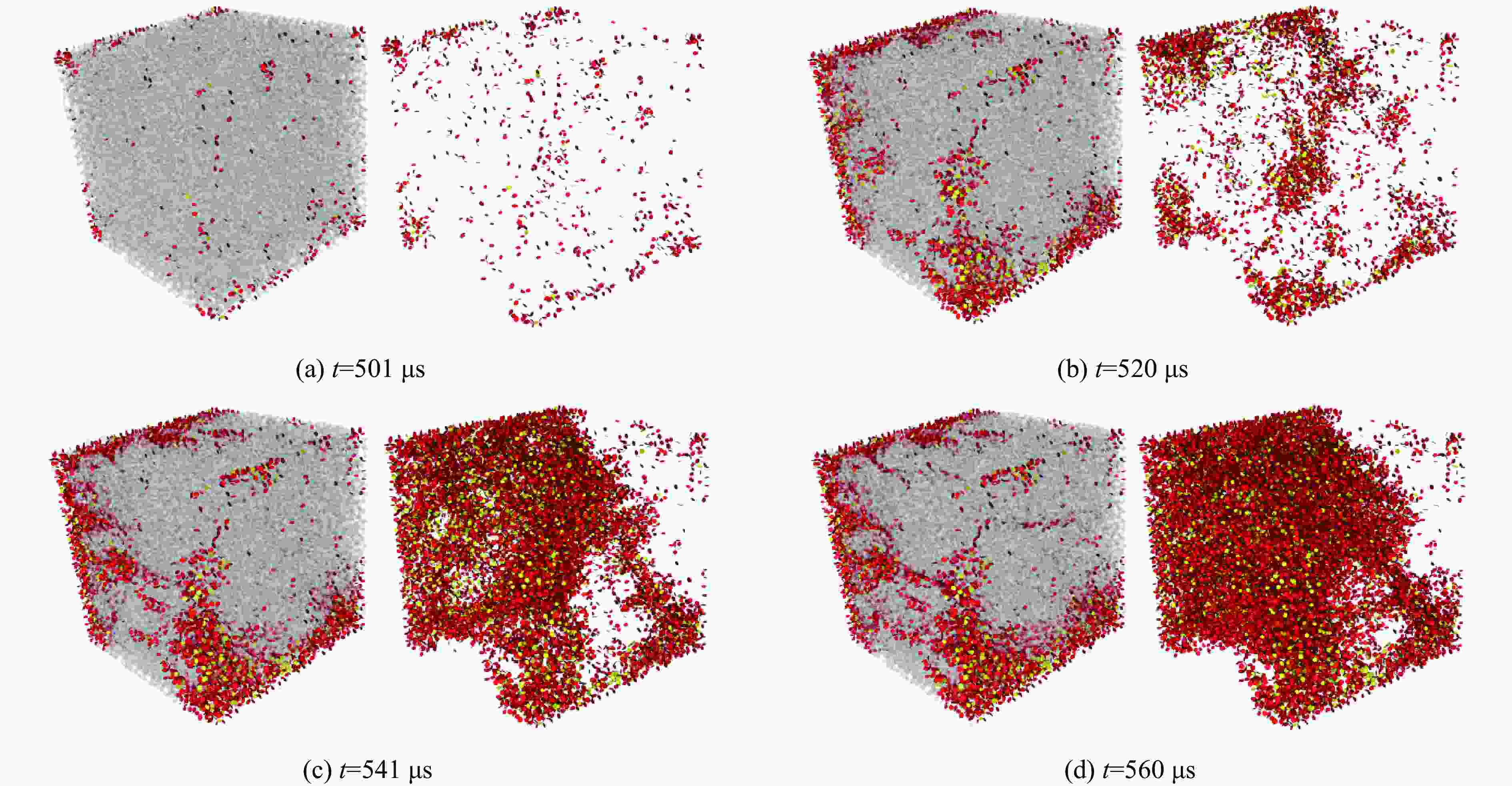
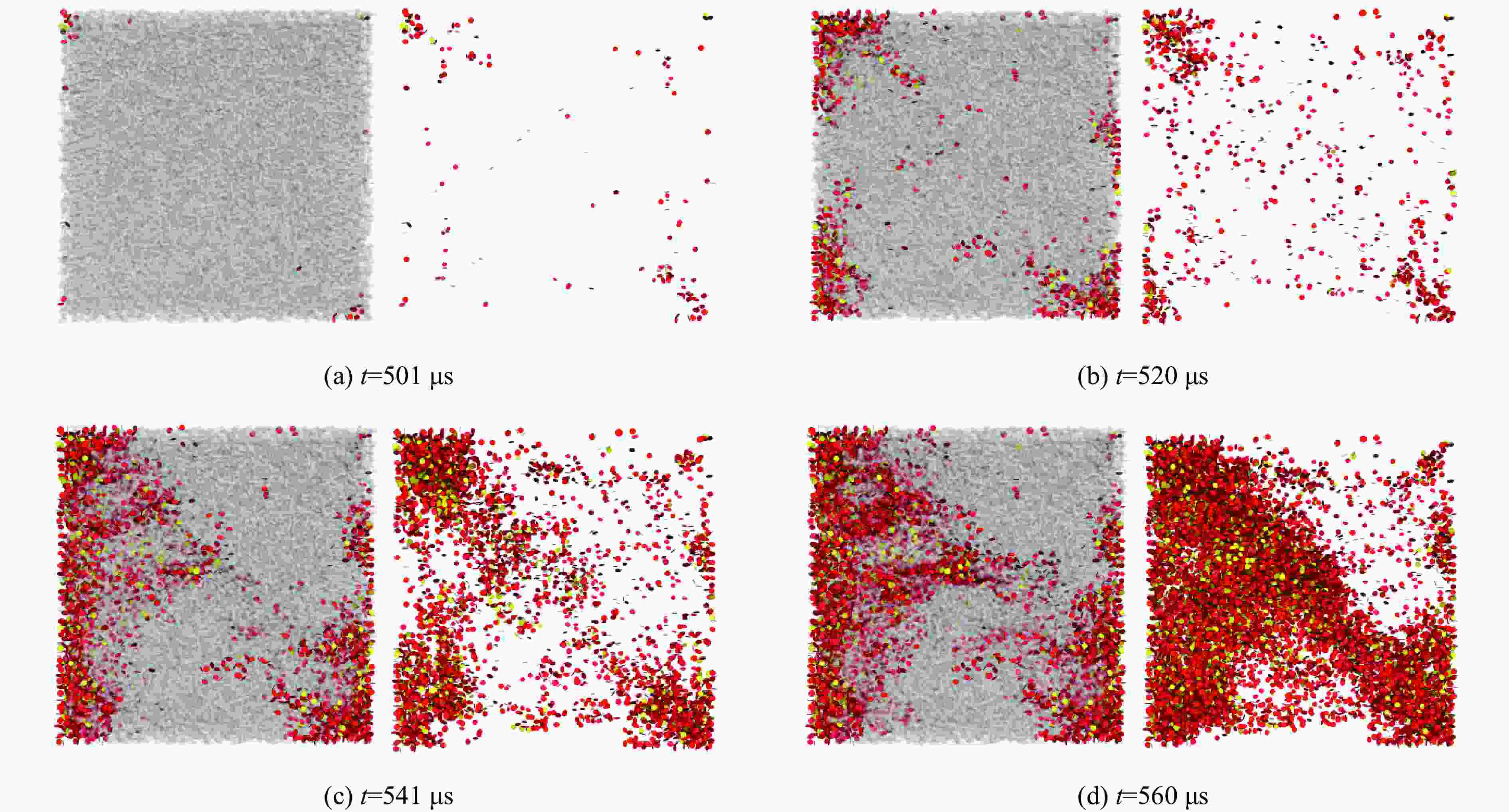
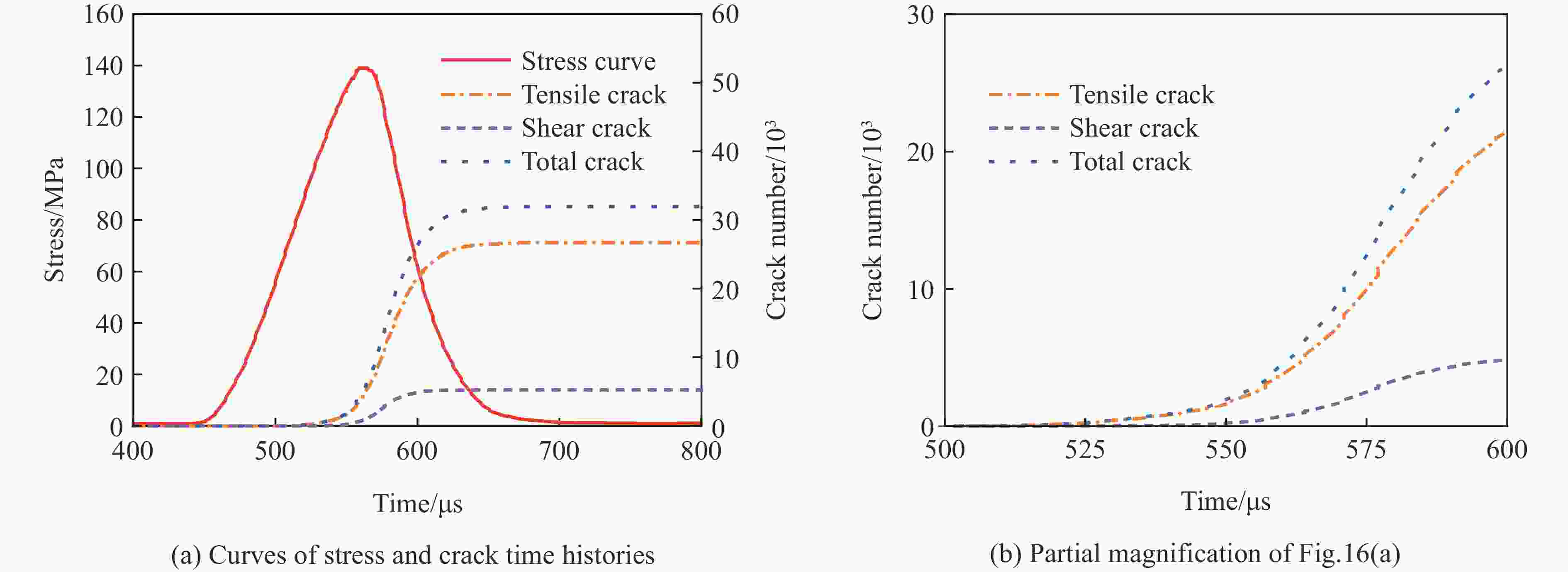
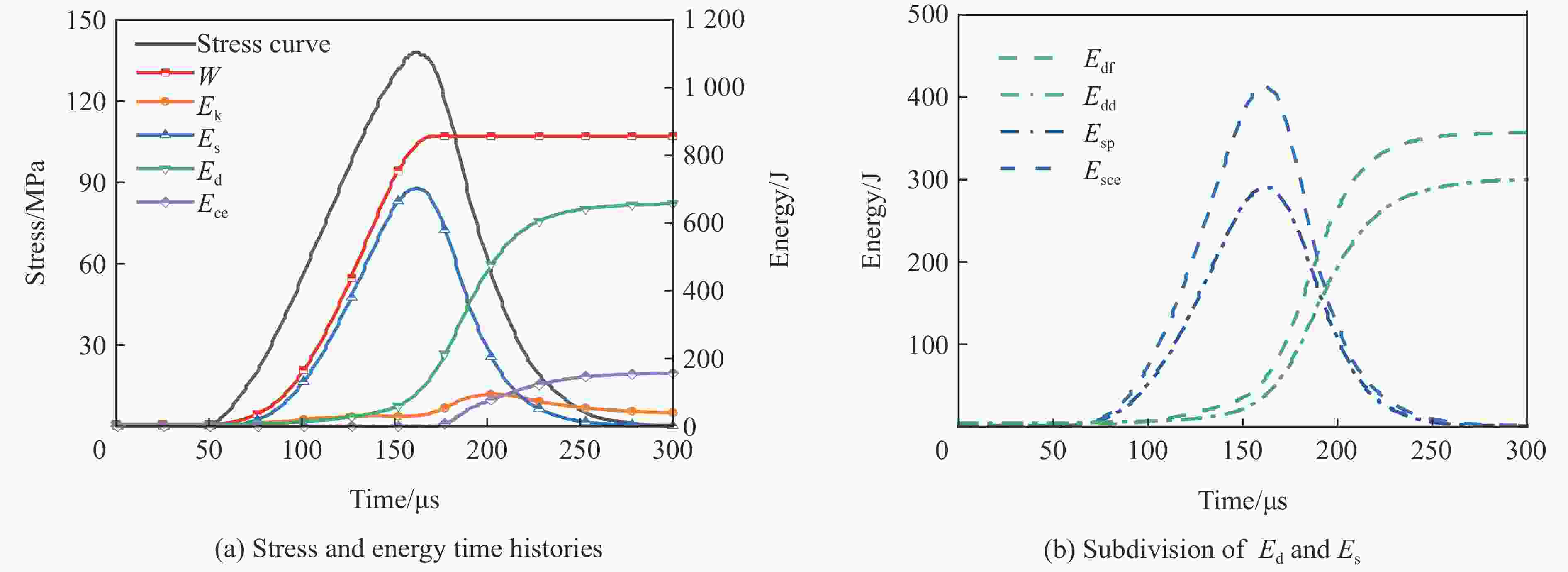
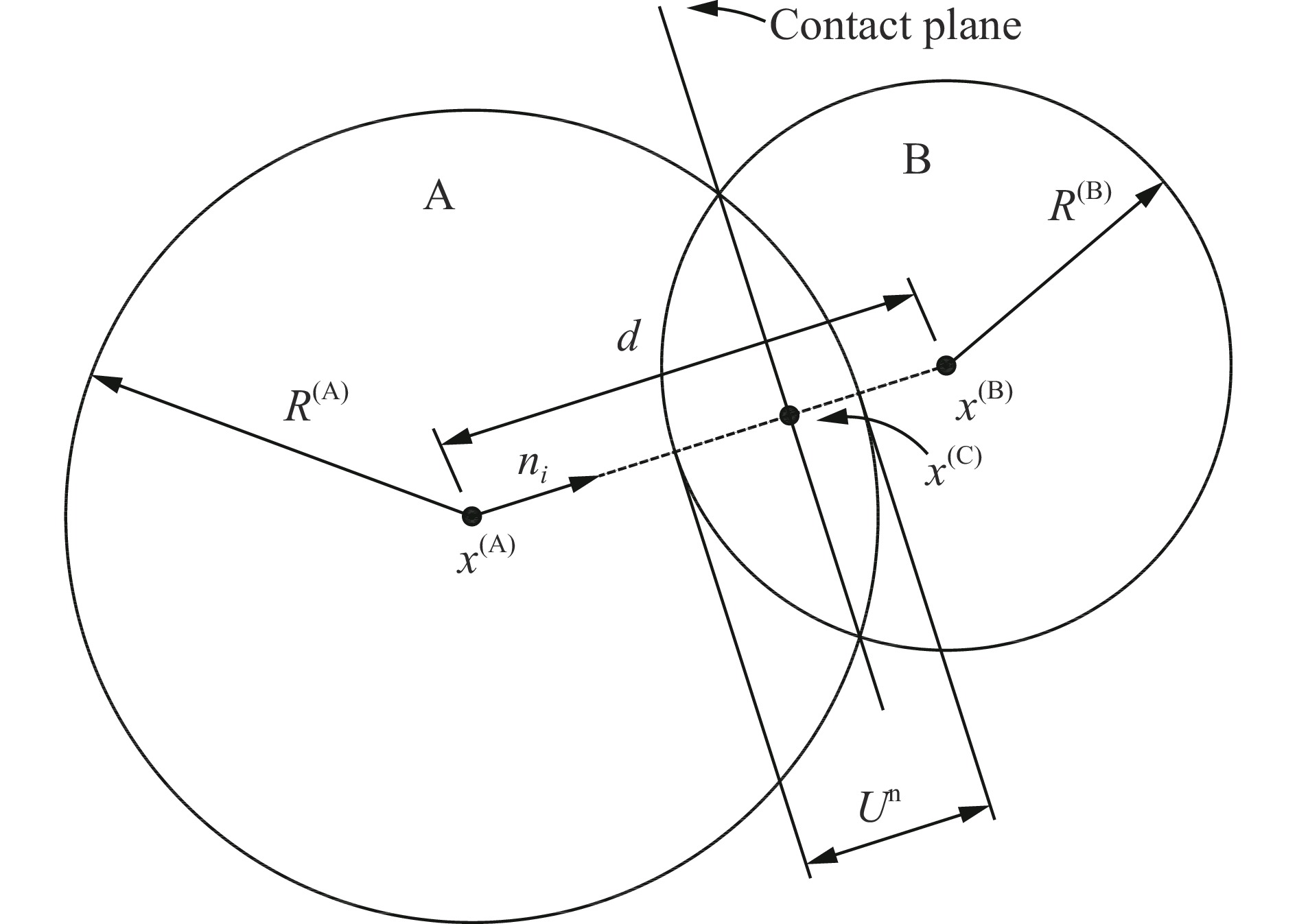
摘要: 为了研究深部大理岩的动态力学特性,首先基于离散元PFC (particle flow code)和有限差分FLAC (fast Lagrangian analysis of continua)耦合法,对大理岩的细观参数进行标定。接着,对三维分离式霍普金森压杆(split Hopkinson pressure bar, SHPB)冲击模拟中的动态应力平衡条件及均匀性假设进行数值验证。最后,对真三轴应力环境下大理岩的应力-应变响应、破碎特征及能量演化机理等问题进行深入分析。结果表明:基于PFC-FLAC耦合理论的真三轴SHPB试验数值结果满足应力均匀性假设,模拟得到的应力-应变曲线与室内试验数据高度一致。峰值应力、峰值应变随着冲击方向上预压(下称“轴向压力”)的增大呈下降趋势。在轴向压力相同时,试样峰值应力增幅随入射应力的提高逐渐变小;当入射应力固定时,轴向压力对试样峰值应力有削弱作用,垂直于冲击方向的侧压则会提升试样的抗压强度。加载过程中声发射事件爆发期整体上发生在应力峰后段,并在此阶段试样内形成较明显的宏观破碎带。在真三轴动态压缩下,大理岩试样主要以拉伸裂纹居多,在总裂纹数中占比超过80%。试样从加载至破坏的过程伴随有能量的变化,达到应力峰值点时试样的应变储能达到极限,之后转化为以耗散能为主、颗粒动能等为辅的能量形式。Abstract: To study the dynamic mechanical properties of deep marble, the micro parameters of deep marble were calibrated based on the coupling method of discrete element (particle flow code, PFC) and finite difference (fast Lagrangian analysis of continua, FLAC). Then, the dynamic stress equilibrium condition and uniformity assumption in the simulated three-dimensional split Hopkinson pressure bar (SHPB) test are numerically validated. Finally, an in-depth analysis is conducted on the stress-strain response, fracture characteristics, and energy evolution mechanism of marble under true triaxial stress environment. It is found that the numerical results of the true triaxial SHPB test based on the PFC-FLAC coupling theory satisfy the assumption of stress uniformity, and the simulated stress-strain curves are highly consistent with the measured ones. Peak stress and peak strain decrease with the increase of pre-pressure in the impact direction (axial pressure hereafter). At the same axial pressure, the peak stress gradually drops down with the increase of incident stress; when the incident stress is fixed, the axial pressure weakens the peak stress of the sample, while the lateral pressure perpendicular to the impact direction increases the compressive strength. During the loading process, the outbreak period of acoustic emission events generally occurs in the post-peak stage, and during this stage, a relatively obvious macroscopic fracture zone is formed within the sample. Under a true triaxial dynamic compression, the samples are mainly characterized by tensile cracks, accounting for over 80% of the total number of cracks. The sample undergoes energy changes from loading to failure. At the peak stress point, the strain energy storage reaches its limit, which is then transformed into an energy form dominated by dissipated energy and supplemented by particle kinetic energy. The relevant conclusions have important guiding significance for the study of the dynamic characteristics of deep marble and the long-term stability evaluation of deep rock engineering.
-
Key words:
- deep marble /
- true triaxial condition /
- mechanical property /
- fragmentation shape /
- coupling analysis
-
表 1 大理岩细观参数
Table 1. Microscopic parameters of marble
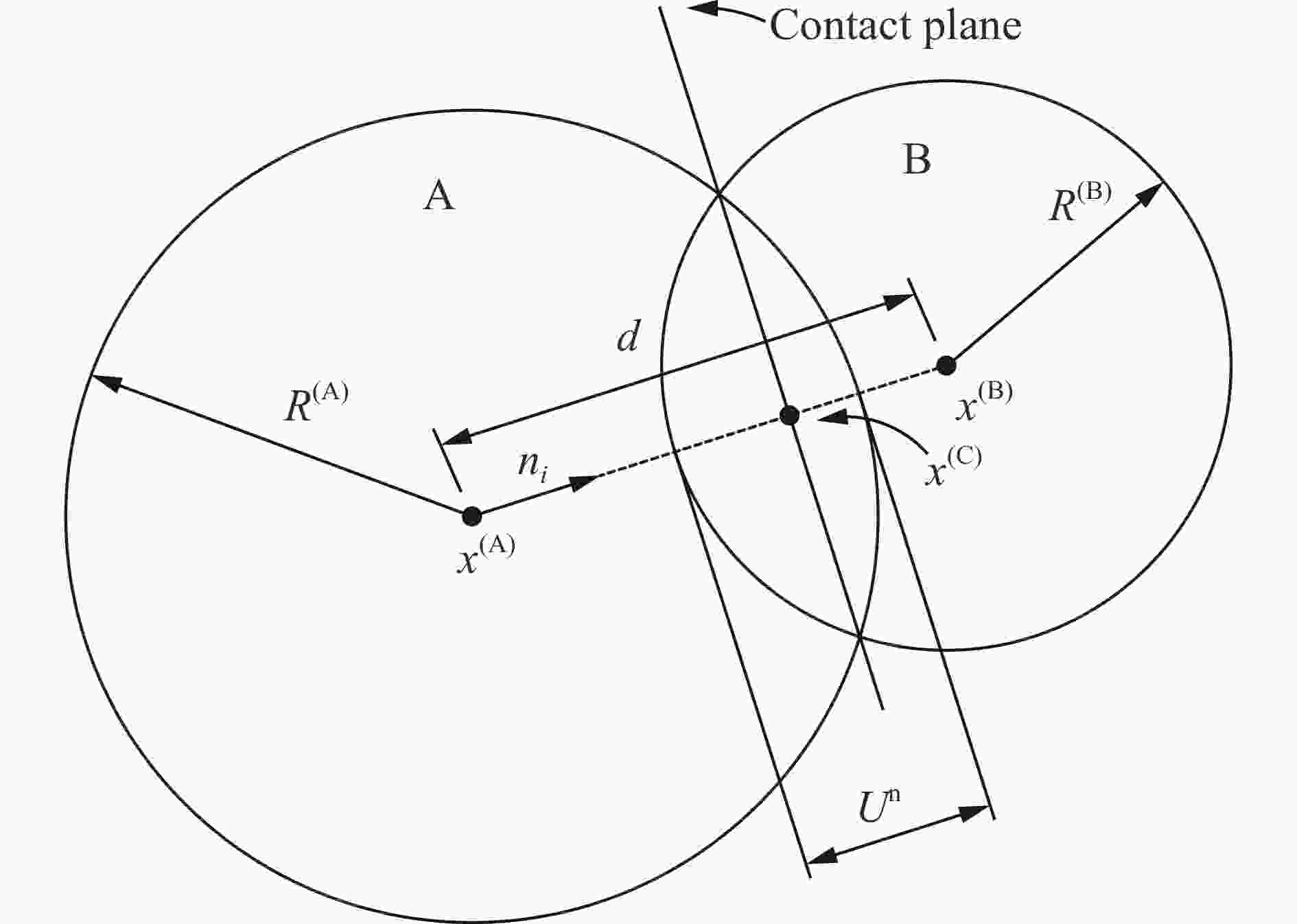
细观参数 含义 标定值 Rmin/mm 最小颗粒半径 0.9 Rmax/Rmin 最大、最小颗粒半径比 1.4 Ec/GPa 颗粒接触模量 30 kn/ks 颗粒刚度比 1.5 ${ \overline{{E}_{\mathrm{c}}}} $/GPa 平行黏结接触模量 10 $ {\overline{{k}_{\mathrm{n}}}/\overline{{k}_{\mathrm{s}}}} $ 平行黏结刚度比 1.5 μ 颗粒摩擦因数 0.5 ${ \overline{{\sigma }_{\mathrm{c}}}} $/MPa 黏结法向强度 70 ${ \overline{{\tau }_{\mathrm{c}}} }$/MPa 黏结切向强度 44 λ 黏结半径乘子 1 -
[1] MA T H, TANG C A, TANG S B, et al. Rockburst mechanism and prediction based on microseismic monitoring [J]. International Journal of Rock Mechanics and Mining Sciences, 2018, 110: 177–188. DOI: 10.1016/j.ijrmms.2018.07.016. [2] SI X F, LI X B, GONG F Q, et al. Experimental investigation on rockburst process and characteristics of a circular opening in layered rock under three-dimensional stress conditions [J]. Tunnelling and Underground Space Technology, 2022, 127: 104603. DOI: 10.1016/j.tust.2022.104603. [3] ZHENG G Q, TANG Y H, ZHANG Y, et al. Study on failure difference of hard rock based on a comparison between the conventional triaxial test and true triaxial test [J]. Frontiers in Earth Science, 2022, 10: 923611. DOI: 10.3389/feart.2022.923611. [4] HAN Z Y, LI D Y, ZHOU T, et al. Experimental study of stress wave propagation and energy characteristics across rock specimens containing cemented mortar joint with various thicknesses [J]. International Journal of Rock Mechanics and Mining Sciences, 2020, 131: 104352. DOI: 10.1016/j.ijrmms.2020.104352. [5] SUN B, CHEN R, PING Y, et al. Dynamic response of rock-like materials based on SHPB pulse waveform characteristics [J]. Materials, 2021, 15(1): 210. DOI: 10.3390/ma15010210. [6] 刘晓辉, 张茹, 刘建锋. 不同应变率下煤岩冲击动力试验研究 [J]. 煤炭学报, 2012, 37(9): 1528–1534. DOI: 10.13225/j.cnki.jccs.2012.09.022.LIU X H, ZHANG R, LIU J F. Dynamic test study of coal rock under different strain rates [J]. Journal of China Coal Society, 2012, 37(9): 1528–1534. DOI: 10.13225/j.cnki.jccs.2012.09.022. [7] 刘晓辉, 薛洋, 郑钰, 等. 冲击荷载下煤岩破碎过程能量释放研究 [J]. 岩石力学与工程学报, 2021, 40(S2): 3201–3211. DOI: 10.13722/j.cnki.jrme.2021.0214.LIU X H, XUE Y, ZHENG Y, et al. Research on energy release in coal rock fragmentation process under impact load [J]. Chinese Journal of Rock Mechanics and Engineering, 2021, 40(S2): 3201–3211. DOI: 10.13722/j.cnki.jrme.2021.0214. [8] LI D Y, HAN Z Y, SUN X L, et al. Dynamic mechanical properties and fracturing behavior of marble specimens containing single and double flaws in SHPB tests [J]. Rock Mechanics and Rock Engineering, 2019, 52(6): 1623–1643. DOI: 10.1007/s00603-018-1652-5. [9] 徐松林, 王鹏飞, 赵坚, 等. 基于三维Hopkinson杆的混凝土动态力学性能研究 [J]. 爆炸与冲击, 2017, 37(2): 180–185. DOI: 10.11883/1001-1455(2017)02-0180-06.XU S L, WANG P F, ZHAO J, et al. Dynamic behavior of concrete under static triaxial loading using 3D-Hopkinson bar [J]. Explosion and Shock Waves, 2017, 37(2): 180–185. DOI: 10.11883/1001-1455(2017)02-0180-06. [10] LUO Y, GONG H L, HUANG J H, et al. Dynamic cumulative damage characteristics of deep-buried granite from Shuangjiangkou hydropower station under true triaxial constraint [J]. International Journal of Impact Engineering, 2022, 165: 104215. DOI: 10.1016/j.ijimpeng.2022.104215. [11] 袁良柱, 苗春贺, 单俊芳, 等. 冲击下混凝土试样应变率效应和惯性效应探讨 [J]. 爆炸与冲击, 2022, 42(1): 013101. DOI: 10.11883/bzycj-2021-0114.YUAN L Z, MIAO C H, SHAN J F, et al. On strain-rate and inertia effects of concrete samples under impact [J]. Explosion and Shock Waves, 2022, 42(1): 013101. DOI: 10.11883/bzycj-2021-0114. [12] XU S L, SHAN J F, ZHANG L, et al. Dynamic compression behaviors of concrete under true triaxial confinement: an experimental technique [J]. Mechanics of Materials, 2020, 140: 103220. DOI: 10.1016/j.mechmat.2019.103220. [13] CHEN M D, XU S L, YUAN L Z, et al. Influence of stress state on dynamic behaviors of concrete under true triaxial confinements [J]. International Journal of Mechanical Sciences, 2023, 253: 108399. DOI: 10.1016/j.ijmecsci.2023.108399. [14] HAERI H, SARFARAZI V, ZHU Z M, et al. The effect of particle size on the edge notched disk (END) using particle flow code in three dimension [J]. Smart Structures and Systems, 2018, 22(6): 663–673. DOI: 10.12989/sss.2018.22.6.663. [15] CHANG L F, KONIETZKY H. Application of the Mohr-Coulomb yield criterion for rocks with multiple joint sets using fast Lagrangian analysis of continua 2D (FLAC2D) software [J]. Energies, 2018, 11(3): 614. DOI: 10.3390/en11030614. [16] JIA M C, YANG Y, LIU B, et al. PFC/FLAC coupled simulation of dynamic compaction in granular soils [J]. Granular Matter, 2018, 20(4): 76. DOI: 10.1007/s10035-018-0841-y. [17] 丛怡, 丛宇, 张黎明, 等. 大理岩加、卸荷破坏过程的三维颗粒流模拟 [J]. 岩土力学, 2019, 40(3): 1179–1186, 1212. DOI: 10.16285/j.rsm.2018.0262.CONG Y, CONG Y, ZHANG L M, et al. 3D particle flow simulation of loading-unloading failure process of marble [J]. Rock and Soil Mechanics, 2019, 40(3): 1179–1186, 1212. DOI: 10.16285/j.rsm.2018.0262. [18] 牛林新, 辛酉阳. 基于正交设计的颗粒流模型宏细观参数相关分析: 以岩石单轴压缩数值试验为例 [J]. 人民长江, 2015, 46(16): 53–57, 71. DOI: 10.16232/j.cnki.1001-4179.2015.16.013.NIU L X, XIN Y Y. Analysis on relationship between macro-parameters and micro-parameters in PFC2D model based on orthogonal design: case of rock uniaxial compression numerical test [J]. Yangtze River, 2015, 46(16): 53–57, 71. DOI: 10.16232/j.cnki.1001-4179.2015.16.013. [19] 丛宇, 王在泉, 郑颖人, 等. 基于颗粒流原理的岩石类材料细观参数的试验研究 [J]. 岩土工程学报, 2015, 37(6): 1031–1040. DOI: 10.11779/CJGE201506009.CONG Y, WANG Z Q, ZHENG Y R, et al. Experimental study on microscopic parameters of brittle materials based on particle flow theory [J]. Chinese Journal of Geotechnical Engineering, 2015, 37(6): 1031–1040. DOI: 10.11779/CJGE201506009. [20] ZHAO R, TAO M, WU C Q, et al. Study on size and load rate effect of dynamic fragmentation and mechanical properties of marble sphere [J]. Engineering Failure Analysis, 2022, 142: 106814. DOI: 10.1016/j.engfailanal.2022.106814. [21] LIU K, ZHANG Q B, WU G, et al. Dynamic mechanical and fracture behaviour of sandstone under multiaxial loads using a triaxial Hopkinson bar [J]. Rock Mechanics and Rock Engineering, 2019, 52(7): 2175–2195. DOI: 10.1007/s00603-018-1691-y. [22] QI C Z, WANG M Y, WANG Z F, et al. Study on the coupling effect of sample size and strain rate on rock compressive strength [J]. Rock Mechanics and Rock Engineering, 2023, 56(7): 5103–5114. DOI: 10.1007/s00603-023-03309-z. [23] HU W R, LIU K, POTYONDY D O, et al. 3D continuum-discrete coupled modelling of triaxial Hopkinson bar tests on rock under multiaxial static-dynamic loads [J]. International Journal of Rock Mechanics and Mining Sciences, 2020, 134: 104448. DOI: 10.1016/j.ijrmms.2020.104448. [24] 许东俊, 耿乃光. 岩石强度随中间主应力变化规律 [J]. 固体力学学报, 1985, 6(1): 72–80. DOI: 10.19636/j.cnki.cjsm42-1250/o3.1985.01.007.XU D J, GENG N G. The variation law of rock strength with increase of intermediate principal stress [J]. Acta Mechanica Solida Sinica, 1985, 6(1): 72–80. DOI: 10.19636/j.cnki.cjsm42-1250/o3.1985.01.007. [25] 周喻, 吴顺川, 许学良, 等. 岩石破裂过程中声发射特性的颗粒流分析 [J]. 岩石力学与工程学报, 2013, 32(5): 951–959. DOI: 10.3969/j.issn.1000-6915.2013.05.013.ZHOU Y, WU S C, XU X L, et al. Particle flow analysis of acoustic emission characteristics during rock failure process [J]. Chinese Journal of Rock Mechanics and Engineering, 2013, 32(5): 951–959. DOI: 10.3969/j.issn.1000-6915.2013.05.013. [26] SONG B, CHEN W. Energy for specimen deformation in a split Hopkinson pressure bar experiment [J]. Experimental Mechanics, 2006, 46(3): 407–410. DOI: 10.1007/s11340-006-6420-x. -






 下载:
下载: