Optimization of detonation parameters for multi-point aggregated explosion effects in concrete
-
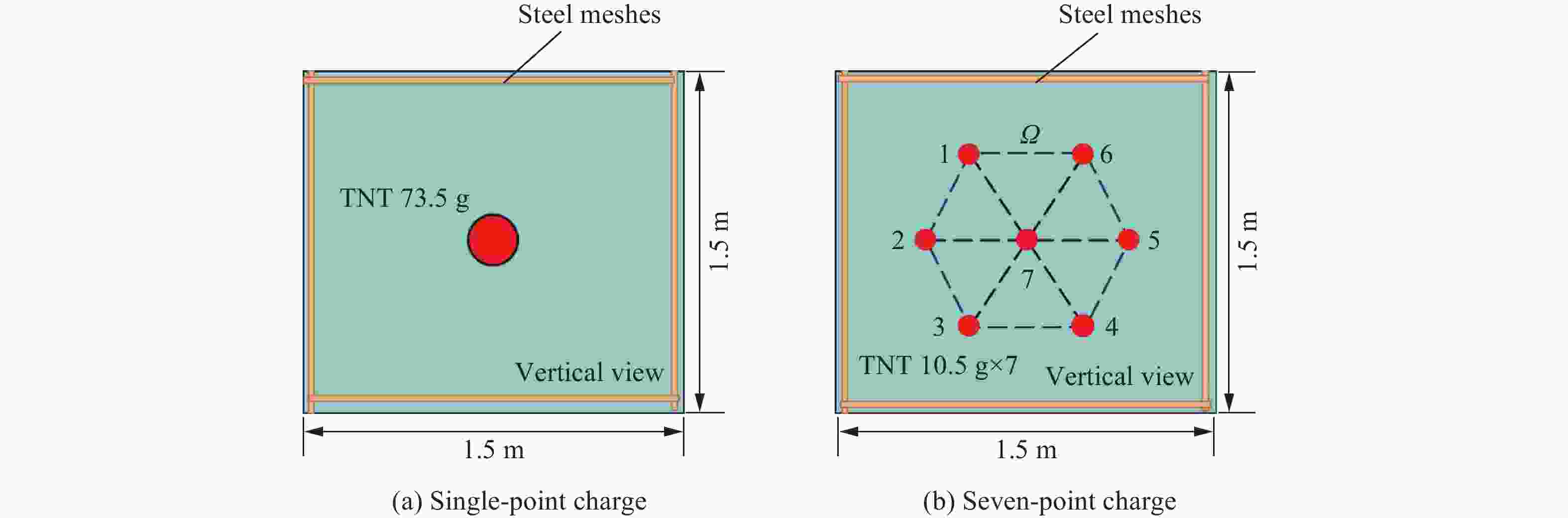
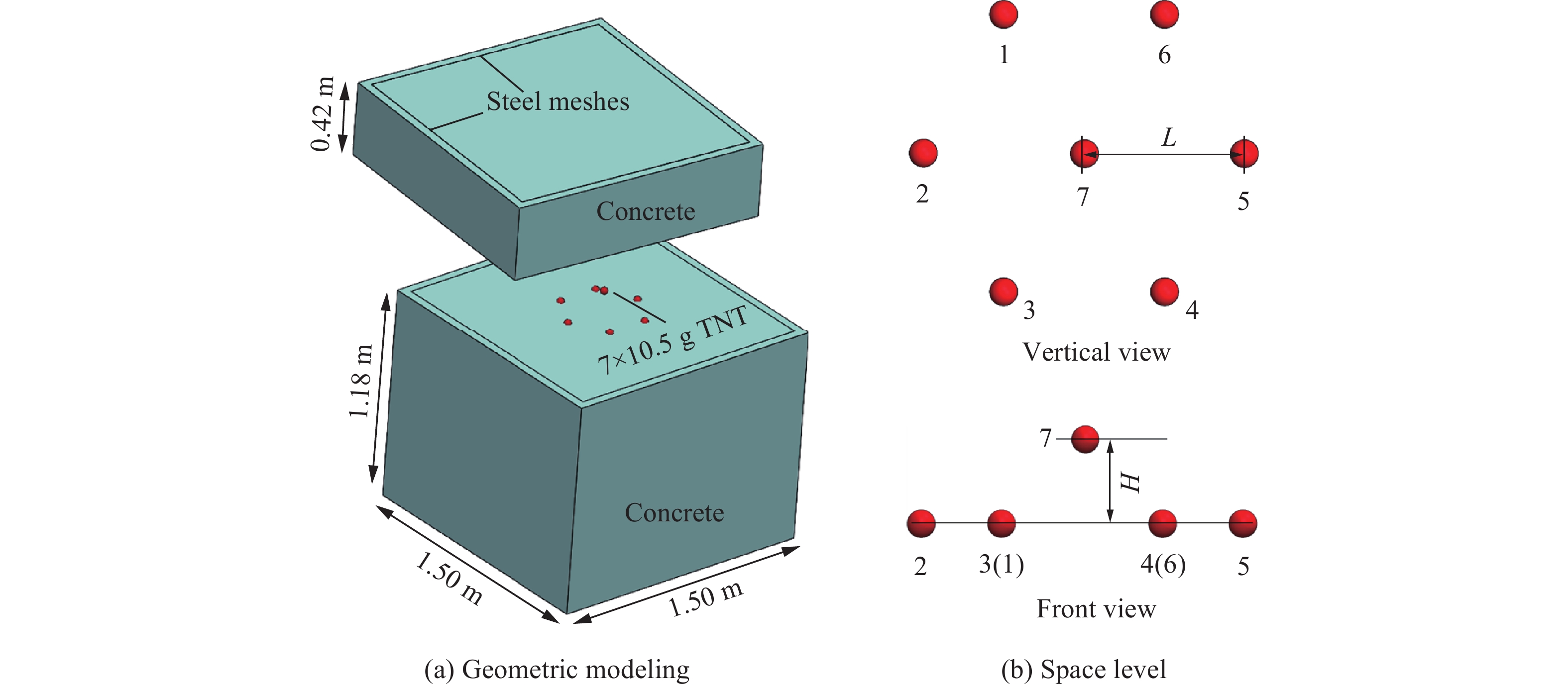
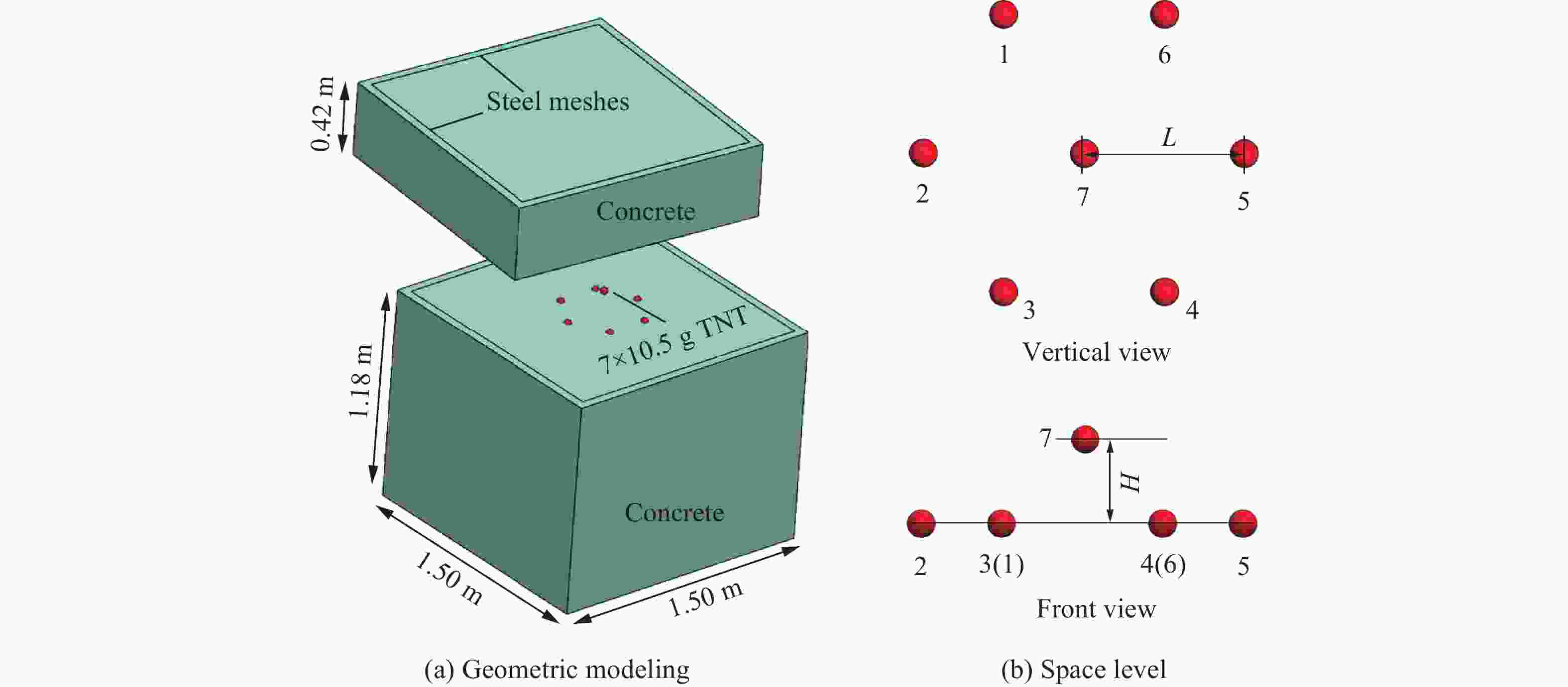
摘要: 混凝土介质中多点同时或彼此微差爆炸可产生复杂的地冲击波叠加聚集效应,从而使特定作用区域内的地冲击波压力显著增强,大大提升爆炸的毁伤威力。为获取多点爆源不同排布方式下爆炸聚集效应及地冲击传播衰减规律,进行了混凝土中单点和七点聚集爆炸的现场和数值模拟试验,基于正交设计方法和灰色系统理论对多点起爆参数进行了优化设计,建立了比例装药间距、比例有源装药高度和比例起爆微差等因素与不同爆心距下峰值压力间的灰色关联度系数及灰色关联度,确定了起爆参数的优选组合,并开展了数值模拟试验检验。分析结果表明:影响地冲击聚集效应的主要因素为比例装药间距,其次为比例起爆微差,最次为比例有源装药高度。在本模拟试验情况下,采用优化的起爆参数时,即在比例装药间距为0.549 m/kg1/3、比例起爆微差为0.239 m/kg1/3和比例有源装药高度为0 m/kg1/3时,地冲击波聚集效应达到最佳,最大可达单点同等装药量产生的地冲击压力的4.7倍。Abstract: Simultaneous or slightly different explosions at multiple points in concrete medium can generate a complex superposition and aggregation effect of ground shock waves, significantly enhancing the pressure of ground shock waves in a specific area and greatly improving the destructive power of the explosion. To obtain the explosion aggregation effect and ground shock propagation attenuation law under the different arrangement of multi-point explosive sources, field tests were first carried out on single and seven-point aggregated explosions in concrete. Then, the reliability of the RHT material model parameters and the SPH numerical algorithm are verified based on experimental data. On this basis the orthogonal design method and gray system theory on the multi-point detonation parameters are adopted for design optimization. Gray correlation coefficients and gray correlations between scaled charge spacing, scaled active charge height, scaled detonation time difference and peak pressure at different proportional bursting center distances are established. Finally, by carrying out single-objective factor optimization and multi-objective factor optimization, a set of preferred combinations of the factors is determined, and simulation tests are conducted to verify the results. The analysis results show that the RHT model of concrete material and the SPH algorithm can reasonably predict the shock wave propagation attenuation characteristics of multipoint charge explosions at different scaled bursting center distances as well as the induced damage and destruction of concrete. The main factors affecting the impact of the ground shock aggregation of explosive effect, in order of magnitude, are: scaled charge spacing, scaled detonation time difference and scaled active charge height. Under the conditions of the present test, the optimized detonation parameters are found as: the proportional charge spacing is 0.549 m/kg1/3, the proportional detonation time difference is 0.239 m/kg1/3, the proportional active charge height is 0. This set of parameters will result in the best ground shock aggregation effect, being up to 4.7 times the ground shock pressure produced by the same amount of single-point group charging.
-
Key words:
- gray theory /
- aggregated explosion /
- concrete /
- degree of association /
- optimal design
-
ρ0/(g·cm−3) A/GPa B/GPa R1 R2 ω D/(km·s−1) E0/GPa 1.63 373.77 3.7471 4.15 0.90 0.35 6.93 6.0 表 2 试验与数值模拟得到的弹坑尺寸
Table 2. Crater dimensions by test and numerical simulation
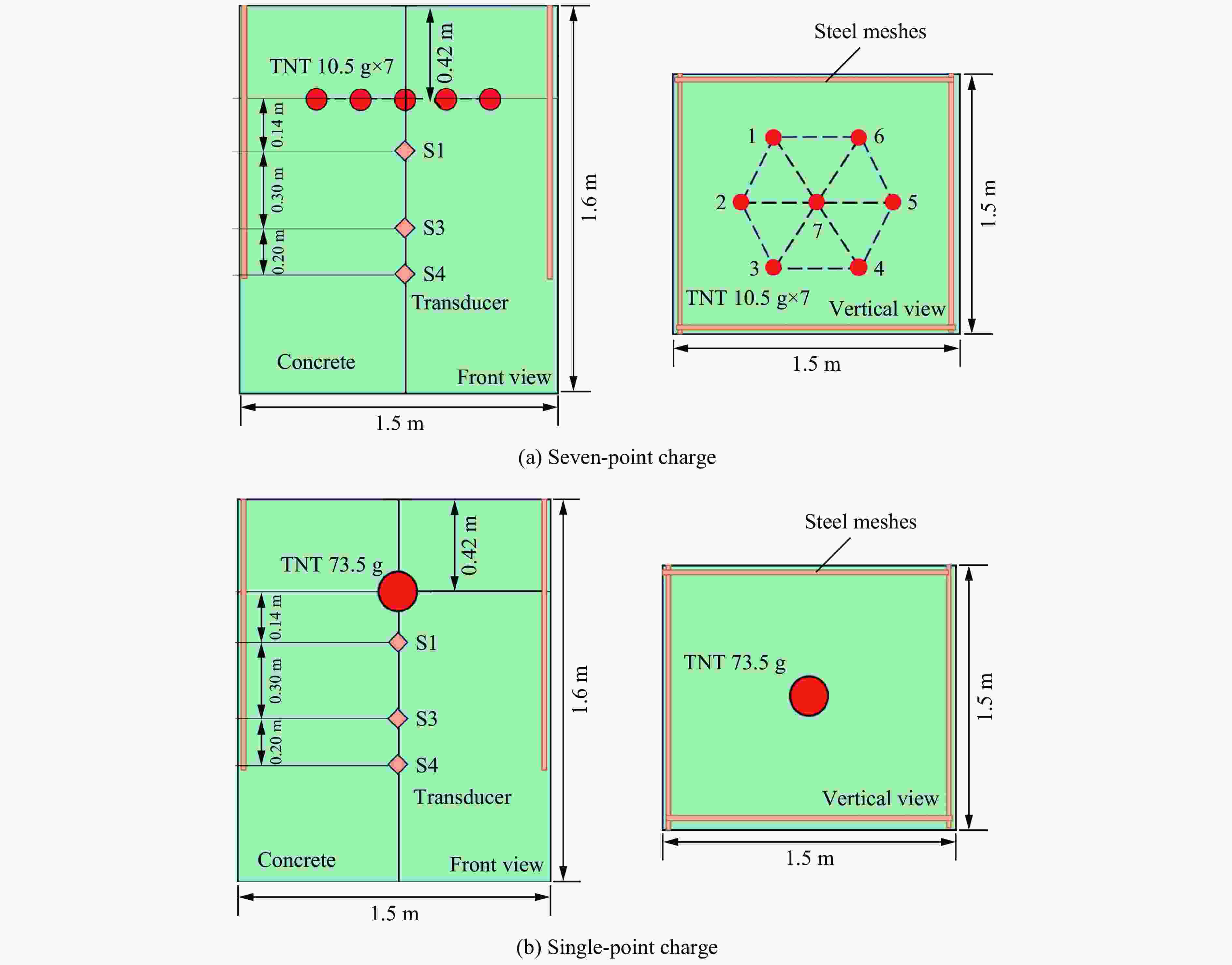
工况 弹坑的深度 弹坑的直径 模拟值/m 试验值/m 偏差/% 模拟值/m 试验值/m 偏差/% 七点爆炸 0.441 0.430 2.56 0.748 0.755 −0.93 单点爆炸 0.530 0.490 8.16 0.225 0.200 12.50 表 3 试验与数值荷载峰值比较
Table 3. Comparison of experimental and numerical peak loads
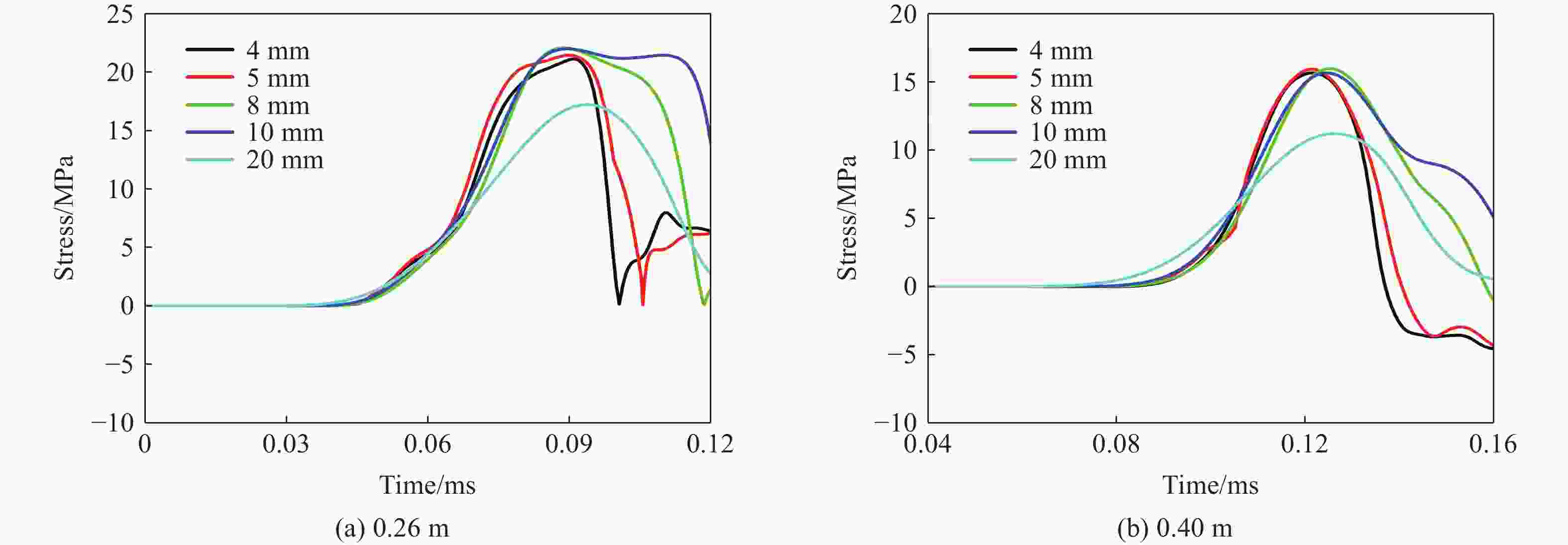
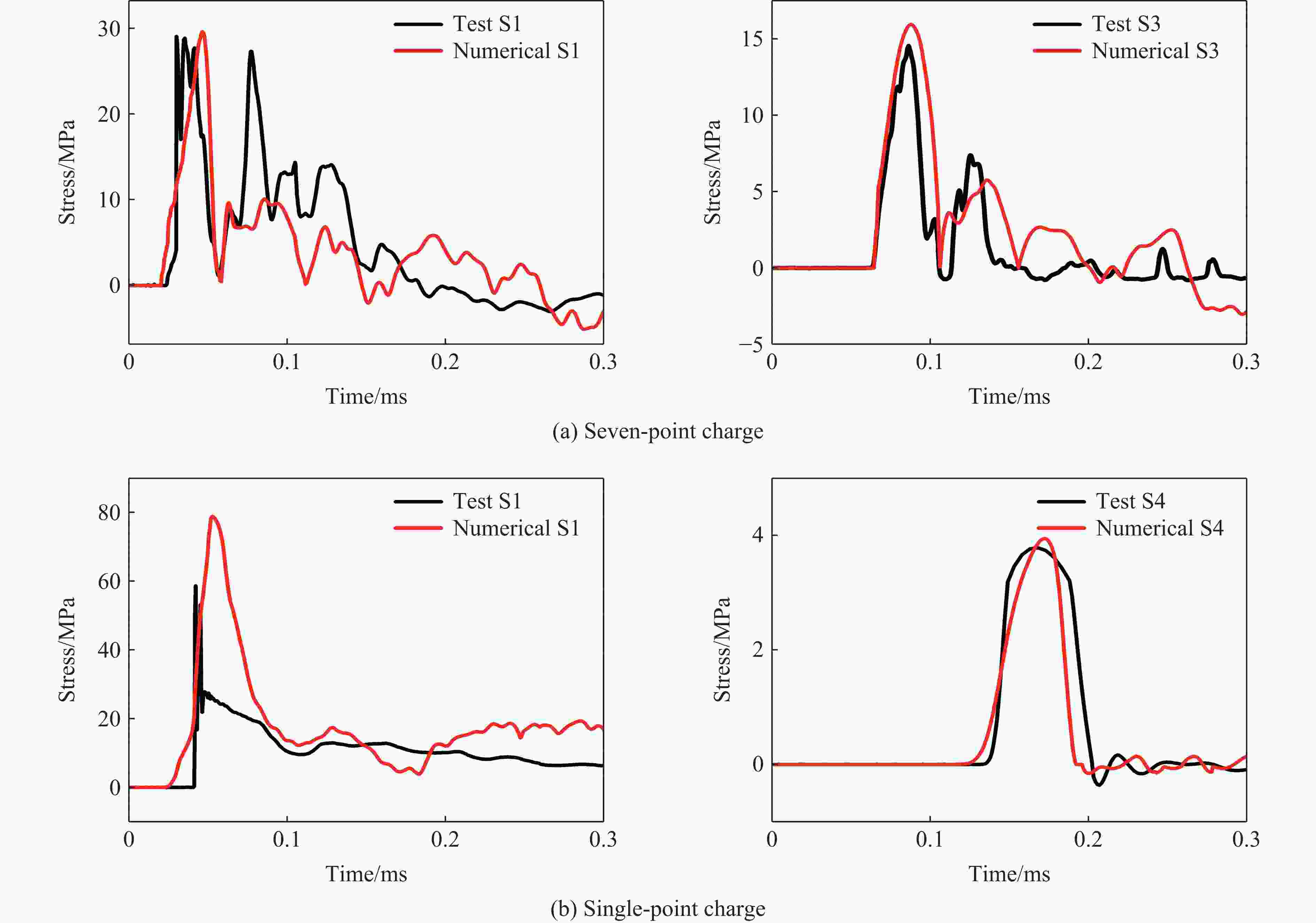
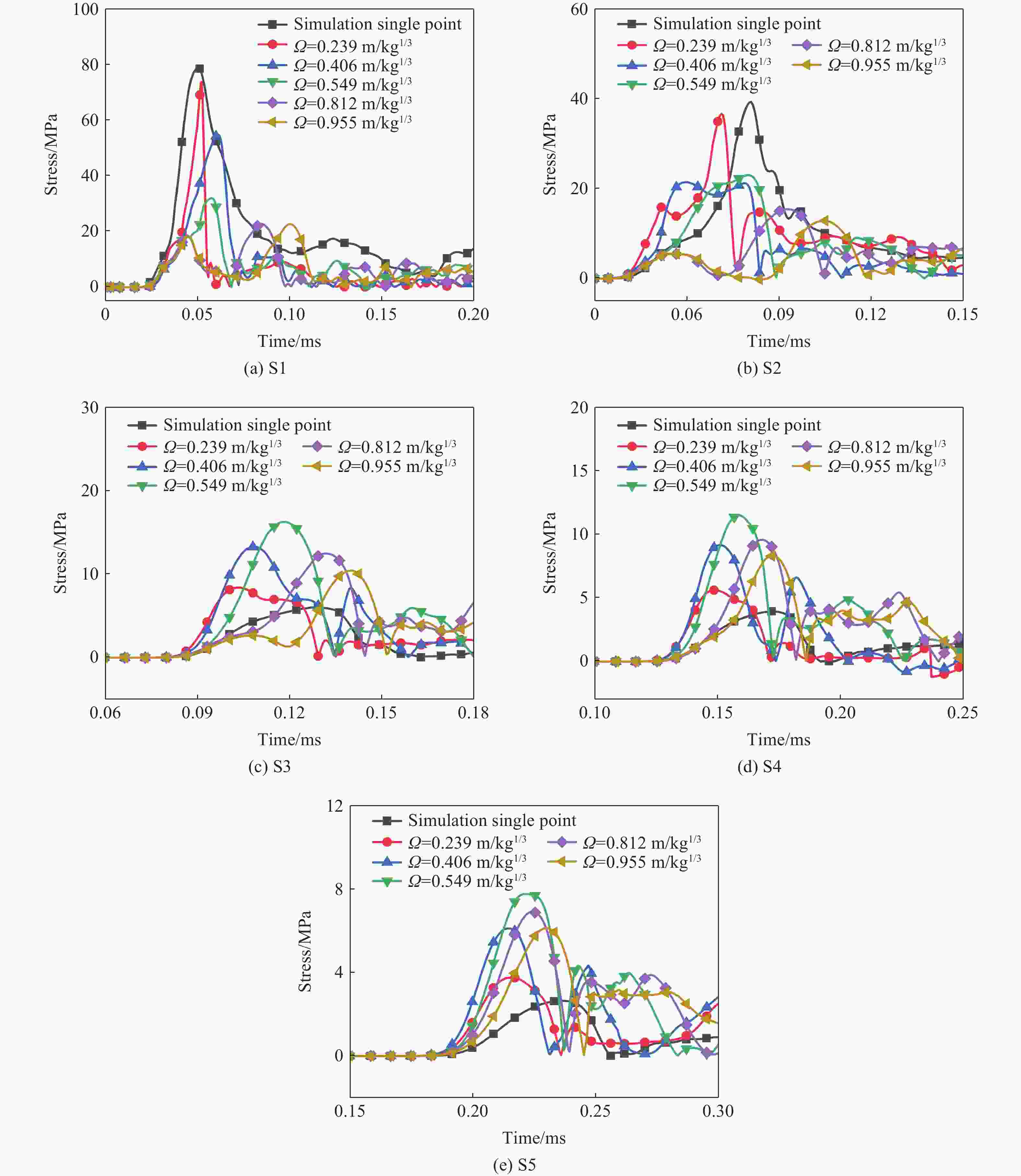
工况 测点 荷载峰值 模拟值/MPa 试验值/MPa 偏差/% 七点爆炸 S1 29.7 29.2 1.71 S3 16.0 14.6 9.59 单点爆炸 S1 79.2 59.0 34.23 S4 3.9 3.7 5.41 表 4 不同比例装药间距下拟合参数
Table 4. Fitting parameters for different proportions of charge spacing
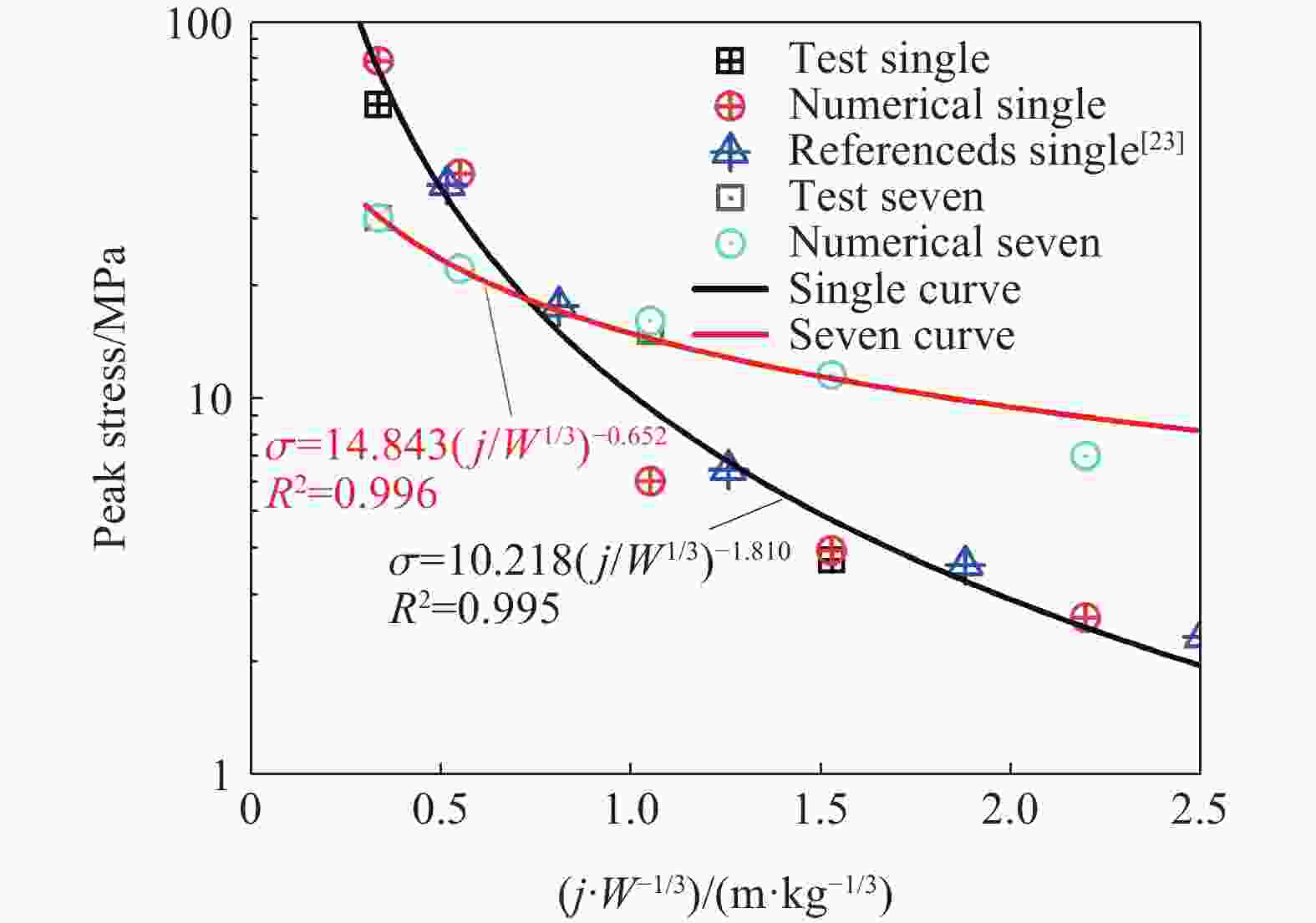
Ω/(m∙kg−1/3) K N Ω/(m∙kg−1/3) K N 0 10.218 −1.810 0.549 14.843 −0.652 0.239 11.826 −1.597 0.812 11.218 −0.627 0.406 13.851 −1.169 0.955 10.220 −0.629 表 5 控制因素和控制水平
Table 5. Control factors and level of control
控制因素 水平 1 2 3 (X1) Ω/(m∙kg−1/3) 0.406 0.549 0.812 (X2)Ψ/(m∙kg−1/3) 0 0.048 0.095 (X3)Γ/(m∙kg−1/3) 0 0.239 0.477 表 6 试验设计L9(34)矩阵
Table 6. Experimental design L9(34) matrix
方案 水平组合 方案 水平组合 Ω Ψ Γ Ω Ψ Γ 1 1 1 1 6 2 3 2 2 1 2 2 7 3 1 2 3 1 3 3 8 3 2 3 4 2 1 3 9 3 3 1 5 2 2 1 表 7 正交试验各序列区间值像
Table 7. Orthogonal test interval values for each sequence
工况 序列区间值像 x1(k) x2(k) x3(k) y1(k) y2(k) y3(k) 1 0.000 0.000 0.000 0.108 0.020 0.000 2 0.000 0.505 0.501 0.302 0.247 0.340 3 0.000 1.000 1.000 0.401 0.408 0.228 4 0.352 0.000 1.000 0.305 0.370 0.325 5 0.352 0.505 0.000 1.000 1.000 1.000 6 0.352 1.000 0.501 0.535 0.399 0.324 7 1.000 0.000 0.501 0.732 0.651 0.469 8 1.000 0.505 1.000 0.000 0.000 0.015 9 1.000 1.000 0.000 0.165 0.546 0.699 表 8 3种因素在3种水平下就S3峰值应力的关联度系数和关联度
Table 8. Correlation coefficients and correlation of peak S3 stress at different levels of different factors
工况 关联度系数 Ω Ψ Γ 1 0.925 0.925 0.925 2 0.773 0.844 0.847 3 0.713 0.618 0.618 4 0.986 0.771 0.580 5 0.598 0.665 0.486 6 0.860 0.680 1.000 7 0.796 0.567 0.823 8 0.486 0.660 0.486 9 0.533 0.533 0.875 关联度 0.741 0.696 0.738 表 9 多目标灰色关联度系数平均值
Table 9. Mean values of gray correlation coefficients for pairs of indicators
控制因素 平均灰色关联系数 1 2 3 Ω 0.816 0.829 0.601 Ψ 0.763 0.696 0.604 Γ 0.590 0.870 0.533 -
[1] 邓国强, 周早生, 郑全平. 钻地弹爆炸聚集效应研究现状及展望 [J]. 解放军理工大学学报(自然科学版), 2002, 3(3): 45–49. DOI: 10.3969/j.issn.1009-3443.2002.03.012.DENG G Q, ZHOU Z S, ZHENG Q P. Study status quo and development of aggregated effect of multiple earth penetrator bursts detonated simultaneously [J]. Journal of the PLA University of Science and Technology, 2002, 3(3): 45–49. DOI: 10.3969/j.issn.1009-3443.2002.03.012. [2] LENG Z D, SUN J S, LU W B, et al. Mechanism of the in-hole detonation wave interactions in dual initiation with electronic detonators in bench blasting operation [J]. Computers and Geotechnics, 2021, 129: 103873. DOI: 10.1016/j.compgeo.2020.103873. [3] LENG Z D, FAN Y, GAO Q D, et al. Evaluation and optimization of blasting approaches to reducing oversize boulders and toes in open-pit mine [J]. International Journal of Mining Science and Technology, 2020, 30(3): 373–380. DOI: 10.1016/j.ijmst.2020.03.010. [4] GAO Q D, LU W B, YAN P, et al. Effect of initiation location on distribution and utilization of explosion energy during rock blasting [J]. Bulletin of Engineering Geology and the Environment, 2019, 78(5): 3433–3447. DOI: 10.1007/s10064-018-1296-4. [5] PHILLIPS J S, BRATTON J L. ground shock analysis of the multiple burst experiments: ADA 088510 [R]. Springfield: NITS, 1978. [6] RUETENIK J R, HOBBS N P, SMILEY R F. Calculation of multiple burst interactions for six simultaneous explosions of 120 Ton ANFO charges: ADA 091978 [R]. Springfield: NITS, 1979. [7] HU H W, SONG P, GUO S F, et al. Shock wave and bubble characteristics of underwater array explosion of charges [J]. Defence Technology, 2022, 18(8): 1445–1453. DOI: 10.1016/J.DT.2021.05.020. [8] IZUMI K, ASO S, NISHIDA M. Experimental and computational studies focusing processes of shock waves reflected from parabolic reflectors [J]. Shock Waves, 1994, 3(3): 213–222. DOI: 10.1007/BF01414715. [9] KISHIGE H, TESHIMA K, NISHIDA M. Focusing of shock waves reflected from an axisymmetrically parabolic wall [C]. Proceedings of the 18th International Symposium on Shock Waves. Sendai: Springer, 1992: 341–345. DOI: 10.1007/978-3-642-77648-9_50. [10] QIU P, YUE Z W, ZHANG S C, et al. An in situ simultaneous measurement system combining photoelasticity and caustics methods for blast-induced dynamic fracture [J]. Review of Scientific Instruments, 2017, 88(11): 115113. DOI: 10.1063/1.4994811. [11] 李旭东, 刘凯欣, 张光升, 等. 冲击波在水泥砂浆板中的聚集效应 [J]. 清华大学学报(自然科学版), 2008, 48(8): 1272–1275. DOI: 10.16511/j.cnki.qhdxxb.2008.08.004.LI X D, LIU K X, ZHANG G S, et al. Focusing of shock waves in cement mortar plates [J]. Journal of Tsinghua University (Science & Technology), 2008, 48(8): 1272–1275. DOI: 10.16511/j.cnki.qhdxxb.2008.08.004. [12] LIN S J, WANG J X, LIU L T, et al. Research on damage effect of underwater multipoint synchronous explosion shock waves on air-backed clamped circular plate [J]. Ocean Engineering, 2021, 240: 109985. DOI: 10.1016/j.oceaneng.2021.109985. [13] KIM H D, KWEON Y H, SETOGUCHI T, et al. A study on the focusing phenomenon of a weak shock wave [J]. Proceedings of the Institution of Mechanical Engineers, Part C: Journal of Mechanical Engineering Science, 2003, 217(11): 1209–1220. DOI: 10.1243/095440603771665241. [14] LIANG S M, TSAI C J, WU L N. Efficient, robust second-order total variation diminishing scheme [J]. AIAA Journal, 1996, 34(1): 193–195. DOI: 10.2514/3.13042. [15] LIANG S M, WU L N, HSU R L. Numerical investigation of axisymmetric shock wave focusing over paraboloidal reflectors [J]. Shock Waves, 1999, 9(6): 367–379. DOI: 10.1007/S001930050167. [16] QIU S, ELIASSON V. Interaction and coalescence of multiple simultaneous and non-simultaneous blast waves [J]. Shock Waves, 2016, 26(3): 287–297. DOI: 10.1007/s00193-015-0567-2. [17] 刘玲, 袁俊明, 刘玉存, 等. 大型商场多点爆炸恐怖袭击事故数值模拟 [C]//中国化学会第29届学术年会摘要集: 第29分会: 公共安全化学. 北京: 中国化学会, 2014. [18] 邓国强, 龙汗, 周早生, 等. 钻地弹砂土中聚集爆炸地冲击试验与预测 [J]. 防护工程, 2001, 23(3): 24–28. [19] 叶海旺, 石文杰, 王二猛, 等. 金堆城露天矿生产爆破合理微差时间的探讨 [J]. 爆破, 2010, 27(1): 96–98. DOI: 10.3963/j.issn.1001-487X.2010.01.026.YE H W, SHI W J, WANG E M, et al. Research of reasonable delay intervals in Jinduicheng open-pit mine [J]. Blasting, 2010, 27(1): 96–98. DOI: 10.3963/j.issn.1001-487X.2010.01.026. [20] 顾强, 张世豪, 安晓红, 等. 基于灰色理论的两点爆炸起爆参数优化设计 [J]. 爆炸与冲击, 2015, 35(3): 359–365. DOI: 10.11883/1001-1455(2015)03-0359-07.GU Q, ZHANG S H, AN X H, et al. Optimization design for priming parameters of two-point explosion based on gray theory [J]. Explosion and Shock Waves, 2015, 35(3): 359–365. DOI: 10.11883/1001-1455(2015)03-0359-07. [21] Century Dynamics Inc. Ansys/Autodyn Version 11.0: user documentation [Z]. Pennsylvania, USA: Century Dynamics Inc., 2007: 89–112. [22] LEE E L, TARVER C M. Phenomenological model of shock initiation in heterogeneous explosives [J]. The Physics of Fluids, 1980, 23(12): 2362–2372. DOI: 10.1063/1.862940. [23] MU C M, ZHOU H, MA H F. Prediction method for ground shock parameters of explosion in concrete [J]. Construction and Building Materials, 2021, 291: 123372. DOI: 10.1016/J.CONBUILDMAT.2021.123372. [24] OSEI F B, DUKER A A, STEIN A. Bayesian structured additive regression modeling of epidemic data: application to cholera [J]. BMC Medical Research Methodology, 2012, 12(1): 118. DOI: 10.1186/1471-2288-12-118. [25] LI F G, LUAN P X. ARMA model for predicting the number of new outbreaks of Newcastle disease during the month [C]. //2011 IEEE International Conference on Computer Science and Automation Engineering. Shanghai, China: IEEE, 2011: 660–663. DOI: 10.1109/CSAE.2011.5952933. [26] KOROSTIL I A, PETERS G W, CORNEBISE J, et al. Adaptive Markov chain Monte Carlo forward projection for statistical analysis in epidemic modelling of human papillomavirus [J]. Statistics in Medicine, 2013, 32(11): 1917–1953. DOI: 10.1002/sim.5590. [27] ROBERTS M G, LAWSON J R, GEMMELL M A. Population dynamics in echinococcosis and cysticercosis: mathematical model of the life-cycles of Taenia hydatigena and T. ovis [J]. Parasitology, 1987, 94(1): 181–197. DOI: 10.1017/S0031182000053555. [28] HUANG J C. Application of grey system theory in telecare [J]. Computers in Biology and Medicine, 2011, 41(5): 302–306. DOI: 10.1016/j.compbiomed.2011.03.007. [29] LEE Y S, TONG L I. Forecasting energy consumption using a grey model improved by incorporating genetic programming [J]. Energy Conversion and Management, 2011, 52(1): 147–152. DOI: 10.1016/j.enconman.2010.06.053. [30] 王莹, 肖巍, 姚熊亮, 等. 水下爆炸冲击波载荷作用下冰层破碎特性及其影响因素 [J]. 爆炸与冲击, 2019, 39(7): 073103. DOI: 10.11883/bzycj-2018-0141.WANG Y, XIAO W, YAO X L, et al. Fragmentation of ice cover subjected to underwater explosion shock wave load and its influence factors [J]. Explosion and Shock Waves, 2019, 39(7): 073103. DOI: 10.11883/bzycj-2018-0141. [31] 吕锋. 灰色系统关联度之分辨系数的研究 [J]. 系统工程理论与实践, 1997, 17(6): 49–54. DOI: 10.3321/j.issn:1000-6788.1997.06.011.LÜ F. Research on the identification coefficient of relational grade for grey system [J]. Systems Engineering: Theory & Practice, 1997, 17(6): 49–54. DOI: 10.3321/j.issn:1000-6788.1997.06.011. -






 下载:
下载: